Difference between revisions of "Nephroid"
From Encyclopedia of Mathematics
(Start article: Nephroid) |
m (image) |
||
| (One intermediate revision by the same user not shown) | |||
| Line 10: | Line 10: | ||
The nephroid is the [[catacaustic]] of the [[cardioid]] with respect to a cusp, and of a circle with respect to a point at infinity; the [[evolute]] of a nephroid is another nephroid. | The nephroid is the [[catacaustic]] of the [[cardioid]] with respect to a cusp, and of a circle with respect to a point at infinity; the [[evolute]] of a nephroid is another nephroid. | ||
| + | |||
| + | [[File:Nephroide.svg|center|300px|Nephroid]] | ||
The '''nephroid of Freeth''' is the [[strophoid]] of a circle with respect to its centre and a point on the circumference. It has equation | The '''nephroid of Freeth''' is the [[strophoid]] of a circle with respect to its centre and a point on the circumference. It has equation | ||
| Line 17: | Line 19: | ||
====References==== | ====References==== | ||
| − | * J.D. Lawrence, | + | * J.D. Lawrence, "A catalog of special plane curves" , Dover (1972) {{ISBN|0-486-60288-5}} {{ZBL|0257.50002}} |
Latest revision as of 19:39, 14 November 2023
2020 Mathematics Subject Classification: Primary: 52A10 [MSN][ZBL]
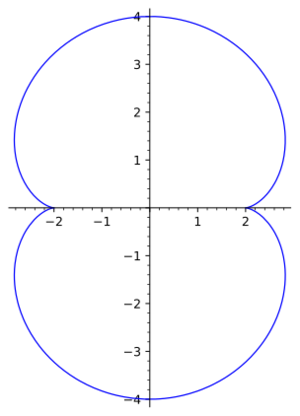
An epicycloid with parameter $m=2$; an algebraic plane curve with equation $$ x= 3r \cos\theta-r\cos\left[3\theta\right] \,, $$ $$ y= 3r \sin\theta-r\sin\left[3\theta\right] \ . $$
The nephroid is the catacaustic of the cardioid with respect to a cusp, and of a circle with respect to a point at infinity; the evolute of a nephroid is another nephroid.
The nephroid of Freeth is the strophoid of a circle with respect to its centre and a point on the circumference. It has equation $$ r = a(1 + 2\sin(\theta/2)) \ . $$
References
- J.D. Lawrence, "A catalog of special plane curves" , Dover (1972) ISBN 0-486-60288-5 Zbl 0257.50002
How to Cite This Entry:
Nephroid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Nephroid&oldid=51379
Nephroid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Nephroid&oldid=51379