Subtangent and subnormal

The directed segments $ QT $ and $ QN $ which are the projections on the $ x $- axis of the segments of the tangent line $ MT $ and the normal $ MN $ to a certain curve at a point $ M $( see Fig.).

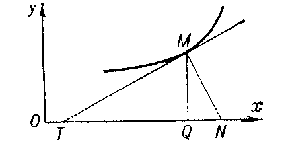
Figure: s091040a

If the curve is the graph of a function $ y = f( x) $, the values of the subtangent and subnormal are equal to

$$ QT = - \frac{f( x) }{f ^ { \prime } ( x) } ,\ \ ON = f( x) f ^ { \prime } ( x), $$

respectively, where $ x $ is the abscissa of the point $ M $. If the curve is given parametrically by

$$ x = \phi ( t),\ y = \psi ( t), $$

then

$$ QT = - \frac{\psi ( t) \phi ^ \prime ( t) }{\psi ^ \prime ( t) } ,\ \ QN = \frac{\psi ( t) \psi ^ \prime ( t) }{\psi ^ \prime ( t) } , $$

where $ t $ is the value of the parameter defining the point $ M $ on the curve.

References

[a1]	M. Berger, "Geometry" , II , Springer (1989)
[a2]	F. Gomes Teixeira, "Traité des courbes" , 1–3 , Chelsea, reprint (1971)
[a3]	H. Lamb, "Infinitesimal calculus" , Cambridge (1924) pp. 118

🛠️ This page contains images that should be replaced by better images in the SVG file format. 🛠️