|
|
| Line 1: |
Line 1: |
| − | An object of study in classical homotopy theory. The calculation of the homotopy groups of the spheres, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866501.png" />, was considered in its time (especially in the 1950's) as one of the central problems in topology. Topologists hoped that these groups could be successfully calculated completely, and that they would help to solve other classification problems in homotopy. These hopes were not to be realized in full: The homotopy groups of the spheres could only be calculated partially, and with the development of [[Generalized cohomology theories|generalized cohomology theories]], the problem of their calculation became less pressing. However, all the information that had been compiled on these groups was not wasted, as it found an unexpected use in differential topology (the classification of differential structures on spheres and multi-dimensional knots). | + | <!-- |
| | + | s0866501.png |
| | + | $#A+1 = 310 n = 4 |
| | + | $#C+1 = 310 : ~/encyclopedia/old_files/data/S086/S.0806650 Spheres, homotopy groups of the |
| | + | Automatically converted into TeX, above some diagnostics. |
| | + | Please remove this comment and the {{TEX|auto}} line below, |
| | + | if TeX found to be correct. |
| | + | --> |
| | + | |
| | + | {{TEX|auto}} |
| | + | {{TEX|done}} |
| | + | |
| | + | An object of study in classical homotopy theory. The calculation of the homotopy groups of the spheres, $ \pi _ {i} ( S ^ {n} ) $, |
| | + | was considered in its time (especially in the 1950's) as one of the central problems in topology. Topologists hoped that these groups could be successfully calculated completely, and that they would help to solve other classification problems in homotopy. These hopes were not to be realized in full: The homotopy groups of the spheres could only be calculated partially, and with the development of [[Generalized cohomology theories|generalized cohomology theories]], the problem of their calculation became less pressing. However, all the information that had been compiled on these groups was not wasted, as it found an unexpected use in differential topology (the classification of differential structures on spheres and multi-dimensional knots). |
| | | | |
| | ==I. General theory.== | | ==I. General theory.== |
| | | | |
| | + | 1) If $ i < n $ |
| | + | or $ i > n= 1 $, |
| | + | then $ \pi _ {i} ( S ^ {n} ) = 0 $. |
| | | | |
| − | 1) If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866502.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866503.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866504.png" />.
| + | 2) $ \pi _ {n} ( S ^ {n} ) = \mathbf Z $( |
| | + | the Brouwer–Hopf theorem); this isomorphism relates an element of the group $ \pi _ {n} ( S ^ {n} ) $ |
| | + | to the degree of the mapping $ S ^ {n} \rightarrow S ^ {n} $ |
| | + | representing it. |
| | | | |
| − | 2) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866505.png" /> (the Brouwer–Hopf theorem); this isomorphism relates an element of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866506.png" /> to the degree of the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866507.png" /> representing it.
| + | 3) The groups $ \pi _ {4m-} 1 ( S ^ {2m} ) $ |
| | + | have rank 1; the other groups $ \pi _ {i} ( S ^ {n} ) $ |
| | + | with $ i \neq n $ |
| | + | are finite. |
| | | | |
| − | 3) The groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866508.png" /> have rank 1; the other groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s0866509.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665010.png" /> are finite.
| + | The [[Suspension|suspension]] homomorphism |
| | | | |
| − | The [[Suspension|suspension]] homomorphism
| + | $$ |
| | + | E : \pi _ {i} ( S ^ {n} ) \rightarrow \pi _ {i+} 1 ( S ^ {n+} 1 ) |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665011.png" /></td> </tr></table>
| + | relates an element of the group $ \pi _ {i} ( S ^ {n} ) $, |
| | + | represented by the mapping $ f: S ^ {i} \rightarrow S ^ {n} $, |
| | + | to the class of the mapping $ Ef : S ^ {i+} 1 \rightarrow S ^ {n+} 1 $, |
| | + | defined by the formula |
| | | | |
| − | relates an element of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665012.png" />, represented by the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665013.png" />, to the class of the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665014.png" />, defined by the formula
| + | $$ |
| | + | Ef( \sqrt {1 - x ^ {2} } \mathbf x , x) = \left \{ |
| | + | \begin{array}{ll} |
| | + | ( \sqrt {1- x ^ {2} } f( \mathbf x ), x), & | x | \leq 1, \\ |
| | + | ( \mathbf 0 , x), & | x | = 1, \\ |
| | + | \end{array} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665015.png" /></td> </tr></table>
| + | \right .$$ |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665016.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665017.png" />. | + | where $ \mathbf x \in S ^ {i} $, |
| | + | $ x \in \mathbf R $. |
| | | | |
| − | 4) The homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665018.png" /> is an isomorphism when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665019.png" />, and an epimorphism when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665020.png" />. | + | 4) The homomorphism $ E $ |
| | + | is an isomorphism when $ i > 2n- 1 $, |
| | + | and an epimorphism when $ i \geq 2n- 1 $. |
| | | | |
| − | Thus, for every <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665021.png" /> the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665022.png" /> can be made terms of a sequence | + | Thus, for every $ k $ |
| | + | the groups $ \pi _ {n+} k ( S ^ {n} ) $ |
| | + | can be made terms of a sequence |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665023.png" /></td> </tr></table>
| + | $$ |
| | + | \pi _ {1+} k ( S ^ {1} ) \ |
| | + | \rightarrow ^ { E } \ |
| | + | \pi _ {2+} k ( S ^ {2} ) \ |
| | + | \rightarrow ^ { E } \ |
| | + | \pi _ {3+} k ( S ^ {3} ) \ |
| | + | \rightarrow ^ { E } \dots , |
| | + | $$ |
| | | | |
| − | at the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665024.png" />-nd term of which stabilization begins; the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665025.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665026.png" /> are called the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665028.png" />-th stable homotopy groups of the spheres, and are denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665029.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665030.png" /> when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665031.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665032.png" />. | + | at the $ ( k+ 2) $- |
| | + | nd term of which stabilization begins; the groups $ \pi _ {n+} k ( S ^ {n} ) $ |
| | + | with $ n \geq k+ 2 $ |
| | + | are called the $ k $- |
| | + | th stable homotopy groups of the spheres, and are denoted by $ \pi _ {k} ^ {s} $. |
| | + | Then $ \pi _ {k} ^ {s} = 0 $ |
| | + | when $ k < 0 $ |
| | + | and $ \pi _ {0} ^ {s} = \mathbf Z $. |
| | | | |
| | As for the homotopy groups (cf. [[Homotopy group|Homotopy group]]) of any topological space, the Whitehead product is defined on the homotopy groups of the spheres: | | As for the homotopy groups (cf. [[Homotopy group|Homotopy group]]) of any topological space, the Whitehead product is defined on the homotopy groups of the spheres: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665033.png" /></td> </tr></table>
| + | $$ |
| | + | \pi _ {i} ( S ^ {n} ) \times \pi _ {j} ( S ^ {n} ) \rightarrow \pi _ {i+} j- 1 ( S ^ {n} ) ,\ \ |
| | + | ( \alpha , \beta ) \rightarrow [ \alpha , \beta ]. |
| | + | $$ |
| | | | |
| | To its usual properties (distributivity, skew commutativity and the Jacobi identity) is added | | To its usual properties (distributivity, skew commutativity and the Jacobi identity) is added |
| | | | |
| − | 5) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665034.png" />. | + | 5) $ E[ \alpha , \beta ] = 0 $. |
| | | | |
| | The Whitehead product enables one to make the following refinement to 4): | | The Whitehead product enables one to make the following refinement to 4): |
| | | | |
| − | 6) The kernel of the epimorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665035.png" /> is generated by the class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665036.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665037.png" /> is a canonical generator of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665038.png" /> (representable by the identity mapping). | + | 6) The kernel of the epimorphism $ E : \pi _ {2n-} 1 ( S ^ {n} ) \rightarrow \pi _ {2n} ( S ^ {n+} 1 ) $ |
| | + | is generated by the class $ [ i _ {n} , i _ {n} ] $, |
| | + | where $ i _ {n} $ |
| | + | is a canonical generator of the group $ \pi _ {n} ( S ^ {n} ) $( |
| | + | representable by the identity mapping). |
| | | | |
| − | Closely linked to the Whitehead product is the [[Hopf invariant|Hopf invariant]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665039.png" />, defined for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665040.png" />. Thus, the element of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665041.png" /> which can be represented by the Hopf mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665042.png" /> that operates according to the formula <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665043.png" /> (in which <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665044.png" /> is interpreted as the unit sphere in the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665045.png" />, while <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665046.png" /> is interpreted as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665047.png" />) has Hopf invariant equal to 1. | + | Closely linked to the Whitehead product is the [[Hopf invariant|Hopf invariant]] $ H( \alpha ) $, |
| | + | defined for $ \alpha \in \pi _ {4m-} 1 ( S ^ {2m} ) $. |
| | + | Thus, the element of the group $ \pi _ {3} ( S ^ {2} ) $ |
| | + | which can be represented by the Hopf mapping $ h: S ^ {3} \rightarrow S ^ {2} $ |
| | + | that operates according to the formula $ h( z _ {1} , z _ {2} ) = z _ {1} : z _ {2} $( |
| | + | in which $ S ^ {3} $ |
| | + | is interpreted as the unit sphere in the space $ \mathbf C ^ {2} $, |
| | + | while $ S ^ {2} $ |
| | + | is interpreted as $ \mathbf C P ^ {1} $) |
| | + | has Hopf invariant equal to 1. |
| | | | |
| − | 7) The mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665048.png" /> is an isomorphism. | + | 7) The mapping $ H: \pi _ {3} ( S ^ {2} ) \rightarrow \mathbf Z $ |
| | + | is an isomorphism. |
| | | | |
| − | 8) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665049.png" />. | + | 8) $ H([ i _ {2m} , i _ {2m} ]) = 2 $. |
| | | | |
| − | A consequence of 8) is that the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665050.png" /> are infinite, a fact already stated in 3). | + | A consequence of 8) is that the groups $ \pi _ {4m-} 1 ( S ^ {2m} ) $ |
| | + | are infinite, a fact already stated in 3). |
| | | | |
| − | 9) When <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665051.png" />, in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665052.png" /> there are no elements of odd Hopf invariant (as was known long before this theorem was proved, its assertion is equivalent to the following Frobenius conjecture: when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665053.png" />, then in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665054.png" /> there is no bilinear multiplication with single-valued division on non-zero elements). | + | 9) When $ m \neq 1, 2, 4 $, |
| | + | in $ \pi _ {4m-} 1 ( S ^ {2m} ) $ |
| | + | there are no elements of odd Hopf invariant (as was known long before this theorem was proved, its assertion is equivalent to the following Frobenius conjecture: when $ l \neq 1, 2, 4, 8 $, |
| | + | then in $ \mathbf R ^ {l} $ |
| | + | there is no bilinear multiplication with single-valued division on non-zero elements). |
| | | | |
| | The composition product | | The composition product |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665055.png" /></td> </tr></table>
| + | $$ |
| | + | \pi _ {i} ( S ^ {j} ) \times \pi _ {j} ( S ^ {n} ) \rightarrow \pi _ {i} ( S ^ {n} ) ,\ \ |
| | + | ( \beta , \alpha ) \rightarrow \alpha \circ \beta , |
| | + | $$ |
| | | | |
| | which can be defined by juxtaposition of mappings, is unique to the spheres. | | which can be defined by juxtaposition of mappings, is unique to the spheres. |
| | | | |
| − | 10) For any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665056.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665057.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665058.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665059.png" />, the following hold: | + | 10) For any $ \alpha , \alpha _ {1} , \alpha _ {2} \in \pi _ {j} ( S ^ {n} ) $, |
| | + | $ \beta , \beta _ {1} , \beta _ {2} \in \pi _ {i} ( S ^ {j} ) $, |
| | + | $ \delta \in \pi _ {i-} 1 ( S ^ {j-} 1 ) $, |
| | + | $ \gamma \in \pi _ {k} ( S ^ {j} ) $, |
| | + | the following hold: |
| | | | |
| − | a) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665060.png" />; | + | a) $ ( \alpha \circ \beta ) \circ \gamma = \alpha \circ ( \beta \circ \gamma ) $; |
| | | | |
| − | b) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665061.png" />; | + | b) $ \alpha \circ ( \beta _ {1} + \beta _ {2} ) = \alpha \circ \beta _ {1} + \alpha \circ \beta _ {2} $; |
| | | | |
| − | c) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665062.png" />; | + | c) $ ( \alpha _ {1} + \alpha _ {2} ) \circ E \delta = \alpha _ {1} \circ E \delta + \alpha _ {2} \circ E \delta $; |
| | | | |
| − | d) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665063.png" />. | + | d) $ E( \alpha \circ \beta ) = E \alpha \circ E \beta $. |
| | | | |
| − | The "left law of distributivity" , <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665064.png" />, generally speaking, does not hold. Assertion d) enables one to define a stable composition product | + | The "left law of distributivity" , $ ( \alpha _ {1} + \alpha _ {2} ) \circ \beta = \alpha _ {1} \circ \beta + \alpha _ {2} \circ \beta $, |
| | + | generally speaking, does not hold. Assertion d) enables one to define a stable composition product |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665065.png" /></td> </tr></table>
| + | $$ |
| | + | \pi _ {q} ^ {s} \times \pi _ {r} ^ {s} \rightarrow \pi _ {q+} r ^ {s} ,\ \ |
| | + | ( \beta , \alpha ) \rightarrow \alpha \circ \beta . |
| | + | $$ |
| | | | |
| − | 11) For any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665066.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665067.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665068.png" />, assertions a) and b) in 10) hold, as do: | + | 11) For any $ \alpha , \alpha _ {1} , \alpha _ {2} \in \pi _ {r} ^ {s} $, |
| | + | $ \beta , \beta _ {1} , \beta _ {2} \in \pi _ {q} ^ {s} $, |
| | + | $ \gamma \in \pi _ {p} ^ {s} $, |
| | + | assertions a) and b) in 10) hold, as do: |
| | | | |
| − | c') <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665069.png" />, | + | c') $ ( \alpha _ {1} + \alpha _ {2} ) \circ \beta = \alpha _ {1} \circ \beta + \alpha _ {2} \circ \beta $, |
| | | | |
| − | d') <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665070.png" />. | + | d') $ \alpha \circ \beta = (- 1) ^ {qr} \beta \circ \alpha $. |
| | | | |
| | ==II. Methods of calculation.== | | ==II. Methods of calculation.== |
| − | The geometric method of L.S. Pontryagin (see [[#References|[1]]]), proposed in the mid-1930s, is based on the following definition. A smooth <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665071.png" />-dimensional compact manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665072.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665073.png" /> is said to be framed if a smooth field of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665074.png" />-frames transversal to it is defined on the manifold; the field itself is said to be a framing. Two framed manifolds <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665075.png" />, without boundary, are said to be cobordant if there exists a framed manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665076.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665077.png" /> for which the restriction of the framing onto <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665078.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665079.png" /> is contained in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665080.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665081.png" />, and, given a natural identification of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665082.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665083.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665084.png" />, is turned into the given framing of the manifolds <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665085.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665086.png" />. The set of classes of cobordant framed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665087.png" />-dimensional manifolds without boundary in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665088.png" /> is denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665089.png" />. | + | The geometric method of L.S. Pontryagin (see [[#References|[1]]]), proposed in the mid-1930s, is based on the following definition. A smooth $ m $- |
| | + | dimensional compact manifold $ X $ |
| | + | in $ \mathbf R ^ {i} $ |
| | + | is said to be framed if a smooth field of $ ( i- m) $- |
| | + | frames transversal to it is defined on the manifold; the field itself is said to be a framing. Two framed manifolds $ X _ {0} , X _ {1} \subset \mathbf R ^ {i} $, |
| | + | without boundary, are said to be cobordant if there exists a framed manifold $ Y \subset \mathbf R ^ {i} \times [ 0, 1] \subset \mathbf R ^ {i+} 1 $ |
| | + | with $ \partial Y = ( X _ {0} \times 0) \cup ( X _ {1} \times 1) $ |
| | + | for which the restriction of the framing onto $ X _ {0} \times 0 $ |
| | + | and $ X _ {1} \times 1 $ |
| | + | is contained in $ \mathbf R ^ {i} \times 0 $ |
| | + | and $ \mathbf R ^ {i} \times 1 $, |
| | + | and, given a natural identification of $ \mathbf R ^ {i} \times 0 $ |
| | + | and $ \mathbf R ^ {i} \times 1 $ |
| | + | with $ \mathbf R ^ {i} $, |
| | + | is turned into the given framing of the manifolds $ X _ {0} $ |
| | + | and $ X _ {1} $. |
| | + | The set of classes of cobordant framed $ m $- |
| | + | dimensional manifolds without boundary in $ \mathbf R ^ {i} $ |
| | + | is denoted by $ \Omega ^ {m} ( i) $. |
| | | | |
| − | 1) There is a one-to-one correspondence between <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665090.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665091.png" />. | + | 1) There is a one-to-one correspondence between $ \pi _ {i} ( S ^ {n} ) $ |
| | + | and $ \Omega ^ {i-} n ( i) $. |
| | | | |
| − | This method gives good results for small <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665092.png" />. It also makes it possible to prove certain of the theorems in section I and provides a variety of geometric information on manifolds of small dimensions. | + | This method gives good results for small $ i- n $. |
| | + | It also makes it possible to prove certain of the theorems in section I and provides a variety of geometric information on manifolds of small dimensions. |
| | | | |
| | Another group of methods consists of elementary algebraic methods comprising the use of homotopy sequences of various fibre bundles, properties of the Whitehead product, the composition product, and the corresponding higher product (Toda brackets, see [[#References|[3]]]), as well as the following theorem of James. | | Another group of methods consists of elementary algebraic methods comprising the use of homotopy sequences of various fibre bundles, properties of the Whitehead product, the composition product, and the corresponding higher product (Toda brackets, see [[#References|[3]]]), as well as the following theorem of James. |
| Line 87: |
Line 194: |
| | 2) There is a sequence of groups and homomorphisms | | 2) There is a sequence of groups and homomorphisms |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665093.png" /></td> </tr></table>
| + | $$ |
| | + | {} \dots \rightarrow \pi _ {i} ( S ^ {n} ) \rightarrow ^ { E } \ |
| | + | \pi _ {i+} 1 ( S ^ {n+} 1 ) \rightarrow ^ { H } \pi _ {i+} 1 ( S ^ {2n+} 1 ) \rightarrow ^ { P } \ |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665094.png" /></td> </tr></table>
| + | $$ |
| | + | \rightarrow ^ { P } \pi _ {i-} 1 ( S ^ {n} ) \rightarrow \dots , |
| | + | $$ |
| | | | |
| − | which is exact for odd <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665095.png" /> and for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665096.png" /> (in this sequence, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665097.png" /> is a generalization of the Hopf invariant). | + | which is exact for odd $ n $ |
| | + | and for $ i < 3n- 1 $( |
| | + | in this sequence, $ H $ |
| | + | is a generalization of the Hopf invariant). |
| | | | |
| − | Elementary algebraic methods prove to be reasonable effective: It is possible to calculate the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665098.png" /> when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s08665099.png" /> almost without having to resort to other methods. | + | Elementary algebraic methods prove to be reasonable effective: It is possible to calculate the groups $ \pi _ {i} ( S ^ {n} ) $ |
| | + | when $ i- n \leq 13 $ |
| | + | almost without having to resort to other methods. |
| | | | |
| − | There is also the method of killing spaces (see [[#References|[5]]] and [[Killing space|Killing space]]). This method is suitable for the calculation of the homotopy groups of any space. It is based on the construction, using a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650100.png" />, of a sequence of killing spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650101.png" /> with the following property: | + | There is also the method of killing spaces (see [[#References|[5]]] and [[Killing space|Killing space]]). This method is suitable for the calculation of the homotopy groups of any space. It is based on the construction, using a space $ X $, |
| | + | of a sequence of killing spaces $ X \mid _ {k} $ |
| | + | with the following property: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650102.png" /></td> </tr></table>
| + | $$ |
| | + | \pi _ {i} ( X \mid _ {k} ) = \left \{ |
| | | | |
| − | Thus, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650103.png" /> and the problem of calculating the homotopy groups reduces to the problem of calculating the homology groups (and the cohomology groups) of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650104.png" />. These homology groups are found by induction, using spectral sequences (cf. [[Spectral sequence|Spectral sequence]]) of fibre bundles: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650105.png" /> is broken down with fibre <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650106.png" /> over the [[Eilenberg–MacLane space|Eilenberg–MacLane space]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650107.png" />. The calculation does not have an automatic character: In order to progress, it is necessary to know as much as possible about the cohomology groups of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650108.png" />, including the action in them of the primary and the higher cohomology operations (cf. [[Cohomology operation|Cohomology operation]]).
| + | \begin{array}{ll} |
| | + | \pi _ {i} ( X), & i \geq k, \\ |
| | + | 0, & i < k. \\ |
| | + | \end{array} |
| | | | |
| − | A more suitable apparatus for calculating the stable homotopy groups of the spheres is the Adams spectral sequence. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650109.png" /> be a prime number, and let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650110.png" /> be the [[Steenrod algebra|Steenrod algebra]] of stable cohomology operations on the cohomology spaces with coefficients in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650111.png" />.
| + | \right .$$ |
| | | | |
| − | 3) There exists a spectral sequence the first term of which coincides with the cohomology groups of the Steenrod algebra (i.e. with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650112.png" />), while the limit term is related to the stable homotopy groups of the spheres factored by the torsion of order relatively prime to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650113.png" />. | + | Thus, $ \pi _ {i} ( X) = \pi _ {i} ( X | _ {i} ) = H _ {i} ( X | _ {i} ) $ |
| | + | and the problem of calculating the homotopy groups reduces to the problem of calculating the homology groups (and the cohomology groups) of $ X \mid _ {i} $. |
| | + | These homology groups are found by induction, using spectral sequences (cf. [[Spectral sequence|Spectral sequence]]) of fibre bundles: $ X \mid _ {k} $ |
| | + | is broken down with fibre $ X \mid _ {k+} 1 $ |
| | + | over the [[Eilenberg–MacLane space|Eilenberg–MacLane space]] $ K( \pi _ {k} ( X), k) $. |
| | + | The calculation does not have an automatic character: In order to progress, it is necessary to know as much as possible about the cohomology groups of $ X $, |
| | + | including the action in them of the primary and the higher cohomology operations (cf. [[Cohomology operation|Cohomology operation]]). |
| | + | |
| | + | A more suitable apparatus for calculating the stable homotopy groups of the spheres is the Adams spectral sequence. Let $ p $ |
| | + | be a prime number, and let $ A _ {(} p) $ |
| | + | be the [[Steenrod algebra|Steenrod algebra]] of stable cohomology operations on the cohomology spaces with coefficients in $ Z _ {p} $. |
| | + | |
| | + | 3) There exists a spectral sequence the first term of which coincides with the cohomology groups of the Steenrod algebra (i.e. with $ \mathop{\rm Ext} _ {A ^ {(} p) } ( \mathbf Z _ {p} , \mathbf Z _ {p} ) $), |
| | + | while the limit term is related to the stable homotopy groups of the spheres factored by the torsion of order relatively prime to $ p $. |
| | | | |
| | The Adams spectral sequence permits one to achieve considerable progress in the calculation of the stable homotopy groups of the spheres. An analogous spectral sequence exists for the calculation of the stable homotopy groups of any space. There is also an unstable analogue of the Adams spectral sequence (see [[#References|[4]]]). | | The Adams spectral sequence permits one to achieve considerable progress in the calculation of the stable homotopy groups of the spheres. An analogous spectral sequence exists for the calculation of the stable homotopy groups of any space. There is also an unstable analogue of the Adams spectral sequence (see [[#References|[4]]]). |
| | | | |
| − | More modern methods of calculating the homotopy groups of the spheres are based on [[Generalized cohomology theories|generalized cohomology theories]]. One of these involves the use of the Adams' <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650115.png" />-invariant, which is closely linked to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650116.png" />-theory. In constructing this <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650117.png" />-invariant, a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650118.png" /> representing a class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650119.png" /> is fixed, and the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650120.png" />, obtained by attaching an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650121.png" />-dimensional cell to the sphere <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650122.png" /> through the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650123.png" />, is examined. It turns out that | + | More modern methods of calculating the homotopy groups of the spheres are based on [[Generalized cohomology theories|generalized cohomology theories]]. One of these involves the use of the Adams' $ e $- |
| | + | invariant, which is closely linked to $ K $- |
| | + | theory. In constructing this $ e $- |
| | + | invariant, a mapping $ f: S ^ {i} \rightarrow S ^ {n} $ |
| | + | representing a class $ \alpha \in \pi _ {i} ( S ^ {n} ) $ |
| | + | is fixed, and the space $ X _ \alpha = S ^ {n} \cup _ {f} D ^ {i+} 1 $, |
| | + | obtained by attaching an $ ( i+ 1) $- |
| | + | dimensional cell to the sphere $ S ^ {n} $ |
| | + | through the mapping $ f $, |
| | + | is examined. It turns out that |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650124.png" /></td> </tr></table>
| + | $$ |
| | + | H _ {n} ( X _ \alpha ; \mathbf Z ) \cong \ |
| | + | H _ {i+} 1 ( X _ \alpha ; \mathbf Z ) |
| | + | \cong \mathbf Z . |
| | + | $$ |
| | | | |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650125.png" /> be the canonical generators of these groups. There exists a complex vector bundle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650126.png" /> over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650127.png" /> with [[Chern character|Chern character]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650128.png" /> satisfying the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650129.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650130.png" /> is a rational number, the residue of which modulo 1 does not depend on the choice of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650131.png" />. This residue is the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650132.png" />-invariant <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650133.png" /> of the class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650134.png" />. The function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650135.png" /> is a homomorphism | + | Let $ \mu , \nu $ |
| | + | be the canonical generators of these groups. There exists a complex vector bundle $ \xi $ |
| | + | over $ X _ \alpha $ |
| | + | with [[Chern character|Chern character]] $ \mathop{\rm ch} \xi $ |
| | + | satisfying the relation $ \langle \mathop{\rm ch} \xi , \mu \rangle = 1 $. |
| | + | Then $ \langle \mathop{\rm ch} \xi , \nu \rangle $ |
| | + | is a rational number, the residue of which modulo 1 does not depend on the choice of $ \xi $. |
| | + | This residue is the $ e $- |
| | + | invariant $ e( \alpha ) $ |
| | + | of the class $ \alpha $. |
| | + | The function $ e $ |
| | + | is a homomorphism |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650136.png" /></td> </tr></table>
| + | $$ |
| | + | e: \pi _ {i} ( S ^ {n} ) \rightarrow \mathbf Q / \mathbf Z , |
| | + | $$ |
| | | | |
| | whose image can be determined (see ). | | whose image can be determined (see ). |
| Line 121: |
Line 283: |
| | ==III. Results of calculations.== | | ==III. Results of calculations.== |
| | | | |
| − | | + | 1) The groups $ \pi _ {i} ( S ^ {n} ) $ |
| − | 1) The groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650137.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650138.png" /> are isomorphic to the groups from the following table:''''''<table border="0" cellpadding="0" cellspacing="0" style="background-color:black;"> <tr><td> <table border="0" cellspacing="1" cellpadding="4" style="background-color:black;"> <tbody> <tr> <td colname="1" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650139.png" /></td> <td colname="2" style="background-color:white;" colspan="1">2</td> <td colname="3" style="background-color:white;" colspan="1">3</td> <td colname="4" style="background-color:white;" colspan="1">4</td> <td colname="5" style="background-color:white;" colspan="1">5</td> <td colname="6" style="background-color:white;" colspan="1">6</td> <td colname="7" style="background-color:white;" colspan="1">7</td> <td colname="8" style="background-color:white;" colspan="1">8</td> <td colname="9" style="background-color:white;" colspan="1">9</td> <td colname="10" style="background-color:white;" colspan="1">10</td> <td colname="11" style="background-color:white;" colspan="1">11</td> <td colname="12" style="background-color:white;" colspan="1">12</td> <td colname="13" style="background-color:white;" colspan="1">stable</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">1</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650140.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650141.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650142.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650143.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650144.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650145.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650146.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650147.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650148.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650149.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650150.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650151.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">2</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650152.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650153.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650154.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650155.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650156.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650157.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650158.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650159.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650160.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650161.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650162.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650163.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">3</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650164.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650165.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650166.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650167.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650168.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650169.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650170.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650171.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650172.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650173.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650174.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650175.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">4</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650176.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650177.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650178.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650179.png" /></td> <td colname="6" style="background-color:white;" colspan="1">0</td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650180.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650181.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650182.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650183.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650184.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650185.png" /></td> <td colname="13" style="background-color:white;" colspan="1">0</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">5</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650186.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650187.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650188.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650189.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650190.png" /></td> <td colname="7" style="background-color:white;" colspan="1">0</td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650191.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650192.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650193.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650194.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650195.png" /></td> <td colname="13" style="background-color:white;" colspan="1">0</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">6</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650196.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650197.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650198.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650199.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650200.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650201.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650202.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650203.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650204.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650205.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650206.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650207.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">7</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650208.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650209.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650210.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650211.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650212.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650213.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650214.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650215.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650216.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650217.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650218.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650219.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">8</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650220.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650221.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650222.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650223.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650224.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650225.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650226.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650227.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650228.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650229.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650230.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650231.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">9</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650232.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650233.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650234.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650235.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650236.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650237.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650238.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650239.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650240.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650241.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650242.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650243.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">10</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650244.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650245.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650246.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650247.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650248.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650249.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650250.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650251.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650252.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650253.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650254.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650255.png" /></td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">11</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650256.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650257.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650258.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650259.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650260.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650261.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650262.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650263.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650264.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650265.png" /></td> <td colname="12" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650266.png" /></td> <td colname="13" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650267.png" /></td> </tr> </tbody> </table> | + | with $ i- n \leq 2 $ |
| | + | are isomorphic to the groups from the following table:<table border="0" cellpadding="0" cellspacing="0" style="background-color:black;"> <tr><td> <table border="0" cellspacing="1" cellpadding="4" style="background-color:black;"> <tbody> <tr> <td colname="1" style="background-color:white;" colspan="1"> $ \begin{array}{c} |
| | + | n \\ |
| | + | i- n |
| | + | \end{array} |
| | + | $ |
| | + | </td> <td colname="2" style="background-color:white;" colspan="1">2</td> <td colname="3" style="background-color:white;" colspan="1">3</td> <td colname="4" style="background-color:white;" colspan="1">4</td> <td colname="5" style="background-color:white;" colspan="1">5</td> <td colname="6" style="background-color:white;" colspan="1">6</td> <td colname="7" style="background-color:white;" colspan="1">7</td> <td colname="8" style="background-color:white;" colspan="1">8</td> <td colname="9" style="background-color:white;" colspan="1">9</td> <td colname="10" style="background-color:white;" colspan="1">10</td> <td colname="11" style="background-color:white;" colspan="1">11</td> <td colname="12" style="background-color:white;" colspan="1">12</td> <td colname="13" style="background-color:white;" colspan="1">stable</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">1</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">2</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">3</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {12} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z \oplus \mathbf Z _ {12} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">4</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {12} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1">0</td> <td colname="7" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1">0</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">5</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1">0</td> <td colname="8" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1">0</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">6</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {3} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} \oplus \mathbf Z _ {3} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">7</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {3} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {15} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {15} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {30} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {60} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {120} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z \oplus \mathbf Z _ {120} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {40} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {240} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">8</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {15} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {4} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">9</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {4} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {5} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {4} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \mathbf Z \oplus \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \cdot $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {3} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">10</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \begin{array}{c} |
| | + | \mathbf Z _ {120} \oplus \\ |
| | + | \mathbf Z _ {12} \oplus \mathbf Z _ {2} |
| | + | \end{array} |
| | + | $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {72} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {72} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \begin{array}{c} |
| | + | \mathbf Z _ {24} \oplus \\ |
| | + | \mathbf Z _ {4} \oplus \mathbf Z _ {2} |
| | + | \end{array} |
| | + | $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \mathbf Z _ {6} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \mathbf Z _ {6} $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {6} $ |
| | + | </td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">11</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {84} \oplus \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {84} \oplus \mathbf Z _ {2} ^ {5} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} \oplus \mathbf Z _ {4} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} $ |
| | + | </td> <td colname="12" style="background-color:white;" colspan="1"> $ \mathbf Z \oplus \mathbf Z _ {504} $ |
| | + | </td> <td colname="13" style="background-color:white;" colspan="1"> $ \mathbf Z _ {504} $ |
| | + | </td> </tr> </tbody> </table> |
| | | | |
| | </td></tr> </table> | | </td></tr> </table> |
| | | | |
| − | 2) The groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650268.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650269.png" /> are isomorphic to the groups from the following table:''''''<table border="0" cellpadding="0" cellspacing="0" style="background-color:black;"> <tr><td> <table border="0" cellspacing="1" cellpadding="4" style="background-color:black;"> <tbody> <tr> <td colname="1" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650270.png" /></td> <td colname="2" style="background-color:white;" colspan="1">13</td> <td colname="3" style="background-color:white;" colspan="1">14</td> <td colname="4" style="background-color:white;" colspan="1">15</td> <td colname="5" style="background-color:white;" colspan="1">16</td> <td colname="6" style="background-color:white;" colspan="1">17</td> <td colname="7" style="background-color:white;" colspan="1">18</td> <td colname="8" style="background-color:white;" colspan="1">19</td> <td colname="9" style="background-color:white;" colspan="1">20</td> <td colname="10" style="background-color:white;" colspan="1">21</td> <td colname="11" style="background-color:white;" colspan="1">22</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">0</td> <td colname="2" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650271.png" /></td> <td colname="3" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650272.png" /></td> <td colname="4" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650273.png" /></td> <td colname="5" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650274.png" /></td> <td colname="6" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650275.png" /></td> <td colname="7" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650276.png" /></td> <td colname="8" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650277.png" /></td> <td colname="9" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650278.png" /></td> <td colname="10" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650279.png" /></td> <td colname="11" style="background-color:white;" colspan="1"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650280.png" /></td> </tr> </tbody> </table> | + | 2) The groups $ \pi _ {k} ^ {s} $ |
| | + | with $ 12 \leq k \leq 22 $ |
| | + | are isomorphic to the groups from the following table:<table border="0" cellpadding="0" cellspacing="0" style="background-color:black;"> <tr><td> <table border="0" cellspacing="1" cellpadding="4" style="background-color:black;"> <tbody> <tr> <td colname="1" style="background-color:white;" colspan="1"> $ k = 12 $ |
| | + | </td> <td colname="2" style="background-color:white;" colspan="1">13</td> <td colname="3" style="background-color:white;" colspan="1">14</td> <td colname="4" style="background-color:white;" colspan="1">15</td> <td colname="5" style="background-color:white;" colspan="1">16</td> <td colname="6" style="background-color:white;" colspan="1">17</td> <td colname="7" style="background-color:white;" colspan="1">18</td> <td colname="8" style="background-color:white;" colspan="1">19</td> <td colname="9" style="background-color:white;" colspan="1">20</td> <td colname="10" style="background-color:white;" colspan="1">21</td> <td colname="11" style="background-color:white;" colspan="1">22</td> </tr> <tr> <td colname="1" style="background-color:white;" colspan="1">0</td> <td colname="2" style="background-color:white;" colspan="1"> $ \mathbf Z _ {3} $ |
| | + | </td> <td colname="3" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="4" style="background-color:white;" colspan="1"> $ \mathbf Z _ {480} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="5" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="6" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {4} $ |
| | + | </td> <td colname="7" style="background-color:white;" colspan="1"> $ \mathbf Z _ {8} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="8" style="background-color:white;" colspan="1"> $ \mathbf Z _ {264} \oplus \mathbf Z _ {2} $ |
| | + | </td> <td colname="9" style="background-color:white;" colspan="1"> $ \mathbf Z _ {24} $ |
| | + | </td> <td colname="10" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> <td colname="11" style="background-color:white;" colspan="1"> $ \mathbf Z _ {2} ^ {2} $ |
| | + | </td> </tr> </tbody> </table> |
| | | | |
| | </td></tr> </table> | | </td></tr> </table> |
| | | | |
| − | For further results on the calculation of the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650281.png" />, see [[#References|[3]]]. Particular progress has been achieved in the calculation of the odd primary components of these groups. | + | For further results on the calculation of the groups $ \pi _ {i} ( S ^ {n} ) $, |
| | + | see [[#References|[3]]]. Particular progress has been achieved in the calculation of the odd primary components of these groups. |
| | | | |
| | For example: | | For example: |
| | | | |
| − | 3) If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650282.png" /> is an odd prime number, then the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650283.png" />-primary component of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650284.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650285.png" /> when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650286.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650287.png" />, and is trivial for other <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650288.png" />. | + | 3) If $ p $ |
| | + | is an odd prime number, then the $ p $- |
| | + | primary component of the group $ \pi _ {k} ^ {s} $ |
| | + | is $ \mathbf Z _ {p} $ |
| | + | when $ k = 2l( p- 1)- 1 $, |
| | + | $ l = 1 \dots ( p- 1) $, |
| | + | and is trivial for other $ k < 2p( p- 1)- 2 $. |
| | | | |
| − | There are many results concerning the homotopy groups of the spheres, the domain of action of which is not restricted by any finite range of values <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650289.png" />. In particular, a large number of infinite series of non-trivial elements of the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650290.png" /> is known (see [[#References|[4]]]). | + | There are many results concerning the homotopy groups of the spheres, the domain of action of which is not restricted by any finite range of values $ i- n $. |
| | + | In particular, a large number of infinite series of non-trivial elements of the groups $ \pi _ {i} ( S ^ {n} ) $ |
| | + | is known (see [[#References|[4]]]). |
| | | | |
| − | 4) The order of the image of the [[Whitehead homomorphism|Whitehead homomorphism]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650291.png" /> is equal to the denominator of the irreducible fraction equal to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650292.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650293.png" /> is the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650294.png" />-th Bernoulli number (cf. [[Bernoulli numbers|Bernoulli numbers]]). In particular, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650295.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650296.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650297.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650298.png" />. | + | 4) The order of the image of the [[Whitehead homomorphism|Whitehead homomorphism]] $ J _ {k} $ |
| | + | is equal to the denominator of the irreducible fraction equal to $ B _ {k} /4k $, |
| | + | where $ B _ {k} $ |
| | + | is the $ k $- |
| | + | th Bernoulli number (cf. [[Bernoulli numbers|Bernoulli numbers]]). In particular, $ \mathop{\rm Card} \mathop{\rm Im} J _ {1} = 24 $, |
| | + | $ \mathop{\rm Card} \mathop{\rm Im} J _ {2} = 240 $, |
| | + | $ \mathop{\rm Card} \mathop{\rm Im} J _ {2} = 504 $, |
| | + | $ \mathop{\rm Card} \mathop{\rm Im} J _ {4} = 480 $. |
| | | | |
| | ====References==== | | ====References==== |
| | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> L.S. Pontryagin, "Smooth manifolds and their applications in homotopy theory" , Moscow (1976) (In Russian)</TD></TR><TR><TD valign="top">[2a]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650299.png" /> I" ''Topology'' , '''2''' (1963) pp. 181–195</TD></TR><TR><TD valign="top">[2b]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650300.png" /> II" ''Topology'' , '''3''' (1966) pp. 137–181</TD></TR><TR><TD valign="top">[2c]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650301.png" /> III" ''Topology'' , '''3''' (1966) pp. 193–222</TD></TR><TR><TD valign="top">[2d]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650302.png" /> IV" ''Topology'' , '''5''' (1966) pp. 21–71</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> H. Toda, "Composition methods in homotopy groups of spheres" , Princeton Univ. Press (1962)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> G.W. Whitehead, "Recent advances in homotopy theory" , Amer. Math. Soc. (1970)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> D.B. Fuks, A.T. Fomenko, V.L. Gutenmakher, "Homotopic topology" , Moscow (1969) (In Russian)</TD></TR></table> | | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> L.S. Pontryagin, "Smooth manifolds and their applications in homotopy theory" , Moscow (1976) (In Russian)</TD></TR><TR><TD valign="top">[2a]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650299.png" /> I" ''Topology'' , '''2''' (1963) pp. 181–195</TD></TR><TR><TD valign="top">[2b]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650300.png" /> II" ''Topology'' , '''3''' (1966) pp. 137–181</TD></TR><TR><TD valign="top">[2c]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650301.png" /> III" ''Topology'' , '''3''' (1966) pp. 193–222</TD></TR><TR><TD valign="top">[2d]</TD> <TD valign="top"> J. Adams, "On the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650302.png" /> IV" ''Topology'' , '''5''' (1966) pp. 21–71</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> H. Toda, "Composition methods in homotopy groups of spheres" , Princeton Univ. Press (1962)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> G.W. Whitehead, "Recent advances in homotopy theory" , Amer. Math. Soc. (1970)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> D.B. Fuks, A.T. Fomenko, V.L. Gutenmakher, "Homotopic topology" , Moscow (1969) (In Russian)</TD></TR></table> |
| − |
| |
| − |
| |
| | | | |
| | ====Comments==== | | ====Comments==== |
| − | The general results <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650303.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650304.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650305.png" /> are also together termed the Hurewicz theorem. The fact that the suspension induces an isomorphism in the appropriate range is known as the Freudenthal suspension theorem. The result that the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650306.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650307.png" />, are finite except for the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650308.png" />, which are of the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650309.png" />(finite), is known as Serre's finiteness theorem. An additional result pertaining to the composition product is the Nishida nilpotence theorem that each <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650310.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650311.png" />, is nilpotent. Further, there is the Cohen–Moore–Neisendorfer exponent theorem, which says that for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650312.png" /> the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650313.png" />-component of the Abelian group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650314.png" /> has exponent <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650315.png" />. | + | The general results $ \pi _ {n} ( S ^ {n} ) = \mathbf Z $, |
| | + | $ \pi _ {m} ( S ^ {n} ) = 0 $ |
| | + | for $ m < n $ |
| | + | are also together termed the Hurewicz theorem. The fact that the suspension induces an isomorphism in the appropriate range is known as the Freudenthal suspension theorem. The result that the $ \pi _ {n+} k ( S ^ {n} ) $, |
| | + | $ k > 0 $, |
| | + | are finite except for the $ \pi _ {4m-} 1 ( S ^ {2m} ) $, |
| | + | which are of the form $ \mathbf Z \oplus $( |
| | + | finite), is known as Serre's finiteness theorem. An additional result pertaining to the composition product is the Nishida nilpotence theorem that each $ \alpha \in \pi _ {k} ^ {s} $, |
| | + | $ k > 0 $, |
| | + | is nilpotent. Further, there is the Cohen–Moore–Neisendorfer exponent theorem, which says that for $ p \geq 5 $ |
| | + | the $ p $- |
| | + | component of the Abelian group $ \pi _ {2i + 1 + j } ( S ^ {2i+} 1 ) $ |
| | + | has exponent $ p ^ {i} $. |
| | | | |
| − | For a very complete discussion of the homotopy groups of the spheres, and in particular the Adams–Novikov spectral sequence and its <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s086/s086650/s086650316.png" /> term, cf. [[#References|[a2]]]. | + | For a very complete discussion of the homotopy groups of the spheres, and in particular the Adams–Novikov spectral sequence and its $ E ^ {2} $ |
| | + | term, cf. [[#References|[a2]]]. |
| | | | |
| | ====References==== | | ====References==== |
| | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Dieudonné, "A history of algebraic and differential topology 1900–1960" , Birkhäuser (1989)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D.C. Ravenel, "Complex cobordism and stable homotopy groups of the spheres" , Acad. Press (1986)</TD></TR></table> | | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Dieudonné, "A history of algebraic and differential topology 1900–1960" , Birkhäuser (1989)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> D.C. Ravenel, "Complex cobordism and stable homotopy groups of the spheres" , Acad. Press (1986)</TD></TR></table> |
An object of study in classical homotopy theory. The calculation of the homotopy groups of the spheres, $ \pi _ {i} ( S ^ {n} ) $,
was considered in its time (especially in the 1950's) as one of the central problems in topology. Topologists hoped that these groups could be successfully calculated completely, and that they would help to solve other classification problems in homotopy. These hopes were not to be realized in full: The homotopy groups of the spheres could only be calculated partially, and with the development of generalized cohomology theories, the problem of their calculation became less pressing. However, all the information that had been compiled on these groups was not wasted, as it found an unexpected use in differential topology (the classification of differential structures on spheres and multi-dimensional knots).
I. General theory.
1) If $ i < n $
or $ i > n= 1 $,
then $ \pi _ {i} ( S ^ {n} ) = 0 $.
2) $ \pi _ {n} ( S ^ {n} ) = \mathbf Z $(
the Brouwer–Hopf theorem); this isomorphism relates an element of the group $ \pi _ {n} ( S ^ {n} ) $
to the degree of the mapping $ S ^ {n} \rightarrow S ^ {n} $
representing it.
3) The groups $ \pi _ {4m-} 1 ( S ^ {2m} ) $
have rank 1; the other groups $ \pi _ {i} ( S ^ {n} ) $
with $ i \neq n $
are finite.
The suspension homomorphism
$$
E : \pi _ {i} ( S ^ {n} ) \rightarrow \pi _ {i+} 1 ( S ^ {n+} 1 )
$$
relates an element of the group $ \pi _ {i} ( S ^ {n} ) $,
represented by the mapping $ f: S ^ {i} \rightarrow S ^ {n} $,
to the class of the mapping $ Ef : S ^ {i+} 1 \rightarrow S ^ {n+} 1 $,
defined by the formula
$$
Ef( \sqrt {1 - x ^ {2} } \mathbf x , x) = \left \{
\begin{array}{ll}
( \sqrt {1- x ^ {2} } f( \mathbf x ), x), & | x | \leq 1, \\
( \mathbf 0 , x), & | x | = 1, \\
\end{array}
\right .$$
where $ \mathbf x \in S ^ {i} $,
$ x \in \mathbf R $.
4) The homomorphism $ E $
is an isomorphism when $ i > 2n- 1 $,
and an epimorphism when $ i \geq 2n- 1 $.
Thus, for every $ k $
the groups $ \pi _ {n+} k ( S ^ {n} ) $
can be made terms of a sequence
$$
\pi _ {1+} k ( S ^ {1} ) \
\rightarrow ^ { E } \
\pi _ {2+} k ( S ^ {2} ) \
\rightarrow ^ { E } \
\pi _ {3+} k ( S ^ {3} ) \
\rightarrow ^ { E } \dots ,
$$
at the $ ( k+ 2) $-
nd term of which stabilization begins; the groups $ \pi _ {n+} k ( S ^ {n} ) $
with $ n \geq k+ 2 $
are called the $ k $-
th stable homotopy groups of the spheres, and are denoted by $ \pi _ {k} ^ {s} $.
Then $ \pi _ {k} ^ {s} = 0 $
when $ k < 0 $
and $ \pi _ {0} ^ {s} = \mathbf Z $.
As for the homotopy groups (cf. Homotopy group) of any topological space, the Whitehead product is defined on the homotopy groups of the spheres:
$$
\pi _ {i} ( S ^ {n} ) \times \pi _ {j} ( S ^ {n} ) \rightarrow \pi _ {i+} j- 1 ( S ^ {n} ) ,\ \
( \alpha , \beta ) \rightarrow [ \alpha , \beta ].
$$
To its usual properties (distributivity, skew commutativity and the Jacobi identity) is added
5) $ E[ \alpha , \beta ] = 0 $.
The Whitehead product enables one to make the following refinement to 4):
6) The kernel of the epimorphism $ E : \pi _ {2n-} 1 ( S ^ {n} ) \rightarrow \pi _ {2n} ( S ^ {n+} 1 ) $
is generated by the class $ [ i _ {n} , i _ {n} ] $,
where $ i _ {n} $
is a canonical generator of the group $ \pi _ {n} ( S ^ {n} ) $(
representable by the identity mapping).
Closely linked to the Whitehead product is the Hopf invariant $ H( \alpha ) $,
defined for $ \alpha \in \pi _ {4m-} 1 ( S ^ {2m} ) $.
Thus, the element of the group $ \pi _ {3} ( S ^ {2} ) $
which can be represented by the Hopf mapping $ h: S ^ {3} \rightarrow S ^ {2} $
that operates according to the formula $ h( z _ {1} , z _ {2} ) = z _ {1} : z _ {2} $(
in which $ S ^ {3} $
is interpreted as the unit sphere in the space $ \mathbf C ^ {2} $,
while $ S ^ {2} $
is interpreted as $ \mathbf C P ^ {1} $)
has Hopf invariant equal to 1.
7) The mapping $ H: \pi _ {3} ( S ^ {2} ) \rightarrow \mathbf Z $
is an isomorphism.
8) $ H([ i _ {2m} , i _ {2m} ]) = 2 $.
A consequence of 8) is that the groups $ \pi _ {4m-} 1 ( S ^ {2m} ) $
are infinite, a fact already stated in 3).
9) When $ m \neq 1, 2, 4 $,
in $ \pi _ {4m-} 1 ( S ^ {2m} ) $
there are no elements of odd Hopf invariant (as was known long before this theorem was proved, its assertion is equivalent to the following Frobenius conjecture: when $ l \neq 1, 2, 4, 8 $,
then in $ \mathbf R ^ {l} $
there is no bilinear multiplication with single-valued division on non-zero elements).
The composition product
$$
\pi _ {i} ( S ^ {j} ) \times \pi _ {j} ( S ^ {n} ) \rightarrow \pi _ {i} ( S ^ {n} ) ,\ \
( \beta , \alpha ) \rightarrow \alpha \circ \beta ,
$$
which can be defined by juxtaposition of mappings, is unique to the spheres.
10) For any $ \alpha , \alpha _ {1} , \alpha _ {2} \in \pi _ {j} ( S ^ {n} ) $,
$ \beta , \beta _ {1} , \beta _ {2} \in \pi _ {i} ( S ^ {j} ) $,
$ \delta \in \pi _ {i-} 1 ( S ^ {j-} 1 ) $,
$ \gamma \in \pi _ {k} ( S ^ {j} ) $,
the following hold:
a) $ ( \alpha \circ \beta ) \circ \gamma = \alpha \circ ( \beta \circ \gamma ) $;
b) $ \alpha \circ ( \beta _ {1} + \beta _ {2} ) = \alpha \circ \beta _ {1} + \alpha \circ \beta _ {2} $;
c) $ ( \alpha _ {1} + \alpha _ {2} ) \circ E \delta = \alpha _ {1} \circ E \delta + \alpha _ {2} \circ E \delta $;
d) $ E( \alpha \circ \beta ) = E \alpha \circ E \beta $.
The "left law of distributivity" , $ ( \alpha _ {1} + \alpha _ {2} ) \circ \beta = \alpha _ {1} \circ \beta + \alpha _ {2} \circ \beta $,
generally speaking, does not hold. Assertion d) enables one to define a stable composition product
$$
\pi _ {q} ^ {s} \times \pi _ {r} ^ {s} \rightarrow \pi _ {q+} r ^ {s} ,\ \
( \beta , \alpha ) \rightarrow \alpha \circ \beta .
$$
11) For any $ \alpha , \alpha _ {1} , \alpha _ {2} \in \pi _ {r} ^ {s} $,
$ \beta , \beta _ {1} , \beta _ {2} \in \pi _ {q} ^ {s} $,
$ \gamma \in \pi _ {p} ^ {s} $,
assertions a) and b) in 10) hold, as do:
c') $ ( \alpha _ {1} + \alpha _ {2} ) \circ \beta = \alpha _ {1} \circ \beta + \alpha _ {2} \circ \beta $,
d') $ \alpha \circ \beta = (- 1) ^ {qr} \beta \circ \alpha $.
II. Methods of calculation.
The geometric method of L.S. Pontryagin (see [1]), proposed in the mid-1930s, is based on the following definition. A smooth $ m $-
dimensional compact manifold $ X $
in $ \mathbf R ^ {i} $
is said to be framed if a smooth field of $ ( i- m) $-
frames transversal to it is defined on the manifold; the field itself is said to be a framing. Two framed manifolds $ X _ {0} , X _ {1} \subset \mathbf R ^ {i} $,
without boundary, are said to be cobordant if there exists a framed manifold $ Y \subset \mathbf R ^ {i} \times [ 0, 1] \subset \mathbf R ^ {i+} 1 $
with $ \partial Y = ( X _ {0} \times 0) \cup ( X _ {1} \times 1) $
for which the restriction of the framing onto $ X _ {0} \times 0 $
and $ X _ {1} \times 1 $
is contained in $ \mathbf R ^ {i} \times 0 $
and $ \mathbf R ^ {i} \times 1 $,
and, given a natural identification of $ \mathbf R ^ {i} \times 0 $
and $ \mathbf R ^ {i} \times 1 $
with $ \mathbf R ^ {i} $,
is turned into the given framing of the manifolds $ X _ {0} $
and $ X _ {1} $.
The set of classes of cobordant framed $ m $-
dimensional manifolds without boundary in $ \mathbf R ^ {i} $
is denoted by $ \Omega ^ {m} ( i) $.
1) There is a one-to-one correspondence between $ \pi _ {i} ( S ^ {n} ) $
and $ \Omega ^ {i-} n ( i) $.
This method gives good results for small $ i- n $.
It also makes it possible to prove certain of the theorems in section I and provides a variety of geometric information on manifolds of small dimensions.
Another group of methods consists of elementary algebraic methods comprising the use of homotopy sequences of various fibre bundles, properties of the Whitehead product, the composition product, and the corresponding higher product (Toda brackets, see [3]), as well as the following theorem of James.
2) There is a sequence of groups and homomorphisms
$$
{} \dots \rightarrow \pi _ {i} ( S ^ {n} ) \rightarrow ^ { E } \
\pi _ {i+} 1 ( S ^ {n+} 1 ) \rightarrow ^ { H } \pi _ {i+} 1 ( S ^ {2n+} 1 ) \rightarrow ^ { P } \
$$
$$
\rightarrow ^ { P } \pi _ {i-} 1 ( S ^ {n} ) \rightarrow \dots ,
$$
which is exact for odd $ n $
and for $ i < 3n- 1 $(
in this sequence, $ H $
is a generalization of the Hopf invariant).
Elementary algebraic methods prove to be reasonable effective: It is possible to calculate the groups $ \pi _ {i} ( S ^ {n} ) $
when $ i- n \leq 13 $
almost without having to resort to other methods.
There is also the method of killing spaces (see [5] and Killing space). This method is suitable for the calculation of the homotopy groups of any space. It is based on the construction, using a space $ X $,
of a sequence of killing spaces $ X \mid _ {k} $
with the following property:
$$
\pi _ {i} ( X \mid _ {k} ) = \left \{
\begin{array}{ll}
\pi _ {i} ( X), & i \geq k, \\
0, & i < k. \\
\end{array}
\right .$$
Thus, $ \pi _ {i} ( X) = \pi _ {i} ( X | _ {i} ) = H _ {i} ( X | _ {i} ) $
and the problem of calculating the homotopy groups reduces to the problem of calculating the homology groups (and the cohomology groups) of $ X \mid _ {i} $.
These homology groups are found by induction, using spectral sequences (cf. Spectral sequence) of fibre bundles: $ X \mid _ {k} $
is broken down with fibre $ X \mid _ {k+} 1 $
over the Eilenberg–MacLane space $ K( \pi _ {k} ( X), k) $.
The calculation does not have an automatic character: In order to progress, it is necessary to know as much as possible about the cohomology groups of $ X $,
including the action in them of the primary and the higher cohomology operations (cf. Cohomology operation).
A more suitable apparatus for calculating the stable homotopy groups of the spheres is the Adams spectral sequence. Let $ p $
be a prime number, and let $ A _ {(} p) $
be the Steenrod algebra of stable cohomology operations on the cohomology spaces with coefficients in $ Z _ {p} $.
3) There exists a spectral sequence the first term of which coincides with the cohomology groups of the Steenrod algebra (i.e. with $ \mathop{\rm Ext} _ {A ^ {(} p) } ( \mathbf Z _ {p} , \mathbf Z _ {p} ) $),
while the limit term is related to the stable homotopy groups of the spheres factored by the torsion of order relatively prime to $ p $.
The Adams spectral sequence permits one to achieve considerable progress in the calculation of the stable homotopy groups of the spheres. An analogous spectral sequence exists for the calculation of the stable homotopy groups of any space. There is also an unstable analogue of the Adams spectral sequence (see [4]).
More modern methods of calculating the homotopy groups of the spheres are based on generalized cohomology theories. One of these involves the use of the Adams' $ e $-
invariant, which is closely linked to $ K $-
theory. In constructing this $ e $-
invariant, a mapping $ f: S ^ {i} \rightarrow S ^ {n} $
representing a class $ \alpha \in \pi _ {i} ( S ^ {n} ) $
is fixed, and the space $ X _ \alpha = S ^ {n} \cup _ {f} D ^ {i+} 1 $,
obtained by attaching an $ ( i+ 1) $-
dimensional cell to the sphere $ S ^ {n} $
through the mapping $ f $,
is examined. It turns out that
$$
H _ {n} ( X _ \alpha ; \mathbf Z ) \cong \
H _ {i+} 1 ( X _ \alpha ; \mathbf Z )
\cong \mathbf Z .
$$
Let $ \mu , \nu $
be the canonical generators of these groups. There exists a complex vector bundle $ \xi $
over $ X _ \alpha $
with Chern character $ \mathop{\rm ch} \xi $
satisfying the relation $ \langle \mathop{\rm ch} \xi , \mu \rangle = 1 $.
Then $ \langle \mathop{\rm ch} \xi , \nu \rangle $
is a rational number, the residue of which modulo 1 does not depend on the choice of $ \xi $.
This residue is the $ e $-
invariant $ e( \alpha ) $
of the class $ \alpha $.
The function $ e $
is a homomorphism
$$
e: \pi _ {i} ( S ^ {n} ) \rightarrow \mathbf Q / \mathbf Z ,
$$
whose image can be determined (see ).
Finally, the potentially most powerful method of calculating the homotopy groups of the spheres (and not only of the spheres) is the Adams–Novikov spectral sequence, an analogue of the Adams spectral sequence, constructed on the basis not of ordinary cohomology groups, but of cobordisms. However, an explicit calculation of the first term of this sequence has inherent difficulties, which have not been overcome yet (1984).
III. Results of calculations.
1) The groups $ \pi _ {i} ( S ^ {n} ) $
with $ i- n \leq 2 $
are isomorphic to the groups from the following table:
<tbody> </tbody> | $ \begin{array}{c}
n \\
i- n
\end{array}
$
| 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | stable | | 1 | $ \mathbf Z $
| $ \mathbf Z _ {2} $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {2} $
| | 2 | $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {2} $
| | 3 | $ \mathbf Z _ {2} $
| $ \mathbf Z _ {12} $
| $ \mathbf Z \oplus \mathbf Z _ {12} $
| $ \mathbf Z _ {24} $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {24} $
| | 4 | $ \mathbf Z _ {12} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {2} $
| 0 | $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| 0 | | 5 | $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z $
| 0 | $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| 0 | | 6 | $ \mathbf Z _ {2} $
| $ \mathbf Z _ {3} $
| $ \mathbf Z _ {24} \oplus \mathbf Z _ {3} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {2} $
| | 7 | $ \mathbf Z _ {3} $
| $ \mathbf Z _ {15} $
| $ \mathbf Z _ {15} $
| $ \mathbf Z _ {30} $
| $ \mathbf Z _ {60} $
| $ \mathbf Z _ {120} $
| $ \mathbf Z \oplus \mathbf Z _ {120} $
| $ \mathbf Z _ {40} $
| $ \cdot $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {240} $
| | 8 | $ \mathbf Z _ {15} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} $
| $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {4} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \cdot $
| $ \cdot $
| $ \mathbf Z _ {2} ^ {2} $
| | 9 | $ \mathbf Z _ {2} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {4} $
| $ \mathbf Z _ {2} ^ {5} $
| $ \mathbf Z _ {2} ^ {4} $
| $ \mathbf Z \oplus \mathbf Z _ {2} ^ {3} $
| $ \mathbf Z _ {2} ^ {3} $
| $ \cdot $
| $ \mathbf Z _ {2} ^ {3} $
| | 10 | $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $
| $ \begin{array}{c}
\mathbf Z _ {120} \oplus \\
\mathbf Z _ {12} \oplus \mathbf Z _ {2}
\end{array}
$
| $ \mathbf Z _ {72} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {72} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $
| $ \begin{array}{c}
\mathbf Z _ {24} \oplus \\
\mathbf Z _ {4} \oplus \mathbf Z _ {2}
\end{array}
$
| $ \mathbf Z _ {24} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {6} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {6} $
| $ \mathbf Z _ {6} $
| | 11 | $ \mathbf Z _ {12} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {84} \oplus \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {84} \oplus \mathbf Z _ {2} ^ {5} $
| $ \mathbf Z _ {504} \oplus \mathbf Z _ {4} $
| $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {504} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {504} $
| $ \mathbf Z _ {504} $
| $ \mathbf Z \oplus \mathbf Z _ {504} $
| $ \mathbf Z _ {504} $
|
|
2) The groups $ \pi _ {k} ^ {s} $
with $ 12 \leq k \leq 22 $
are isomorphic to the groups from the following table:
<tbody> </tbody> | $ k = 12 $
| 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | | 0 | $ \mathbf Z _ {3} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {480} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {2} ^ {4} $
| $ \mathbf Z _ {8} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {264} \oplus \mathbf Z _ {2} $
| $ \mathbf Z _ {24} $
| $ \mathbf Z _ {2} ^ {2} $
| $ \mathbf Z _ {2} ^ {2} $
|
|
For further results on the calculation of the groups $ \pi _ {i} ( S ^ {n} ) $,
see [3]. Particular progress has been achieved in the calculation of the odd primary components of these groups.
For example:
3) If $ p $
is an odd prime number, then the $ p $-
primary component of the group $ \pi _ {k} ^ {s} $
is $ \mathbf Z _ {p} $
when $ k = 2l( p- 1)- 1 $,
$ l = 1 \dots ( p- 1) $,
and is trivial for other $ k < 2p( p- 1)- 2 $.
There are many results concerning the homotopy groups of the spheres, the domain of action of which is not restricted by any finite range of values $ i- n $.
In particular, a large number of infinite series of non-trivial elements of the groups $ \pi _ {i} ( S ^ {n} ) $
is known (see [4]).
4) The order of the image of the Whitehead homomorphism $ J _ {k} $
is equal to the denominator of the irreducible fraction equal to $ B _ {k} /4k $,
where $ B _ {k} $
is the $ k $-
th Bernoulli number (cf. Bernoulli numbers). In particular, $ \mathop{\rm Card} \mathop{\rm Im} J _ {1} = 24 $,
$ \mathop{\rm Card} \mathop{\rm Im} J _ {2} = 240 $,
$ \mathop{\rm Card} \mathop{\rm Im} J _ {2} = 504 $,
$ \mathop{\rm Card} \mathop{\rm Im} J _ {4} = 480 $.
References
| [1] | L.S. Pontryagin, "Smooth manifolds and their applications in homotopy theory" , Moscow (1976) (In Russian) |
| [2a] | J. Adams, "On the groups  I" Topology , 2 (1963) pp. 181–195 I" Topology , 2 (1963) pp. 181–195 |
| [2b] | J. Adams, "On the groups  II" Topology , 3 (1966) pp. 137–181 II" Topology , 3 (1966) pp. 137–181 |
| [2c] | J. Adams, "On the groups 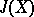 III" Topology , 3 (1966) pp. 193–222 III" Topology , 3 (1966) pp. 193–222 |
| [2d] | J. Adams, "On the groups  IV" Topology , 5 (1966) pp. 21–71 IV" Topology , 5 (1966) pp. 21–71 |
| [3] | H. Toda, "Composition methods in homotopy groups of spheres" , Princeton Univ. Press (1962) |
| [4] | G.W. Whitehead, "Recent advances in homotopy theory" , Amer. Math. Soc. (1970) |
| [5] | D.B. Fuks, A.T. Fomenko, V.L. Gutenmakher, "Homotopic topology" , Moscow (1969) (In Russian) |
The general results $ \pi _ {n} ( S ^ {n} ) = \mathbf Z $,
$ \pi _ {m} ( S ^ {n} ) = 0 $
for $ m < n $
are also together termed the Hurewicz theorem. The fact that the suspension induces an isomorphism in the appropriate range is known as the Freudenthal suspension theorem. The result that the $ \pi _ {n+} k ( S ^ {n} ) $,
$ k > 0 $,
are finite except for the $ \pi _ {4m-} 1 ( S ^ {2m} ) $,
which are of the form $ \mathbf Z \oplus $(
finite), is known as Serre's finiteness theorem. An additional result pertaining to the composition product is the Nishida nilpotence theorem that each $ \alpha \in \pi _ {k} ^ {s} $,
$ k > 0 $,
is nilpotent. Further, there is the Cohen–Moore–Neisendorfer exponent theorem, which says that for $ p \geq 5 $
the $ p $-
component of the Abelian group $ \pi _ {2i + 1 + j } ( S ^ {2i+} 1 ) $
has exponent $ p ^ {i} $.
For a very complete discussion of the homotopy groups of the spheres, and in particular the Adams–Novikov spectral sequence and its $ E ^ {2} $
term, cf. [a2].
References
| [a1] | J. Dieudonné, "A history of algebraic and differential topology 1900–1960" , Birkhäuser (1989) |
| [a2] | D.C. Ravenel, "Complex cobordism and stable homotopy groups of the spheres" , Acad. Press (1986) |
 I" Topology , 2 (1963) pp. 181–195
I" Topology , 2 (1963) pp. 181–195 II" Topology , 3 (1966) pp. 137–181
II" Topology , 3 (1966) pp. 137–181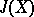 III" Topology , 3 (1966) pp. 193–222
III" Topology , 3 (1966) pp. 193–222 IV" Topology , 5 (1966) pp. 21–71
IV" Topology , 5 (1966) pp. 21–71