Difference between revisions of "Dirichlet L-function"
m (links) |
m (→References: latexify) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | d0328903.png | ||
| + | $#A+1 = 182 n = 3 | ||
| + | $#C+1 = 182 : ~/encyclopedia/old_files/data/D032/D.0302890 Dirichlet \BMI L\EMI\AAhfunction, | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | + | ''Dirichlet $ L $- | |
| + | series, $ L $- | ||
| + | series'' | ||
| − | + | A function of a complex variable $ s = \sigma + it $ | |
| + | that is defined for any [[Dirichlet character|Dirichlet character]] $ \chi $ | ||
| + | $ \mathop{\rm mod} d $ | ||
| + | by the series | ||
| − | + | $$ \tag{1 } | |
| + | L ( s , \chi ) = \sum _ {n = 1 } ^ \infty | ||
| − | + | \frac{\chi ( n) }{n ^ {s} } | |
| + | . | ||
| + | $$ | ||
| − | + | As functions of a real variable these were introduced by P.G.L. Dirichlet [[#References|[1]]] in 1837 in the context of the proof that the number of primes in an arithmetic progression $ \{ {dm + l } : {m = 0 , 1 ,\dots } \} $, | |
| + | where the difference $ d $ | ||
| + | and the first term $ l $ | ||
| + | are relatively prime numbers, is infinite. They are a natural generalization of the Riemann [[Zeta-function|zeta-function]] $ \zeta ( s) $ | ||
| + | to an arithmetic progression and are a powerful tool in analytic number theory [[#References|[2]]]–[[#References|[4]]]. | ||
| − | + | The series (1), known as a [[Dirichlet series]], converges absolutely and uniformly in any bounded domain in the complex $ s $- | |
| + | plane for which $ \sigma \geq 1 + \gamma $, | ||
| + | $ \gamma > 0 $. | ||
| + | If $ \chi $ | ||
| + | is a non-principal character, one has | ||
| − | + | $$ \tag{2 } | |
| + | L ( s , \chi ) = s \int\limits _ { 1 } ^ \infty \sum _ {n \leq u } | ||
| + | \chi ( n) u ^ {- s - 1 } d u . | ||
| + | $$ | ||
| − | + | Since the sum in the integrand is bounded, this formula gives an analytic continuation of $ L ( s , \chi ) $ | |
| + | to a regular function in the half-plane $ \sigma > 0 $. | ||
| − | + | For any $ \chi $ | |
| + | $ \mathop{\rm mod} d $ | ||
| + | it is possible to represent $ L ( s , \chi ) $ | ||
| + | as an Euler product over prime numbers $ p $: | ||
| − | + | $$ \tag{3 } | |
| + | L ( s , \chi ) = \prod _ { p } \left ( 1 - | ||
| + | \frac{\chi ( p) }{p ^ {s} } | ||
| + | \right ) ^ {-} 1 ,\ \sigma > 1 . | ||
| + | $$ | ||
| − | + | Hence, if $ \chi = \chi _ {0} $ | |
| + | is a [[principal character]] $ \mathop{\rm mod} d $, | ||
| + | one has, for $ d = 1 $, | ||
| − | + | $$ | |
| + | L ( s , \chi _ {0} ) = \zeta ( s) , | ||
| + | $$ | ||
| − | + | and for $ d > 1 $, | |
| − | + | $$ | |
| + | L ( s , \chi _ {0} ) = \zeta ( s) \prod _ {p \mid d } | ||
| + | \left ( 1 - | ||
| + | \frac{1}{p ^ {s} } | ||
| + | \right ) . | ||
| + | $$ | ||
| − | + | For this reason the properties of $ L ( s , \chi _ {0} ) $ | |
| + | in the entire complex plane are mainly determined by the properties of $ \zeta ( s) $. | ||
| + | In particular, the function $ L ( s , \chi _ {0} ) $ | ||
| + | is regular for all $ s $, | ||
| + | except for $ s = 1 $ | ||
| + | where it has a simple pole with residue $ \phi ( d) / d $; | ||
| + | here $ \phi $ | ||
| + | is Euler's function. If, on the other hand, $ \chi \neq \chi _ {0} $ | ||
| + | and if $ \chi ^ {*} $ | ||
| + | is the primitive character inducing the character $ \chi $ | ||
| + | $ \mathop{\rm mod} d $, | ||
| + | then | ||
| + | |||
| + | $$ \tag{4 } | ||
| + | L ( s , \chi ) = L ( s , \chi ^ {*} ) \prod _ {p \mid d } | ||
| + | \left ( 1 - | ||
| + | \frac{\chi ^ {*} ( p) }{p ^ {s} } | ||
| + | \right ) . | ||
| + | $$ | ||
| + | |||
| + | Thus, it is no essential restriction to consider only Dirichlet $ L $- | ||
| + | functions for primitive characters. This property of Dirichlet $ L $- | ||
| + | functions is important, since many results concerning $ L ( s , \chi ) $ | ||
| + | have a simple form for primitive characters only. If $ \chi $ | ||
| + | $ \mathop{\rm mod} d $ | ||
| + | is primitive, the analytic continuation to the entire plane and the functional equation for the function $ L ( s , \chi ) $ | ||
| + | are obtained by direct generalization of Riemann's method for $ \zeta ( s) $. | ||
| + | Putting | ||
| + | |||
| + | $$ | ||
| + | \xi ( s , \chi ) = \left ( | ||
| + | \frac{d} \pi | ||
| + | \right ) ^ | ||
| + | {( s + \delta ) / 2 } \Gamma \left ( | ||
| + | \frac{s + \delta }{2} | ||
| + | \right ) | ||
| + | L ( s , \chi ) ,\ \delta = | ||
| + | \frac{1 - \chi ( - 1 ) }{2} | ||
| + | , | ||
| + | $$ | ||
the result has the form | the result has the form | ||
| − | + | $$ \tag{5 } | |
| + | \xi ( 1 - s , \overline \chi \; ) = \epsilon ( \chi ) \xi ( s , \chi ) , | ||
| + | $$ | ||
| + | |||
| + | where $ \Gamma $ | ||
| + | is the gamma-function, $ \epsilon ( \chi ) = i ^ \delta d ^ {1/2 } / \tau ( \chi ) $, | ||
| + | $ | \epsilon ( \chi ) | = 1 $, | ||
| + | $ \tau ( \chi ) $ | ||
| + | is a [[Gauss sum|Gauss sum]], and $ \overline \chi \; $ | ||
| + | is the complex conjugate character to $ \chi $. | ||
| + | This equation is known as the functional equation of the function $ L( s, \chi ) $. | ||
| + | It follows from this formula and from formulas (2) and (4) that the functions $ L( s, \chi ) $ | ||
| + | and $ \xi ( x, \chi ) $ | ||
| + | are entire functions for all $ \chi \neq \chi _ {0} $; | ||
| + | if $ \sigma \leq 0 $, | ||
| + | $ L( s, \chi ) = 0 $ | ||
| + | only at the points $ s = - 2 \nu - \delta $, | ||
| + | $ \nu = 0 , 1 \dots $ | ||
| + | and at the points $ s $ | ||
| + | where the product in (4) vanishes; these points are known as the trivial zeros of $ L ( s , \chi ) $. | ||
| + | The remaining zeros of $ L ( s , \chi ) $ | ||
| + | are said to be the non-trivial zeros. If $ \sigma > 1 $, | ||
| + | then $ L ( s , \chi ) \neq 0 $. | ||
| + | Ch.J. de la Vallée-Poussin showed that $ L ( 1 + it, \chi ) \neq 0 $, | ||
| + | so that all non-trivial zeros of a Dirichlet $ L $- | ||
| + | function lie in the domain $ 0 < \sigma < 1 $, | ||
| + | which is known as the critical strip. | ||
| − | + | The distribution of the non-trivial zeros, and of the values of $ L ( s , \chi ) $ | |
| + | in the critical strip in general, is the most important problem in the theory of Dirichlet $ L $- | ||
| + | functions, and is of fundamental importance in number theory. | ||
| − | + | That each function $ L ( s , \chi ) $ | |
| + | has infinitely many non-trivial zeros, and that the laws governing the distribution of primes in arithmetic progressions directly depend on the distribution of these zeros, is shown by the corresponding analogues of Riemann's formulas. In fact, let $ N ( T , \chi ) $ | ||
| + | be the number of zeros of the function $ L ( s , \chi ) $ | ||
| + | with a primitive character $ \chi $ | ||
| + | $ \mathop{\rm mod} d $ | ||
| + | in the rectangle $ 0 < \sigma < 1 $, | ||
| + | $ | t | < T $, | ||
| + | $ T \geq 2 $. | ||
| + | Then | ||
| − | + | $$ | |
| − | + | \frac{N( T, \chi ) }{2} | |
| + | = | ||
| + | \frac{T}{2 \pi } | ||
| + | \mathop{\rm ln} | ||
| + | \frac{Td}{2 \pi } | ||
| + | - | ||
| − | + | \frac{T}{2 \pi } | |
| + | + O( \mathop{\rm ln} Td ). | ||
| + | $$ | ||
| − | + | Let $ \Lambda ( n) $ | |
| + | be the [[Mangoldt function|von Mangoldt function]], $ 1 \leq l \leq d $, | ||
| + | $ ( l , d) = 1 $, | ||
| + | and let | ||
| − | + | $$ | |
| + | \psi ( x ; d , l) = \sum _ {\begin{array}{c} | ||
| + | n \leq x , \\ | ||
| + | n \equiv l ( \mathop{\rm mod} d | ||
| + | ) | ||
| + | \end{array} | ||
| + | } \Lambda ( n) , | ||
| + | $$ | ||
| + | |||
| + | $$ | ||
| + | \psi ( x ; \chi ) = \sum _ {n \leq x } \chi ( n) \Lambda ( n) . | ||
| + | $$ | ||
Then it follows from the orthogonality property of the characters that | Then it follows from the orthogonality property of the characters that | ||
| − | + | $$ \tag{6 } | |
| + | \psi ( x ; d , l) = | ||
| + | \frac{1}{\phi ( d) } | ||
| + | \sum _ {\chi \mathop{\rm mod} \ | ||
| + | d } \overline \chi \; ( l) \psi ( x ; \chi ) , | ||
| + | $$ | ||
| + | |||
| + | where the summation is extended over all characters $ \chi $ | ||
| + | $ \mathop{\rm mod} d $. | ||
| + | Moreover, for a primitive character $ \chi $ | ||
| + | and for $ \alpha = 1 - \delta $: | ||
| − | + | $$ | |
| + | \psi ( x ; \chi ) = - \sum _ \rho | ||
| + | \frac{x ^ \rho } \rho | ||
| + | + \sum _ { m= } 1 ^ \infty | ||
| + | \frac{x ^ {\delta - 2m } }{2m - \delta } | ||
| + | + | ||
| + | $$ | ||
| − | + | $$ | |
| + | - \lim\limits _ {s \rightarrow 0 } \left \{ | ||
| + | \frac{L ^ \prime ( \alpha s , \chi ) }{L ( \alpha s , \chi ) } | ||
| + | - | ||
| + | \frac \alpha {s} | ||
| + | \right \} - \alpha \mathop{\rm ln} x , | ||
| + | $$ | ||
| − | + | where $ \rho = \beta + i \gamma $ | |
| + | runs through the non-trivial zeros of $ L ( s , \chi ) $, | ||
| + | and $ L ^ \prime $ | ||
| + | is the derivative of $ L $ | ||
| + | with respect to $ s $. | ||
| − | + | Approximate formulas for $ \psi ( x ; \chi ) $ | |
| + | are more useful in practice: For arbitrary $ \chi \neq \chi _ {0} $ | ||
| + | and for $ 2 \leq T \leq x $ | ||
| + | one has | ||
| − | + | $$ \tag{7 } | |
| + | \psi ( x ; \chi ) = - \sum _ {| \gamma | < T } | ||
| + | \frac{x ^ \rho } \rho | ||
| + | + \sum _ {| \gamma | < 1 } | ||
| + | \frac{1} \rho | ||
| + | + O \left ( | ||
| + | \frac{x}{T} | ||
| − | + | \mathop{\rm ln} ^ {2} xd \right ) , | |
| + | $$ | ||
| − | and for | + | and for $ \chi = \chi _ {0} $, |
| − | + | $$ \tag{8 } | |
| + | \psi ( x ; \chi _ {0} ) = \sum _ {n \leq x } \Lambda ( n) + O ( | ||
| + | \mathop{\rm ln} x \mathop{\rm ln} d ) . | ||
| + | $$ | ||
The quantity in (8) is the principal term of the sum in (6). | The quantity in (8) is the principal term of the sum in (6). | ||
| − | According to the so-called extended Riemann hypothesis, all non-trivial zeros of a Dirichlet | + | According to the so-called extended Riemann hypothesis, all non-trivial zeros of a Dirichlet $ L $- |
| + | function lie on the straight line $ \sigma = 1/2 $. | ||
| + | If this hypothesis is valid, one has, for $ d \leq x $, | ||
| − | + | $$ | |
| + | \psi ( x ; d, l ) = | ||
| + | \frac{x}{\phi ( d) } | ||
| + | + O ( \sqrt x \mathop{\rm ln} | ||
| + | ^ {2} x ) , | ||
| + | $$ | ||
| − | and many other important problems in number theory would have their final solution. However, problems concerning the distribution of the non-trivial zeros of a Dirichlet | + | and many other important problems in number theory would have their final solution. However, problems concerning the distribution of the non-trivial zeros of a Dirichlet $ L $- |
| + | function are exceptionally difficult, and relatively little is yet (1988) known on the subject. Stronger results were obtained for complex rather than for real characters. | ||
| − | A generalization of the method proposed in 1899 by de la Vallée-Poussin for the function | + | A generalization of the method proposed in 1899 by de la Vallée-Poussin for the function $ \zeta ( s) $ |
| + | yields a bound on the non-trivial zeros of $ L ( s, \chi ) $: | ||
| + | For a complex character $ \chi $ | ||
| + | $ \mathop{\rm mod} d $ | ||
| + | there exists an absolute constant $ C $ | ||
| + | such that $ L ( s , \chi ) $ | ||
| + | has no zeros in the domain | ||
| − | + | $$ | |
| + | \sigma > 1 - | ||
| + | \frac{C}{ \mathop{\rm ln} d ( | t | + 2 ) } | ||
| + | . | ||
| + | $$ | ||
| − | However, if | + | However, if $ \chi $ |
| + | is a real non-principal character modulo $ d $, | ||
| + | then $ L ( s , \chi ) $ | ||
| + | may have in this domain at most one simple real ( $ t= 0 $) | ||
| + | zero, known as the exceptional zero of $ L ( s , \chi ) $. | ||
| + | The following inequality was deduced for the exceptional zero $ \beta $ | ||
| + | from the analytic class number formula for quadratic fields: | ||
| − | + | $$ | |
| + | \beta \leq 1 - | ||
| + | \frac{C}{d ^ {1/2} \mathop{\rm ln} ^ {2} d } | ||
| + | . | ||
| + | $$ | ||
| − | A well-known best (pre 1975) bound for | + | A well-known best (pre 1975) bound for $ \beta $ |
| + | was obtained in 1935 by C.L. Siegel: For any $ \epsilon > 0 $ | ||
| + | there exists a positive number $ C ( \epsilon ) $ | ||
| + | such that | ||
| − | + | $$ | |
| + | \beta \leq 1 - C ( \epsilon ) d ^ {- \epsilon } . | ||
| + | $$ | ||
| − | However, this estimate has the major drawback of being ineffective in the sense that the knowledge of | + | However, this estimate has the major drawback of being ineffective in the sense that the knowledge of $ \epsilon $ |
| + | is insufficient to make an estimate for the numerical constant $ C ( \epsilon ) $. | ||
| + | This is also the disadvantage of the number-theoretic results based on Siegel's estimate. | ||
| − | From the above bounds for the non-trivial zeros of Dirichlet | + | From the above bounds for the non-trivial zeros of Dirichlet $ L $- |
| + | functions and formulas (6)–(8), the following asymptotic law for the distribution of prime numbers can be derived: | ||
| − | + | $$ | |
| + | \psi ( x ; d , l ) = | ||
| + | \frac{x}{\phi ( d) } | ||
| + | + O ( x \mathop{\rm exp} [ | ||
| + | - C _ {1} \sqrt { \mathop{\rm ln} x } ] ) . | ||
| + | $$ | ||
| − | Here | + | Here $ C _ {1} $ |
| + | is an effectively computable constant for $ d \leq ( \mathop{\rm ln} x ) ^ {1 - \gamma } $ | ||
| + | for some $ \gamma > 0 $. | ||
| + | Otherwise, one has $ C _ {1} = C _ {1} ( N) $ | ||
| + | ineffectively, where $ N > 0 $ | ||
| + | is such that $ d \leq ( \mathop{\rm ln} x ) ^ {N} $. | ||
| − | These results are the best results available in the problem of uniform distribution of prime numbers in arithmetic progressions with increasing difference | + | These results are the best results available in the problem of uniform distribution of prime numbers in arithmetic progressions with increasing difference $ d $. |
| + | A little more is known in the case where the value of $ d $ | ||
| + | is fixed. In such a case the theory of Dirichlet $ L $- | ||
| + | functions for $ t \neq 0 $ | ||
| + | resembles in many respects the theory of the Riemann zeta-function [[#References|[5]]], and the most recent bound on the zeros of $ L ( s , \chi ) $, | ||
| + | obtained by the [[Vinogradov method|Vinogradov method]] for estimating trigonometric sums, has the form: | ||
| − | + | $$ | |
| + | L ( s , \chi ) \neq 0 | ||
| + | $$ | ||
for | for | ||
| − | + | $$ | |
| + | \sigma > 1 - | ||
| + | \frac{C}{ \mathop{\rm ln} ^ {2/3} ( | t | + 2 ) \mathop{\rm ln} ^ {1/3} \mathop{\rm ln} ( | t | + 2 ) } | ||
| + | , | ||
| + | $$ | ||
| − | where | + | where $ C $ |
| + | is a positive constant depending on $ d $. | ||
| − | To this bound for the non-trivial zeros of Dirichlet | + | To this bound for the non-trivial zeros of Dirichlet $ L $- |
| + | functions modulo a fixed $ d $ | ||
| + | corresponds the best (1977) remainder term in the asymptotic formula for $ \psi ( x ; d, l) $: | ||
| − | + | $$ | |
| + | \ll x \mathop{\rm exp} [ - C \mathop{\rm ln} ^ {3/5} \ | ||
| + | x \mathop{\rm ln} ^ {1/5} \mathop{\rm ln} x ] . | ||
| + | $$ | ||
| − | All formulas concerning the asymptotics of the function | + | All formulas concerning the asymptotics of the function $ \psi ( x; d, l) $ |
| + | have analogues for the function $ \pi ( x; d, l) $, | ||
| + | viz. for the number of primes $ p \leq x $, | ||
| + | $ p \equiv l $( | ||
| + | $ \mathop{\rm mod} d $), | ||
| + | with principal term $ \mathop{\rm li} x/ \phi ( d) $ | ||
| + | instead of $ x/ \phi ( d) $ | ||
| + | and a residual term which is smaller by a factor $ \mathop{\rm ln} x $. | ||
| − | A major subject in modern studies on the theory of Dirichlet | + | A major subject in modern studies on the theory of Dirichlet $ L $- |
| + | functions is research on the density of the distribution of the non-trivial zeros of such functions. This research is concerned with giving estimates for the quantities | ||
| − | + | $$ | |
| + | N ( \sigma , T, \chi ) ,\ \sum _ {\chi \mathop{\rm mod} d } | ||
| + | N ( \sigma , T, \chi ) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \sum _ {d \leq D } \sum _ {\chi ^ {*} \mathop{\rm mod} d } N ( \sigma , T, \chi ) , | ||
| + | $$ | ||
| − | where | + | where $ N ( \sigma , T, \chi ) $ |
| + | denotes the number of zeros of $ L ( s, \chi ) $ | ||
| + | in the rectangle $ 0 < \alpha \leq \sigma < 1 $, | ||
| + | $ | t | < T $, | ||
| + | and $ \chi ^ {*} $ | ||
| + | is a primitive character $ \mathop{\rm mod} d $. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> P.G.L. Dirichlet, "Vorlesungen über Zahlentheorie" , Vieweg (1894)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> H. Davenport, "Multiplicative number theory" , Springer (1980)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> K. Prachar, "Primzahlverteilung" , Springer (1957)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.G. Chudakov, "Introductions to the theory of Dirichlet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d032/d032890/d032890177.png" />-functions" , Moscow-Leningrad (1947) (In Russian)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> A. Walfisz, "Weylsche Exponentialsummen in der neueren Zahlentheorie" , Deutsch. Verlag Wissenschaft. (1963)</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> H. Montgomery, "Topics in multiplicative number theory" , Springer (1971)</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> A.F. Lavrik, "Development of the method of density of zeros of Dirichlet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d032/d032890/d032890178.png" />-functions" ''Math. Notes'' , '''17''' : 5 (1975) pp. 483–488 ''Mat. Zametki'' , '''17''' : 5 (1975) pp. 809–817</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> P.G.L. Dirichlet, "Vorlesungen über Zahlentheorie" , Vieweg (1894)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> H. Davenport, "Multiplicative number theory" , Springer (1980)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> K. Prachar, "Primzahlverteilung" , Springer (1957)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.G. Chudakov, "Introductions to the theory of Dirichlet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d032/d032890/d032890177.png" />-functions" , Moscow-Leningrad (1947) (In Russian)</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> A. Walfisz, "Weylsche Exponentialsummen in der neueren Zahlentheorie" , Deutsch. Verlag Wissenschaft. (1963)</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> H. Montgomery, "Topics in multiplicative number theory" , Springer (1971)</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> A.F. Lavrik, "Development of the method of density of zeros of Dirichlet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d032/d032890/d032890178.png" />-functions" ''Math. Notes'' , '''17''' : 5 (1975) pp. 483–488 ''Mat. Zametki'' , '''17''' : 5 (1975) pp. 809–817</TD></TR></table> | ||
| + | ====Comments==== | ||
| + | The effective bound | ||
| + | |||
| + | $$ | ||
| + | \beta \leq 1 - | ||
| + | \frac{C}{d ^ {1/2} \mathop{\rm ln} ^ {2} d } | ||
| + | |||
| + | $$ | ||
| + | for the exceptional zero $ \beta $ | ||
| + | of $ L ( s , \chi ) $, | ||
| + | where $ \chi $ | ||
| + | is a real non-principal character $ \mathop{\rm mod} d $, | ||
| + | was improved by D. Goldfeld and A. Schinzel [[#References|[a1]]] to | ||
| − | + | $$ | |
| − | + | \beta \leq 1 - | |
| + | \frac{C \mathop{\rm ln} d }{d ^ {1/2} } | ||
| − | + | $$ | |
| − | for | + | for $ d > 0 $ |
| + | and | ||
| − | + | $$ | |
| + | \beta \leq 1 - | ||
| + | \frac{C}{d ^ {1/2} } | ||
| − | + | $$ | |
| − | < | + | for $ d < 0 $. |
| + | Here $ C $ | ||
| + | is an effectively computable constant. Using work of B.H. Gross and D. Zagier [[#References|[a2]]] the result for $ d < 0 $ | ||
| + | can be improved to | ||
| − | + | $$ | |
| + | \beta \leq 1 - | ||
| + | \frac{C ( \epsilon ) ( \mathop{\rm ln} d ) ^ {1 - \epsilon } }{d ^ {1/2} } | ||
| − | + | $$ | |
| − | for any | + | for any $ \epsilon > 0 $, |
| + | where $ C ( \epsilon ) $ | ||
| + | is an effective constant. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> D. Goldfeld, A. Schinzel, "On Siegel's zero" ''Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4)'' , '''2''' (1975) pp. 571–583</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> B.H. Gross, D. Zagier, "Heegner points and derivatives of | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> D. Goldfeld, A. Schinzel, "On Siegel's zero" ''Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4)'' , '''2''' (1975) pp. 571–583</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> B.H. Gross, D. Zagier, "Heegner points and derivatives of L-series" ''Invent. Math.'' , '''84''' (1986) pp. 225–320</TD></TR></table> |
Latest revision as of 11:50, 26 March 2023
Dirichlet $ L $-
series, $ L $-
series
A function of a complex variable $ s = \sigma + it $ that is defined for any Dirichlet character $ \chi $ $ \mathop{\rm mod} d $ by the series
$$ \tag{1 } L ( s , \chi ) = \sum _ {n = 1 } ^ \infty \frac{\chi ( n) }{n ^ {s} } . $$
As functions of a real variable these were introduced by P.G.L. Dirichlet [1] in 1837 in the context of the proof that the number of primes in an arithmetic progression $ \{ {dm + l } : {m = 0 , 1 ,\dots } \} $, where the difference $ d $ and the first term $ l $ are relatively prime numbers, is infinite. They are a natural generalization of the Riemann zeta-function $ \zeta ( s) $ to an arithmetic progression and are a powerful tool in analytic number theory [2]–[4].
The series (1), known as a Dirichlet series, converges absolutely and uniformly in any bounded domain in the complex $ s $- plane for which $ \sigma \geq 1 + \gamma $, $ \gamma > 0 $. If $ \chi $ is a non-principal character, one has
$$ \tag{2 } L ( s , \chi ) = s \int\limits _ { 1 } ^ \infty \sum _ {n \leq u } \chi ( n) u ^ {- s - 1 } d u . $$
Since the sum in the integrand is bounded, this formula gives an analytic continuation of $ L ( s , \chi ) $ to a regular function in the half-plane $ \sigma > 0 $.
For any $ \chi $ $ \mathop{\rm mod} d $ it is possible to represent $ L ( s , \chi ) $ as an Euler product over prime numbers $ p $:
$$ \tag{3 } L ( s , \chi ) = \prod _ { p } \left ( 1 - \frac{\chi ( p) }{p ^ {s} } \right ) ^ {-} 1 ,\ \sigma > 1 . $$
Hence, if $ \chi = \chi _ {0} $ is a principal character $ \mathop{\rm mod} d $, one has, for $ d = 1 $,
$$ L ( s , \chi _ {0} ) = \zeta ( s) , $$
and for $ d > 1 $,
$$ L ( s , \chi _ {0} ) = \zeta ( s) \prod _ {p \mid d } \left ( 1 - \frac{1}{p ^ {s} } \right ) . $$
For this reason the properties of $ L ( s , \chi _ {0} ) $ in the entire complex plane are mainly determined by the properties of $ \zeta ( s) $. In particular, the function $ L ( s , \chi _ {0} ) $ is regular for all $ s $, except for $ s = 1 $ where it has a simple pole with residue $ \phi ( d) / d $; here $ \phi $ is Euler's function. If, on the other hand, $ \chi \neq \chi _ {0} $ and if $ \chi ^ {*} $ is the primitive character inducing the character $ \chi $ $ \mathop{\rm mod} d $, then
$$ \tag{4 } L ( s , \chi ) = L ( s , \chi ^ {*} ) \prod _ {p \mid d } \left ( 1 - \frac{\chi ^ {*} ( p) }{p ^ {s} } \right ) . $$
Thus, it is no essential restriction to consider only Dirichlet $ L $- functions for primitive characters. This property of Dirichlet $ L $- functions is important, since many results concerning $ L ( s , \chi ) $ have a simple form for primitive characters only. If $ \chi $ $ \mathop{\rm mod} d $ is primitive, the analytic continuation to the entire plane and the functional equation for the function $ L ( s , \chi ) $ are obtained by direct generalization of Riemann's method for $ \zeta ( s) $. Putting
$$ \xi ( s , \chi ) = \left ( \frac{d} \pi \right ) ^ {( s + \delta ) / 2 } \Gamma \left ( \frac{s + \delta }{2} \right ) L ( s , \chi ) ,\ \delta = \frac{1 - \chi ( - 1 ) }{2} , $$
the result has the form
$$ \tag{5 } \xi ( 1 - s , \overline \chi \; ) = \epsilon ( \chi ) \xi ( s , \chi ) , $$
where $ \Gamma $ is the gamma-function, $ \epsilon ( \chi ) = i ^ \delta d ^ {1/2 } / \tau ( \chi ) $, $ | \epsilon ( \chi ) | = 1 $, $ \tau ( \chi ) $ is a Gauss sum, and $ \overline \chi \; $ is the complex conjugate character to $ \chi $. This equation is known as the functional equation of the function $ L( s, \chi ) $. It follows from this formula and from formulas (2) and (4) that the functions $ L( s, \chi ) $ and $ \xi ( x, \chi ) $ are entire functions for all $ \chi \neq \chi _ {0} $; if $ \sigma \leq 0 $, $ L( s, \chi ) = 0 $ only at the points $ s = - 2 \nu - \delta $, $ \nu = 0 , 1 \dots $ and at the points $ s $ where the product in (4) vanishes; these points are known as the trivial zeros of $ L ( s , \chi ) $. The remaining zeros of $ L ( s , \chi ) $ are said to be the non-trivial zeros. If $ \sigma > 1 $, then $ L ( s , \chi ) \neq 0 $. Ch.J. de la Vallée-Poussin showed that $ L ( 1 + it, \chi ) \neq 0 $, so that all non-trivial zeros of a Dirichlet $ L $- function lie in the domain $ 0 < \sigma < 1 $, which is known as the critical strip.
The distribution of the non-trivial zeros, and of the values of $ L ( s , \chi ) $ in the critical strip in general, is the most important problem in the theory of Dirichlet $ L $- functions, and is of fundamental importance in number theory.
That each function $ L ( s , \chi ) $ has infinitely many non-trivial zeros, and that the laws governing the distribution of primes in arithmetic progressions directly depend on the distribution of these zeros, is shown by the corresponding analogues of Riemann's formulas. In fact, let $ N ( T , \chi ) $ be the number of zeros of the function $ L ( s , \chi ) $ with a primitive character $ \chi $ $ \mathop{\rm mod} d $ in the rectangle $ 0 < \sigma < 1 $, $ | t | < T $, $ T \geq 2 $. Then
$$ \frac{N( T, \chi ) }{2} = \frac{T}{2 \pi } \mathop{\rm ln} \frac{Td}{2 \pi } - \frac{T}{2 \pi } + O( \mathop{\rm ln} Td ). $$
Let $ \Lambda ( n) $ be the von Mangoldt function, $ 1 \leq l \leq d $, $ ( l , d) = 1 $, and let
$$ \psi ( x ; d , l) = \sum _ {\begin{array}{c} n \leq x , \\ n \equiv l ( \mathop{\rm mod} d ) \end{array} } \Lambda ( n) , $$
$$ \psi ( x ; \chi ) = \sum _ {n \leq x } \chi ( n) \Lambda ( n) . $$
Then it follows from the orthogonality property of the characters that
$$ \tag{6 } \psi ( x ; d , l) = \frac{1}{\phi ( d) } \sum _ {\chi \mathop{\rm mod} \ d } \overline \chi \; ( l) \psi ( x ; \chi ) , $$
where the summation is extended over all characters $ \chi $ $ \mathop{\rm mod} d $. Moreover, for a primitive character $ \chi $ and for $ \alpha = 1 - \delta $:
$$ \psi ( x ; \chi ) = - \sum _ \rho \frac{x ^ \rho } \rho + \sum _ { m= } 1 ^ \infty \frac{x ^ {\delta - 2m } }{2m - \delta } + $$
$$ - \lim\limits _ {s \rightarrow 0 } \left \{ \frac{L ^ \prime ( \alpha s , \chi ) }{L ( \alpha s , \chi ) } - \frac \alpha {s} \right \} - \alpha \mathop{\rm ln} x , $$
where $ \rho = \beta + i \gamma $ runs through the non-trivial zeros of $ L ( s , \chi ) $, and $ L ^ \prime $ is the derivative of $ L $ with respect to $ s $.
Approximate formulas for $ \psi ( x ; \chi ) $ are more useful in practice: For arbitrary $ \chi \neq \chi _ {0} $ and for $ 2 \leq T \leq x $ one has
$$ \tag{7 } \psi ( x ; \chi ) = - \sum _ {| \gamma | < T } \frac{x ^ \rho } \rho + \sum _ {| \gamma | < 1 } \frac{1} \rho + O \left ( \frac{x}{T} \mathop{\rm ln} ^ {2} xd \right ) , $$
and for $ \chi = \chi _ {0} $,
$$ \tag{8 } \psi ( x ; \chi _ {0} ) = \sum _ {n \leq x } \Lambda ( n) + O ( \mathop{\rm ln} x \mathop{\rm ln} d ) . $$
The quantity in (8) is the principal term of the sum in (6).
According to the so-called extended Riemann hypothesis, all non-trivial zeros of a Dirichlet $ L $- function lie on the straight line $ \sigma = 1/2 $. If this hypothesis is valid, one has, for $ d \leq x $,
$$ \psi ( x ; d, l ) = \frac{x}{\phi ( d) } + O ( \sqrt x \mathop{\rm ln} ^ {2} x ) , $$
and many other important problems in number theory would have their final solution. However, problems concerning the distribution of the non-trivial zeros of a Dirichlet $ L $- function are exceptionally difficult, and relatively little is yet (1988) known on the subject. Stronger results were obtained for complex rather than for real characters.
A generalization of the method proposed in 1899 by de la Vallée-Poussin for the function $ \zeta ( s) $ yields a bound on the non-trivial zeros of $ L ( s, \chi ) $: For a complex character $ \chi $ $ \mathop{\rm mod} d $ there exists an absolute constant $ C $ such that $ L ( s , \chi ) $ has no zeros in the domain
$$ \sigma > 1 - \frac{C}{ \mathop{\rm ln} d ( | t | + 2 ) } . $$
However, if $ \chi $ is a real non-principal character modulo $ d $, then $ L ( s , \chi ) $ may have in this domain at most one simple real ( $ t= 0 $) zero, known as the exceptional zero of $ L ( s , \chi ) $. The following inequality was deduced for the exceptional zero $ \beta $ from the analytic class number formula for quadratic fields:
$$ \beta \leq 1 - \frac{C}{d ^ {1/2} \mathop{\rm ln} ^ {2} d } . $$
A well-known best (pre 1975) bound for $ \beta $ was obtained in 1935 by C.L. Siegel: For any $ \epsilon > 0 $ there exists a positive number $ C ( \epsilon ) $ such that
$$ \beta \leq 1 - C ( \epsilon ) d ^ {- \epsilon } . $$
However, this estimate has the major drawback of being ineffective in the sense that the knowledge of $ \epsilon $ is insufficient to make an estimate for the numerical constant $ C ( \epsilon ) $. This is also the disadvantage of the number-theoretic results based on Siegel's estimate.
From the above bounds for the non-trivial zeros of Dirichlet $ L $- functions and formulas (6)–(8), the following asymptotic law for the distribution of prime numbers can be derived:
$$ \psi ( x ; d , l ) = \frac{x}{\phi ( d) } + O ( x \mathop{\rm exp} [ - C _ {1} \sqrt { \mathop{\rm ln} x } ] ) . $$
Here $ C _ {1} $ is an effectively computable constant for $ d \leq ( \mathop{\rm ln} x ) ^ {1 - \gamma } $ for some $ \gamma > 0 $. Otherwise, one has $ C _ {1} = C _ {1} ( N) $ ineffectively, where $ N > 0 $ is such that $ d \leq ( \mathop{\rm ln} x ) ^ {N} $.
These results are the best results available in the problem of uniform distribution of prime numbers in arithmetic progressions with increasing difference $ d $. A little more is known in the case where the value of $ d $ is fixed. In such a case the theory of Dirichlet $ L $- functions for $ t \neq 0 $ resembles in many respects the theory of the Riemann zeta-function [5], and the most recent bound on the zeros of $ L ( s , \chi ) $, obtained by the Vinogradov method for estimating trigonometric sums, has the form:
$$ L ( s , \chi ) \neq 0 $$
for
$$ \sigma > 1 - \frac{C}{ \mathop{\rm ln} ^ {2/3} ( | t | + 2 ) \mathop{\rm ln} ^ {1/3} \mathop{\rm ln} ( | t | + 2 ) } , $$
where $ C $ is a positive constant depending on $ d $.
To this bound for the non-trivial zeros of Dirichlet $ L $- functions modulo a fixed $ d $ corresponds the best (1977) remainder term in the asymptotic formula for $ \psi ( x ; d, l) $:
$$ \ll x \mathop{\rm exp} [ - C \mathop{\rm ln} ^ {3/5} \ x \mathop{\rm ln} ^ {1/5} \mathop{\rm ln} x ] . $$
All formulas concerning the asymptotics of the function $ \psi ( x; d, l) $ have analogues for the function $ \pi ( x; d, l) $, viz. for the number of primes $ p \leq x $, $ p \equiv l $( $ \mathop{\rm mod} d $), with principal term $ \mathop{\rm li} x/ \phi ( d) $ instead of $ x/ \phi ( d) $ and a residual term which is smaller by a factor $ \mathop{\rm ln} x $.
A major subject in modern studies on the theory of Dirichlet $ L $- functions is research on the density of the distribution of the non-trivial zeros of such functions. This research is concerned with giving estimates for the quantities
$$ N ( \sigma , T, \chi ) ,\ \sum _ {\chi \mathop{\rm mod} d } N ( \sigma , T, \chi ) , $$
$$ \sum _ {d \leq D } \sum _ {\chi ^ {*} \mathop{\rm mod} d } N ( \sigma , T, \chi ) , $$
where $ N ( \sigma , T, \chi ) $ denotes the number of zeros of $ L ( s, \chi ) $ in the rectangle $ 0 < \alpha \leq \sigma < 1 $, $ | t | < T $, and $ \chi ^ {*} $ is a primitive character $ \mathop{\rm mod} d $.
References
| [1] | P.G.L. Dirichlet, "Vorlesungen über Zahlentheorie" , Vieweg (1894) |
| [2] | H. Davenport, "Multiplicative number theory" , Springer (1980) |
| [3] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
| [4] | N.G. Chudakov, "Introductions to the theory of Dirichlet 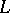 -functions" , Moscow-Leningrad (1947) (In Russian) -functions" , Moscow-Leningrad (1947) (In Russian) |
| [5] | A. Walfisz, "Weylsche Exponentialsummen in der neueren Zahlentheorie" , Deutsch. Verlag Wissenschaft. (1963) |
| [6] | H. Montgomery, "Topics in multiplicative number theory" , Springer (1971) |
| [7] | A.F. Lavrik, "Development of the method of density of zeros of Dirichlet 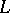 -functions" Math. Notes , 17 : 5 (1975) pp. 483–488 Mat. Zametki , 17 : 5 (1975) pp. 809–817 -functions" Math. Notes , 17 : 5 (1975) pp. 483–488 Mat. Zametki , 17 : 5 (1975) pp. 809–817 |
Comments
The effective bound
$$ \beta \leq 1 - \frac{C}{d ^ {1/2} \mathop{\rm ln} ^ {2} d } $$
for the exceptional zero $ \beta $ of $ L ( s , \chi ) $, where $ \chi $ is a real non-principal character $ \mathop{\rm mod} d $, was improved by D. Goldfeld and A. Schinzel [a1] to
$$ \beta \leq 1 - \frac{C \mathop{\rm ln} d }{d ^ {1/2} } $$
for $ d > 0 $ and
$$ \beta \leq 1 - \frac{C}{d ^ {1/2} } $$
for $ d < 0 $. Here $ C $ is an effectively computable constant. Using work of B.H. Gross and D. Zagier [a2] the result for $ d < 0 $ can be improved to
$$ \beta \leq 1 - \frac{C ( \epsilon ) ( \mathop{\rm ln} d ) ^ {1 - \epsilon } }{d ^ {1/2} } $$
for any $ \epsilon > 0 $, where $ C ( \epsilon ) $ is an effective constant.
References
| [a1] | D. Goldfeld, A. Schinzel, "On Siegel's zero" Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) , 2 (1975) pp. 571–583 |
| [a2] | B.H. Gross, D. Zagier, "Heegner points and derivatives of L-series" Invent. Math. , 84 (1986) pp. 225–320 |
Dirichlet L-function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dirichlet_L-function&oldid=36155