Difference between revisions of "Semi-group of operators"
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
(→References: zbl link) |
||
| (3 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | s0841401.png | ||
| + | $#A+1 = 469 n = 2 | ||
| + | $#C+1 = 469 : ~/encyclopedia/old_files/data/S084/S.0804140 Semi\AAhgroup of operators | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | If | + | A family $ \{ T \} $ |
| + | of operators on a [[Banach space|Banach space]] or [[Topological vector space|topological vector space]] with the property that the composite of any two operators in the family is again a member of the family. If the operators $ T $ | ||
| + | are "indexed" by elements of some abstract [[Semi-group|semi-group]] $ \mathfrak A $ | ||
| + | and the binary operation of the latter is compatible with the composition of operators, $ \{ T \} $ | ||
| + | is known as a representation of the semi-group $ \mathfrak A $. | ||
| + | The most detailed attention has been given to one-parameter semi-groups (cf. [[One-parameter semi-group|One-parameter semi-group]]) of bounded linear operators on a Banach space $ X $, | ||
| + | which yield a representation of the additive semi-group of all positive real numbers, i.e. families $ T ( t) $ | ||
| + | with the property | ||
| + | |||
| + | $$ | ||
| + | T ( t + \tau ) x = T ( t) T ( \tau ) x ,\ t , \tau > 0 ,\ x \in X . | ||
| + | $$ | ||
| + | |||
| + | If $ T ( t) $ | ||
| + | is strongly measurable, $ t > 0 $, | ||
| + | then $ T ( t) $ | ||
| + | is a [[Strongly-continuous semi-group|strongly-continuous semi-group]]; this will be assumed in the sequel. | ||
The limit | The limit | ||
| − | + | $$ | |
| + | \omega = \lim\limits _ {t \rightarrow \infty } \ | ||
| + | t ^ {-1} \mathop{\rm ln} \| T ( t) \| | ||
| + | $$ | ||
| − | exists; it is known as the type of the semi-group. The functions | + | exists; it is known as the type of the semi-group. The functions $ T ( t) x $ |
| + | increase at most exponentially. | ||
An important characteristic is the infinitesimal operator (infinitesimal generator) of the semi-group: | An important characteristic is the infinitesimal operator (infinitesimal generator) of the semi-group: | ||
| − | + | $$ | |
| + | A _ {0} x = \lim\limits _ {t \rightarrow 0 } t ^ {-1} [ T ( t) x - x ] , | ||
| + | $$ | ||
| − | defined on the linear set | + | defined on the linear set $ D ( A _ {0} ) $ |
| + | of all elements $ x $ | ||
| + | for which the limit exists; the closure, $ A $, | ||
| + | of this operator (if it exists) is known as the generating operator, or generator, of the semi-group. Let $ X _ {0} $ | ||
| + | be the subspace defined as the closure of the union of all values $ T ( t) x $; | ||
| + | then $ D ( A _ {0} ) $ | ||
| + | is dense in $ X _ {0} $. | ||
| + | If there are no non-zero elements in $ X _ {0} $ | ||
| + | such that $ T ( t) x \equiv 0 $, | ||
| + | then the generating operator $ A $ | ||
| + | exists. In the sequel it will be assumed that $ X _ {0} = X $ | ||
| + | and that $ T ( t) x \equiv 0 $ | ||
| + | implies $ x = 0 $. | ||
| − | The simplest class of semi-groups, denoted by | + | The simplest class of semi-groups, denoted by $ C _ {0} $, |
| + | is defined by the condition: $ T ( t) x \rightarrow x $ | ||
| + | as $ t \rightarrow 0 $ | ||
| + | for any $ x \in X $. | ||
| + | This is equivalent to the condition: The function $ \| T ( t) \| $ | ||
| + | is bounded on any interval $ ( 0 , a ] $. | ||
| + | In that case $ T ( t) $ | ||
| + | has a generating operator $ A = A _ {0} $ | ||
| + | whose [[Resolvent|resolvent]] $ R ( \lambda , A ) = ( A - \lambda I ) ^ {-1} $ | ||
| + | satisfies the inequalities | ||
| − | + | $$ \tag{1 } | |
| + | \| R ^ {n} ( \lambda , A ) \| \leq M ( \lambda - \omega ) ^ {-} n ,\ \ | ||
| + | n = 1 , 2 , . . . ; \ \lambda > \omega , | ||
| + | $$ | ||
| − | where | + | where $ \omega $ |
| + | is the type of the semi-group. Conversely, if $ A $ | ||
| + | is a [[Closed operator|closed operator]] with domain of definition dense in $ X $ | ||
| + | and with a resolvent satisfying (1), then it is the generating operator of some semi-group $ T ( t) $ | ||
| + | of class $ C _ {0} $ | ||
| + | such that $ \| T ( t) \| \leq M e ^ {\omega t } $. | ||
| + | Condition (1) is satisfied if | ||
| − | + | $$ | |
| + | \| R ( \lambda , A ) \| \leq ( \lambda - \omega ) ^ {-1} | ||
| + | $$ | ||
| − | (the Hill–Yosida condition). If, moreover, | + | (the Hill–Yosida condition). If, moreover, $ \omega = 0 $, |
| + | then $ T ( t) $ | ||
| + | is a [[Contraction semi-group|contraction semi-group]]: $ \| T ( t) \| \leq 1 $. | ||
| − | A summable semi-group is a semi-group for which the functions | + | A summable semi-group is a semi-group for which the functions $ \| T ( t) x \| $ |
| + | are summable on any finite interval for all $ x \in X $. | ||
| + | A summable semi-group has a generating operator $ A = \overline{ {A _ {0} }}\; $. | ||
| + | The operator $ A _ {0} $ | ||
| + | is closed if and only if, for every $ x \in X $, | ||
| − | + | $$ | |
| + | \lim\limits _ {t \rightarrow 0 } | ||
| + | \frac{1}{t} | ||
| + | \int\limits _ { 0 } ^ { t } T ( s) x d s = x . | ||
| + | $$ | ||
| − | For | + | For $ \mathop{\rm Re} \lambda > \omega $ |
| + | one can define the Laplace transform of a summable semi-group, | ||
| − | + | $$ \tag{2 } | |
| + | \int\limits _ { 0 } ^ \infty e ^ {- \lambda t } T ( t) | ||
| + | x d t = - R ( \lambda ) x , | ||
| + | $$ | ||
| − | giving a bounded linear operator | + | giving a bounded linear operator $ R ( \lambda ) $ |
| + | which has many properties of a resolvent operator. | ||
| − | A closed operator | + | A closed operator $ A $ |
| + | with domain of definition dense in $ X $ | ||
| + | is the generating operator of a summable semi-group $ T ( t) $ | ||
| + | if and only if, for some $ \omega $, | ||
| + | the resolvent $ R ( \lambda , A ) $ | ||
| + | exists for $ \mathop{\rm Re} \lambda > \omega $ | ||
| + | and the following conditions hold: a) $ \| R ( \lambda , A ) \| \leq M $, | ||
| + | $ \mathop{\rm Re} \lambda > \omega $; | ||
| + | b) there exist a non-negative function $ \phi ( t , x ) $, | ||
| + | $ t > 0 $, | ||
| + | $ x \in X $, | ||
| + | jointly continuous in all its variables, and a non-negative function $ \phi ( t) $, | ||
| + | bounded on any interval $ [ a , b ] \subset ( 0 , \infty ) $, | ||
| + | such that, for $ \omega _ {1} > \omega $, | ||
| − | + | $$ | |
| + | \int\limits _ { 0 } ^ \infty e ^ {- \omega _ {1} t } \phi ( t , x ) dt < \infty , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \overline{\lim\limits}\; _ {t \rightarrow \infty } t ^ {-1} \mathop{\rm ln} \phi ( t) < \infty | ||
| + | ,\ \phi ( t , x ) \leq \phi ( t) \| x \| , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \| R ^ {n} ( \lambda , A ) x \| \leq | ||
| + | \frac{1}{ | ||
| + | ( n - 1 ) ! } | ||
| + | \int\limits _ { 0 } ^ \infty t ^ {n-1} e ^ {- \lambda t } \phi ( t , x ) dt . | ||
| + | $$ | ||
Under these conditions | Under these conditions | ||
| − | + | $$ | |
| + | \| T ( t) x \| \leq \phi ( t , x ) ,\ \ | ||
| + | \| T ( t) \| \leq \phi ( t) . | ||
| + | $$ | ||
| − | If one requires in addition that the function | + | If one requires in addition that the function $ \| T ( t) \| $ |
| + | be summable on finite intervals, a necessary and sufficient condition is the existence of a continuous function $ \phi ( t) $ | ||
| + | such that, for $ \omega _ {1} > \omega $, | ||
| − | + | $$ \tag{3 } | |
| + | \int\limits _ { 0 } ^ \infty \phi ( t) e ^ {- \omega _ {1} t } dt < \infty , | ||
| + | $$ | ||
| − | + | $$ \tag{4 } | |
| + | \| R ^ {n} ( \lambda , A ) \| \leq | ||
| + | \frac{1}{( n - 1 | ||
| + | ) ! } | ||
| + | \int\limits _ { 0 } ^ \infty t ^ {n-1} e ^ {- \lambda t } \phi ( t) dt , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \lambda > \omega ,\ n= 1 , 2 , . . . . | ||
| + | $$ | ||
| − | Under these conditions, | + | Under these conditions, $ \| T ( t) \| \leq \phi ( t) $. |
| + | By choosing different functions satisfying (3), one can define different subclasses of summable semi-groups. If $ \phi ( t) = Me ^ {\omega t } $, | ||
| + | the result is the class $ C _ {0} $ | ||
| + | and (1) follows from (4). If $ \phi ( t) = Mt ^ {- \alpha } e ^ {\omega t } $, | ||
| + | $ 0 \leq \alpha < 1 $, | ||
| + | condition (4) implies the condition | ||
| − | + | $$ | |
| + | \| R ^ {n} ( \lambda , A ) \| \leq | ||
| + | \frac{M \Gamma ( n - \alpha ) }{( n - 1 ) ! ( \lambda - \omega ) ^ {n - \alpha } } | ||
| + | ,\ \ | ||
| + | \lambda > \omega ,\ n = 1 , 2 ,\dots. | ||
| + | $$ | ||
==Semi-groups with power singularities.== | ==Semi-groups with power singularities.== | ||
| − | If in the previous example | + | If in the previous example $ \alpha \geq 1 $, |
| + | then the integrals in (4) are divergent for $ n \leq \alpha - 1 $. | ||
| + | Hence the generating operator for the corresponding semi-group may not have a resolvent for any $ \lambda $, | ||
| + | i.e. it may have a spectrum equal to the entire complex plane. However, for $ n $ | ||
| + | large enough one can define for such operators functions $ S _ {n} ( \lambda , A ) $ | ||
| + | which coincide with the functions $ R ^ {n+1} ( \lambda , A ) $ | ||
| + | in the previous cases. The operator function $ S _ {n} ( \lambda , A ) $ | ||
| + | is called a resolvent of order $ n $ | ||
| + | if it is analytic in some domain $ G \subset \mathbf C $ | ||
| + | and if for $ \lambda \in G $, | ||
| + | |||
| + | $$ | ||
| + | S _ {n} ( \lambda , A ) Ax = A S _ {n} ( \lambda , A ) x ,\ \ | ||
| + | x \in D ( A) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | S _ {n} ( \lambda , A ) ( A - \lambda ) ^ {n+1} x = x ,\ x \in D ( A ^ {n+1} ) , | ||
| + | $$ | ||
| − | + | and if $ S _ {n} ( \lambda , A ) x = 0 $ | |
| + | for all $ \lambda \in G $ | ||
| + | implies $ x = 0 $. | ||
| + | If $ \overline{D}\; ( A ^ {n+1} ) = X $, | ||
| + | the operator may have a unique resolvent of order $ n $, | ||
| + | for which there is a maximal domain of analyticity, known as the resolvent set of order $ n $. | ||
| − | + | Let $ T ( t) $ | |
| + | be a strongly-continuous semi-group such that the inequality | ||
| − | + | $$ | |
| + | \| T ( t) \| \leq M t ^ {- \alpha } e ^ {\omega t } | ||
| + | $$ | ||
| − | + | holds for $ \alpha \geq 1 $. | |
| + | Then its generating operator $ B $ | ||
| + | has a resolvent of order $ n $ | ||
| + | for $ n > \alpha - 1 $, | ||
| + | and, moreover, | ||
| − | + | $$ | |
| + | S _ {n} ( \lambda , B ) x = \ | ||
| − | + | \frac{1}{n!} | |
| + | \int\limits _ { 0 } ^ \infty t ^ {n} e ^ {- \lambda t } T | ||
| + | ( t) x dt ,\ \mathop{\rm Re} \lambda > \omega , | ||
| + | $$ | ||
| − | + | $$ \tag{5 } | |
| + | \left \| | ||
| + | \frac{d ^ {k} S _ {n} ( \lambda , B ) }{d \lambda ^ {k} } | ||
| + | x \right \| \leq | ||
| + | \frac{M \Gamma ( k + n + 1 - \alpha ) }{n | ||
| + | ! ( \mathop{\rm Re} \lambda - \omega ) ^ {k + n + 1 - \alpha } } | ||
| + | , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Re} \lambda > \omega ,\ k = 0 , 1 ,\dots . | ||
| + | $$ | ||
| − | Conversely, suppose that for | + | Conversely, suppose that for $ \mathop{\rm Re} \lambda > 0 $ |
| + | the operator $ B $ | ||
| + | has a resolvent $ S _ {n} ( \lambda , B ) $ | ||
| + | of order $ n $ | ||
| + | satisfying (5) with $ n > \alpha - 1 $. | ||
| + | Then there exists a unique semi-group $ T ( t) $ | ||
| + | such that | ||
| − | + | $$ | |
| + | \| T ( t) \| \leq M t ^ {- \alpha } e ^ {\omega t } , | ||
| + | $$ | ||
| − | and the generating operator | + | and the generating operator $ A $ |
| + | of this semi-group is such that $ S _ {n} ( \lambda , A ) = S _ {n} ( \lambda , B ) $. | ||
==Smooth semi-groups.== | ==Smooth semi-groups.== | ||
| − | If | + | If $ x \in D ( A _ {0} ) $, |
| + | the function $ T ( t) x $ | ||
| + | is continuously differentiable and | ||
| + | |||
| + | $$ | ||
| − | + | \frac{d T ( t) }{dt} | |
| + | x = A _ {0} T | ||
| + | ( t) x = T ( t) A _ {0} x . | ||
| + | $$ | ||
| − | There exist semi-groups of class | + | There exist semi-groups of class $ C _ {0} $ |
| + | such that, if $ x \notin D ( A _ {0} ) = D ( A) $, | ||
| + | the functions $ T ( t) x $ | ||
| + | are non-differentiable for all $ t $. | ||
| + | However, there are important classes of semi-groups for which the degree of smoothness increases with increasing $ t $. | ||
| + | If the functions $ T ( t) x $, | ||
| + | $ t > t _ {0} $, | ||
| + | are differentiable for any $ x \in X $, | ||
| + | then it follows from the semi-group property that the $ T ( t) x $ | ||
| + | are twice differentiable if $ t > 2 t _ {0} $, | ||
| + | three times differentiable if $ t > 3 t _ {0} $, | ||
| + | etc. Therefore, if these functions are differentiable at any $ t > 0 $ | ||
| + | for $ x \in X $, | ||
| + | then $ T ( t) x $ | ||
| + | is infinitely differentiable. | ||
| − | Given a semi-group of class | + | Given a semi-group of class $ C _ {0} $, |
| + | a necessary and sufficient condition for the functions $ T ( t) x $ | ||
| + | to be differentiable for all $ x \in X $ | ||
| + | and $ t > t _ {0} $, | ||
| + | where $ t _ {0} \geq 0 $, | ||
| + | is that there exist numbers $ a , b , c > 0 $ | ||
| + | such that the resolvent $ R ( \lambda , A ) $ | ||
| + | is defined in the domain | ||
| − | + | $$ | |
| + | \mathop{\rm Re} \lambda > a - b \mathop{\rm ln} | \mathop{\rm Im} \lambda | , | ||
| + | $$ | ||
while in this domain | while in this domain | ||
| − | + | $$ | |
| + | \| R( \lambda , A ) \| \leq c | \mathop{\rm Im} \lambda | . | ||
| + | $$ | ||
| − | A necessary and sufficient condition for | + | A necessary and sufficient condition for $ T ( t) x $ |
| + | to be infinitely differentiable for all $ x \in X $ | ||
| + | and $ t > 0 $ | ||
| + | is that, for every $ b > 0 $, | ||
| + | there exist $ a _ {b} , c _ {b} $ | ||
| + | such that the resolvent $ R ( \lambda , A ) $ | ||
| + | is defined in the domain | ||
| − | + | $$ | |
| + | \mathop{\rm Re} \lambda > a _ {b} - b \mathop{\rm ln} | \mathop{\rm Im} \lambda | , | ||
| + | $$ | ||
and such that | and such that | ||
| − | + | $$ | |
| + | \| R ( \lambda , A ) \| \leq c _ {b} | \mathop{\rm Im} \lambda | . | ||
| + | $$ | ||
| − | Sufficient conditions are: If there exists a | + | Sufficient conditions are: If there exists a $ \mu > \omega $ |
| + | for which | ||
| − | + | $$ | |
| + | \overline{\lim\limits}\; _ {\tau \rightarrow \infty } \mathop{\rm ln} | \tau | \| R ( \mu + i \tau , A ) \| = t _ {0} < \infty , | ||
| + | $$ | ||
| − | then the | + | then the $ T ( t) x $ |
| + | are differentiable for $ t > t _ {0} $ | ||
| + | and $ x \in X $; | ||
| + | if $ t _ {0} = 0 $, | ||
| + | then the $ T ( t) x $ | ||
| + | are infinitely differentiable for all $ t > 0 $ | ||
| + | and $ x \in X $. | ||
| − | The degree of smoothness of a semi-group may sometimes be inferred from its behaviour at zero; for example, suppose that for every | + | The degree of smoothness of a semi-group may sometimes be inferred from its behaviour at zero; for example, suppose that for every $ c > 0 $ |
| + | there exists a $ \delta _ {c} $ | ||
| + | such that, for $ 0 < t < \delta _ {c} $, | ||
| − | + | $$ | |
| + | \| I - T ( t) \| \leq 2 - ct \mathop{\rm ln} t ^ {-1} , | ||
| + | $$ | ||
| − | then the | + | then the $ T ( t) x $ |
| + | are infinitely differentiable for all $ t > 0 $, | ||
| + | $ x \in X $. | ||
| − | There are smoothness conditions for summable semi-groups and semi-groups of polynomial growth. If a semi-group has polynomial growth of degree | + | There are smoothness conditions for summable semi-groups and semi-groups of polynomial growth. If a semi-group has polynomial growth of degree $ \alpha $ |
| + | and is infinitely differentiable for $ t > 0 $, | ||
| + | then the function | ||
| − | + | $$ | |
| + | |||
| + | \frac{d T ( t) }{dt} | ||
| + | x = A T ( t) x | ||
| + | $$ | ||
also has polynomial growth: | also has polynomial growth: | ||
| − | + | $$ | |
| + | \| A T ( t) \| \leq M _ {1} t ^ {- \beta } e ^ {\omega t } . | ||
| + | $$ | ||
| − | In the general case there is no rigorous relationship between the numbers | + | In the general case there is no rigorous relationship between the numbers $ \alpha $ |
| + | and $ \beta $, | ||
| + | and $ \beta $ | ||
| + | can be utilized for a more detailed classification of infinitely-differentiable semi-groups of polynomial growth. | ||
==Analytic semi-groups.== | ==Analytic semi-groups.== | ||
| − | An important class of semi-groups, related to partial differential equations of parabolic type, comprises those semi-groups | + | An important class of semi-groups, related to partial differential equations of parabolic type, comprises those semi-groups $ T ( t) $ |
| + | which admit an analytic continuation to some sector of the complex plane containing the positive real axis. A semi-group of class $ C _ {0} $ | ||
| + | has this property if and only if its resolvent satisfies the following inequality in some right half-plane $ \mathop{\rm Re} \lambda > \omega $: | ||
| − | + | $$ | |
| + | \| R ( \lambda , A ) \| \leq M | \lambda - \omega | ^ {-1} . | ||
| + | $$ | ||
Another necessary and sufficient conditions is: The semi-group is strongly differentiable and its derivative satisfies the estimate | Another necessary and sufficient conditions is: The semi-group is strongly differentiable and its derivative satisfies the estimate | ||
| − | + | $$ | |
| + | \left \| | ||
| + | \frac{d T }{dt} | ||
| + | ( t) \right \| \leq M t ^ {-1} e ^ {\omega t } . | ||
| + | $$ | ||
Finally, the inequality | Finally, the inequality | ||
| − | + | $$ | |
| + | \overline{\lim\limits}\; _ {t \rightarrow 0 } \| I - T ( t) \| < 2 | ||
| + | $$ | ||
| − | is also a sufficient condition for | + | is also a sufficient condition for $ T ( t) $ |
| + | to be analytic. | ||
| − | If a semi-group | + | If a semi-group $ T ( t) $ |
| + | has an analytic continuation $ T ( z) $ | ||
| + | to a sector $ | \mathop{\rm arg} z | < \phi \leq \pi / 2 $ | ||
| + | and has polynomial growth at zero, $ \| T ( z) \| \leq c | z | ^ \alpha $, | ||
| + | $ \alpha > 0 $, | ||
| + | then the resolvent $ S _ {n} ( \lambda , A ) $ | ||
| + | of order $ n > \alpha - 1 $ | ||
| + | of its generating operator $ A $ | ||
| + | has an analytic continuation to the sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \phi $, | ||
| + | and satisfies the following estimate in any sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \psi $, | ||
| + | $ \psi < \phi $: | ||
| − | + | $$ | |
| + | \| S _ {n} ( \lambda , A ) \| \leq | \lambda | ^ | ||
| + | {\alpha - n - 1 } M ( \psi ) . | ||
| + | $$ | ||
| − | Conversely, suppose that the resolvent | + | Conversely, suppose that the resolvent $ S _ {n} ( \lambda , B ) $ |
| + | of an operator $ B $ | ||
| + | is defined in a sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \psi $ | ||
| + | and that | ||
| − | + | $$ | |
| + | \| S _ {n} ( \lambda , B ) \| \leq \lambda ^ {\alpha - n - 1 } M . | ||
| + | $$ | ||
| − | Then there exists a semi-group | + | Then there exists a semi-group $ T ( z) $ |
| + | of growth $ \alpha $, | ||
| + | analytic in the sector $ | \mathop{\rm arg} z | < \psi $, | ||
| + | whose generating operator $ A $ | ||
| + | is such that $ S _ {n} ( \lambda , A ) = S _ {n} ( \lambda , B ) $. | ||
==Distribution semi-groups.== | ==Distribution semi-groups.== | ||
| − | In accordance with the general concept of the theory of distributions (cf. [[Generalized function|Generalized function]]), one can drop the requirement that the operator-valued function | + | In accordance with the general concept of the theory of distributions (cf. [[Generalized function|Generalized function]]), one can drop the requirement that the operator-valued function $ T ( t) $ |
| + | be defined for every $ t > 0 $, | ||
| + | demanding only that it be possible to evaluate the integrals $ \int _ {- \infty } ^ {+ \infty } T ( t) \phi ( t) dt $ | ||
| + | for all $ \phi $ | ||
| + | in the space $ D ( \mathbf R ) $ | ||
| + | of infinitely-differentiable functions with compact support. Hence the following definition: A distribution semi-group on a Banach space $ X $ | ||
| + | is a continuous linear mapping $ T ( \phi ) $ | ||
| + | of $ D ( \mathbf R ) $ | ||
| + | into the space $ L ( X) $ | ||
| + | of all bounded linear operators on $ X $, | ||
| + | with the following properties: a) $ T ( \phi ) = 0 $ | ||
| + | if $ \supp \phi \subset ( - \infty , 0 ) $; | ||
| + | b) if $ \phi , \psi $ | ||
| + | are functions in the subspace $ D ^ + ( \mathbf R ) $ | ||
| + | of all functions in $ D ( \mathbf R ) $ | ||
| + | with support in $ ( 0 , \infty ) $, | ||
| + | then $ T ( \phi * \psi ) = T ( \phi ) T ( \psi ) $, | ||
| + | where the star denotes convolution: | ||
| − | + | $$ | |
| + | \phi * \psi = \int\limits _ {- \infty } ^ \infty \phi ( t - s ) \psi ( s) d s | ||
| + | $$ | ||
| − | (the semi-group property); c) if | + | (the semi-group property); c) if $ T ( \phi ) x = 0 $ |
| + | for all $ \phi \in D ^ + ( \mathbf R ) $, | ||
| + | then $ x = 0 $; | ||
| + | d) the linear hull of the set of all values of $ T ( \phi ) x $, | ||
| + | $ \phi \in D ^ + ( \mathbf R ) $, | ||
| + | $ x \in X $, | ||
| + | is dense in $ X $; | ||
| + | e) for any $ y = T ( \psi ) x $, | ||
| + | $ \psi \in D ^ + ( \mathbf R ) $, | ||
| + | there exists a continuous $ u ( t) $ | ||
| + | on $ ( 0 , \infty ) $ | ||
| + | with values in $ X $, | ||
| + | so that $ u( 0) = y $ | ||
| + | and | ||
| − | + | $$ | |
| + | T ( \phi ) y = \int\limits _ { 0 } ^ \infty \phi ( t) u ( t) dt | ||
| + | $$ | ||
| − | for all | + | for all $ \phi \in D ( \mathbf R ) $. |
| − | The infinitesimal operator | + | The infinitesimal operator $ A _ {0} $ |
| + | of a distribution semi-group is defined as follows. If there exists a delta-sequence $ \{ \rho _ {n} \} \subset D ^ + ( \mathbf R ) $ | ||
| + | such that $ T ( \rho _ {n} ) x \rightarrow x $ | ||
| + | and $ T ( - \rho _ {n} ^ \prime ) x \rightarrow y $ | ||
| + | as $ n \rightarrow \infty $, | ||
| + | then $ x \in D ( A _ {0} ) $ | ||
| + | and $ y = A _ {0} x $. | ||
| + | The infinitesimal operator has a closure $ A = \overline{ {A _ {0} }}\; $, | ||
| + | known as the infinitesimal generator of the distribution semi-group. The set $ \cap_{n=1}^ \infty D ( A _ {0} ^ {n} ) $ | ||
| + | is dense in $ X $ | ||
| + | and contains $ T ( \phi ) X $ | ||
| + | for any $ \phi \in D ^ + ( \mathbf R ) $. | ||
| − | A closed linear operator | + | A closed linear operator $ A $ |
| + | with a dense domain of definition in $ X $ | ||
| + | is the infinitesimal generator of a distribution semi-group if and only if there exist numbers $ a , b \geq 0 $, | ||
| + | $ c > 0 $ | ||
| + | and a natural number $ m $ | ||
| + | such that the resolvent $ R ( \lambda , A ) $ | ||
| + | exists for $ \mathop{\rm Re} \lambda \geq a \mathop{\rm ln} ( 1 + | \lambda | ) + b $ | ||
| + | and satisfies the inequality | ||
| − | + | $$ \tag{6 } | |
| + | \| R ( \lambda , A ) \| \leq c ( 1 + | \lambda | ) ^ {m} . | ||
| + | $$ | ||
| − | If | + | If $ A $ |
| + | is a closed linear operator on $ X $, | ||
| + | then the set $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ | ||
| + | can be made into a [[Fréchet space|Fréchet space]] $ X _ \infty $ | ||
| + | by introducing the system of norms | ||
| − | + | $$ | |
| + | \| x \| _ {n} = \sum_{k=0}^n \| A ^ {k} x \| . | ||
| + | $$ | ||
| − | The restriction | + | The restriction $ A _ \infty $ |
| + | of $ A $ | ||
| + | to $ X _ \infty $ | ||
| + | leaves $ X _ \infty $ | ||
| + | invariant. If $ A $ | ||
| + | is the infinitesimal generator of a semi-group, then $ A _ \infty $ | ||
| + | is the infinitesimal generator of a semi-group of class $ C _ {0} $( | ||
| + | continuous for $ t \geq 0 $, | ||
| + | $ T ( 0 ) = I $) | ||
| + | on $ X _ \infty $. | ||
| + | Conversely, if $ X _ \infty $ | ||
| + | is dense in $ X $, | ||
| + | the operator $ A $ | ||
| + | has a non-empty resolvent set and $ A $ | ||
| + | is the infinitesimal generator of a semi-group of class $ C _ {0} $ | ||
| + | on $ X _ \infty $, | ||
| + | then $ A $ | ||
| + | is the infinitesimal generator of a distribution semi-group on $ X $. | ||
| − | A distribution semi-group has exponential growth of order at most | + | A distribution semi-group has exponential growth of order at most $ q $, |
| + | $ 1 \leq q < \infty $, | ||
| + | if there exists an $ \omega > 0 $ | ||
| + | such that $ \mathop{\rm exp} ( - \omega t ^ {q} ) T ( \phi ) $ | ||
| + | is a continuous mapping in the topology induced on $ D ^ + $ | ||
| + | by the space $ S ( \mathbf R ) $ | ||
| + | of rapidly-decreasing functions. A closed linear operator is the infinitesimal generator of a distribution semi-group with the above property if and only if it has a resolvent $ R ( \lambda , A ) $ | ||
| + | which satisfies (6) in the domain | ||
| − | + | $$ | |
| + | \{ \lambda : { \mathop{\rm Re} \lambda \geq [ \alpha \mathop{\rm ln} ( 1 + | \mathop{\rm Im} \ | ||
| + | \lambda | + \beta ) ] ^ {1- 1/q } , \mathop{\rm Re} \lambda > \omega } \} | ||
| + | , | ||
| + | $$ | ||
| − | where | + | where $ \alpha , \beta > 0 $. |
| + | In particular, if $ q = 1 $ | ||
| + | the semi-group is said to be exponential and inequality (6) is valid in some half-plane. There exists a characterization of the semi-groups of the above types in terms of the operator $ A _ \infty $. | ||
| + | Questions of smoothness and analyticity have also been investigated for distribution semi-groups. | ||
| − | ==Semi-groups of operators in a (separable) locally convex space | + | ==Semi-groups of operators in a (separable) locally convex space $ X $.== |
| − | The definition of a strongly-continuous semi-group of operators | + | The definition of a strongly-continuous semi-group of operators $ T ( t ) $ |
| + | continuous on $ X $ | ||
| + | remains the same as for a Banach space. Similarly, the class $ C _ {0} $ | ||
| + | is defined by the property $ T ( t ) x \rightarrow x $ | ||
| + | as $ t \rightarrow 0 $ | ||
| + | for any $ x \in X $. | ||
| + | A semi-group is said to be locally equicontinuous (of class $ lC _ {0} $) | ||
| + | if the family of operators $ T ( t ) $ | ||
| + | is equicontinuous when $ t $ | ||
| + | ranges over any finite interval in $ ( 0 , \infty ) $. | ||
| + | In a [[Barrelled space|barrelled space]], a semi-group of class $ C _ {0} $ | ||
| + | is always equicontinuous (cf. [[Equicontinuity|Equicontinuity]]). | ||
| − | A semi-group is said to be equicontinuous (of class | + | A semi-group is said to be equicontinuous (of class $ uC _ {0} $) |
| + | if the family $ T ( t ) $, | ||
| + | $ 0 \leq t < \infty $, | ||
| + | is equicontinuous. | ||
Infinitesimal operators and infinitesimal generators are defined as in the Banach space case. | Infinitesimal operators and infinitesimal generators are defined as in the Banach space case. | ||
| − | Assume from now on that the space | + | Assume from now on that the space $ X $ |
| + | is sequentially complete. The infinitesimal generator $ A $ | ||
| + | of a semi-group of class $ l C _ {0} $ | ||
| + | is identical to the infinitesimal operator; its domain of definition, $ D ( A) $, | ||
| + | is dense in $ X $ | ||
| + | and, moreover, the set $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ | ||
| + | is dense in $ X $. | ||
| + | The semi-group $ T ( t ) $ | ||
| + | leaves $ D ( A) $ | ||
| + | invariant and | ||
| + | |||
| + | $$ | ||
| − | + | \frac{dT}{dt} | |
| + | ( t) x = \ | ||
| + | AT ( t ) x = T ( t ) Ax ,\ 0 \leq t < \infty ,\ x \in D ( A ) . | ||
| + | $$ | ||
| − | If | + | If $ A $ |
| + | is the infinitesimal generator of a semi-group of class $ u C _ {0} $, | ||
| + | the resolvent $ R ( \lambda , A ) $ | ||
| + | is defined for $ \mathop{\rm Re} \lambda > 0 $ | ||
| + | and is the Laplace transform of the semi-group. | ||
| − | A linear operator | + | A linear operator $ A $ |
| + | is the infinitesimal generator of a semi-group of class $ u C _ {0} $ | ||
| + | if and only if it is closed, has dense domain of definition in $ X $, | ||
| + | and if there exists a sequence of positive numbers $ \lambda _ {k} \rightarrow \infty $ | ||
| + | such that, for any $ \lambda _ {k} $, | ||
| + | the resolvent $ R ( \lambda _ {k} , A ) $ | ||
| + | is defined and the family of operators $ [ \lambda _ {k} R ( \lambda _ {k} , A ) ] ^ {n} $, | ||
| + | $ k , n = 1 , 2 \dots $ | ||
| + | is equicontinuous. In this situation the semi-group can be constructed by the formula | ||
| − | + | $$ | |
| + | ( t ) x = \lim\limits _ {k \rightarrow \infty } \ | ||
| + | \left ( \mathop{\rm exp} \left [ - \lambda _ {k} - \lambda _ {k} ^ {2} R ( \lambda _ {k} , A ) \right ] t \right ) x , | ||
| + | $$ | ||
| − | + | $$ | |
| + | t \geq 0 ,\ x \in X . | ||
| + | $$ | ||
| − | In a non-normed locally convex space, the infinitesimal generator of a semi-group of class | + | In a non-normed locally convex space, the infinitesimal generator of a semi-group of class $ lC _ {0} $ |
| + | may have no resolvent at any point. An example is: $ A = d / ds $ | ||
| + | in the space $ C ^ \infty $ | ||
| + | of infinitely-differentiable functions of $ s $ | ||
| + | on $ \mathbf R $. | ||
| + | As a substitute for the resolvent one can take a continuous operator whose product with $ A - \lambda I $, | ||
| + | from the right and the left, differs by a "small amount" from the identity operator. | ||
| − | A continuous operator | + | A continuous operator $ R ( \lambda ) $ |
| + | defined for $ \lambda $ | ||
| + | in a set $ \Lambda \subset \mathbf C $ | ||
| + | is called an asymptotic resolvent for a linear operator $ A $ | ||
| + | if $ AR ( \lambda ) $ | ||
| + | is continuous on $ X $, | ||
| + | the operator $ R ( \lambda ) A $ | ||
| + | can be extended from $ D ( A) $ | ||
| + | to a continuous operator $ B ( \lambda ) $ | ||
| + | on $ X $, | ||
| + | and if there exists a limit point $ \lambda _ {0} $ | ||
| + | of the set $ \Lambda $ | ||
| + | such that $ H ^ + ( \lambda ) x \rightarrow 0 $, | ||
| + | $ H ^ {-} ( \lambda ) x \rightarrow 0 $ | ||
| + | as $ \lambda \rightarrow \lambda _ {0} $ | ||
| + | for any $ x \in X $, | ||
| + | where | ||
| − | + | $$ | |
| + | H ^ + ( \lambda ) = ( A - \lambda I ) R ( \lambda ) - I ,\ \ | ||
| + | H ^ {-} ( \lambda ) = B ( \lambda ) - \lambda R ( \lambda ) - I . | ||
| + | $$ | ||
An asymptotic resolvent possesses various properties resembling those of the ordinary resolvent. | An asymptotic resolvent possesses various properties resembling those of the ordinary resolvent. | ||
| − | A closed linear operator | + | A closed linear operator $ A $ |
| + | with a dense domain of definition in $ X $ | ||
| + | is the infinitesimal generator of a semi-group of class $ l C _ {0} $ | ||
| + | if and only if there exist numbers $ \omega $ | ||
| + | and $ \alpha > 0 $ | ||
| + | such that, for $ \lambda > \omega $, | ||
| + | there exists an asymptotic resolvent $ R ( \lambda ) $ | ||
| + | of $ A $ | ||
| + | with the properties: the functions $ R ( \lambda ) $, | ||
| + | $ H ^ + ( \lambda ) $, | ||
| + | $ H ^ {-} ( \lambda ) $ | ||
| + | are strongly infinitely differentiable for $ \lambda > \omega $, | ||
| + | and the families of operators | ||
| − | + | $$ | |
| + | e ^ {\alpha \lambda } | ||
| + | \frac{d ^ {n} H ^ \pm ( \lambda ) }{d \lambda | ||
| + | ^ {n} } | ||
| + | ,\ | ||
| + | \frac{\lambda ^ {n+1} }{n ! } | ||
| + | |||
| + | \frac{d ^ {n} R ( \lambda ) }{d \lambda ^ {n} } | ||
| + | ,\ \lambda > \omega ,\ n = 0 , 1 \dots | ||
| + | $$ | ||
are equicontinuous. | are equicontinuous. | ||
| Line 223: | Line 680: | ||
==Adjoint semi-groups.== | ==Adjoint semi-groups.== | ||
| − | If | + | If $ T ( t ) $ |
| + | is a semi-group of class $ C _ {0} $ | ||
| + | on a Banach space $ X $, | ||
| + | then the adjoint operators form a semi-group of bounded operators on the adjoint space $ X ^ \prime $. | ||
| + | However, the assertion that $ T ^ \prime ( t ) f \rightarrow f $ | ||
| + | as $ t \rightarrow 0 $ | ||
| + | for any $ f \in X ^ \prime $ | ||
| + | is valid only in the sense of the weak- topology $ \sigma ( X ^ \prime , X ) $. | ||
| + | If $ A $ | ||
| + | is the generating operator, its adjoint $ A ^ \prime $ | ||
| + | is a weak infinitesimal generator for $ T ^ \prime ( t ) $, | ||
| + | in the sense that $ D ( A ^ \prime ) $ | ||
| + | is the set of all $ f $ | ||
| + | for which the limit of $ t ^ {-1} [ T ^ \prime ( t ) - I ] f $ | ||
| + | as $ t\rightarrow 0 $ | ||
| + | exists in the sense of weak- convergence and is equal to $ A ^ \prime f $. | ||
| + | The domain of definition $ D ( A ^ \prime ) $ | ||
| + | is dense in $ X ^ \prime $— | ||
| + | again in the sense of the weak- topology — and the operator $ A ^ \prime $ | ||
| + | is closed in the weak- topology. | ||
| − | Let | + | Let $ X ^ + $ |
| + | be the set of all elements in $ X ^ \prime $ | ||
| + | such that $ T ^ \prime ( f ) \rightarrow f $ | ||
| + | as $ t \rightarrow 0 $ | ||
| + | in the strong sense; then $ X ^ + $ | ||
| + | is a closed subspace of $ X ^ \prime $ | ||
| + | that is invariant under all $ T ^ \prime ( t ) $. | ||
| + | On $ X ^ + $ | ||
| + | the operators $ T ^ \prime ( t ) $ | ||
| + | form a semi-group of class $ C _ {0} $. | ||
| + | The space $ X ^ + $ | ||
| + | is also the strong closure of the set $ D ( A ^ \prime ) $ | ||
| + | in $ X ^ \prime $. | ||
| + | If the original space is reflexive, then $ X ^ + = X ^ \prime $. | ||
| + | Analogous propositions hold for semi-groups of class $ C _ {0} $ | ||
| + | in locally convex spaces. Semi-groups of classes $ l C _ {0} $ | ||
| + | and $ u C _ {0} $ | ||
| + | generate semi-groups of the same classes in $ X ^ + $. | ||
==Distribution semi-groups in a (separable) locally convex space.== | ==Distribution semi-groups in a (separable) locally convex space.== | ||
| − | A distribution semi-group | + | A distribution semi-group $ T $ |
| + | in a sequentially complete locally convex space is defined just as in a Banach space. A semi-group $ T $ | ||
| + | is said to be locally equicontinuous (of class $ lD ^ \prime $) | ||
| + | if, for any compact subset $ K \subset D ( \mathbf R ) $, | ||
| + | the family of operators $ \{ T ( \phi ) \} $, | ||
| + | $ \phi \in K $, | ||
| + | is equicontinuous. In a barrelled space $ X $, | ||
| + | any distribution semi-group is defined by analogy to the Banach case. For semi-groups of class $ l D ^ \prime $, | ||
| + | the infinitesimal operator is closed $ ( A _ {0} = A ) $, | ||
| + | $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ | ||
| + | is dense in $ X $, | ||
| + | and for any $ x \in X $ | ||
| + | and $ \phi \in D ( \mathbf R ) $, | ||
| − | + | $$ \tag{7 } | |
| + | \left . | ||
| + | \begin{array}{c} | ||
| − | A | + | {T ( \phi ) x \in D ( A ) ,\ |
| + | T ^ \prime ( \phi ) x = AT ( \phi ) x + \phi ( 0 ) x , } | ||
| + | \\ | ||
| − | + | {T ^ \prime ( \phi ) x = T ( \phi ) Ax + \phi ( 0 ) x ,\ x \in D ( A ). } | |
| + | \end{array} | ||
| − | + | \right \} | |
| + | $$ | ||
| − | + | A generalized function $ T $ | |
| + | with support in $ [ 0 , \infty ) $, | ||
| + | possessing the properties (7), is naturally called the fundamental function of the operator $ ( d / dt ) - A $. | ||
| + | Thus, if $ A $ | ||
| + | is the infinitesimal operator of a semi-group $ T $ | ||
| + | of class $ l D ^ \prime $, | ||
| + | then $ T $ | ||
| + | is the fundamental function of the operator $ ( d / dt ) - A $. | ||
| + | The converse statement is true under certain additional assumptions about the order of singularity of the fundamental function $ T $( | ||
| + | or, more precisely, of the function $ f ( T ( \phi ) x ) $, | ||
| + | where $ f \in X ^ \prime $). | ||
| − | + | A useful notion for the characterization of semi-groups in a locally convex space is that of the generalized resolvent. Let $ \widehat \phi $ | |
| + | denote the Laplace transform of a function $ \phi \in D ( \mathbf R ) $, | ||
| + | and let $ \widehat{D} ( \mathbf R ) $ | ||
| + | be the space of all such transforms. A topology is induced in this space, via the Laplace transform, from the topology of $ D ( \mathbf R ) $. | ||
| + | The Laplace transform of an $ X $- | ||
| + | valued generalized function $ F $ | ||
| + | is defined by $ \widehat{F} ( \widehat \phi ) = F ( \phi ) $. | ||
| + | Under these conditions, $ \widehat{F} $ | ||
| + | is a continuous mapping of $ \widehat{D} ( \mathbf R ) $ | ||
| + | into the space $ L ( X ) $ | ||
| + | of continuous linear operators on $ X $. | ||
| + | Let $ \widehat{D} {} _ + ^ \prime $ | ||
| + | be the space of all $ \widehat{F} $ | ||
| + | obtained from functions $ F $ | ||
| + | with support in $ ( 0 , \infty ) $, | ||
| + | with the natural topology. If $ A $ | ||
| + | is a linear operator on $ X $, | ||
| + | it can be "lifted" to an operator $ \widetilde{A} $ | ||
| + | on $ \widehat{D} {} _ + ^ \prime $ | ||
| + | via the equality | ||
| − | + | $$ | |
| + | ( \widetilde{A} \widehat{F} ) = A ( \widehat{F} ( \widehat \phi ) ) = A F ( \phi ) . | ||
| + | $$ | ||
| − | + | Thus, it is defined for all $ \widehat{F} \in \widehat{D} {} _ + ^ \prime $ | |
| + | such that the right-hand side of the equality is defined for any $ \phi \in D ( \mathbf R ) $ | ||
| + | and it extends to a generalized function in $ \widehat{D} {} _ + ^ \prime $. | ||
| + | The continuous operator $ \widetilde \lambda $ | ||
| + | on $ \widehat{D} {} _ + ^ \prime $ | ||
| + | is defined by | ||
| − | + | $$ | |
| + | ( \widetilde \lambda \widehat{F} ) ( \widehat \phi ) = \lambda \widehat{F} ( \widehat \phi ) = F ^ \prime | ||
| + | ( \phi ) = - F ( \phi ^ \prime ) . | ||
| + | $$ | ||
| − | + | If the operator $ \widetilde{A} - \widetilde \lambda $ | |
| + | has a continuous inverse $ \widetilde{R} $ | ||
| + | on $ \widehat{D} {} _ + ^ \prime $, | ||
| + | then $ \widetilde{R} $ | ||
| + | is called the generalized resolvent of $ A $. | ||
| − | + | An operator $ A $ | |
| + | has a generalized resolvent if and only if the operator $ ( d / dt ) - A $ | ||
| + | has a locally equicontinuous fundamental function $ T $, | ||
| + | constructed by the formula | ||
| − | + | $$ | |
| − | + | T ( \phi ) x = ( \widetilde{R} ( 1 \otimes x ) ) ( \widehat \phi ) ,\ \phi \in D ( \mathbf R ) ,\ \ | |
| − | + | x \in X , | |
| − | + | $$ | |
| − | |||
| − | |||
| + | where | ||
| + | $$ | ||
| + | ( 1 \otimes x ) ( \widehat \phi ) = ( \delta \otimes x ) \phi = \phi ( 0 ) x . | ||
| + | $$ | ||
| − | + | Subject to certain additional assumptions, $ T $ | |
| + | is a distribution semi-group. An extension theorem for semi-groups of class $ l C _ {0} $ | ||
| + | has also been proved in terms of generalized resolvents. | ||
| + | See also [[Semi-group of non-linear operators]]. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[ | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> E. Hille, R.S. Phillips, "Functional analysis and semi-groups" , Amer. Math. Soc. (1957) {{MR|0089373}} {{ZBL|0392.46001}} {{ZBL|0033.06501}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> Yu.M. Vuvunikyan, "Evolutionary representations of algebras of generalized functions" , ''Theory of operators in function spaces'' , Novosibirsk (1977) pp. 99–120 (In Russian)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> P.P. Zabreiko, A.V. Zafievskii, "On a certain class of semigroups" ''Soviet Math. Dokl.'' , '''10''' : 6 (1969) pp. 1523–1526 ''Dokl. Akad. Nauk SSSR'' , '''189''' : 5 (1969) pp. 934–937 {{MR|264459}} {{ZBL|}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> A.V. Zafievskii, ''Trudy Mat. Inst. Voronezh. Univ.'' , '''1''' (1970) pp. 206–210</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, §1 {{MR|0617913}} {{ZBL|0435.46002}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> S.G. Krein, "Linear differential equations in Banach space" , ''Transl. Math. Monogr.'' , '''29''' , Amer. Math. Soc. (1971) (Translated from Russian) {{MR|0342804}} {{ZBL|0179.20701}} </TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> Yu.T. Sil'chenko, "An evolutionary equation with an operator generating a nonlinear semigroup" ''Differential Equations'' , '''15''' : 2 (1979) pp. 255–258 ''Differentsial'nye Uravneniya'' , '''15''' : 2 (1979) pp. 363–366 {{MR|}} {{ZBL|0505.34046}} </TD></TR> |
| + | <TR><TD valign="top">[8]</TD> <TD valign="top"> J. Chazarain, "Problèmes de Cauchy abstraits et applications à quelques problèmes mixtes" ''J. Funct. Anal.'' , '''7''' : 3 (1971) pp. 386–446 {{ZBL|0211.12902}} </TD></TR> | ||
| + | <TR><TD valign="top">[9]</TD> <TD valign="top"> I. Ciorânescu, "La caracterisation spectrale d'opérateur, générateurs des semi-groupes distributions d'ordre fini de croissance" ''J. Math. Anal. Appl.'' , '''34''' (1971) pp. 34–41</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> I. Ciorânescu, "A characterization of distribution semigroups of finite growth order" ''Rev. Roum. Math. Pures Appl.'' , '''22''' : 8 (1977) pp. 1053–1068 {{MR|500280}} {{ZBL|0374.46032}} </TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> T. Kato, "A characterization of holomorphic semigroups" ''Proc. Amer. Math. Soc.'' , '''25''' : 3 (1970) pp. 495–498 {{MR|0264456}} {{ZBL|0199.45604}} </TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> J. Lions, "Les semigroupes distributions" ''Portugal. Math.'' , '''19''' (1960) pp. 141–164</TD></TR><TR><TD valign="top">[13]</TD> <TD valign="top"> A. Pazy, "On the differentiability and compactness of semi-groups of linear operators" ''J. Math. Mech.'' , '''17''' : 12 (1968) pp. 1131–1141 {{MR|231242}} {{ZBL|0162.45903}} </TD></TR><TR><TD valign="top">[14]</TD> <TD valign="top"> A. Pazy, "Approximations of the identity operator by semigroups of linear operators" ''Proc. Amer. Math. Soc.'' , '''30''' : 1 (1971) pp. 147–150 {{MR|0287362}} {{ZBL|}} </TD></TR><TR><TD valign="top">[15]</TD> <TD valign="top"> T. Ushijima, "On the abstract Cauchy problems and semi-groups of linear operators in locally convex spaces" ''Sci. Papers College Gen. Educ. Univ. Tokyo'' , '''21''' (1971) pp. 93–122 {{MR|0312324}} {{ZBL|0239.47031}} </TD></TR><TR><TD valign="top">[16]</TD> <TD valign="top"> T. Ushijima, "On the generation and smoothness of semi-groups of linear operators" ''J. Fac. Sci. Univ. Tokyo, Sec. 1A'' , '''19''' : 1 (1972) pp. 65–127 {{MR|0308854}} {{ZBL|0239.47032}} </TD></TR><TR><TD valign="top">[17]</TD> <TD valign="top"> C. Wild, "Semi-groupes de croissance <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s084/s084140/s084140481.png" /> holomorphes" ''C.R. Acad. Sci. Paris Sér. A'' , '''285''' (1977) pp. 437–440 (English abstract) {{MR|448159}} {{ZBL|0359.47024}} </TD></TR><TR><TD valign="top">[18]</TD> <TD valign="top"> J.A. Gol'dstein, "Semigroups of linear operators and application" , Oxford Univ. Press (1985) (Translated from Russian)</TD></TR><TR><TD valign="top">[19]</TD> <TD valign="top"> A. Pazy, "Semigroups of linear operators and application to partial differential equations" , Springer (1983) {{MR|0710486}} {{ZBL|}} </TD></TR><TR><TD valign="top">[20]</TD> <TD valign="top"> Ph. Clément, H.J.A.M. Heijmans, S. Angenent, C.J. van Duijn, B. de Pagter, "One-parameter semigroups" , ''CWI Monographs'' , '''5''' , North-Holland (1987) {{MR|0915552}} {{ZBL|0636.47051}} </TD></TR> | ||
| + | <TR><TD valign="top">[a1]</TD> <TD valign="top"> P. Butzer, H. Berens, "Semigroups of operators and approximation" , Springer (1967) {{MR|230022}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> H. Kellermann, M. Hieber, "Integrated semigroups" ''J. Funct. Anal.'' , '''84''' (1989) pp. 160–180 {{MR|0999494}} {{ZBL|0604.47025}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> I. Miyadera, N. Tanaka, "Exponentially bounded $c$-semigroups and integrated semigroups" ''Tokyo J. Math.'' , '''12''' (1989) pp. 99–115 {{MR|1001735}} {{ZBL|}} </TD></TR></table> | ||
Latest revision as of 09:12, 21 January 2024
A family $ \{ T \} $
of operators on a Banach space or topological vector space with the property that the composite of any two operators in the family is again a member of the family. If the operators $ T $
are "indexed" by elements of some abstract semi-group $ \mathfrak A $
and the binary operation of the latter is compatible with the composition of operators, $ \{ T \} $
is known as a representation of the semi-group $ \mathfrak A $.
The most detailed attention has been given to one-parameter semi-groups (cf. One-parameter semi-group) of bounded linear operators on a Banach space $ X $,
which yield a representation of the additive semi-group of all positive real numbers, i.e. families $ T ( t) $
with the property
$$ T ( t + \tau ) x = T ( t) T ( \tau ) x ,\ t , \tau > 0 ,\ x \in X . $$
If $ T ( t) $ is strongly measurable, $ t > 0 $, then $ T ( t) $ is a strongly-continuous semi-group; this will be assumed in the sequel.
The limit
$$ \omega = \lim\limits _ {t \rightarrow \infty } \ t ^ {-1} \mathop{\rm ln} \| T ( t) \| $$
exists; it is known as the type of the semi-group. The functions $ T ( t) x $ increase at most exponentially.
An important characteristic is the infinitesimal operator (infinitesimal generator) of the semi-group:
$$ A _ {0} x = \lim\limits _ {t \rightarrow 0 } t ^ {-1} [ T ( t) x - x ] , $$
defined on the linear set $ D ( A _ {0} ) $ of all elements $ x $ for which the limit exists; the closure, $ A $, of this operator (if it exists) is known as the generating operator, or generator, of the semi-group. Let $ X _ {0} $ be the subspace defined as the closure of the union of all values $ T ( t) x $; then $ D ( A _ {0} ) $ is dense in $ X _ {0} $. If there are no non-zero elements in $ X _ {0} $ such that $ T ( t) x \equiv 0 $, then the generating operator $ A $ exists. In the sequel it will be assumed that $ X _ {0} = X $ and that $ T ( t) x \equiv 0 $ implies $ x = 0 $.
The simplest class of semi-groups, denoted by $ C _ {0} $, is defined by the condition: $ T ( t) x \rightarrow x $ as $ t \rightarrow 0 $ for any $ x \in X $. This is equivalent to the condition: The function $ \| T ( t) \| $ is bounded on any interval $ ( 0 , a ] $. In that case $ T ( t) $ has a generating operator $ A = A _ {0} $ whose resolvent $ R ( \lambda , A ) = ( A - \lambda I ) ^ {-1} $ satisfies the inequalities
$$ \tag{1 } \| R ^ {n} ( \lambda , A ) \| \leq M ( \lambda - \omega ) ^ {-} n ,\ \ n = 1 , 2 , . . . ; \ \lambda > \omega , $$
where $ \omega $ is the type of the semi-group. Conversely, if $ A $ is a closed operator with domain of definition dense in $ X $ and with a resolvent satisfying (1), then it is the generating operator of some semi-group $ T ( t) $ of class $ C _ {0} $ such that $ \| T ( t) \| \leq M e ^ {\omega t } $. Condition (1) is satisfied if
$$ \| R ( \lambda , A ) \| \leq ( \lambda - \omega ) ^ {-1} $$
(the Hill–Yosida condition). If, moreover, $ \omega = 0 $, then $ T ( t) $ is a contraction semi-group: $ \| T ( t) \| \leq 1 $.
A summable semi-group is a semi-group for which the functions $ \| T ( t) x \| $ are summable on any finite interval for all $ x \in X $. A summable semi-group has a generating operator $ A = \overline{ {A _ {0} }}\; $. The operator $ A _ {0} $ is closed if and only if, for every $ x \in X $,
$$ \lim\limits _ {t \rightarrow 0 } \frac{1}{t} \int\limits _ { 0 } ^ { t } T ( s) x d s = x . $$
For $ \mathop{\rm Re} \lambda > \omega $ one can define the Laplace transform of a summable semi-group,
$$ \tag{2 } \int\limits _ { 0 } ^ \infty e ^ {- \lambda t } T ( t) x d t = - R ( \lambda ) x , $$
giving a bounded linear operator $ R ( \lambda ) $ which has many properties of a resolvent operator.
A closed operator $ A $ with domain of definition dense in $ X $ is the generating operator of a summable semi-group $ T ( t) $ if and only if, for some $ \omega $, the resolvent $ R ( \lambda , A ) $ exists for $ \mathop{\rm Re} \lambda > \omega $ and the following conditions hold: a) $ \| R ( \lambda , A ) \| \leq M $, $ \mathop{\rm Re} \lambda > \omega $; b) there exist a non-negative function $ \phi ( t , x ) $, $ t > 0 $, $ x \in X $, jointly continuous in all its variables, and a non-negative function $ \phi ( t) $, bounded on any interval $ [ a , b ] \subset ( 0 , \infty ) $, such that, for $ \omega _ {1} > \omega $,
$$ \int\limits _ { 0 } ^ \infty e ^ {- \omega _ {1} t } \phi ( t , x ) dt < \infty , $$
$$ \overline{\lim\limits}\; _ {t \rightarrow \infty } t ^ {-1} \mathop{\rm ln} \phi ( t) < \infty ,\ \phi ( t , x ) \leq \phi ( t) \| x \| , $$
$$ \| R ^ {n} ( \lambda , A ) x \| \leq \frac{1}{ ( n - 1 ) ! } \int\limits _ { 0 } ^ \infty t ^ {n-1} e ^ {- \lambda t } \phi ( t , x ) dt . $$
Under these conditions
$$ \| T ( t) x \| \leq \phi ( t , x ) ,\ \ \| T ( t) \| \leq \phi ( t) . $$
If one requires in addition that the function $ \| T ( t) \| $ be summable on finite intervals, a necessary and sufficient condition is the existence of a continuous function $ \phi ( t) $ such that, for $ \omega _ {1} > \omega $,
$$ \tag{3 } \int\limits _ { 0 } ^ \infty \phi ( t) e ^ {- \omega _ {1} t } dt < \infty , $$
$$ \tag{4 } \| R ^ {n} ( \lambda , A ) \| \leq \frac{1}{( n - 1 ) ! } \int\limits _ { 0 } ^ \infty t ^ {n-1} e ^ {- \lambda t } \phi ( t) dt , $$
$$ \lambda > \omega ,\ n= 1 , 2 , . . . . $$
Under these conditions, $ \| T ( t) \| \leq \phi ( t) $. By choosing different functions satisfying (3), one can define different subclasses of summable semi-groups. If $ \phi ( t) = Me ^ {\omega t } $, the result is the class $ C _ {0} $ and (1) follows from (4). If $ \phi ( t) = Mt ^ {- \alpha } e ^ {\omega t } $, $ 0 \leq \alpha < 1 $, condition (4) implies the condition
$$ \| R ^ {n} ( \lambda , A ) \| \leq \frac{M \Gamma ( n - \alpha ) }{( n - 1 ) ! ( \lambda - \omega ) ^ {n - \alpha } } ,\ \ \lambda > \omega ,\ n = 1 , 2 ,\dots. $$
Semi-groups with power singularities.
If in the previous example $ \alpha \geq 1 $, then the integrals in (4) are divergent for $ n \leq \alpha - 1 $. Hence the generating operator for the corresponding semi-group may not have a resolvent for any $ \lambda $, i.e. it may have a spectrum equal to the entire complex plane. However, for $ n $ large enough one can define for such operators functions $ S _ {n} ( \lambda , A ) $ which coincide with the functions $ R ^ {n+1} ( \lambda , A ) $ in the previous cases. The operator function $ S _ {n} ( \lambda , A ) $ is called a resolvent of order $ n $ if it is analytic in some domain $ G \subset \mathbf C $ and if for $ \lambda \in G $,
$$ S _ {n} ( \lambda , A ) Ax = A S _ {n} ( \lambda , A ) x ,\ \ x \in D ( A) , $$
$$ S _ {n} ( \lambda , A ) ( A - \lambda ) ^ {n+1} x = x ,\ x \in D ( A ^ {n+1} ) , $$
and if $ S _ {n} ( \lambda , A ) x = 0 $ for all $ \lambda \in G $ implies $ x = 0 $. If $ \overline{D}\; ( A ^ {n+1} ) = X $, the operator may have a unique resolvent of order $ n $, for which there is a maximal domain of analyticity, known as the resolvent set of order $ n $.
Let $ T ( t) $ be a strongly-continuous semi-group such that the inequality
$$ \| T ( t) \| \leq M t ^ {- \alpha } e ^ {\omega t } $$
holds for $ \alpha \geq 1 $. Then its generating operator $ B $ has a resolvent of order $ n $ for $ n > \alpha - 1 $, and, moreover,
$$ S _ {n} ( \lambda , B ) x = \ \frac{1}{n!} \int\limits _ { 0 } ^ \infty t ^ {n} e ^ {- \lambda t } T ( t) x dt ,\ \mathop{\rm Re} \lambda > \omega , $$
$$ \tag{5 } \left \| \frac{d ^ {k} S _ {n} ( \lambda , B ) }{d \lambda ^ {k} } x \right \| \leq \frac{M \Gamma ( k + n + 1 - \alpha ) }{n ! ( \mathop{\rm Re} \lambda - \omega ) ^ {k + n + 1 - \alpha } } , $$
$$ \mathop{\rm Re} \lambda > \omega ,\ k = 0 , 1 ,\dots . $$
Conversely, suppose that for $ \mathop{\rm Re} \lambda > 0 $ the operator $ B $ has a resolvent $ S _ {n} ( \lambda , B ) $ of order $ n $ satisfying (5) with $ n > \alpha - 1 $. Then there exists a unique semi-group $ T ( t) $ such that
$$ \| T ( t) \| \leq M t ^ {- \alpha } e ^ {\omega t } , $$
and the generating operator $ A $ of this semi-group is such that $ S _ {n} ( \lambda , A ) = S _ {n} ( \lambda , B ) $.
Smooth semi-groups.
If $ x \in D ( A _ {0} ) $, the function $ T ( t) x $ is continuously differentiable and
$$ \frac{d T ( t) }{dt} x = A _ {0} T ( t) x = T ( t) A _ {0} x . $$
There exist semi-groups of class $ C _ {0} $ such that, if $ x \notin D ( A _ {0} ) = D ( A) $, the functions $ T ( t) x $ are non-differentiable for all $ t $. However, there are important classes of semi-groups for which the degree of smoothness increases with increasing $ t $. If the functions $ T ( t) x $, $ t > t _ {0} $, are differentiable for any $ x \in X $, then it follows from the semi-group property that the $ T ( t) x $ are twice differentiable if $ t > 2 t _ {0} $, three times differentiable if $ t > 3 t _ {0} $, etc. Therefore, if these functions are differentiable at any $ t > 0 $ for $ x \in X $, then $ T ( t) x $ is infinitely differentiable.
Given a semi-group of class $ C _ {0} $, a necessary and sufficient condition for the functions $ T ( t) x $ to be differentiable for all $ x \in X $ and $ t > t _ {0} $, where $ t _ {0} \geq 0 $, is that there exist numbers $ a , b , c > 0 $ such that the resolvent $ R ( \lambda , A ) $ is defined in the domain
$$ \mathop{\rm Re} \lambda > a - b \mathop{\rm ln} | \mathop{\rm Im} \lambda | , $$
while in this domain
$$ \| R( \lambda , A ) \| \leq c | \mathop{\rm Im} \lambda | . $$
A necessary and sufficient condition for $ T ( t) x $ to be infinitely differentiable for all $ x \in X $ and $ t > 0 $ is that, for every $ b > 0 $, there exist $ a _ {b} , c _ {b} $ such that the resolvent $ R ( \lambda , A ) $ is defined in the domain
$$ \mathop{\rm Re} \lambda > a _ {b} - b \mathop{\rm ln} | \mathop{\rm Im} \lambda | , $$
and such that
$$ \| R ( \lambda , A ) \| \leq c _ {b} | \mathop{\rm Im} \lambda | . $$
Sufficient conditions are: If there exists a $ \mu > \omega $ for which
$$ \overline{\lim\limits}\; _ {\tau \rightarrow \infty } \mathop{\rm ln} | \tau | \| R ( \mu + i \tau , A ) \| = t _ {0} < \infty , $$
then the $ T ( t) x $ are differentiable for $ t > t _ {0} $ and $ x \in X $; if $ t _ {0} = 0 $, then the $ T ( t) x $ are infinitely differentiable for all $ t > 0 $ and $ x \in X $.
The degree of smoothness of a semi-group may sometimes be inferred from its behaviour at zero; for example, suppose that for every $ c > 0 $ there exists a $ \delta _ {c} $ such that, for $ 0 < t < \delta _ {c} $,
$$ \| I - T ( t) \| \leq 2 - ct \mathop{\rm ln} t ^ {-1} , $$
then the $ T ( t) x $ are infinitely differentiable for all $ t > 0 $, $ x \in X $.
There are smoothness conditions for summable semi-groups and semi-groups of polynomial growth. If a semi-group has polynomial growth of degree $ \alpha $ and is infinitely differentiable for $ t > 0 $, then the function
$$ \frac{d T ( t) }{dt} x = A T ( t) x $$
also has polynomial growth:
$$ \| A T ( t) \| \leq M _ {1} t ^ {- \beta } e ^ {\omega t } . $$
In the general case there is no rigorous relationship between the numbers $ \alpha $ and $ \beta $, and $ \beta $ can be utilized for a more detailed classification of infinitely-differentiable semi-groups of polynomial growth.
Analytic semi-groups.
An important class of semi-groups, related to partial differential equations of parabolic type, comprises those semi-groups $ T ( t) $ which admit an analytic continuation to some sector of the complex plane containing the positive real axis. A semi-group of class $ C _ {0} $ has this property if and only if its resolvent satisfies the following inequality in some right half-plane $ \mathop{\rm Re} \lambda > \omega $:
$$ \| R ( \lambda , A ) \| \leq M | \lambda - \omega | ^ {-1} . $$
Another necessary and sufficient conditions is: The semi-group is strongly differentiable and its derivative satisfies the estimate
$$ \left \| \frac{d T }{dt} ( t) \right \| \leq M t ^ {-1} e ^ {\omega t } . $$
Finally, the inequality
$$ \overline{\lim\limits}\; _ {t \rightarrow 0 } \| I - T ( t) \| < 2 $$
is also a sufficient condition for $ T ( t) $ to be analytic.
If a semi-group $ T ( t) $ has an analytic continuation $ T ( z) $ to a sector $ | \mathop{\rm arg} z | < \phi \leq \pi / 2 $ and has polynomial growth at zero, $ \| T ( z) \| \leq c | z | ^ \alpha $, $ \alpha > 0 $, then the resolvent $ S _ {n} ( \lambda , A ) $ of order $ n > \alpha - 1 $ of its generating operator $ A $ has an analytic continuation to the sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \phi $, and satisfies the following estimate in any sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \psi $, $ \psi < \phi $:
$$ \| S _ {n} ( \lambda , A ) \| \leq | \lambda | ^ {\alpha - n - 1 } M ( \psi ) . $$
Conversely, suppose that the resolvent $ S _ {n} ( \lambda , B ) $ of an operator $ B $ is defined in a sector $ | \mathop{\rm arg} \lambda | \leq \pi / 2 + \psi $ and that
$$ \| S _ {n} ( \lambda , B ) \| \leq \lambda ^ {\alpha - n - 1 } M . $$
Then there exists a semi-group $ T ( z) $ of growth $ \alpha $, analytic in the sector $ | \mathop{\rm arg} z | < \psi $, whose generating operator $ A $ is such that $ S _ {n} ( \lambda , A ) = S _ {n} ( \lambda , B ) $.
Distribution semi-groups.
In accordance with the general concept of the theory of distributions (cf. Generalized function), one can drop the requirement that the operator-valued function $ T ( t) $ be defined for every $ t > 0 $, demanding only that it be possible to evaluate the integrals $ \int _ {- \infty } ^ {+ \infty } T ( t) \phi ( t) dt $ for all $ \phi $ in the space $ D ( \mathbf R ) $ of infinitely-differentiable functions with compact support. Hence the following definition: A distribution semi-group on a Banach space $ X $ is a continuous linear mapping $ T ( \phi ) $ of $ D ( \mathbf R ) $ into the space $ L ( X) $ of all bounded linear operators on $ X $, with the following properties: a) $ T ( \phi ) = 0 $ if $ \supp \phi \subset ( - \infty , 0 ) $; b) if $ \phi , \psi $ are functions in the subspace $ D ^ + ( \mathbf R ) $ of all functions in $ D ( \mathbf R ) $ with support in $ ( 0 , \infty ) $, then $ T ( \phi * \psi ) = T ( \phi ) T ( \psi ) $, where the star denotes convolution:
$$ \phi * \psi = \int\limits _ {- \infty } ^ \infty \phi ( t - s ) \psi ( s) d s $$
(the semi-group property); c) if $ T ( \phi ) x = 0 $ for all $ \phi \in D ^ + ( \mathbf R ) $, then $ x = 0 $; d) the linear hull of the set of all values of $ T ( \phi ) x $, $ \phi \in D ^ + ( \mathbf R ) $, $ x \in X $, is dense in $ X $; e) for any $ y = T ( \psi ) x $, $ \psi \in D ^ + ( \mathbf R ) $, there exists a continuous $ u ( t) $ on $ ( 0 , \infty ) $ with values in $ X $, so that $ u( 0) = y $ and
$$ T ( \phi ) y = \int\limits _ { 0 } ^ \infty \phi ( t) u ( t) dt $$
for all $ \phi \in D ( \mathbf R ) $.
The infinitesimal operator $ A _ {0} $ of a distribution semi-group is defined as follows. If there exists a delta-sequence $ \{ \rho _ {n} \} \subset D ^ + ( \mathbf R ) $ such that $ T ( \rho _ {n} ) x \rightarrow x $ and $ T ( - \rho _ {n} ^ \prime ) x \rightarrow y $ as $ n \rightarrow \infty $, then $ x \in D ( A _ {0} ) $ and $ y = A _ {0} x $. The infinitesimal operator has a closure $ A = \overline{ {A _ {0} }}\; $, known as the infinitesimal generator of the distribution semi-group. The set $ \cap_{n=1}^ \infty D ( A _ {0} ^ {n} ) $ is dense in $ X $ and contains $ T ( \phi ) X $ for any $ \phi \in D ^ + ( \mathbf R ) $.
A closed linear operator $ A $ with a dense domain of definition in $ X $ is the infinitesimal generator of a distribution semi-group if and only if there exist numbers $ a , b \geq 0 $, $ c > 0 $ and a natural number $ m $ such that the resolvent $ R ( \lambda , A ) $ exists for $ \mathop{\rm Re} \lambda \geq a \mathop{\rm ln} ( 1 + | \lambda | ) + b $ and satisfies the inequality
$$ \tag{6 } \| R ( \lambda , A ) \| \leq c ( 1 + | \lambda | ) ^ {m} . $$
If $ A $ is a closed linear operator on $ X $, then the set $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ can be made into a Fréchet space $ X _ \infty $ by introducing the system of norms
$$ \| x \| _ {n} = \sum_{k=0}^n \| A ^ {k} x \| . $$
The restriction $ A _ \infty $ of $ A $ to $ X _ \infty $ leaves $ X _ \infty $ invariant. If $ A $ is the infinitesimal generator of a semi-group, then $ A _ \infty $ is the infinitesimal generator of a semi-group of class $ C _ {0} $( continuous for $ t \geq 0 $, $ T ( 0 ) = I $) on $ X _ \infty $. Conversely, if $ X _ \infty $ is dense in $ X $, the operator $ A $ has a non-empty resolvent set and $ A $ is the infinitesimal generator of a semi-group of class $ C _ {0} $ on $ X _ \infty $, then $ A $ is the infinitesimal generator of a distribution semi-group on $ X $.
A distribution semi-group has exponential growth of order at most $ q $, $ 1 \leq q < \infty $, if there exists an $ \omega > 0 $ such that $ \mathop{\rm exp} ( - \omega t ^ {q} ) T ( \phi ) $ is a continuous mapping in the topology induced on $ D ^ + $ by the space $ S ( \mathbf R ) $ of rapidly-decreasing functions. A closed linear operator is the infinitesimal generator of a distribution semi-group with the above property if and only if it has a resolvent $ R ( \lambda , A ) $ which satisfies (6) in the domain
$$ \{ \lambda : { \mathop{\rm Re} \lambda \geq [ \alpha \mathop{\rm ln} ( 1 + | \mathop{\rm Im} \ \lambda | + \beta ) ] ^ {1- 1/q } , \mathop{\rm Re} \lambda > \omega } \} , $$
where $ \alpha , \beta > 0 $. In particular, if $ q = 1 $ the semi-group is said to be exponential and inequality (6) is valid in some half-plane. There exists a characterization of the semi-groups of the above types in terms of the operator $ A _ \infty $. Questions of smoothness and analyticity have also been investigated for distribution semi-groups.
Semi-groups of operators in a (separable) locally convex space $ X $.
The definition of a strongly-continuous semi-group of operators $ T ( t ) $ continuous on $ X $ remains the same as for a Banach space. Similarly, the class $ C _ {0} $ is defined by the property $ T ( t ) x \rightarrow x $ as $ t \rightarrow 0 $ for any $ x \in X $. A semi-group is said to be locally equicontinuous (of class $ lC _ {0} $) if the family of operators $ T ( t ) $ is equicontinuous when $ t $ ranges over any finite interval in $ ( 0 , \infty ) $. In a barrelled space, a semi-group of class $ C _ {0} $ is always equicontinuous (cf. Equicontinuity).
A semi-group is said to be equicontinuous (of class $ uC _ {0} $) if the family $ T ( t ) $, $ 0 \leq t < \infty $, is equicontinuous.
Infinitesimal operators and infinitesimal generators are defined as in the Banach space case.
Assume from now on that the space $ X $ is sequentially complete. The infinitesimal generator $ A $ of a semi-group of class $ l C _ {0} $ is identical to the infinitesimal operator; its domain of definition, $ D ( A) $, is dense in $ X $ and, moreover, the set $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ is dense in $ X $. The semi-group $ T ( t ) $ leaves $ D ( A) $ invariant and
$$ \frac{dT}{dt} ( t) x = \ AT ( t ) x = T ( t ) Ax ,\ 0 \leq t < \infty ,\ x \in D ( A ) . $$
If $ A $ is the infinitesimal generator of a semi-group of class $ u C _ {0} $, the resolvent $ R ( \lambda , A ) $ is defined for $ \mathop{\rm Re} \lambda > 0 $ and is the Laplace transform of the semi-group.
A linear operator $ A $ is the infinitesimal generator of a semi-group of class $ u C _ {0} $ if and only if it is closed, has dense domain of definition in $ X $, and if there exists a sequence of positive numbers $ \lambda _ {k} \rightarrow \infty $ such that, for any $ \lambda _ {k} $, the resolvent $ R ( \lambda _ {k} , A ) $ is defined and the family of operators $ [ \lambda _ {k} R ( \lambda _ {k} , A ) ] ^ {n} $, $ k , n = 1 , 2 \dots $ is equicontinuous. In this situation the semi-group can be constructed by the formula
$$ ( t ) x = \lim\limits _ {k \rightarrow \infty } \ \left ( \mathop{\rm exp} \left [ - \lambda _ {k} - \lambda _ {k} ^ {2} R ( \lambda _ {k} , A ) \right ] t \right ) x , $$
$$ t \geq 0 ,\ x \in X . $$
In a non-normed locally convex space, the infinitesimal generator of a semi-group of class $ lC _ {0} $ may have no resolvent at any point. An example is: $ A = d / ds $ in the space $ C ^ \infty $ of infinitely-differentiable functions of $ s $ on $ \mathbf R $. As a substitute for the resolvent one can take a continuous operator whose product with $ A - \lambda I $, from the right and the left, differs by a "small amount" from the identity operator.
A continuous operator $ R ( \lambda ) $ defined for $ \lambda $ in a set $ \Lambda \subset \mathbf C $ is called an asymptotic resolvent for a linear operator $ A $ if $ AR ( \lambda ) $ is continuous on $ X $, the operator $ R ( \lambda ) A $ can be extended from $ D ( A) $ to a continuous operator $ B ( \lambda ) $ on $ X $, and if there exists a limit point $ \lambda _ {0} $ of the set $ \Lambda $ such that $ H ^ + ( \lambda ) x \rightarrow 0 $, $ H ^ {-} ( \lambda ) x \rightarrow 0 $ as $ \lambda \rightarrow \lambda _ {0} $ for any $ x \in X $, where
$$ H ^ + ( \lambda ) = ( A - \lambda I ) R ( \lambda ) - I ,\ \ H ^ {-} ( \lambda ) = B ( \lambda ) - \lambda R ( \lambda ) - I . $$
An asymptotic resolvent possesses various properties resembling those of the ordinary resolvent.
A closed linear operator $ A $ with a dense domain of definition in $ X $ is the infinitesimal generator of a semi-group of class $ l C _ {0} $ if and only if there exist numbers $ \omega $ and $ \alpha > 0 $ such that, for $ \lambda > \omega $, there exists an asymptotic resolvent $ R ( \lambda ) $ of $ A $ with the properties: the functions $ R ( \lambda ) $, $ H ^ + ( \lambda ) $, $ H ^ {-} ( \lambda ) $ are strongly infinitely differentiable for $ \lambda > \omega $, and the families of operators
$$ e ^ {\alpha \lambda } \frac{d ^ {n} H ^ \pm ( \lambda ) }{d \lambda ^ {n} } ,\ \frac{\lambda ^ {n+1} }{n ! } \frac{d ^ {n} R ( \lambda ) }{d \lambda ^ {n} } ,\ \lambda > \omega ,\ n = 0 , 1 \dots $$
are equicontinuous.
Generation theorems have also been proved for other classes of semi-groups of operators on a locally convex space.
Adjoint semi-groups.
If $ T ( t ) $ is a semi-group of class $ C _ {0} $ on a Banach space $ X $, then the adjoint operators form a semi-group of bounded operators on the adjoint space $ X ^ \prime $. However, the assertion that $ T ^ \prime ( t ) f \rightarrow f $ as $ t \rightarrow 0 $ for any $ f \in X ^ \prime $ is valid only in the sense of the weak- topology $ \sigma ( X ^ \prime , X ) $. If $ A $ is the generating operator, its adjoint $ A ^ \prime $ is a weak infinitesimal generator for $ T ^ \prime ( t ) $, in the sense that $ D ( A ^ \prime ) $ is the set of all $ f $ for which the limit of $ t ^ {-1} [ T ^ \prime ( t ) - I ] f $ as $ t\rightarrow 0 $ exists in the sense of weak- convergence and is equal to $ A ^ \prime f $. The domain of definition $ D ( A ^ \prime ) $ is dense in $ X ^ \prime $— again in the sense of the weak- topology — and the operator $ A ^ \prime $ is closed in the weak- topology.
Let $ X ^ + $ be the set of all elements in $ X ^ \prime $ such that $ T ^ \prime ( f ) \rightarrow f $ as $ t \rightarrow 0 $ in the strong sense; then $ X ^ + $ is a closed subspace of $ X ^ \prime $ that is invariant under all $ T ^ \prime ( t ) $. On $ X ^ + $ the operators $ T ^ \prime ( t ) $ form a semi-group of class $ C _ {0} $. The space $ X ^ + $ is also the strong closure of the set $ D ( A ^ \prime ) $ in $ X ^ \prime $. If the original space is reflexive, then $ X ^ + = X ^ \prime $. Analogous propositions hold for semi-groups of class $ C _ {0} $ in locally convex spaces. Semi-groups of classes $ l C _ {0} $ and $ u C _ {0} $ generate semi-groups of the same classes in $ X ^ + $.
Distribution semi-groups in a (separable) locally convex space.
A distribution semi-group $ T $ in a sequentially complete locally convex space is defined just as in a Banach space. A semi-group $ T $ is said to be locally equicontinuous (of class $ lD ^ \prime $) if, for any compact subset $ K \subset D ( \mathbf R ) $, the family of operators $ \{ T ( \phi ) \} $, $ \phi \in K $, is equicontinuous. In a barrelled space $ X $, any distribution semi-group is defined by analogy to the Banach case. For semi-groups of class $ l D ^ \prime $, the infinitesimal operator is closed $ ( A _ {0} = A ) $, $ \cap_{n=1}^ \infty D ( A ^ {n} ) $ is dense in $ X $, and for any $ x \in X $ and $ \phi \in D ( \mathbf R ) $,
$$ \tag{7 } \left . \begin{array}{c} {T ( \phi ) x \in D ( A ) ,\ T ^ \prime ( \phi ) x = AT ( \phi ) x + \phi ( 0 ) x , } \\ {T ^ \prime ( \phi ) x = T ( \phi ) Ax + \phi ( 0 ) x ,\ x \in D ( A ). } \end{array} \right \} $$
A generalized function $ T $ with support in $ [ 0 , \infty ) $, possessing the properties (7), is naturally called the fundamental function of the operator $ ( d / dt ) - A $. Thus, if $ A $ is the infinitesimal operator of a semi-group $ T $ of class $ l D ^ \prime $, then $ T $ is the fundamental function of the operator $ ( d / dt ) - A $. The converse statement is true under certain additional assumptions about the order of singularity of the fundamental function $ T $( or, more precisely, of the function $ f ( T ( \phi ) x ) $, where $ f \in X ^ \prime $).
A useful notion for the characterization of semi-groups in a locally convex space is that of the generalized resolvent. Let $ \widehat \phi $ denote the Laplace transform of a function $ \phi \in D ( \mathbf R ) $, and let $ \widehat{D} ( \mathbf R ) $ be the space of all such transforms. A topology is induced in this space, via the Laplace transform, from the topology of $ D ( \mathbf R ) $. The Laplace transform of an $ X $- valued generalized function $ F $ is defined by $ \widehat{F} ( \widehat \phi ) = F ( \phi ) $. Under these conditions, $ \widehat{F} $ is a continuous mapping of $ \widehat{D} ( \mathbf R ) $ into the space $ L ( X ) $ of continuous linear operators on $ X $. Let $ \widehat{D} {} _ + ^ \prime $ be the space of all $ \widehat{F} $ obtained from functions $ F $ with support in $ ( 0 , \infty ) $, with the natural topology. If $ A $ is a linear operator on $ X $, it can be "lifted" to an operator $ \widetilde{A} $ on $ \widehat{D} {} _ + ^ \prime $ via the equality
$$ ( \widetilde{A} \widehat{F} ) = A ( \widehat{F} ( \widehat \phi ) ) = A F ( \phi ) . $$
Thus, it is defined for all $ \widehat{F} \in \widehat{D} {} _ + ^ \prime $ such that the right-hand side of the equality is defined for any $ \phi \in D ( \mathbf R ) $ and it extends to a generalized function in $ \widehat{D} {} _ + ^ \prime $. The continuous operator $ \widetilde \lambda $ on $ \widehat{D} {} _ + ^ \prime $ is defined by
$$ ( \widetilde \lambda \widehat{F} ) ( \widehat \phi ) = \lambda \widehat{F} ( \widehat \phi ) = F ^ \prime ( \phi ) = - F ( \phi ^ \prime ) . $$
If the operator $ \widetilde{A} - \widetilde \lambda $ has a continuous inverse $ \widetilde{R} $ on $ \widehat{D} {} _ + ^ \prime $, then $ \widetilde{R} $ is called the generalized resolvent of $ A $.
An operator $ A $ has a generalized resolvent if and only if the operator $ ( d / dt ) - A $ has a locally equicontinuous fundamental function $ T $, constructed by the formula
$$ T ( \phi ) x = ( \widetilde{R} ( 1 \otimes x ) ) ( \widehat \phi ) ,\ \phi \in D ( \mathbf R ) ,\ \ x \in X , $$
where
$$ ( 1 \otimes x ) ( \widehat \phi ) = ( \delta \otimes x ) \phi = \phi ( 0 ) x . $$
Subject to certain additional assumptions, $ T $ is a distribution semi-group. An extension theorem for semi-groups of class $ l C _ {0} $ has also been proved in terms of generalized resolvents.
See also Semi-group of non-linear operators.
References
| [1] | E. Hille, R.S. Phillips, "Functional analysis and semi-groups" , Amer. Math. Soc. (1957) MR0089373 Zbl 0392.46001 Zbl 0033.06501 |
| [2] | Yu.M. Vuvunikyan, "Evolutionary representations of algebras of generalized functions" , Theory of operators in function spaces , Novosibirsk (1977) pp. 99–120 (In Russian) |
| [3] | P.P. Zabreiko, A.V. Zafievskii, "On a certain class of semigroups" Soviet Math. Dokl. , 10 : 6 (1969) pp. 1523–1526 Dokl. Akad. Nauk SSSR , 189 : 5 (1969) pp. 934–937 MR264459 |
| [4] | A.V. Zafievskii, Trudy Mat. Inst. Voronezh. Univ. , 1 (1970) pp. 206–210 |
| [5] | K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, §1 MR0617913 Zbl 0435.46002 |
| [6] | S.G. Krein, "Linear differential equations in Banach space" , Transl. Math. Monogr. , 29 , Amer. Math. Soc. (1971) (Translated from Russian) MR0342804 Zbl 0179.20701 |
| [7] | Yu.T. Sil'chenko, "An evolutionary equation with an operator generating a nonlinear semigroup" Differential Equations , 15 : 2 (1979) pp. 255–258 Differentsial'nye Uravneniya , 15 : 2 (1979) pp. 363–366 Zbl 0505.34046 |
| [8] | J. Chazarain, "Problèmes de Cauchy abstraits et applications à quelques problèmes mixtes" J. Funct. Anal. , 7 : 3 (1971) pp. 386–446 Zbl 0211.12902 |
| [9] | I. Ciorânescu, "La caracterisation spectrale d'opérateur, générateurs des semi-groupes distributions d'ordre fini de croissance" J. Math. Anal. Appl. , 34 (1971) pp. 34–41 |
| [10] | I. Ciorânescu, "A characterization of distribution semigroups of finite growth order" Rev. Roum. Math. Pures Appl. , 22 : 8 (1977) pp. 1053–1068 MR500280 Zbl 0374.46032 |
| [11] | T. Kato, "A characterization of holomorphic semigroups" Proc. Amer. Math. Soc. , 25 : 3 (1970) pp. 495–498 MR0264456 Zbl 0199.45604 |
| [12] | J. Lions, "Les semigroupes distributions" Portugal. Math. , 19 (1960) pp. 141–164 |
| [13] | A. Pazy, "On the differentiability and compactness of semi-groups of linear operators" J. Math. Mech. , 17 : 12 (1968) pp. 1131–1141 MR231242 Zbl 0162.45903 |
| [14] | A. Pazy, "Approximations of the identity operator by semigroups of linear operators" Proc. Amer. Math. Soc. , 30 : 1 (1971) pp. 147–150 MR0287362 |
| [15] | T. Ushijima, "On the abstract Cauchy problems and semi-groups of linear operators in locally convex spaces" Sci. Papers College Gen. Educ. Univ. Tokyo , 21 (1971) pp. 93–122 MR0312324 Zbl 0239.47031 |
| [16] | T. Ushijima, "On the generation and smoothness of semi-groups of linear operators" J. Fac. Sci. Univ. Tokyo, Sec. 1A , 19 : 1 (1972) pp. 65–127 MR0308854 Zbl 0239.47032 |
| [17] | C. Wild, "Semi-groupes de croissance 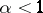 holomorphes" C.R. Acad. Sci. Paris Sér. A , 285 (1977) pp. 437–440 (English abstract) MR448159 Zbl 0359.47024 holomorphes" C.R. Acad. Sci. Paris Sér. A , 285 (1977) pp. 437–440 (English abstract) MR448159 Zbl 0359.47024 |
| [18] | J.A. Gol'dstein, "Semigroups of linear operators and application" , Oxford Univ. Press (1985) (Translated from Russian) |
| [19] | A. Pazy, "Semigroups of linear operators and application to partial differential equations" , Springer (1983) MR0710486 |
| [20] | Ph. Clément, H.J.A.M. Heijmans, S. Angenent, C.J. van Duijn, B. de Pagter, "One-parameter semigroups" , CWI Monographs , 5 , North-Holland (1987) MR0915552 Zbl 0636.47051 |
| [a1] | P. Butzer, H. Berens, "Semigroups of operators and approximation" , Springer (1967) MR230022 |
| [a2] | H. Kellermann, M. Hieber, "Integrated semigroups" J. Funct. Anal. , 84 (1989) pp. 160–180 MR0999494 Zbl 0604.47025 |
| [a3] | I. Miyadera, N. Tanaka, "Exponentially bounded $c$-semigroups and integrated semigroups" Tokyo J. Math. , 12 (1989) pp. 99–115 MR1001735 |
Semi-group of operators. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Semi-group_of_operators&oldid=24563