|
|
| Line 1: |
Line 1: |
| − | The name elliptic genus has been given to various multiplicative [[Cobordism|cobordism]] invariants taking values in a ring of modular forms. The following is an attempt to present the simplest case — level-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100701.png" /> genera in characteristic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100702.png" /> — in a unified way. It is convenient to use N. Katz's approach to modular forms (cf. [[#References|[a7]]]) and view a [[Modular form|modular form]] as a function of elliptic curves with a chosen invariant differential (cf. also [[Elliptic curve|Elliptic curve]]). A similar approach to elliptic genera was used by J. Franke [[#References|[a3]]]. | + | <!-- |
| | + | e1100701.png |
| | + | $#A+1 = 211 n = 2 |
| | + | $#C+1 = 211 : ~/encyclopedia/old_files/data/E110/E.1100070 Elliptic genera |
| | + | Automatically converted into TeX, above some diagnostics. |
| | + | Please remove this comment and the {{TEX|auto}} line below, |
| | + | if TeX found to be correct. |
| | + | --> |
| | + | |
| | + | {{TEX|auto}} |
| | + | {{TEX|done}} |
| | + | |
| | + | The name elliptic genus has been given to various multiplicative [[Cobordism|cobordism]] invariants taking values in a ring of modular forms. The following is an attempt to present the simplest case — level- $ 2 $ |
| | + | genera in characteristic $ \neq 2 $— |
| | + | in a unified way. It is convenient to use N. Katz's approach to modular forms (cf. [[#References|[a7]]]) and view a [[Modular form|modular form]] as a function of elliptic curves with a chosen invariant differential (cf. also [[Elliptic curve|Elliptic curve]]). A similar approach to elliptic genera was used by J. Franke [[#References|[a3]]]. |
| | | | |
| | ==Jacobi functions.== | | ==Jacobi functions.== |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100703.png" /> be any [[Perfect field|perfect field]] of characteristic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100704.png" /> and fix an algebraic closure <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100705.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100706.png" /> (cf. [[Algebraically closed field|Algebraically closed field]]). Consider a triple <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100707.png" /> consisting of: | + | Let $ K $ |
| | + | be any [[Perfect field|perfect field]] of characteristic $ \neq 2 $ |
| | + | and fix an algebraic closure $ {\overline{K}\; } $ |
| | + | of $ K $( |
| | + | cf. [[Algebraically closed field|Algebraically closed field]]). Consider a triple $ ( E, \omega, \alpha ) $ |
| | + | consisting of: |
| | | | |
| − | i) an elliptic curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100708.png" /> over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e1100709.png" />, i.e. a smooth curve of genus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007010.png" /> with a specified <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007011.png" />-rational base-point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007012.png" />; | + | i) an elliptic curve $ E $ |
| | + | over $ K $, |
| | + | i.e. a smooth curve of genus $ 1 $ |
| | + | with a specified $ K $- |
| | + | rational base-point $ O $; |
| | | | |
| − | ii) an invariant <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007013.png" />-rational differential <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007014.png" />; | + | ii) an invariant $ K $- |
| | + | rational differential $ \omega $; |
| | | | |
| − | iii) a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007015.png" />-rational primitive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007016.png" />-division point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007017.png" />. Following J.I. Igusa [[#References|[a6]]] (up to a point), one can associate to these data two functions, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007018.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007019.png" />, as follows. | + | iii) a $ K $- |
| | + | rational primitive $ 2 $- |
| | + | division point $ \alpha $. |
| | + | Following J.I. Igusa [[#References|[a6]]] (up to a point), one can associate to these data two functions, $ x $ |
| | + | and $ y $, |
| | + | as follows. |
| | | | |
| − | The set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007020.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007021.png" />-division points on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007022.png" /> can be described as follows. There are four <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007023.png" />-division points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007024.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007025.png" /> is one of them), four primitive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007026.png" />-division points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007027.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007028.png" />, and eight primitive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007029.png" />-division points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007030.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007031.png" />. Consider the degree-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007032.png" /> divisor <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007033.png" />. Since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007034.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007035.png" /> and since Galois symmetries transform <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007036.png" /> into itself, Abel's theorem (cf., for example, [[#References|[a11]]], III.3.5.1, or [[Abel theorem|Abel theorem]]) implies that there is a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007037.png" />, uniquely defined up to a multiplicative constant, such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007038.png" />. | + | The set $ E _ {4} \subset E ( {\overline{K}\; } ) $ |
| | + | of $ 4 $- |
| | + | division points on $ E $ |
| | + | can be described as follows. There are four $ 2 $- |
| | + | division points $ t $( |
| | + | $ \alpha $ |
| | + | is one of them), four primitive $ 4 $- |
| | + | division points $ r $ |
| | + | such that $ 2r = \alpha $, |
| | + | and eight primitive $ 4 $- |
| | + | division points $ s $ |
| | + | such that $ 2s \neq \alpha $. |
| | + | Consider the degree- $ 0 $ |
| | + | divisor $ D = \sum ( t ) - \sum ( r ) $. |
| | + | Since $ \sum t - \sum r = 0 $ |
| | + | in $ E $ |
| | + | and since Galois symmetries transform $ D $ |
| | + | into itself, Abel's theorem (cf., for example, [[#References|[a11]]], III.3.5.1, or [[Abel theorem|Abel theorem]]) implies that there is a function $ x \in K ( E ) ^ \times $, |
| | + | uniquely defined up to a multiplicative constant, such that $ { \mathop{\rm div} } ( x ) = D $. |
| | | | |
| − | The function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007039.png" /> is odd, satisfies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007040.png" />, and undergoes sign changes under the two other translations of exact order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007041.png" />. Moreover, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007042.png" /> satisfies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007043.png" />, then translation by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007044.png" /> transforms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007045.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007046.png" /> for some non-zero constant <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007047.png" />. This constant depends on the choice of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007048.png" /> but only up to sign. It follows that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007049.png" /> does not depend on the choice of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007050.png" />. This constant is written as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007051.png" />, i.e. | + | The function $ x $ |
| | + | is odd, satisfies $ x ( u + \alpha ) \equiv x ( u ) $, |
| | + | and undergoes sign changes under the two other translations of exact order $ 2 $. |
| | + | Moreover, if $ r \in E _ {4} $ |
| | + | satisfies $ 2r = \alpha $, |
| | + | then translation by $ r $ |
| | + | transforms $ x $ |
| | + | into $ Cx ^ {- 1 } $ |
| | + | for some non-zero constant $ C $. |
| | + | This constant depends on the choice of $ r $ |
| | + | but only up to sign. It follows that $ x ^ {2} ( u + r ) x ^ {2} ( u ) $ |
| | + | does not depend on the choice of $ r $. |
| | + | This constant is written as $ \varepsilon ^ {- 1 } $, |
| | + | i.e. |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007052.png" /></td> </tr></table>
| + | $$ |
| | + | \varepsilon \equiv x ^ {- 2 } ( u + r ) x ^ {- 2 } ( u ) . |
| | + | $$ |
| | | | |
| | One also defines | | One also defines |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007053.png" /></td> </tr></table>
| + | $$ |
| | + | \delta = { |
| | + | \frac{1}{8} |
| | + | } \sum x ^ {- 2 } ( s ) |
| | + | $$ |
| | | | |
| − | (the summation is over the primitive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007054.png" />-division points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007055.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007056.png" />). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007057.png" /> is one of the values of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007058.png" />, the other values are <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007059.png" />, each taken twice. It follows that | + | (the summation is over the primitive $ 4 $- |
| | + | division points $ s $ |
| | + | such that $ 2s \neq \alpha $). |
| | + | If $ a $ |
| | + | is one of the values of $ x ( s ) $, |
| | + | the other values are $ \pm a, \pm \varepsilon ^ {- {1 / 2 } } a ^ {- 1 } $, |
| | + | each taken twice. It follows that |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007060.png" /></td> </tr></table>
| + | $$ |
| | + | \delta = { |
| | + | \frac{1}{2} |
| | + | } ( a ^ {- 2 } + \varepsilon a ^ {2} ) |
| | + | $$ |
| | | | |
| | and | | and |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007061.png" /></td> </tr></table>
| + | $$ |
| | + | \prod ( X - x ( s ) ) = \varepsilon ^ {- 2 } ( 1 - 2 \delta X ^ {2} + \varepsilon X ^ {4} ) ^ {2} = \varepsilon ^ {- 2 } R ( X ) ^ {2} . |
| | + | $$ |
| | | | |
| | It is now easy to see that | | It is now easy to see that |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007062.png" /></td> </tr></table>
| + | $$ |
| | + | { \mathop{\rm div} } ( R ( x ) ) = 2 \left ( \sum ( s ) - 2 \sum ( r ) \right ) . |
| | + | $$ |
| | | | |
| − | Using once more Abel's theorem, one sees that there is a unique <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007063.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007064.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007065.png" />. Since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007066.png" />, one has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007067.png" />. | + | Using once more Abel's theorem, one sees that there is a unique $ y \in K ( E ) ^ \times $ |
| | + | such that $ { \mathop{\rm div} } ( y ) = \sum ( s ) - 2 \sum ( r ) $, |
| | + | and $ y ( O ) = 1 $. |
| | + | Since $ x ( O ) = 0 $, |
| | + | one has $ y ^ {2} = R ( x ) $. |
| | | | |
| − | The differential <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007068.png" /> has four double poles <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007069.png" />. Also, it is easy to see that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007070.png" /> is a double zero of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007071.png" />, hence a simple zero of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007072.png" />. One concludes that | + | The differential $ dx $ |
| | + | has four double poles $ r $. |
| | + | Also, it is easy to see that $ s $ |
| | + | is a double zero of $ x - x ( s ) $, |
| | + | hence a simple zero of $ dx $. |
| | + | One concludes that |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007073.png" /></td> </tr></table>
| + | $$ |
| | + | { \mathop{\rm div} } ( dx ) = \sum ( s ) - 2 \sum ( r ) = { \mathop{\rm div} } ( y ) . |
| | + | $$ |
| | | | |
| − | and that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007074.png" /> is an invariant differential on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007075.png" />. | + | and that $ { {dx } / y } $ |
| | + | is an invariant differential on $ E $. |
| | | | |
| | A slight modification of the argument given in [[#References|[a6]]] shows that the [[Jacobi elliptic functions|Jacobi elliptic functions]] satisfy the Euler addition formula | | A slight modification of the argument given in [[#References|[a6]]] shows that the [[Jacobi elliptic functions|Jacobi elliptic functions]] satisfy the Euler addition formula |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007076.png" /></td> </tr></table>
| + | $$ |
| | + | x ( u + v ) ( 1 - \varepsilon x ^ {2} ( u ) x ^ {2} ( v ) ) = x ( u ) y ( v ) + x ( v ) y ( u ) . |
| | + | $$ |
| | | | |
| − | Accordingly, one defines the Euler formal group law <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007077.png" /> by | + | Accordingly, one defines the Euler formal group law $ F ( U,V ) \in K [ [ U,V ] ] $ |
| | + | by |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007078.png" /></td> </tr></table>
| + | $$ |
| | + | F ( U,V ) = { |
| | + | \frac{U \sqrt {R ( V ) } + V \sqrt {R ( U ) } }{1 - \varepsilon U ^ {2} V ^ {2} } |
| | + | } . |
| | + | $$ |
| | | | |
| − | Notice that since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007079.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007080.png" /> is defined over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007081.png" />. | + | Notice that since $ { \mathop{\rm char} } K \neq 2 $, |
| | + | $ F ( U,V ) $ |
| | + | is defined over $ K $. |
| | | | |
| | ==The elliptic genus.== | | ==The elliptic genus.== |
| − | At this point, one normalizes <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007082.png" /> over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007083.png" /> by requiring that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007084.png" /> (the given invariant differential). All the objects <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007085.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007086.png" /> are now completely determined by the initial data. Replacing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007087.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007088.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007089.png" />) yields: | + | At this point, one normalizes $ x $ |
| | + | over $ K $ |
| | + | by requiring that $ { {dx } / y } = \omega $( |
| | + | the given invariant differential). All the objects $ x, y, \delta, \varepsilon $, |
| | + | and $ F ( U,V ) $ |
| | + | are now completely determined by the initial data. Replacing $ \omega $ |
| | + | by $ \lambda \omega $( |
| | + | $ \lambda \in K ^ \times $) |
| | + | yields: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007090.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a1)</td></tr></table>
| + | $$ \tag{a1 } |
| | + | x \asR \lambda x, \quad y \asR y, \quad \delta \asR \lambda ^ {- 2 } \delta, |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007091.png" /></td> </tr></table>
| + | $$ |
| | + | \varepsilon \asR \lambda ^ {- 4 } \varepsilon, \quad F ( U,V ) \asR \lambda F ( \lambda ^ {- 1 } U, \lambda ^ {- 1 } V ) . |
| | + | $$ |
| | | | |
| − | As any [[Formal group|formal group]] law, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007092.png" /> is classified by a unique ring homomorphism | + | As any [[Formal group|formal group]] law, $ F ( U,V ) $ |
| | + | is classified by a unique ring homomorphism |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007093.png" /></td> </tr></table>
| + | $$ |
| | + | \psi : {\Omega _ {*} ^ { { \mathop{\rm U} } } } \rightarrow K |
| | + | $$ |
| | | | |
| − | from the complex cobordism ring. Since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007094.png" />, it is easy to see that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007095.png" /> uniquely factors through a ring homomorphism | + | from the complex cobordism ring. Since $ F ( - U, - V ) = - F ( U,V ) $, |
| | + | it is easy to see that $ \psi $ |
| | + | uniquely factors through a ring homomorphism |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007096.png" /></td> </tr></table>
| + | $$ |
| | + | \varphi : {\Omega _ {*} ^ { { \mathop{\rm SO} } } } \rightarrow K |
| | + | $$ |
| | | | |
| − | from the oriented cobordism ring. By definition, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007097.png" /> is the level-<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e11007099.png" /> elliptic genus. Suppose now that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070100.png" />. Define a local parameter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070101.png" /> near <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070102.png" /> so that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070103.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070104.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070105.png" /> can be expanded into a formal power series <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070106.png" /> which clearly satisfies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070107.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070108.png" />. In this case, the elliptic genus can be defined as the Hirzebruch genus (cf. [[#References|[a4]]] or [[#References|[a5]]]) corresponding to the series <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070109.png" />. Since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070110.png" />, the logarithm <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070111.png" /> of this elliptic genus is given by the [[Elliptic integral|elliptic integral]] | + | from the oriented cobordism ring. By definition, $ \varphi $ |
| | + | is the level- $ 2 $ |
| | + | elliptic genus. Suppose now that $ { \mathop{\rm char} } K = 0 $. |
| | + | Define a local parameter $ z $ |
| | + | near $ O $ |
| | + | so that $ z ( O ) = 0 $ |
| | + | and $ dz = \omega $. |
| | + | Then $ x $ |
| | + | can be expanded into a formal power series $ x ( z ) \in K [ [ z ] ] $ |
| | + | which clearly satisfies $ x ( z ) = z + o ( z ) $ |
| | + | and $ x ( - z ) = - x ( z ) $. |
| | + | In this case, the elliptic genus can be defined as the Hirzebruch genus (cf. [[#References|[a4]]] or [[#References|[a5]]]) corresponding to the series $ P ( z ) = {z / {x ( z ) } } $. |
| | + | Since $ { {d x ( z ) } / {dz } } = y ( z ) $, |
| | + | the logarithm $ g ( z ) $ |
| | + | of this elliptic genus is given by the [[Elliptic integral|elliptic integral]] |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070112.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a2)</td></tr></table>
| + | $$ \tag{a2 } |
| | + | g ( z ) = \int\limits _ { 0 } ^ { z } { |
| | + | \frac{dt }{\sqrt {1 - 2 \delta t ^ {2} + \varepsilon t ^ {4} } } |
| | + | } , |
| | + | $$ |
| | | | |
| | which gives the original definition in [[#References|[a9]]]. | | which gives the original definition in [[#References|[a9]]]. |
| | | | |
| | ==Modularity.== | | ==Modularity.== |
| − | For any closed oriented [[Manifold|manifold]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070113.png" /> of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070114.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070115.png" /> is a function of the triple <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070116.png" />. As easily follows from (a1), multiplying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070117.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070118.png" /> results in multiplying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070119.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070120.png" />. Also, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070121.png" /> depends only on the isomorphism class of the triple <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070122.png" /> and commutes with arbitrary extensions of the scalar field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070123.png" />. In the terminology of Katz ([[#References|[a7]]]; adapted here to modular forms over fields), <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070124.png" /> is a [[Modular form|modular form]] of level <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070125.png" /> and weight <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070126.png" />. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070127.png" /> be the graded ring of all such modular forms. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070128.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070129.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070130.png" />. Moreover, one can prove that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070131.png" />. If one identifies these two isomorphic rings, the elliptic genus becomes the Hirzebruch genus | + | For any closed oriented [[Manifold|manifold]] $ M $ |
| | + | of dimension $ 4k $, |
| | + | $ \varphi ( M ) $ |
| | + | is a function of the triple $ ( E, \omega, \alpha ) $. |
| | + | As easily follows from (a1), multiplying $ \omega $ |
| | + | by $ \lambda $ |
| | + | results in multiplying $ \varphi ( M ) $ |
| | + | by $ \lambda ^ {- 2k } $. |
| | + | Also, $ \varphi ( M ) $ |
| | + | depends only on the isomorphism class of the triple $ ( E, \omega, \alpha ) $ |
| | + | and commutes with arbitrary extensions of the scalar field $ K $. |
| | + | In the terminology of Katz ([[#References|[a7]]]; adapted here to modular forms over fields), $ \varphi ( M ) $ |
| | + | is a [[Modular form|modular form]] of level $ 2 $ |
| | + | and weight $ 2k $. |
| | + | Let $ {\mathcal M} _ {*} $ |
| | + | be the graded ring of all such modular forms. Then $ \varphi ( M ) \in {\mathcal M} _ {2k } $, |
| | + | $ \delta \in {\mathcal M} _ {2} $, |
| | + | $ \varepsilon \in {\mathcal M} _ {4} $. |
| | + | Moreover, one can prove that $ {\mathcal M} _ {*} \cong \mathbf Z [ {1 / 2 } , \delta, \varepsilon ] $. |
| | + | If one identifies these two isomorphic rings, the elliptic genus becomes the Hirzebruch genus |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070132.png" /></td> </tr></table>
| + | $$ |
| | + | \varphi : {\Omega _ {*} ^ { { \mathop{\rm SO} } } } \rightarrow {\mathbf Z [ {1 / 2 } , \delta, \varepsilon ] } |
| | + | $$ |
| | | | |
| | with logarithm given by the formal integral (a2). | | with logarithm given by the formal integral (a2). |
| Line 81: |
Line 240: |
| | Consider | | Consider |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070133.png" /></td> </tr></table>
| + | $$ |
| | + | { {\widetilde \varphi } } : {\Omega _ {*} ^ { { \mathop{\rm Spin} } } } \rightarrow { {\mathcal M} _ {*} } , |
| | + | $$ |
| | | | |
| − | i.e., the composition of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070134.png" /> with the forgetful homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070135.png" />. As is shown in [[#References|[a2]]], | + | i.e., the composition of $ \varphi $ |
| | + | with the forgetful homomorphism $ \Omega _ {*} ^ { { \mathop{\rm Spin} } } \rightarrow \Omega _ {*} ^ { { \mathop{\rm SO} } } $. |
| | + | As is shown in [[#References|[a2]]], |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070136.png" /></td> </tr></table>
| + | $$ |
| | + | {\widetilde \varphi } ( \Omega _ {*} ^ { { \mathop{\rm Spin} } } ) = \mathbf Z [ 8 \delta, \varepsilon ] . |
| | + | $$ |
| | | | |
| − | The ring <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070137.png" /> agrees with the ring <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070138.png" /> of modular forms over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070139.png" />. Thus: If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070140.png" /> is a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070141.png" />-manifold of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070142.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070143.png" />. | + | The ring $ \mathbf Z [ 8 \delta, \varepsilon ] $ |
| | + | agrees with the ring $ {\mathcal M} _ {*} ( \mathbf Z ) $ |
| | + | of modular forms over $ \mathbf Z $. |
| | + | Thus: If $ M $ |
| | + | is a $ { \mathop{\rm Spin} } $- |
| | + | manifold of dimension $ 4k $, |
| | + | then $ \varphi ( M ) \in {\mathcal M} _ {2k } ( \mathbf Z ) $. |
| | | | |
| | ==Example: the Tate curve.== | | ==Example: the Tate curve.== |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070144.png" /> be a [[Local field|local field]], complete with respect to a discrete [[Valuation|valuation]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070145.png" />, and let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070146.png" /> be any element satisfying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070147.png" />. Consider <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070148.png" />. It is well-known (cf. [[#References|[a11]]], § C.14) that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070149.png" /> can be identified with the elliptic curve (known as the Tate curve) | + | Let $ K $ |
| | + | be a [[Local field|local field]], complete with respect to a discrete [[Valuation|valuation]] $ v $, |
| | + | and let $ q \in K ^ \times $ |
| | + | be any element satisfying $ v ( q ) < 0 $. |
| | + | Consider $ E = K ^ \times /q ^ {2 \mathbf Z } $. |
| | + | It is well-known (cf. [[#References|[a11]]], § C.14) that $ E $ |
| | + | can be identified with the elliptic curve (known as the Tate curve) |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070150.png" /></td> </tr></table>
| + | $$ |
| | + | E _ {q ^ {2} } : Y ^ {2} + XY = X ^ {3} + a _ {4} X + a _ {6} , |
| | + | $$ |
| | | | |
| | where | | where |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070151.png" /></td> </tr></table>
| + | $$ |
| | + | a _ {4} = \sum _ {m \geq 1 } ( - 5m ^ {3} ) { |
| | + | \frac{q ^ {2m } }{1 - q ^ {2m } } |
| | + | } , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070152.png" /></td> </tr></table>
| + | $$ |
| | + | a _ {6} = \sum _ {m \geq 1 } \left ( - { |
| | + | \frac{5m ^ {3} + 7m ^ {5} }{12 } |
| | + | } \right ) { |
| | + | \frac{q ^ {2m } }{1 - q ^ {2m } } |
| | + | } . |
| | + | $$ |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070153.png" /> can be treated as an elliptic curve over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070154.png" /> with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070155.png" />. Fix the invariant differential <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070156.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070157.png" />) on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070158.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070159.png" /> corresponds to the differential <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070160.png" /> on the Tate curve). <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070161.png" /> has three <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070162.png" />-rational primitive <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070163.png" />-division points: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070164.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070165.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070166.png" />. To describe the corresponding Jacobi function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070167.png" />, consider the [[Theta-function|theta-function]]
| + | $ E $ |
| | + | can be treated as an elliptic curve over $ K $ |
| | + | with $ O = 1 $. |
| | + | Fix the invariant differential $ \omega = { {du } / u } $( |
| | + | $ u \in K ^ \times $) |
| | + | on $ E $( |
| | + | $ \omega $ |
| | + | corresponds to the differential $ \omega _ {\textrm{ can } } = { {dX } / {( 2Y + X ) } } $ |
| | + | on the Tate curve). $ E $ |
| | + | has three $ K $- |
| | + | rational primitive $ 2 $- |
| | + | division points: $ - 1 $, |
| | + | $ q $ |
| | + | and $ - q $. |
| | + | To describe the corresponding Jacobi function $ x $, |
| | + | consider the [[Theta-function|theta-function]] |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070168.png" /></td> </tr></table>
| + | $$ |
| | + | \Theta ( u ) = ( 1 - u ^ {- 2 } ) \prod _ {n > 0 } ( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) . |
| | + | $$ |
| | | | |
| − | This is a "holomorphic" function on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070169.png" /> with simple zeros at points of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070170.png" /> (cf. [[#References|[a10]]] for a justification of this terminology), satisfying | + | This is a "holomorphic" function on $ K ^ \times $ |
| | + | with simple zeros at points of $ \pm q ^ {\mathbf Z} $( |
| | + | cf. [[#References|[a10]]] for a justification of this terminology), satisfying |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070171.png" /></td> </tr></table>
| + | $$ |
| | + | \Theta ( - u ) = \Theta ( u ) , \quad \Theta ( q ^ {- 1 } u ) = - u ^ {2} \Theta ( u ) . |
| | + | $$ |
| | | | |
| − | Consider the case where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070172.png" />. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070173.png" /> be any square root of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070174.png" />, and let | + | Consider the case where $ \alpha = - 1 $. |
| | + | Let $ i \in {\overline{K}\; } $ |
| | + | be any square root of $ - 1 $, |
| | + | and let |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070175.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a3)</td></tr></table>
| + | $$ \tag{a3 } |
| | + | f ( u ) = { |
| | + | \frac{\Theta ( u ) }{\Theta ( iu ) } |
| | + | } = |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070176.png" /></td> </tr></table>
| + | $$ |
| | + | = |
| | + | { |
| | + | \frac{u ^ {2} - 1 }{u ^ {2} + 1 } |
| | + | } \prod _ {n > 0 } { |
| | + | \frac{( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) }{( 1 + q ^ {2n } u ^ {- 2 } ) ( 1 + q ^ {2n } u ^ {2} ) } |
| | + | } . |
| | + | $$ |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070177.png" /> is a [[Meromorphic function|meromorphic function]] on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070178.png" /> satisfying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070179.png" /> and
| + | $ f $ |
| | + | is a [[Meromorphic function|meromorphic function]] on $ E $ |
| | + | satisfying $ f ( iu ) = {1 / {f ( u ) } } $ |
| | + | and |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070180.png" /></td> </tr></table>
| + | $$ |
| | + | { \mathop{\rm div} } ( f ) = ( 1 ) + ( - 1 ) + ( q ) + ( - q ) + |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070181.png" /></td> </tr></table>
| + | $$ |
| | + | - ( i ) - ( - i ) - ( iq ) - ( - iq ) , |
| | + | $$ |
| | | | |
| − | i.e., <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070182.png" /> is a multiple of the Jacobi function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070183.png" /> of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070184.png" />. | + | i.e., $ f $ |
| | + | is a multiple of the Jacobi function $ x $ |
| | + | of $ ( E, \omega, - 1 ) $. |
| | | | |
| − | Notice now that the normalization condition <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070185.png" /> can be written as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070186.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070187.png" /> is the derivative with respect to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070188.png" />. Since <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070189.png" />, one has <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070190.png" />. Differentiating (a3), one obtains | + | Notice now that the normalization condition $ { {du } / u } = { {dx } / y } $ |
| | + | can be written as $ y ( u ) = ux ^ \prime ( u ) $, |
| | + | where $ x ^ \prime ( u ) $ |
| | + | is the derivative with respect to $ u $. |
| | + | Since $ y ( 1 ) = 0 $, |
| | + | one has $ x ^ \prime ( 1 ) = 1 $. |
| | + | Differentiating (a3), one obtains |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070191.png" /></td> </tr></table>
| + | $$ |
| | + | f ^ \prime ( 1 ) = \prod _ {n > 0 } \left ( { |
| | + | \frac{1 - q ^ {2n } }{1 + q ^ {2n } } |
| | + | } \right ) ^ {2} , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070192.png" /></td> </tr></table>
| + | $$ |
| | + | x ( u ) = { |
| | + | \frac{u ^ {2} - 1 }{u ^ {2} + 1 } |
| | + | } \prod _ {n > 0 } { |
| | + | \frac{( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) ( 1 + q ^ {2n } ) ^ {2} }{( 1 + q ^ {2n } u ^ {- 2 } ) ( 1 + q ^ {2n } u ^ {2} ) ( 1 - q ^ {2n } ) ^ {2} } |
| | + | } , |
| | + | $$ |
| | | | |
| | and | | and |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070193.png" /></td> </tr></table>
| + | $$ |
| | + | \varepsilon = \prod _ {n > 0 } \left ( { |
| | + | \frac{1 - q ^ {2n } }{1 + q ^ {2n } } |
| | + | } \right ) ^ {8} . |
| | + | $$ |
| | | | |
| − | Finally, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070194.png" />, the function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070195.png" /> satisfies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070196.png" />. It follows that the generating series <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070197.png" /> is given by | + | Finally, if $ { \mathop{\rm char} } K = 0 $, |
| | + | the function $ z = { \mathop{\rm log} } u $ |
| | + | satisfies $ dz = { {du } / u } $. |
| | + | It follows that the generating series $ P ( z ) = {z / {x ( z ) } } $ |
| | + | is given by |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070198.png" /></td> </tr></table>
| + | $$ |
| | + | P ( z ) = |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070199.png" /></td> </tr></table>
| + | $$ |
| | + | = |
| | + | { |
| | + | \frac{z}{ { \mathop{\rm tanh} } z } |
| | + | } \prod _ {n > 0 } { |
| | + | \frac{( 1 + q ^ {2n } e ^ {- 2z } ) ( 1 + q ^ {2n } e ^ {2z } ) ( 1 - q ^ {2n } ) ^ {2} }{( 1 - q ^ {2n } e ^ {- 2z } ) ( 1 - q ^ {2n } e ^ {2z } ) ( 1 + q ^ {2n } ) ^ {2} } |
| | + | } . |
| | + | $$ |
| | | | |
| − | The cases where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070200.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070201.png" /> are treated similarly, with | + | The cases where $ \alpha = q $ |
| | + | or $ \alpha = - q $ |
| | + | are treated similarly, with |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070202.png" /></td> </tr></table>
| + | $$ |
| | + | f ( u ) = { |
| | + | \frac{u \Theta ( u ) }{\Theta ( q ^ {- 1/2 } u ) } |
| | + | } |
| | + | $$ |
| | | | |
| | and | | and |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070203.png" /></td> </tr></table>
| + | $$ |
| | + | f ( u ) = { |
| | + | \frac{u \Theta ( u ) }{\Theta ( iq ^ {- 1/2 } u ) } |
| | + | } , |
| | + | $$ |
| | | | |
| | respectively. | | respectively. |
| | | | |
| | ==Strict multiplicativity.== | | ==Strict multiplicativity.== |
| − | The following theorem, also known (in an equivalent form) as the Witten conjecture, was proven first by C. Taubes [[#References|[a12]]], then by R. Bott and Taubes [[#References|[a1]]]. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070204.png" /> be a principal <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070206.png" />-bundle (cf. also [[Principal G-object|Principal <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070207.png" />-object]]) over an oriented manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070208.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070209.png" /> is a compact connected [[Lie group|Lie group]], and suppose <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070210.png" /> acts on a compact <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070211.png" />-manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070212.png" />. Then | + | The following theorem, also known (in an equivalent form) as the Witten conjecture, was proven first by C. Taubes [[#References|[a12]]], then by R. Bott and Taubes [[#References|[a1]]]. Let $ P $ |
| | + | be a principal $ G $- |
| | + | bundle (cf. also [[Principal G-object|Principal $ G $- |
| | + | object]]) over an oriented manifold $ B $, |
| | + | where $ G $ |
| | + | is a compact connected [[Lie group|Lie group]], and suppose $ G $ |
| | + | acts on a compact $ { \mathop{\rm Spin} } $- |
| | + | manifold $ M $. |
| | + | Then |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/e/e110/e110070/e110070213.png" /></td> </tr></table>
| + | $$ |
| | + | \varphi ( P \times _ {G} M ) = \varphi ( B ) \varphi ( M ) . |
| | + | $$ |
| | | | |
| | For the history of this conjecture, cf. [[#References|[a8]]]. | | For the history of this conjecture, cf. [[#References|[a8]]]. |
The name elliptic genus has been given to various multiplicative cobordism invariants taking values in a ring of modular forms. The following is an attempt to present the simplest case — level- $ 2 $
genera in characteristic $ \neq 2 $—
in a unified way. It is convenient to use N. Katz's approach to modular forms (cf. [a7]) and view a modular form as a function of elliptic curves with a chosen invariant differential (cf. also Elliptic curve). A similar approach to elliptic genera was used by J. Franke [a3].
Jacobi functions.
Let $ K $
be any perfect field of characteristic $ \neq 2 $
and fix an algebraic closure $ {\overline{K}\; } $
of $ K $(
cf. Algebraically closed field). Consider a triple $ ( E, \omega, \alpha ) $
consisting of:
i) an elliptic curve $ E $
over $ K $,
i.e. a smooth curve of genus $ 1 $
with a specified $ K $-
rational base-point $ O $;
ii) an invariant $ K $-
rational differential $ \omega $;
iii) a $ K $-
rational primitive $ 2 $-
division point $ \alpha $.
Following J.I. Igusa [a6] (up to a point), one can associate to these data two functions, $ x $
and $ y $,
as follows.
The set $ E _ {4} \subset E ( {\overline{K}\; } ) $
of $ 4 $-
division points on $ E $
can be described as follows. There are four $ 2 $-
division points $ t $(
$ \alpha $
is one of them), four primitive $ 4 $-
division points $ r $
such that $ 2r = \alpha $,
and eight primitive $ 4 $-
division points $ s $
such that $ 2s \neq \alpha $.
Consider the degree- $ 0 $
divisor $ D = \sum ( t ) - \sum ( r ) $.
Since $ \sum t - \sum r = 0 $
in $ E $
and since Galois symmetries transform $ D $
into itself, Abel's theorem (cf., for example, [a11], III.3.5.1, or Abel theorem) implies that there is a function $ x \in K ( E ) ^ \times $,
uniquely defined up to a multiplicative constant, such that $ { \mathop{\rm div} } ( x ) = D $.
The function $ x $
is odd, satisfies $ x ( u + \alpha ) \equiv x ( u ) $,
and undergoes sign changes under the two other translations of exact order $ 2 $.
Moreover, if $ r \in E _ {4} $
satisfies $ 2r = \alpha $,
then translation by $ r $
transforms $ x $
into $ Cx ^ {- 1 } $
for some non-zero constant $ C $.
This constant depends on the choice of $ r $
but only up to sign. It follows that $ x ^ {2} ( u + r ) x ^ {2} ( u ) $
does not depend on the choice of $ r $.
This constant is written as $ \varepsilon ^ {- 1 } $,
i.e.
$$
\varepsilon \equiv x ^ {- 2 } ( u + r ) x ^ {- 2 } ( u ) .
$$
One also defines
$$
\delta = {
\frac{1}{8}
} \sum x ^ {- 2 } ( s )
$$
(the summation is over the primitive $ 4 $-
division points $ s $
such that $ 2s \neq \alpha $).
If $ a $
is one of the values of $ x ( s ) $,
the other values are $ \pm a, \pm \varepsilon ^ {- {1 / 2 } } a ^ {- 1 } $,
each taken twice. It follows that
$$
\delta = {
\frac{1}{2}
} ( a ^ {- 2 } + \varepsilon a ^ {2} )
$$
and
$$
\prod ( X - x ( s ) ) = \varepsilon ^ {- 2 } ( 1 - 2 \delta X ^ {2} + \varepsilon X ^ {4} ) ^ {2} = \varepsilon ^ {- 2 } R ( X ) ^ {2} .
$$
It is now easy to see that
$$
{ \mathop{\rm div} } ( R ( x ) ) = 2 \left ( \sum ( s ) - 2 \sum ( r ) \right ) .
$$
Using once more Abel's theorem, one sees that there is a unique $ y \in K ( E ) ^ \times $
such that $ { \mathop{\rm div} } ( y ) = \sum ( s ) - 2 \sum ( r ) $,
and $ y ( O ) = 1 $.
Since $ x ( O ) = 0 $,
one has $ y ^ {2} = R ( x ) $.
The differential $ dx $
has four double poles $ r $.
Also, it is easy to see that $ s $
is a double zero of $ x - x ( s ) $,
hence a simple zero of $ dx $.
One concludes that
$$
{ \mathop{\rm div} } ( dx ) = \sum ( s ) - 2 \sum ( r ) = { \mathop{\rm div} } ( y ) .
$$
and that $ { {dx } / y } $
is an invariant differential on $ E $.
A slight modification of the argument given in [a6] shows that the Jacobi elliptic functions satisfy the Euler addition formula
$$
x ( u + v ) ( 1 - \varepsilon x ^ {2} ( u ) x ^ {2} ( v ) ) = x ( u ) y ( v ) + x ( v ) y ( u ) .
$$
Accordingly, one defines the Euler formal group law $ F ( U,V ) \in K [ [ U,V ] ] $
by
$$
F ( U,V ) = {
\frac{U \sqrt {R ( V ) } + V \sqrt {R ( U ) } }{1 - \varepsilon U ^ {2} V ^ {2} }
} .
$$
Notice that since $ { \mathop{\rm char} } K \neq 2 $,
$ F ( U,V ) $
is defined over $ K $.
The elliptic genus.
At this point, one normalizes $ x $
over $ K $
by requiring that $ { {dx } / y } = \omega $(
the given invariant differential). All the objects $ x, y, \delta, \varepsilon $,
and $ F ( U,V ) $
are now completely determined by the initial data. Replacing $ \omega $
by $ \lambda \omega $(
$ \lambda \in K ^ \times $)
yields:
$$ \tag{a1 }
x \asR \lambda x, \quad y \asR y, \quad \delta \asR \lambda ^ {- 2 } \delta,
$$
$$
\varepsilon \asR \lambda ^ {- 4 } \varepsilon, \quad F ( U,V ) \asR \lambda F ( \lambda ^ {- 1 } U, \lambda ^ {- 1 } V ) .
$$
As any formal group law, $ F ( U,V ) $
is classified by a unique ring homomorphism
$$
\psi : {\Omega _ {*} ^ { { \mathop{\rm U} } } } \rightarrow K
$$
from the complex cobordism ring. Since $ F ( - U, - V ) = - F ( U,V ) $,
it is easy to see that $ \psi $
uniquely factors through a ring homomorphism
$$
\varphi : {\Omega _ {*} ^ { { \mathop{\rm SO} } } } \rightarrow K
$$
from the oriented cobordism ring. By definition, $ \varphi $
is the level- $ 2 $
elliptic genus. Suppose now that $ { \mathop{\rm char} } K = 0 $.
Define a local parameter $ z $
near $ O $
so that $ z ( O ) = 0 $
and $ dz = \omega $.
Then $ x $
can be expanded into a formal power series $ x ( z ) \in K [ [ z ] ] $
which clearly satisfies $ x ( z ) = z + o ( z ) $
and $ x ( - z ) = - x ( z ) $.
In this case, the elliptic genus can be defined as the Hirzebruch genus (cf. [a4] or [a5]) corresponding to the series $ P ( z ) = {z / {x ( z ) } } $.
Since $ { {d x ( z ) } / {dz } } = y ( z ) $,
the logarithm $ g ( z ) $
of this elliptic genus is given by the elliptic integral
$$ \tag{a2 }
g ( z ) = \int\limits _ { 0 } ^ { z } {
\frac{dt }{\sqrt {1 - 2 \delta t ^ {2} + \varepsilon t ^ {4} } }
} ,
$$
which gives the original definition in [a9].
Modularity.
For any closed oriented manifold $ M $
of dimension $ 4k $,
$ \varphi ( M ) $
is a function of the triple $ ( E, \omega, \alpha ) $.
As easily follows from (a1), multiplying $ \omega $
by $ \lambda $
results in multiplying $ \varphi ( M ) $
by $ \lambda ^ {- 2k } $.
Also, $ \varphi ( M ) $
depends only on the isomorphism class of the triple $ ( E, \omega, \alpha ) $
and commutes with arbitrary extensions of the scalar field $ K $.
In the terminology of Katz ([a7]; adapted here to modular forms over fields), $ \varphi ( M ) $
is a modular form of level $ 2 $
and weight $ 2k $.
Let $ {\mathcal M} _ {*} $
be the graded ring of all such modular forms. Then $ \varphi ( M ) \in {\mathcal M} _ {2k } $,
$ \delta \in {\mathcal M} _ {2} $,
$ \varepsilon \in {\mathcal M} _ {4} $.
Moreover, one can prove that $ {\mathcal M} _ {*} \cong \mathbf Z [ {1 / 2 } , \delta, \varepsilon ] $.
If one identifies these two isomorphic rings, the elliptic genus becomes the Hirzebruch genus
$$
\varphi : {\Omega _ {*} ^ { { \mathop{\rm SO} } } } \rightarrow {\mathbf Z [ {1 / 2 } , \delta, \varepsilon ] }
$$
with logarithm given by the formal integral (a2).
Integrality.
Consider
$$
{ {\widetilde \varphi } } : {\Omega _ {*} ^ { { \mathop{\rm Spin} } } } \rightarrow { {\mathcal M} _ {*} } ,
$$
i.e., the composition of $ \varphi $
with the forgetful homomorphism $ \Omega _ {*} ^ { { \mathop{\rm Spin} } } \rightarrow \Omega _ {*} ^ { { \mathop{\rm SO} } } $.
As is shown in [a2],
$$
{\widetilde \varphi } ( \Omega _ {*} ^ { { \mathop{\rm Spin} } } ) = \mathbf Z [ 8 \delta, \varepsilon ] .
$$
The ring $ \mathbf Z [ 8 \delta, \varepsilon ] $
agrees with the ring $ {\mathcal M} _ {*} ( \mathbf Z ) $
of modular forms over $ \mathbf Z $.
Thus: If $ M $
is a $ { \mathop{\rm Spin} } $-
manifold of dimension $ 4k $,
then $ \varphi ( M ) \in {\mathcal M} _ {2k } ( \mathbf Z ) $.
Example: the Tate curve.
Let $ K $
be a local field, complete with respect to a discrete valuation $ v $,
and let $ q \in K ^ \times $
be any element satisfying $ v ( q ) < 0 $.
Consider $ E = K ^ \times /q ^ {2 \mathbf Z } $.
It is well-known (cf. [a11], § C.14) that $ E $
can be identified with the elliptic curve (known as the Tate curve)
$$
E _ {q ^ {2} } : Y ^ {2} + XY = X ^ {3} + a _ {4} X + a _ {6} ,
$$
where
$$
a _ {4} = \sum _ {m \geq 1 } ( - 5m ^ {3} ) {
\frac{q ^ {2m } }{1 - q ^ {2m } }
} ,
$$
$$
a _ {6} = \sum _ {m \geq 1 } \left ( - {
\frac{5m ^ {3} + 7m ^ {5} }{12 }
} \right ) {
\frac{q ^ {2m } }{1 - q ^ {2m } }
} .
$$
$ E $
can be treated as an elliptic curve over $ K $
with $ O = 1 $.
Fix the invariant differential $ \omega = { {du } / u } $(
$ u \in K ^ \times $)
on $ E $(
$ \omega $
corresponds to the differential $ \omega _ {\textrm{ can } } = { {dX } / {( 2Y + X ) } } $
on the Tate curve). $ E $
has three $ K $-
rational primitive $ 2 $-
division points: $ - 1 $,
$ q $
and $ - q $.
To describe the corresponding Jacobi function $ x $,
consider the theta-function
$$
\Theta ( u ) = ( 1 - u ^ {- 2 } ) \prod _ {n > 0 } ( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) .
$$
This is a "holomorphic" function on $ K ^ \times $
with simple zeros at points of $ \pm q ^ {\mathbf Z} $(
cf. [a10] for a justification of this terminology), satisfying
$$
\Theta ( - u ) = \Theta ( u ) , \quad \Theta ( q ^ {- 1 } u ) = - u ^ {2} \Theta ( u ) .
$$
Consider the case where $ \alpha = - 1 $.
Let $ i \in {\overline{K}\; } $
be any square root of $ - 1 $,
and let
$$ \tag{a3 }
f ( u ) = {
\frac{\Theta ( u ) }{\Theta ( iu ) }
} =
$$
$$
=
{
\frac{u ^ {2} - 1 }{u ^ {2} + 1 }
} \prod _ {n > 0 } {
\frac{( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) }{( 1 + q ^ {2n } u ^ {- 2 } ) ( 1 + q ^ {2n } u ^ {2} ) }
} .
$$
$ f $
is a meromorphic function on $ E $
satisfying $ f ( iu ) = {1 / {f ( u ) } } $
and
$$
{ \mathop{\rm div} } ( f ) = ( 1 ) + ( - 1 ) + ( q ) + ( - q ) +
$$
$$
- ( i ) - ( - i ) - ( iq ) - ( - iq ) ,
$$
i.e., $ f $
is a multiple of the Jacobi function $ x $
of $ ( E, \omega, - 1 ) $.
Notice now that the normalization condition $ { {du } / u } = { {dx } / y } $
can be written as $ y ( u ) = ux ^ \prime ( u ) $,
where $ x ^ \prime ( u ) $
is the derivative with respect to $ u $.
Since $ y ( 1 ) = 0 $,
one has $ x ^ \prime ( 1 ) = 1 $.
Differentiating (a3), one obtains
$$
f ^ \prime ( 1 ) = \prod _ {n > 0 } \left ( {
\frac{1 - q ^ {2n } }{1 + q ^ {2n } }
} \right ) ^ {2} ,
$$
$$
x ( u ) = {
\frac{u ^ {2} - 1 }{u ^ {2} + 1 }
} \prod _ {n > 0 } {
\frac{( 1 - q ^ {2n } u ^ {- 2 } ) ( 1 - q ^ {2n } u ^ {2} ) ( 1 + q ^ {2n } ) ^ {2} }{( 1 + q ^ {2n } u ^ {- 2 } ) ( 1 + q ^ {2n } u ^ {2} ) ( 1 - q ^ {2n } ) ^ {2} }
} ,
$$
and
$$
\varepsilon = \prod _ {n > 0 } \left ( {
\frac{1 - q ^ {2n } }{1 + q ^ {2n } }
} \right ) ^ {8} .
$$
Finally, if $ { \mathop{\rm char} } K = 0 $,
the function $ z = { \mathop{\rm log} } u $
satisfies $ dz = { {du } / u } $.
It follows that the generating series $ P ( z ) = {z / {x ( z ) } } $
is given by
$$
P ( z ) =
$$
$$
=
{
\frac{z}{ { \mathop{\rm tanh} } z }
} \prod _ {n > 0 } {
\frac{( 1 + q ^ {2n } e ^ {- 2z } ) ( 1 + q ^ {2n } e ^ {2z } ) ( 1 - q ^ {2n } ) ^ {2} }{( 1 - q ^ {2n } e ^ {- 2z } ) ( 1 - q ^ {2n } e ^ {2z } ) ( 1 + q ^ {2n } ) ^ {2} }
} .
$$
The cases where $ \alpha = q $
or $ \alpha = - q $
are treated similarly, with
$$
f ( u ) = {
\frac{u \Theta ( u ) }{\Theta ( q ^ {- 1/2 } u ) }
}
$$
and
$$
f ( u ) = {
\frac{u \Theta ( u ) }{\Theta ( iq ^ {- 1/2 } u ) }
} ,
$$
respectively.
Strict multiplicativity.
The following theorem, also known (in an equivalent form) as the Witten conjecture, was proven first by C. Taubes [a12], then by R. Bott and Taubes [a1]. Let $ P $
be a principal $ G $-
bundle (cf. also Principal $ G $-
object) over an oriented manifold $ B $,
where $ G $
is a compact connected Lie group, and suppose $ G $
acts on a compact $ { \mathop{\rm Spin} } $-
manifold $ M $.
Then
$$
\varphi ( P \times _ {G} M ) = \varphi ( B ) \varphi ( M ) .
$$
For the history of this conjecture, cf. [a8].
References
| [a1] | R. Bott, C. Taubes, "On the rigidity theorems of Witten" J. Amer. Math. Soc. , 2 (1989) pp. 137–186 MR0954493 Zbl 0667.57009 |
| [a2] | D.V. Chudnovsky, G.V. Chudnovsky, P.S. Landweber, S. Ochanine, R.E. Stong, "Integrality and divisibility of the elliptic genus" Preprint (1988) |
| [a3] | J. Franke, "On the construction of elliptic cohomology" Math. Nachr. , 158 (1992) pp. 43–65 MR1235295 Zbl 0777.55003 |
| [a4] | F. Hirzebruch, "Topological methods in algebraic geometry" , Grundlehren math. Wiss. , Springer (1966) (Edition: Third) MR0202713 Zbl 0138.42001 |
| [a5] | F. Hirzebruch, Th. Berger, R. Jung, "Manifolds and modular forms" , Aspects of Mathematics , E20 , Vieweg (1992) (Appendices by Nils-Peter Skoruppa and by Paul Baum) MR1189136 Zbl 0752.57013 Zbl 0767.57014 |
| [a6] | J.-I. Igusa, "On the transformation theory of elliptic functions" Amer. J. Math. , 81 (1959) pp. 436–452 MR0104668 Zbl 0131.28102 |
| [a7] | N.M. Katz, "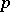 -adic properties of modular schemes and modular forms" W. Kuyk (ed.) J.-P. Serre (ed.) , Modular Functions in One Variable III. Proc. Internat. Summer School, Univ. of Antwerp, RUCA, July 17--August 3, 1972 , Lecture Notes in Mathematics , 350 (1973) pp. 69–190 MR0447119 Zbl 0271.10033 -adic properties of modular schemes and modular forms" W. Kuyk (ed.) J.-P. Serre (ed.) , Modular Functions in One Variable III. Proc. Internat. Summer School, Univ. of Antwerp, RUCA, July 17--August 3, 1972 , Lecture Notes in Mathematics , 350 (1973) pp. 69–190 MR0447119 Zbl 0271.10033 |
| [a8] | P.S. Landweber, "Elliptic genera: An introductory overview" P.S. Landweber (ed.) , Elliptic Curves and Modular Forms in Algebraic Topology (Proc., Princeton 1986) , Lecture Notes in Mathematics , 1326 , Springer (1988) pp. 1–10 MR0970279 Zbl 0649.57021 |
| [a9] | S. Ochanine, "Sur les genres multiplicatifs définis par des intégrales elliptiques" Topology , 26 (1987) pp. 143–151 MR0895567 Zbl 0626.57014 |
| [a10] | P. Roquette, "Analytic theory of elliptic functions over local fields" , Hamburger Math. Einzelschrift. , 1 , Vandenhoeck and Ruprecht (1970) MR0260753 Zbl 0194.52002 |
| [a11] | J.H. Silverman, "The arithmetic of elliptic curves" , GTM , 106 , Springer (1986) MR0817210 Zbl 0585.14026 |
| [a12] | C. Taubes, "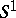 actions and elliptic genera" Comm. Math. Phys. , 122 (1989) pp. 455–526 MR0998662 Zbl 0683.58043 actions and elliptic genera" Comm. Math. Phys. , 122 (1989) pp. 455–526 MR0998662 Zbl 0683.58043 |
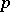 -adic properties of modular schemes and modular forms" W. Kuyk (ed.) J.-P. Serre (ed.) , Modular Functions in One Variable III. Proc. Internat. Summer School, Univ. of Antwerp, RUCA, July 17--August 3, 1972 , Lecture Notes in Mathematics , 350 (1973) pp. 69–190 MR0447119 Zbl 0271.10033
-adic properties of modular schemes and modular forms" W. Kuyk (ed.) J.-P. Serre (ed.) , Modular Functions in One Variable III. Proc. Internat. Summer School, Univ. of Antwerp, RUCA, July 17--August 3, 1972 , Lecture Notes in Mathematics , 350 (1973) pp. 69–190 MR0447119 Zbl 0271.10033 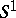 actions and elliptic genera" Comm. Math. Phys. , 122 (1989) pp. 455–526 MR0998662 Zbl 0683.58043
actions and elliptic genera" Comm. Math. Phys. , 122 (1989) pp. 455–526 MR0998662 Zbl 0683.58043