Difference between revisions of "Jones unknotting conjecture"
m (link) |
(Tex done) |
||
| Line 5: | Line 5: | ||
Figure: j130050a | Figure: j130050a | ||
| − | The conjecture has been confirmed for several families of knots, including alternating and adequate knots, knots up to | + | The conjecture has been confirmed for several families of knots, including alternating and adequate knots, knots up to $18$ crossings and $2$-algebraic knots (cf. [[Knot theory]]) up to $21$ crossings [[#References|[a5]]], [[#References|[a7]]]. Recently (2001), S. Yamada announced that the conjecture holds for knots with up to $20$ crossings. The analogous conjecture for links does not hold, as M.B. Thistlethwaite found a $15$-crossing link whose Jones polynomial coincides with a trivial link of two components, cf. Fig.a1. This and similar examples constructed since are $2$-satellites on a Hopf link [[#References|[a6]]], [[#References|[a1]]]. |
| − | L.H. Kauffman showed that there are non-trivial virtual knots with Jones polynomial equal to | + | L.H. Kauffman showed that there are non-trivial virtual knots with Jones polynomial equal to $1$, [[#References|[a4]]]. |
It is still an open problem (as of 2001) whether a simple (non-satellite) link can have a Jones polynomial of an unlink. | It is still an open problem (as of 2001) whether a simple (non-satellite) link can have a Jones polynomial of an unlink. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> S. Eliahou, L.H. Kauffman, M. Thistlethwaite, "Infinite families of links with trivial Jones polynomial" ''preprint'' (2001)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> V.F.R. Jones, "Hecke algebra representations of braid groups and link polynomials" ''Ann. of Math.'' , '''126''' : 2 (1987) pp. 335–388</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> V.F.R. Jones, "Ten problems" , ''Mathematics: Frontiers and Perspectives'' , Amer. Math. Soc. (2000) pp. 79–91</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> L.H. Kauffman, "A survey of virtual knot theory" , ''Knots in Hellas '98'' , ''Ser. on Knots and Everything'' , '''24''' (2000) pp. 143–202</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> W.B.R. Lickorish, M.B. Thistlethwaite, "Some links with non-trivial polynomials and their crossing-numbers" ''Comment. Math. Helv.'' , '''63''' (1988) pp. 527–539</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M.B. Thistlethwaite, "Links with trivial Jones polynomial" ''J. Knot Th. Ramifications'' , '''10''' : 4 (2001) pp. 641–643</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> S. Yamada, "How to find knots with unit Jones polynomials" , ''Knot Theory, Proc. Conf. Dedicated to Professor Kunio Murasugi for his 70th Birthday (Toronto, July 13th-17th 1999)'' (2000) pp. 355–361</TD></TR></table> | + | <table> |
| + | <TR><TD valign="top">[a1]</TD> <TD valign="top"> S. Eliahou, L.H. Kauffman, M. Thistlethwaite, "Infinite families of links with trivial Jones polynomial" ''preprint'' (2001)</TD></TR> | ||
| + | <TR><TD valign="top">[a2]</TD> <TD valign="top"> V.F.R. Jones, "Hecke algebra representations of braid groups and link polynomials" ''Ann. of Math.'' , '''126''' : 2 (1987) pp. 335–388</TD></TR> | ||
| + | <TR><TD valign="top">[a3]</TD> <TD valign="top"> V.F.R. Jones, "Ten problems" , ''Mathematics: Frontiers and Perspectives'' , Amer. Math. Soc. (2000) pp. 79–91</TD></TR> | ||
| + | <TR><TD valign="top">[a4]</TD> <TD valign="top"> L.H. Kauffman, "A survey of virtual knot theory" , ''Knots in Hellas '98'' , ''Ser. on Knots and Everything'' , '''24''' (2000) pp. 143–202</TD></TR> | ||
| + | <TR><TD valign="top">[a5]</TD> <TD valign="top"> W.B.R. Lickorish, M.B. Thistlethwaite, "Some links with non-trivial polynomials and their crossing-numbers" ''Comment. Math. Helv.'' , '''63''' (1988) pp. 527–539</TD></TR> | ||
| + | <TR><TD valign="top">[a6]</TD> <TD valign="top"> M.B. Thistlethwaite, "Links with trivial Jones polynomial" ''J. Knot Th. Ramifications'' , '''10''' : 4 (2001) pp. 641–643</TD></TR> | ||
| + | <TR><TD valign="top">[a7]</TD> <TD valign="top"> S. Yamada, "How to find knots with unit Jones polynomials" , ''Knot Theory, Proc. Conf. Dedicated to Professor Kunio Murasugi for his 70th Birthday (Toronto, July 13th-17th 1999)'' (2000) pp. 355–361</TD></TR> | ||
| + | </table> | ||
| + | |||
| + | {{TEX|done}} | ||
Revision as of 06:25, 3 October 2016
Every non-trivial knot has a non-trivial Jones polynomial.
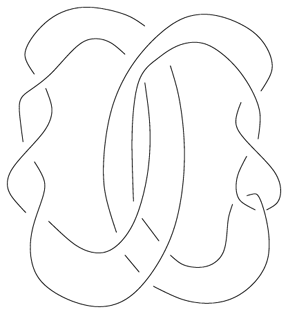
Figure: j130050a
The conjecture has been confirmed for several families of knots, including alternating and adequate knots, knots up to $18$ crossings and $2$-algebraic knots (cf. Knot theory) up to $21$ crossings [a5], [a7]. Recently (2001), S. Yamada announced that the conjecture holds for knots with up to $20$ crossings. The analogous conjecture for links does not hold, as M.B. Thistlethwaite found a $15$-crossing link whose Jones polynomial coincides with a trivial link of two components, cf. Fig.a1. This and similar examples constructed since are $2$-satellites on a Hopf link [a6], [a1].
L.H. Kauffman showed that there are non-trivial virtual knots with Jones polynomial equal to $1$, [a4].
It is still an open problem (as of 2001) whether a simple (non-satellite) link can have a Jones polynomial of an unlink.
References
| [a1] | S. Eliahou, L.H. Kauffman, M. Thistlethwaite, "Infinite families of links with trivial Jones polynomial" preprint (2001) |
| [a2] | V.F.R. Jones, "Hecke algebra representations of braid groups and link polynomials" Ann. of Math. , 126 : 2 (1987) pp. 335–388 |
| [a3] | V.F.R. Jones, "Ten problems" , Mathematics: Frontiers and Perspectives , Amer. Math. Soc. (2000) pp. 79–91 |
| [a4] | L.H. Kauffman, "A survey of virtual knot theory" , Knots in Hellas '98 , Ser. on Knots and Everything , 24 (2000) pp. 143–202 |
| [a5] | W.B.R. Lickorish, M.B. Thistlethwaite, "Some links with non-trivial polynomials and their crossing-numbers" Comment. Math. Helv. , 63 (1988) pp. 527–539 |
| [a6] | M.B. Thistlethwaite, "Links with trivial Jones polynomial" J. Knot Th. Ramifications , 10 : 4 (2001) pp. 641–643 |
| [a7] | S. Yamada, "How to find knots with unit Jones polynomials" , Knot Theory, Proc. Conf. Dedicated to Professor Kunio Murasugi for his 70th Birthday (Toronto, July 13th-17th 1999) (2000) pp. 355–361 |
Jones unknotting conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Jones_unknotting_conjecture&oldid=39362