Difference between revisions of "Modular curve"
Ulf Rehmann (talk | contribs) m (tex done, typos) |
m (fix tex) |
||
| Line 11: | Line 11: | ||
{{TEX|done}} | {{TEX|done}} | ||
| − | A complete [[ | + | A complete [[algebraic curve]] $ X _ {\widetilde \Gamma } $ |
uniformized by a subgroup $ \widetilde \Gamma $ | uniformized by a subgroup $ \widetilde \Gamma $ | ||
of finite index in the [[Modular group|modular group]] $ \Gamma $; | of finite index in the [[Modular group|modular group]] $ \Gamma $; | ||
| Line 20: | Line 20: | ||
The best known examples of subgroups $ \widetilde \Gamma $ | The best known examples of subgroups $ \widetilde \Gamma $ | ||
of finite index in $ \Gamma $ | of finite index in $ \Gamma $ | ||
| − | are the congruence | + | are the [[congruence subgroup]]s containing a principal congruence subgroup $ \Gamma ( N) $ |
of level $ N $ | of level $ N $ | ||
for some integer $ N > 1 $, | for some integer $ N > 1 $, | ||
| Line 26: | Line 26: | ||
$$ | $$ | ||
| − | A \in \ | + | A \in \mathrm{SL} _ {2} ( \mathbf Z ) ,\ \ |
A \equiv \ | A \equiv \ | ||
\left ( | \left ( | ||
| Line 36: | Line 36: | ||
$$ | $$ | ||
| − | (see [[ | + | (see [[Modular group]]). The least such $ N $ |
is called the level of the subgroup $ \widetilde \Gamma $. | is called the level of the subgroup $ \widetilde \Gamma $. | ||
In particular, the subgroup $ \Gamma _ {0} ( N) $ | In particular, the subgroup $ \Gamma _ {0} ( N) $ | ||
| Line 61: | Line 61: | ||
\prod _ {p \mid N } | \prod _ {p \mid N } | ||
| − | ( 1 - p ^ {-} | + | ( 1 - p ^ {-2} ) , |
$$ | $$ | ||
| Line 95: | Line 95: | ||
does not contain $ - 1 $, | does not contain $ - 1 $, | ||
compactified by degenerate curves above the parabolic points of $ X _ {\widetilde \Gamma } $. | compactified by degenerate curves above the parabolic points of $ X _ {\widetilde \Gamma } $. | ||
| − | Powers $ E _ { | + | Powers $ E _ { {\widetilde \Gamma } } ^ {( w) } $, |
where $ w \geq 1 $ | where $ w \geq 1 $ | ||
| − | is an integer, are called Kuga varieties (see [[#References|[3]]], [[#References|[5]]]). The zeta- functions of $ E _ { | + | is an integer, are called Kuga varieties (see [[#References|[3]]], [[#References|[5]]]). The zeta- functions of $ E _ {{\widetilde \Gamma } } ^ {( w) } $ |
are related to the Mellin transforms of modular forms, and their homology to the periods of modular forms (see [[#References|[3]]], [[#References|[7]]]). | are related to the Mellin transforms of modular forms, and their homology to the periods of modular forms (see [[#References|[3]]], [[#References|[7]]]). | ||
| Line 104: | Line 104: | ||
The investigation of the geometry and arithmetic of modular curves is based on the use of groups of automorphisms of the projective limit of the curves $ X _ {\widetilde \Gamma } $ | The investigation of the geometry and arithmetic of modular curves is based on the use of groups of automorphisms of the projective limit of the curves $ X _ {\widetilde \Gamma } $ | ||
with respect to decreasing $ \widetilde \Gamma $, | with respect to decreasing $ \widetilde \Gamma $, | ||
| − | which (in essence) coincides with the group $ \ | + | which (in essence) coincides with the group $ \mathrm{ SL} _ {2} ( A) $ |
over the ring $ A $ | over the ring $ A $ | ||
of rational adèles. On each modular curve $ X _ {\widetilde \Gamma } $ | of rational adèles. On each modular curve $ X _ {\widetilde \Gamma } $ | ||
Revision as of 18:44, 14 June 2020
A complete algebraic curve $ X _ {\widetilde \Gamma } $
uniformized by a subgroup $ \widetilde \Gamma $
of finite index in the modular group $ \Gamma $;
more precisely, a modular curve is a complete algebraic curve obtained from a quotient space $ H / \widetilde \Gamma $,
where $ H $
is the upper half-plane, together with a finite number of parabolic points (the equivalence classes relative to $ \widetilde \Gamma $
of the rational points of the boundary of $ H $).
The best known examples of subgroups $ \widetilde \Gamma $
of finite index in $ \Gamma $
are the congruence subgroups containing a principal congruence subgroup $ \Gamma ( N) $
of level $ N $
for some integer $ N > 1 $,
represented by the matrices
$$ A \in \mathrm{SL} _ {2} ( \mathbf Z ) ,\ \ A \equiv \ \left ( \begin{array}{cc} 1 & 0 \\ 0 & 1 \\ \end{array} \right ) \mathop{\rm mod} N $$
(see Modular group). The least such $ N $ is called the level of the subgroup $ \widetilde \Gamma $. In particular, the subgroup $ \Gamma _ {0} ( N) $ represented by matrices which are congruent $ \mathop{\rm mod} N $ to upper-triangular matrices has level $ N $. Corresponding to each subgroup $ \widetilde \Gamma $ of finite index there is a covering of the modular curve $ X _ {\widetilde \Gamma } \rightarrow X _ \Gamma $, which ramifies only over the images of the points $ z = i $, $ z = ( 1 + i \sqrt 3 ) / 2 $, $ z = \infty $. For a congruence subgroup $ \widetilde \Gamma $ the ramification of this covering allows one to determine the genus of $ X _ {\widetilde \Gamma } $ and to prove the existence of subgroups $ \widetilde \Gamma $ of finite index in $ \Gamma $ which are not congruence subgroups (see [4], Vol. 2, [2]). The genus of $ X _ {\Gamma ( N) } $ is $ 0 $ for $ N \leq 2 $ and equals
$$ 1 + \frac{N ^ {2} ( N - 6 ) }{24} \prod _ {p \mid N } ( 1 - p ^ {-2} ) , $$
$ p $ a prime number, for $ N > 2 $. A modular curve is always defined over an algebraic number field (usually over $ \mathbf Q $ or a cyclic extension of it). The rational functions on a modular curve lift to modular functions (of a higher level) and form a field; the automorphisms of this field have been studied (see [2]). A holomorphic differential form on a modular curve $ X _ {\widetilde \Gamma } $ is given on $ H $ by a differential $ f ( z) d z $( where $ f ( z) $ is a holomorphic function) which is invariant under the transformations $ z \rightarrow \gamma ( z) $ of $ \widetilde \Gamma $; here $ f ( z) $ is a cusp form of weight 2 relative to $ \widetilde \Gamma $. The zeta-function of a modular curve is a product of the Mellin transforms (cf. Mellin transform) of modular forms and, consequently, has a meromorphic continuation and satisfies a functional equation. This fact serves as the point of departure for the Langlands–Weil theory on the relationship between modular forms and Dirichlet series (see [7], [8]). In particular, there is a hypothesis that each elliptic curve over $ \mathbf Q $( with conductor $ N $) can be uniformized by modular functions of level $ N $. The homology of a modular curve is connected with modular symbols, which allows one to investigate the arithmetic of the values of the zeta-function of a modular curve in the centre of the critical strip and to construct the $ p $- adic zeta-function of a modular curve (see [1]).
A modular curve parametrizes a family of elliptic curves, being their moduli variety (see [7], Vol. 2). In particular, for $ \widetilde \Gamma = \Gamma ( N) $ a point $ z $ of $ H / \Gamma ( N) $ is in one-to-one correspondence with a pair consisting of an elliptic curve $ E _ {z} $( analytically equivalent to a complex torus $ \mathbf C / ( \mathbf Z + \mathbf Z z) $) and a point of order $ N $ on $ E _ {z} $( the image of $ z / N $).
Over each modular curve $ X _ {\widetilde \Gamma } $ there is a natural algebraic fibre bundle $ E _ {\widetilde \Gamma } \rightarrow X _ {\widetilde \Gamma } $ of elliptic curves if $ \widetilde \Gamma $ does not contain $ - 1 $, compactified by degenerate curves above the parabolic points of $ X _ {\widetilde \Gamma } $. Powers $ E _ { {\widetilde \Gamma } } ^ {( w) } $, where $ w \geq 1 $ is an integer, are called Kuga varieties (see [3], [5]). The zeta- functions of $ E _ {{\widetilde \Gamma } } ^ {( w) } $ are related to the Mellin transforms of modular forms, and their homology to the periods of modular forms (see [3], [7]).
The rational points on a modular curve correspond to elliptic curves having rational points of finite order (or rational subgroups of points); their description (see [6]) made it possible to solve the problem of determining the possible torsion subgroups of elliptic curves over $ \mathbf Q $.
The investigation of the geometry and arithmetic of modular curves is based on the use of groups of automorphisms of the projective limit of the curves $ X _ {\widetilde \Gamma } $ with respect to decreasing $ \widetilde \Gamma $, which (in essence) coincides with the group $ \mathrm{ SL} _ {2} ( A) $ over the ring $ A $ of rational adèles. On each modular curve $ X _ {\widetilde \Gamma } $ this gives a non-trivial ring of correspondences $ R _ {\widetilde \Gamma } $( a Hecke ring), which has applications in the theory of modular forms (cf. Modular form, [3]).
References
| [1] | Yu.I. Manin, "Parabolic points and zeta-functions of modular curves" Math. USSR Izv. , 6 : 1 (1972) pp. 19–64 Izv. Akad. Nauk SSSR Ser. Mat. , 36 : 1 (1972) pp. 19–66 |
| [2] | G. Shimura, "Introduction to the arithmetic theory of automorphic functions" , Math. Soc. Japan (1971) |
| [3] | V.V. [V.V. Shokurov] Šokurov, "Holomorphic differential forms of higher degree on Kuga's modular varieties" Math. USSR Sb. , 30 : 1 (1976) pp. 119–142 Mat. Sb. , 101 : 1 (1976) pp. 131–157 |
| [4] | F. Klein, R. Fricke, "Vorlesungen über die Theorie der elliptischen Modulfunktionen" , 1–2 , Teubner (1890–1892) |
| [5] | M. Kuga, G. Shimura, "On the zeta function of a fibre variety whose fibres are abelian varieties" Ann. of Math. , 82 (1965) pp. 478–539 |
| [6] | B. Mazur, J.-P. Serre, "Points rationnels des courbes modulaires 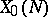 (d'après A. Ogg)" , Sem. Bourbaki 1974/1975 , Lect. notes in math. , 514 , Springer (1976) pp. 238–255 (d'après A. Ogg)" , Sem. Bourbaki 1974/1975 , Lect. notes in math. , 514 , Springer (1976) pp. 238–255 |
| [7] | J.-P. Serre (ed.) P. Deligne (ed.) W. Kuyk (ed.) , Modular functions of one variable. 1–6 , Lect. notes in math. , 320; 349; 350; 476; 601; 627 , Springer (1973–1977) |
| [8] | A. Weil, "Ueber die Bestimmung Dirichletscher Reihen durch Funktionalgleichungen" Math. Ann. , 168 (1967) pp. 149–156 |
Comments
References
| [a1] | N.M. Katz, B. Mazur, "Arithmetic moduli of elliptic curves" , Princeton Univ. Press (1985) |
Modular curve. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Modular_curve&oldid=49313