Difference between revisions of "Morse surgery"
m (fixing spaces) |
m (fixing spaces) |
||
| Line 88: | Line 88: | ||
of a manifold $ V $ | of a manifold $ V $ | ||
of index $ \lambda $ | of index $ \lambda $ | ||
| − | defines a [[Bordism|bordism]] (cobordism) $ ( W ; V , V ^ { \prime } ) $( | + | defines a [[Bordism|bordism]] (cobordism) $ ( W ; V , V ^ { \prime } ) $ (obtained from the product $ V \times [ 0 , 1 ] $ |
| − | obtained from the product $ V \times [ 0 , 1 ] $ | ||
by glueing a handle of index $ \lambda $ | by glueing a handle of index $ \lambda $ | ||
to its "right-hand boundary" $ V \times \{ 1 \} $), | to its "right-hand boundary" $ V \times \{ 1 \} $), | ||
| Line 97: | Line 96: | ||
on which there is such a Morse function is obtained in this way. Hence (and from the existence of Morse functions on triples) it follows that two manifolds are bordant if one can be obtained from the other by a sequence of surgeries. | on which there is such a Morse function is obtained in this way. Hence (and from the existence of Morse functions on triples) it follows that two manifolds are bordant if one can be obtained from the other by a sequence of surgeries. | ||
| − | With the known precautions on the treatment of orientations, the result of a surgery on an oriented manifold is again an oriented manifold. In general, for any structure series $ ( B , \phi ) $( | + | With the known precautions on the treatment of orientations, the result of a surgery on an oriented manifold is again an oriented manifold. In general, for any structure series $ ( B , \phi ) $ (see [[B-Phi-structure| $ ( B , \phi ) $-structure]]) it is possible to define the idea of $ ( B , \phi ) $-surgery; in this connection, two manifolds are $ ( B , \phi ) $-bordant if they are connected by a finite sequence of $ ( B , \phi ) $-surgeries. |
| − | see [[B-Phi-structure| $ ( B , \phi ) $-structure]]) it is possible to define the idea of $ ( B , \phi ) $-surgery; in this connection, two manifolds are $ ( B , \phi ) $-bordant if they are connected by a finite sequence of $ ( B , \phi ) $-surgeries. | ||
The important role of surgery in the topology of manifolds is explained by the fact that it allows one to "delicately" (without infringing on the various properties of manifolds) kill "superfluous" homotopy groups (the operation usually used to this end in homotopy theory, i.e. the operation of "glueing" cells, instantaneously leads out of the class of manifolds). In practice, all theorems on the classification of structures on manifolds are based on the question: Given a mapping $ f : M \rightarrow X $ | The important role of surgery in the topology of manifolds is explained by the fact that it allows one to "delicately" (without infringing on the various properties of manifolds) kill "superfluous" homotopy groups (the operation usually used to this end in homotopy theory, i.e. the operation of "glueing" cells, instantaneously leads out of the class of manifolds). In practice, all theorems on the classification of structures on manifolds are based on the question: Given a mapping $ f : M \rightarrow X $ | ||
Latest revision as of 10:13, 21 March 2022
surgery
A transformation of smooth manifolds to which the level manifold of a smooth function is subjected on passage through a non-degenerate critical point; it is the most important construction in the topology of manifolds.
Let $ V $ be a smooth $ n $-dimensional manifold (without boundary) in which a $ ( \lambda - 1 ) $-dimensional sphere $ S ^ {\lambda - 1 } $ is (smoothly) imbedded. Suppose that the normal bundle of $ S ^ {\lambda - 1 } $ in $ V $ is trivial, that is, a closed tubular neighbourhood $ T $ of $ S ^ {\lambda - 1 } $ in $ V $ decomposes into a direct product $ T = S ^ {\lambda - 1 } \times D ^ {n - \lambda + 1 } $, where $ D ^ {n - \lambda + 1 } $ is a disc of dimension $ n - \lambda + 1 $. After having chosen such a decomposition, remove the interior of $ T $ from $ V $. A manifold is obtained whose boundary decomposes into a product $ S ^ {\lambda - 1 } \times S ^ {n - \lambda } $ of spheres. But the manifold $ D ^ \lambda \times S ^ {n - \lambda } $ has precisely the same boundary. Identifying the boundaries of $ V \setminus \mathop{\rm Int} T $ and $ D ^ \lambda \times S ^ {n - \lambda } $ by a diffeomorphism preserving the product structure of $ S ^ {\lambda - 1 } \times S ^ {n - \lambda } $, a manifold $ V ^ { \prime } $ without boundary is again obtained. It is called the result of surgery of $ V $ along $ S ^ {\lambda - 1 } $.
In the realization of a surgery it is necessary to give a decomposition of the neighbourhood $ T $ of $ S ^ {\lambda - 1 } $ into a direct product, that is, a trivialization of the normal bundle of $ S ^ {\lambda - 1 } $ in $ V $; in this connection different trivializations (riggings) may give essentially distinct (even homotopically) manifolds $ V ^ { \prime } $.
The number $ \lambda $ is called the index of the surgery, and the pair $ ( \lambda , n - \lambda + 1 ) $ its type. If $ V ^ { \prime } $ is obtained from $ V $ by a surgery of type $ ( \lambda , n - \lambda + 1 ) $, then $ V $ is obtained from $ V ^ { \prime } $ by a surgery of type $ ( n - \lambda + 1 , \lambda ) $. For $ \lambda = 0 $, $ V ^ { \prime } $ is a disjoint union of $ V $( which may be empty) and $ S ^ {n} $. The construction of a surgery may also be carried out for piecewise-linear and topological manifolds.
Example. For $ V = S ^ {2} $ and $ \lambda = 2 $ the result of surgery is a disjoint union of spheres, and for $ \lambda = 1 $ a torus. For $ V = S ^ {3} $ and $ \lambda = 2 $ the product $ S ^ {1} \times S ^ {2} $ is obtained. The case $ V = S ^ {3} $ and $ \lambda = 1 $ is more complicated: if $ S ^ {\lambda - 1 } = S ^ {1} $ is imbedded in $ S ^ {3} $ in the standard way (as a great circle), then, depending on the choice of the trivialization of the normal bundle, all lens spaces (cf. Lens space) are obtained; if, however, a knotting of $ S ^ {1} $ is allowed, then a still larger set of three-dimensional manifolds is obtained.
If $ V $ is the boundary of an $ ( n + 1 ) $-dimensional manifold $ M $, then $ V ^ { \prime } $ will be the boundary of the manifold $ M ^ { \prime } $ obtained from $ M $ by glueing a handle of the index of $ M ^ { \prime } $. In particular, if $ f $ is a smooth function on $ M $ and if $ a < b $ are numbers such that $ f ^ { - 1 } [ a , b ] $ is compact and contains a unique non-degenerate critical point $ p $, then $ V ^ {b} = f ^ { - 1 } ( b) $ is obtained from $ V ^ {a} = f ^ { - 1 } ( a) $ by a surgery of index $ \lambda $, where $ \lambda $ is the Morse index of $ p $. In a more general form, any surgery $ V ^ { \prime } $ of a manifold $ V $ of index $ \lambda $ defines a bordism (cobordism) $ ( W ; V , V ^ { \prime } ) $ (obtained from the product $ V \times [ 0 , 1 ] $ by glueing a handle of index $ \lambda $ to its "right-hand boundary" $ V \times \{ 1 \} $), and on the triple $ ( W ; V , V ^ { \prime } ) $ there is a Morse function with a unique critical point of index $ \lambda $; moreover, any bordism $ ( W ; V , V ^ { \prime } ) $ on which there is such a Morse function is obtained in this way. Hence (and from the existence of Morse functions on triples) it follows that two manifolds are bordant if one can be obtained from the other by a sequence of surgeries.
With the known precautions on the treatment of orientations, the result of a surgery on an oriented manifold is again an oriented manifold. In general, for any structure series $ ( B , \phi ) $ (see $ ( B , \phi ) $-structure) it is possible to define the idea of $ ( B , \phi ) $-surgery; in this connection, two manifolds are $ ( B , \phi ) $-bordant if they are connected by a finite sequence of $ ( B , \phi ) $-surgeries.
The important role of surgery in the topology of manifolds is explained by the fact that it allows one to "delicately" (without infringing on the various properties of manifolds) kill "superfluous" homotopy groups (the operation usually used to this end in homotopy theory, i.e. the operation of "glueing" cells, instantaneously leads out of the class of manifolds). In practice, all theorems on the classification of structures on manifolds are based on the question: Given a mapping $ f : M \rightarrow X $ of a closed manifold $ M $ into a CW-complex $ X $, when does there exist a bordism $ ( W ; M , N ) $ and a mapping $ F : W \rightarrow X $ such that $ F \mid _ {M} = f $ and $ F \mid _ {N} : N \rightarrow X $ is a homotopy equivalence. The natural route to the solution of this problem is to annihilate the kernels of the homomorphisms $ f _ {*} : \pi _ {i} ( M) \rightarrow \pi _ {i} ( X) $ by a sequence of surgeries (where $ \pi _ {i} $ are the homotopy groups). If this succeeds, the resulting mapping will be a homotopy equivalence. The study of the corresponding obstructions (lying in the so-called Wall groups, see [4] and Wall group) is one of the most important stimuli in the development of algebraic $ K $-theory.
References
| [1] | M. Morse, "The calculus of variations in the large" , Amer. Math. Soc. (1934) MR1451874 MR1501555 MR1561686 MR1501489 MR1501428 Zbl 0011.02802 Zbl 60.0450.01 |
| [2] | S.P. Novikov, "Homotopically equivalent smooth manifolds I" Transl. Amer. Math. Soc. , 48 (1965) pp. 271–396 Izv. Akad. Nauk SSSR Ser. Mat. , 28 : 2 (1964) pp. 365–474 MR0162246 Zbl 0151.32103 |
| [3] | M.A. Kervaire, J.W. Milnor, "Groups of homotopy spheres: I" Ann. of Math. , 77 (1963) pp. 504–537 MR0148075 Zbl 0115.40505 |
| [4] | A.S. Mishchenko, "Hermitian 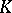 -theory. The theory of characteristic classes and methods of functional analysis" Russian Math. Surveys , 31 : 2 (1976) pp. 71–138 Uspekhi Mat. Nauk , 31 : 2 (1976) pp. 69–134 MR2810191 MR2551881 MR2407859 MR2342865 MR2276911 MR2171671 MR1865951 MR1865950 MR1841760 MR1401584 MR1209904 MR1189162 MR1181028 MR1143142 MR1261408 MR0979315 MR0979309 MR0865932 MR0831650 MR0836571 -theory. The theory of characteristic classes and methods of functional analysis" Russian Math. Surveys , 31 : 2 (1976) pp. 71–138 Uspekhi Mat. Nauk , 31 : 2 (1976) pp. 69–134 MR2810191 MR2551881 MR2407859 MR2342865 MR2276911 MR2171671 MR1865951 MR1865950 MR1841760 MR1401584 MR1209904 MR1189162 MR1181028 MR1143142 MR1261408 MR0979315 MR0979309 MR0865932 MR0831650 MR0836571 |
Comments
There are clear relations between surgery and Morse theory, as indicated above, which is why in the Russian literature the term Morse surgery is frequently used. In the Western literature one simply speaks of surgery. The construction was invented by J.W. Milnor ([a4]).
References
| [a1] | W.B. Browder, "Surgery on simply-connected manifolds" , Springer (1972) MR0358813 Zbl 0239.57016 |
| [a2] | C.T.C. Wall, "Surgery on compact manifolds" , Acad. Press (1970) MR0431216 Zbl 0219.57024 |
| [a3] | C.T.-C. Wall, "Surgery on non-simply connected manifolds" Ann. of Math. , 84 (1966) pp. 217–276 |
| [a4] | J.W. Milnor, "A procedure for killing the homotopy groups of differentiable manifolds" , Differential geometry , Proc. Symp. Pure Math. , 3 , Amer. Math. Soc. (1961) pp. 39–55 MR130696 |
| [a5] | J.W. Milnor, "Morse theory" , Princeton Univ. Press (1963) MR0163331 Zbl 0108.10401 |
| [a6] | M.W. Hirsch, "Differential topology" , Springer (1976) pp. Chapt. 5, Sect. 3 MR0448362 Zbl 0356.57001 |
Morse surgery. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Morse_surgery&oldid=52253