Difference between revisions of "Conformal geometry"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
(latex details) |
||
| (2 intermediate revisions by the same user not shown) | |||
| Line 56: | Line 56: | ||
$$ | $$ | ||
x _ {k} ^ {*} = \ | x _ {k} ^ {*} = \ | ||
| − | \sum _ { l= } | + | \sum _ { l= 1 }^ { 4 } |
p _ {k} ^ {l} x _ {l} ,\ \ | p _ {k} ^ {l} x _ {l} ,\ \ | ||
k = 1 \dots 4 ; \ \ | k = 1 \dots 4 ; \ \ | ||
| Line 172: | Line 172: | ||
$$ | $$ | ||
| − | \mathbf x = \ | + | \mathbf x = \sum_{i=1}^ { 2 } \alpha ^ {i} \mathbf x _ {i} |
| − | \alpha ^ {i} \mathbf x _ {i} | + | $$ |
under the extra condition | under the extra condition | ||
| Line 205: | Line 205: | ||
$$ | $$ | ||
| − | \mathbf x = \ | + | \mathbf x = \sum_{i=1}^ { 2 } |
\alpha ^ {i} \mathbf x _ {i} \ \ | \alpha ^ {i} \mathbf x _ {i} \ \ | ||
\textrm{ and } \ \widetilde{\mathbf x} = \ | \textrm{ and } \ \widetilde{\mathbf x} = \ | ||
| − | \ | + | \sum_{i=1}^ { 2 } |
\beta ^ {i} \widetilde{\mathbf x} _ {i} $$ | \beta ^ {i} \widetilde{\mathbf x} _ {i} $$ | ||
| Line 286: | Line 286: | ||
The use of methods of mathematical analysis in conformal geometry leads to the creation of [[Conformal-differential geometry|conformal-differential geometry]]. The geometry of a space with a [[Conformal connection|conformal connection]] is constructed on the basis of conformal geometry, and this geometry is related to conformal geometry in the same way as Riemannian geometry is related to Euclidean geometry. The following terminology is also customary for conformal geometry: the geometry of inverse radii, circular geometry, inversion geometry, as well as Möbius geometry (named after A. Möbius who first studied the geometry of circular transformations). | The use of methods of mathematical analysis in conformal geometry leads to the creation of [[Conformal-differential geometry|conformal-differential geometry]]. The geometry of a space with a [[Conformal connection|conformal connection]] is constructed on the basis of conformal geometry, and this geometry is related to conformal geometry in the same way as Riemannian geometry is related to Euclidean geometry. The following terminology is also customary for conformal geometry: the geometry of inverse radii, circular geometry, inversion geometry, as well as Möbius geometry (named after A. Möbius who first studied the geometry of circular transformations). | ||
| − | |||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| Line 294: | Line 291: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> H. Schwerdtfeger, "Geometry of complex numbers" , Dover, reprint (1979)</TD></TR></table> | + | <table> |
| + | <TR><TD valign="top">[1]</TD> <TD valign="top"> F. Klein, "Vorlesungen über höhere Geometrie" , Springer (1926)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> W. Blaschke, "Vorlesungen über Differentialgeometrie und geometrische Grundlagen von Einstein's Relativitätstheorie" , '''3. Differentialgeometrie der Kreisen und Kugeln''' , Springer (1929)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> G.V. Bushmanova, A.P. Norden, "Elements of conformal geometry" , Kazan' (1972) (In Russian)</TD></TR><TR><TD valign="top">[a1]</TD> <TD valign="top"> H. Schwerdtfeger, "Geometry of complex numbers" , Dover, reprint (1979)</TD></TR> | ||
| + | </table> | ||
| + | |||
| + | {{OldImage}} | ||
Latest revision as of 12:57, 13 January 2024
The branch of geometry in which properties of figures are studied that are invariant under conformal transformations (cf. Conformal transformation). The main invariant in conformal geometry is the angle between two directions.
Conformal geometry is the geometry defined in Euclidean space extended by a single (ideal) point at infinity having as corresponding fundamental group of transformations the group of point transformations taking spheres into spheres. This space is called the conformal space $ M _ {n} $, and its fundamental group is called the group of conformal transformations. In conformal space, a plane is a sphere passing through the point at infinity.
This definition of conformal geometry is valid for Euclidean spaces of arbitrary dimension; in the two-dimensional case one speaks about circles instead of spheres. For dimension $ n \geq 3 $, the transformations taking spheres into spheres account for all angle-preserving transformations (Liouville's theorem). For $ n = 2 $, the group of transformations preserving angles is larger; however, even in this case the name conformal geometry is kept for geometries having as fundamental group the group of point transformations taking circles into circles.
Each transformation in the fundamental group of a conformal geometry decomposes into a finite number of Euclidean motions, similarity transformations and inversions.
The fundamental group of the conformal geometry of the plane $ M _ {2} $ is isomorphic to a subgroup of the projective group, namely the subgroup of projective transformations of the $ 3 $- dimensional projective space $ P _ {3} $ taking an oval surface of the second order (an elliptic quadric) into itself, that is, the group of hyperbolic motions of the three-dimensional space. This enables one to apply to conformal geometry a similar analytic apparatus as is used in non-Euclidean geometries.
Every point of $ P _ {3} $ is determined by four homogeneous coordinates $ x _ {i} $, $ i = 1 \dots 4 $, or by the pseudo-vector $ \mathbf x $ with these coordinates. Let
$$ ( \mathbf x \mathbf y ) = \ x _ {1} y _ {1} + x _ {2} y _ {2} + x _ {3} y _ {3} - x _ {4} y _ {4} $$
be a form in two vectors $ \mathbf x , \mathbf y $, and let $ K $ be the elliptic quadric in $ P _ {3} $ defined by the equation $ x _ {1} ^ {2} + x _ {2} ^ {2} + x _ {3} ^ {2} - x _ {4} ^ {2} = 0 $, or by $ ( \mathbf x \mathbf x ) = 0 $. One has $ ( \mathbf x \mathbf x ) > 0 $ for the points outside $ K $ and $ ( \mathbf x \mathbf x ) < 0 $ for those inside. By using the absolute $ K $, the stereographic projection can be performed, taking points on and outside the absolute into the conformal plane and its set of circles. The coordinates $ x _ {i} $, $ i = 1 \dots 4 $, of points of $ P _ {3} $ are called the tetracyclic coordinates of the points and the circles on the plane $ M _ {2} $. Since under stereographic projection points on the absolute are taking to points in the plane, while points outside the absolute are taking to circles in the plane, the group of hyperbolic motions in $ P _ {3} $ with absolute $ K $ corresponds to the group of transformations of the plane under which points are taken to points and circles to circles, that is, the fundamental group of the conformal geometry of the plane. This group is given analytically by the formulas
$$ x _ {k} ^ {*} = \ \sum _ { l= 1 }^ { 4 } p _ {k} ^ {l} x _ {l} ,\ \ k = 1 \dots 4 ; \ \ \mathop{\rm det} \| p _ {k} ^ {l} \| \neq 0 , $$
where $ x _ {i} $ and $ x _ {i} ^ {*} $ are coordinates of a point before and after transformation, with the restriction that the expression
$$ ( \mathbf x \mathbf x ) = \ x _ {1} ^ {2} + x _ {2} ^ {2} + x _ {3} ^ {2} - x _ {4} ^ {2} $$
differs from
$$ ( \mathbf x ^ {*} \mathbf x ^ {*} ) = \ ( x _ {1} ^ {*} ) ^ {2} + ( x _ {2} ^ {*} ) ^ {2} + ( x _ {3} ^ {*} ) ^ {2} - ( x _ {4} ^ {*} ) ^ {2} $$
only by a factor. By setting
$$ e ^ {ij} = p _ {1} ^ {i} p _ {1} ^ {j} + p _ {2} ^ {i} p _ {2} ^ {j} + p _ {3} ^ {i} p _ {3} ^ {j} - p _ {4} ^ {i} p _ {4} ^ {j} , $$
the conditions of preservation of the quadratic form can be written as
$$ - e ^ {44} = e ^ {11} = \ e ^ {22} = e ^ {33} = 1 ; \ \ e ^ {ij} = 0 \ \textrm{ if } \ i \neq j . $$
Under conformal transformations, the point at infinity can be taken to any other point, therefore a circle can be taken to a line and vice versa. If it is required that the point at infinity be taken to itself, i.e. that lines be taken to lines, then the group of such transformations is the group of similarity transformations (homothety and Euclidean motion).
The similarity subgroup in $ P _ {3} $ corresponds to the subgroup of hyperbolic motions leaving some given point of the absolute fixed.
Another important class of conformal transformations consists of the inversions (cf. Inversion). An inversion in $ P _ {3} $ corresponds to a polar homology, that is, a hyperbolic motion under which each pair of corresponding points $ p $ and $ p ^ {*} $ lies on a line passing through some fixed point $ C $ outside the absolute and such that for the cross ratio the condition $ ( p : p ^ {*} : C : N ) = - 1 $ is satisfied, where $ N $ is the point of intersection of the above line with the plane that is polar to $ C $ with respect to the absolute. Just as each hyperbolic motion can be obtained as composition of a finite number of polar homologies, any conformal transformation can be obtained as composition of a finite number of inversions.
The main invariant in conformal geometry on the plane is the angle $ \phi $ between two circles. It is expressed by the formula
$$ \cos ^ {2} \phi = \ \frac{( \mathbf x \mathbf y ) ^ {2} }{( \mathbf x \mathbf x ) ( \mathbf y \mathbf y ) } , $$
where $ \mathbf x $ and $ \mathbf y $ are the vectors corresponding to the two circles in tetracyclic coordinates $ x _ {i} $ and $ y _ {i} $, $ i = 1 \dots 4 $. In the hyperbolic geometry of $ P _ {3} $, the angle between two circles in the plane is equal to the non-Euclidean distance between the points in space corresponding to the circles. The invariance of angles follows from that of distances. The condition of orthogonality of two circles is $ ( \mathbf x \mathbf y ) = 0 $, and the condition of tangency is $ ( \mathbf x\mathbf x ) ( \mathbf y\mathbf y ) - ( \mathbf x\mathbf y ) ^ {2} = 0 $. If one of the circles reduces to a point, $ ( \mathbf x \mathbf x ) = 0 $, then one obtains the condition of incidence of the point and the circle, $ ( \mathbf x \mathbf y ) = 0 $.
The simplest figure in $ M _ {2} $ is a pencil of circles. It is defined by an equation $ \mathbf t = \alpha \mathbf p + \beta \mathbf q $, where $ \mathbf p $ and $ \mathbf q $ are fixed circles of the pencil. In dependence on the sign of $ \Delta = ( \mathbf p \mathbf p ) ( \mathbf q \mathbf q ) - ( \mathbf p \mathbf q ) ^ {2} $, the pencils become: a) elliptic $ ( \Delta > 0 ) $; b) hyperbolic $ ( \Delta < 0 ) $; or c) parabolic $ ( \Delta = 0 ) $( see Fig. a).
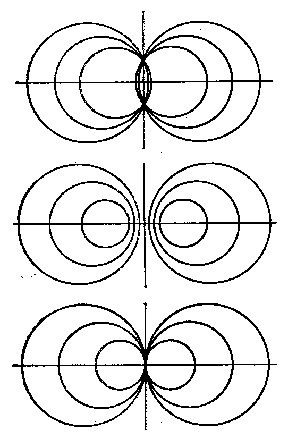
Figure: c024770a
In $ P _ {3} $ straight lines correspond to pencils of circles. An elliptic pencil corresponds to a straight line not intersecting the absolute, a hyperbolic pencil — to a straight line intersecting the absolute, and a parabolic pencil — to a straight line tangent to the absolute. Since each line of $ P _ {3} $ has a conjugate, each pencil in $ M _ {2} $ has a conjugate pencil.
The transformations belonging to the fundamental group of the conformal geometry of the plane are given by the fractional-linear functions of a complex variable.
In the conformal geometry of the three-dimensional space $ M _ {3} $ the main objects are points and spheres. They are defined by pentaspherical coordinates $ x _ {i} $, $ i = 1 \dots 5 $, or by a pseudo-vector $ \mathbf x $ in the five-dimensional space. The angle between spheres is defined by the same formula as the angle between circles in the plane.
The simplest figures in $ M _ {3} $ are: pencils of spheres, $ \mathbf w = \alpha \mathbf y + \beta \mathbf z $, two-parameter bundles, $ \mathbf w = \alpha \mathbf x + \beta \mathbf y + \gamma \mathbf z $, and three-parameter bundles, $ \mathbf w = \alpha \mathbf x + \beta \mathbf y + \gamma \mathbf z + \delta \mathbf t $, of spheres.
A circle in $ M _ {3} $ is defined by an elliptic pencil of spheres, that is, by a formula
$$ \mathbf x = \sum_{i=1}^ { 2 } \alpha ^ {i} \mathbf x _ {i} $$
under the extra condition
$$ ( \mathbf x _ {1} \mathbf x _ {1} ) ( \mathbf x _ {2} \mathbf x _ {2} ) - ( \mathbf x _ {1} \mathbf x _ {2} ) ^ {2} > 0 . $$
The angle $ \theta $ between circles determined by the spheres $ \mathbf x _ {1} $, $ \mathbf x _ {2} $ and the sphere $ \mathbf y $ is defined by the formula
$$ \cos ^ {2} \theta = \ \frac{A ^ {\alpha \beta } ( \mathbf x _ \alpha \mathbf y ) ( \mathbf x _ \beta \mathbf y ) }{( \mathbf y \mathbf y ) } , $$
where $ A ^ {\alpha \beta } $ are the cofactors of the elements of the determinant formed from the $ A _ {\alpha \beta } = ( \mathbf x _ \alpha \mathbf x _ \beta ) $, $ \alpha , \beta = 1 , 2 $. The pair of circles
$$ \mathbf x = \sum_{i=1}^ { 2 } \alpha ^ {i} \mathbf x _ {i} \ \ \textrm{ and } \ \widetilde{\mathbf x} = \ \sum_{i=1}^ { 2 } \beta ^ {i} \widetilde{\mathbf x} _ {i} $$
has the absolute invariants
$$ k = \frac{s ^ {2} }{A \widetilde{A} } \ \textrm{ and } \ \ h = \frac{1}{2} A ^ {ij} \widetilde{A} ^ {kl} S _ {ik} S _ {jl} , $$
where
$$ A _ {ij} = ( \mathbf x _ {i} \mathbf x _ {j} ) ,\ \widetilde{A} _ {ij} = ( \widetilde{\mathbf x} _ {i} \widetilde{\mathbf x} _ {j} ) ,\ \ A = \mathop{\rm det} \| A _ {ij} \| , $$
$$ \widetilde{A} = \mathop{\rm det} \| \widetilde{A} _ {ij} \| ,\ S _ {ij} = ( \mathbf x _ {i} \widetilde{\mathbf x} _ {j} ) . $$
For each pair of circles one can choose from the components of their pencils two principal spheres. The latter are defined by the property that for the pencils in terms of these spheres the conditions $ A _ {11} = A _ {22} = \widetilde{A} _ {11} = \widetilde{A} _ {22} = 1 $, $ A _ {12} = \widetilde{A} _ {12} = 0 $, $ S _ {12} = S _ {21} = 0 $ are satisfied. The pencils themselves are defined in terms of these spheres by
$$ \mathbf x = \mathbf x _ {1} \ \cos \phi + \mathbf x _ {2} \ \sin \phi ,\ \widetilde{\mathbf x} = \widetilde{\mathbf x} _ {1} \cos \ \phi + \widetilde{\mathbf x} _ {2} \sin \phi , $$
where $ \phi $( $ \phi _ {1} $) is the angle between the sphere $ \mathbf x _ {1} $( $ \widetilde{\mathbf x} _ {2} $) and the sphere $ \widetilde{\mathbf x} _ {1} $( $ \widetilde{\mathbf x} _ {2} $). The angles $ \theta _ {1} $ and $ \theta _ {2} $ at which the principal spheres of the first circle intersect those of the second circle are called the principal angles of the circles (they are the same as the angles at which the principal spheres of the second circle intersect those of the first circle). The invariants of a pair of circles are expressed in terms of the principal angles as follows:
$$ k = \cos ^ {2} \ \theta _ {1} \cos ^ {2} \theta _ {2} ,\ \ h = \frac{1}{2} ( \cos ^ {2} \theta _ {1} + \cos ^ {2} \ \theta _ {2} ) . $$
The principal angles $ \theta _ {1} $ and $ \theta _ {2} $ define the extremal values of the angles that the spheres of the first circle form with those of the other. If $ \theta _ {1} = \theta _ {2} $, then for all spheres of the pair $ \theta = \theta _ {1} = \theta _ {2} $, and such a pair of circles is called isogonal. The mutual position of the two circles can be characterized by means of the invariants of the pair: a) linked $ ( 1 - 2 h + k > 0 ) $; b) disjoint $ ( 1 - 2 h + k < 0 ) $; or c) intersecting $ ( 1 - 2 h + k = 0 ) $; and the condition of linear independence of the spheres $ \mathbf x _ {i} $ and $ \widetilde{\mathbf x} _ {i} $( see Fig. b).
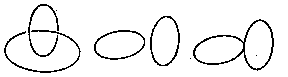
Figure: c024770b
A necessary and sufficient condition for isogonality of a pair of circles is $ h ^ {2} - k = 0 $.
The use of methods of mathematical analysis in conformal geometry leads to the creation of conformal-differential geometry. The geometry of a space with a conformal connection is constructed on the basis of conformal geometry, and this geometry is related to conformal geometry in the same way as Riemannian geometry is related to Euclidean geometry. The following terminology is also customary for conformal geometry: the geometry of inverse radii, circular geometry, inversion geometry, as well as Möbius geometry (named after A. Möbius who first studied the geometry of circular transformations).
Comments
An exhausting treatment of Möbius geometry in dimension 2 is given in [a1].
References
| [1] | F. Klein, "Vorlesungen über höhere Geometrie" , Springer (1926) |
| [2] | W. Blaschke, "Vorlesungen über Differentialgeometrie und geometrische Grundlagen von Einstein's Relativitätstheorie" , 3. Differentialgeometrie der Kreisen und Kugeln , Springer (1929) |
| [3] | G.V. Bushmanova, A.P. Norden, "Elements of conformal geometry" , Kazan' (1972) (In Russian) |
| [a1] | H. Schwerdtfeger, "Geometry of complex numbers" , Dover, reprint (1979) |
Conformal geometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conformal_geometry&oldid=46454