Difference between revisions of "Smoothness, modulus of"
(Importing text file) |
(latex details) |
||
| (One intermediate revision by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | s0859201.png | ||
| + | $#A+1 = 49 n = 1 | ||
| + | $#C+1 = 49 : ~/encyclopedia/old_files/data/S085/S.0805920 Smoothness, modulus of | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | + | The modulus of continuity of the derivative of order $ m \geq 1 $ | |
| + | of a function $ f $ | ||
| + | defined on a Banach space $ X $, | ||
| + | i.e. the expression | ||
| − | + | $$ | |
| + | \omega _ {m} ( f, \delta , X) = | ||
| + | $$ | ||
| − | + | $$ | |
| + | = \ | ||
| + | \sup _ {\begin{array}{c} | ||
| + | h,x \in X \\ | ||
| + | \| h \| _ {X} \leq \delta | ||
| + | \end{array} | ||
| − | + | } \left \| \sum _ {i = 0 } ^ { m } (- 1) ^ {m - i } \left ( \begin{array}{c} | |
| + | m \\ | ||
| + | i | ||
| + | \end{array} | ||
| + | \right ) f \left ( x + ( m - 2i) { | ||
| + | \frac{h}{2} | ||
| + | } \right ) \right \| _ {X} , | ||
| + | $$ | ||
| − | + | where $ ( x \pm mh/2) \in X $. | |
| + | If $ m = 1 $, | ||
| + | the modulus of smoothness is the ordinary modulus of continuity (cf. [[Continuity, modulus of|Continuity, modulus of]]) of $ f $. | ||
| + | Basic properties of the modulus of smoothness (in the case $ X = \mathbf C $ | ||
| + | and the space of continuous functions) are: | ||
| − | + | $$ | |
| + | \omega _ {m} ( f, 0, \mathbf C ) = 0; | ||
| + | $$ | ||
| − | + | $ \omega _ {m} ( f, \delta , \mathbf C ) $ | |
| + | does not decrease together with $ \delta $; | ||
| − | + | if $ k $ | |
| + | $ \geq 1 $ | ||
| + | is an integer, then | ||
| − | + | $$ | |
| + | \omega _ {m} ( f, k \delta , \mathbf C ) \leq \ | ||
| + | k ^ {m} \omega _ {m} ( f, \delta , \mathbf C ); | ||
| + | $$ | ||
| − | + | for any $ \lambda > 0 $, | |
| − | + | $$ | |
| + | \omega _ {m} ( f, \lambda \delta , \mathbf C ) \leq \ | ||
| + | ( \lambda + 1) ^ {m} \omega _ {m} ( f, \delta , \mathbf C ); | ||
| + | $$ | ||
| − | + | if $ \nu > m $, | |
| + | then | ||
| − | + | $$ | |
| + | \omega _ \nu ( f, \delta , \mathbf C ) \leq \ | ||
| + | 2 ^ {\nu - m } \omega _ {m} ( f, \delta , \mathbf C ); | ||
| + | $$ | ||
| − | + | if $ \nu > m $, | |
| + | then | ||
| − | + | $$ | |
| + | \omega _ {m} ( f, \delta , \mathbf C ) | ||
| + | \leq A _ {\nu , m } \delta ^ \nu | ||
| + | \int\limits _ \delta ^ { a } | ||
| + | |||
| + | \frac{\omega _ {m} ( f, u , \mathbf C ) }{u ^ {\nu + 1 } } | ||
| + | \ | ||
| + | du + O ( \delta ^ \nu ), | ||
| + | $$ | ||
| + | |||
| + | where $ A _ {\nu , m } $ | ||
| + | and $ a $ | ||
| + | are constants independent of $ f $. | ||
| + | |||
| + | Certain problems in the theory of approximation of functions can ultimately be solved only in terms of a modulus of smoothness of order $ m \geq 2 $. | ||
| + | In the theory of approximations of functions an important class is the class of periodic continuous functions with period $ 2 \pi $ | ||
| + | and with second-order modulus of smoothness satisfying the condition | ||
| + | |||
| + | $$ | ||
| + | \omega _ {2} ( f, \delta , C _ {2 \pi } ) \leq \delta . | ||
| + | $$ | ||
The modulus of continuity of such functions satisfies the condition | The modulus of continuity of such functions satisfies the condition | ||
| − | + | $$ | |
| + | \omega _ {1} ( f, \delta , C _ {2 \pi } ) \leq \ | ||
| + | \left [ | ||
| + | \frac{1}{ \mathop{\rm ln} ( \sqrt {2 } + 1) } | ||
| + | \right ] | ||
| + | \delta \mathop{\rm ln} { | ||
| + | \frac \pi \delta | ||
| + | } + O ( \delta ), | ||
| + | $$ | ||
| − | < | + | $ 0 < \delta \leq \pi $, |
| + | and the constant $ 1/ \mathop{\rm ln} ( \sqrt {2 } + 1) $ | ||
| + | cannot be improved [[#References|[4]]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> S.N. Bernshtein, "Sur l'ordre de la meilleure approximation des fonctions continués par de polynomes de degré donné" ''Mem. Publ. Classe Sci. Acad. Belgique (2)'' , '''4''' (1912) pp. 1–103</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Marchaud, "Sur les dérivées et sur les différences des fonctions de variables réelles" ''J. Math. Pures Appl.'' , '''6''' (1927) pp. 337–425</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Zygmund, "Smooth functions" ''Duke Math. J.'' , '''12''' (1945) pp. 47–76</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> A.V. Efimov, "Estimate of the modules of continuity of a function in the class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085920/s08592031.png" />" ''Izv. Akad. Nauk SSSR Ser Mat.'' , '''21''' (1957) pp. 283–288 (In Russian)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> S.N. Bernshtein, "Sur l'ordre de la meilleure approximation des fonctions continués par de polynomes de degré donné" ''Mem. Publ. Classe Sci. Acad. Belgique (2)'' , '''4''' (1912) pp. 1–103</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Marchaud, "Sur les dérivées et sur les différences des fonctions de variables réelles" ''J. Math. Pures Appl.'' , '''6''' (1927) pp. 337–425</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Zygmund, "Smooth functions" ''Duke Math. J.'' , '''12''' (1945) pp. 47–76</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> A.V. Efimov, "Estimate of the modules of continuity of a function in the class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085920/s08592031.png" />" ''Izv. Akad. Nauk SSSR Ser Mat.'' , '''21''' (1957) pp. 283–288 (In Russian)</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | The modulus of smoothness | + | The modulus of smoothness $ \omega _ {m} ( f, \delta ) $ |
| + | is also written in terms of symmetric differences, as $ \omega _ {m} ( f , \delta ) = \sup _ {0 < h \leq \delta } \| \Delta _ {h} ^ {m} f \| $, | ||
| + | where | ||
| − | + | $$ | |
| + | \Delta _ {h} ^ {1} f ( x) = \ | ||
| + | f \left ( x + | ||
| + | \frac{h}{2} | ||
| + | \right ) - f \left ( x - | ||
| + | \frac{h}{2} | ||
| + | \right ) | ||
| + | $$ | ||
and | and | ||
| − | + | $$ | |
| + | \Delta _ {h} ^ {m} f ( x) = \ | ||
| + | \Delta _ {n} ( \Delta _ {n} ^ {m-1} f( x)) = \ | ||
| + | \sum_{i=0}^ { m } (- 1) ^ {m-i} \left ( \begin{array}{c} | ||
| + | m \\ | ||
| + | i | ||
| + | \end{array} | ||
| + | \right ) | ||
| + | f \left ( x + ( m- 2i) | ||
| + | \frac{h}{2} | ||
| + | \right ) . | ||
| + | $$ | ||
This gives a recurrent procedure for computing (approximations of) it. | This gives a recurrent procedure for computing (approximations of) it. | ||
| − | To overcome certain shortcomings of this (classical) modulus of smoothness (especially its ability to characterize the order of the best polynomial approximation | + | To overcome certain shortcomings of this (classical) modulus of smoothness (especially its ability to characterize the order of the best polynomial approximation $ E _ {n} ( f ) $ |
| + | to a function $ f \in L _ {p} [- 1 , 1] $), | ||
| + | new moduli of smoothness have been introduced. They use so-called step-weight functions $ \varphi ( x) $, | ||
| + | and are defined by | ||
| − | + | $$ | |
| + | \omega _ \varphi ^ {n} ( f , \delta ) _ {p} = \ | ||
| + | \sup _ {0 < h \leq \delta } \ | ||
| + | \| \Delta _ {h\varphi } ^ {m} f \| _ {L _ {p} } . | ||
| + | $$ | ||
| − | The function | + | The function $ \varphi ( x) $ |
| + | is chosen for the problem at hand. Note that here the increment $ h \varphi ( x) $ | ||
| + | varies with $ x $. | ||
| + | A basic result is that $ E _ {n} ( f ) _ {p} = O( n ^ {- \alpha } ) $ | ||
| + | if and only if $ \omega _ \varphi ^ {m} ( f , \delta ) _ {p} = O( t ^ \alpha ) $. | ||
| + | (Here $ 0< \alpha < m $, | ||
| + | $ 1 \leq p \leq \infty $, | ||
| + | $ \varphi ( x) = ( 1- x ^ {2} ) ^ {1/2} $, | ||
| + | $ f \in L _ {p} [- 1, 1] $, | ||
| + | and approximation is in $ L _ {p} [- 1, 1] $.) | ||
| + | For more on such moduli, their use in $ L _ {p} $ | ||
| + | approximation problems and in the interpolation of spaces, see [[#References|[a1]]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> Z. Ditzian, V. Totik, "Moduli of smoothness" , Springer (1987)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> Z. Ditzian, V. Totik, "Moduli of smoothness" , Springer (1987)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966)</TD></TR></table> | ||
Latest revision as of 16:48, 20 January 2024
The modulus of continuity of the derivative of order $ m \geq 1 $
of a function $ f $
defined on a Banach space $ X $,
i.e. the expression
$$ \omega _ {m} ( f, \delta , X) = $$
$$ = \ \sup _ {\begin{array}{c} h,x \in X \\ \| h \| _ {X} \leq \delta \end{array} } \left \| \sum _ {i = 0 } ^ { m } (- 1) ^ {m - i } \left ( \begin{array}{c} m \\ i \end{array} \right ) f \left ( x + ( m - 2i) { \frac{h}{2} } \right ) \right \| _ {X} , $$
where $ ( x \pm mh/2) \in X $. If $ m = 1 $, the modulus of smoothness is the ordinary modulus of continuity (cf. Continuity, modulus of) of $ f $. Basic properties of the modulus of smoothness (in the case $ X = \mathbf C $ and the space of continuous functions) are:
$$ \omega _ {m} ( f, 0, \mathbf C ) = 0; $$
$ \omega _ {m} ( f, \delta , \mathbf C ) $ does not decrease together with $ \delta $;
if $ k $ $ \geq 1 $ is an integer, then
$$ \omega _ {m} ( f, k \delta , \mathbf C ) \leq \ k ^ {m} \omega _ {m} ( f, \delta , \mathbf C ); $$
for any $ \lambda > 0 $,
$$ \omega _ {m} ( f, \lambda \delta , \mathbf C ) \leq \ ( \lambda + 1) ^ {m} \omega _ {m} ( f, \delta , \mathbf C ); $$
if $ \nu > m $, then
$$ \omega _ \nu ( f, \delta , \mathbf C ) \leq \ 2 ^ {\nu - m } \omega _ {m} ( f, \delta , \mathbf C ); $$
if $ \nu > m $, then
$$ \omega _ {m} ( f, \delta , \mathbf C ) \leq A _ {\nu , m } \delta ^ \nu \int\limits _ \delta ^ { a } \frac{\omega _ {m} ( f, u , \mathbf C ) }{u ^ {\nu + 1 } } \ du + O ( \delta ^ \nu ), $$
where $ A _ {\nu , m } $ and $ a $ are constants independent of $ f $.
Certain problems in the theory of approximation of functions can ultimately be solved only in terms of a modulus of smoothness of order $ m \geq 2 $. In the theory of approximations of functions an important class is the class of periodic continuous functions with period $ 2 \pi $ and with second-order modulus of smoothness satisfying the condition
$$ \omega _ {2} ( f, \delta , C _ {2 \pi } ) \leq \delta . $$
The modulus of continuity of such functions satisfies the condition
$$ \omega _ {1} ( f, \delta , C _ {2 \pi } ) \leq \ \left [ \frac{1}{ \mathop{\rm ln} ( \sqrt {2 } + 1) } \right ] \delta \mathop{\rm ln} { \frac \pi \delta } + O ( \delta ), $$
$ 0 < \delta \leq \pi $, and the constant $ 1/ \mathop{\rm ln} ( \sqrt {2 } + 1) $ cannot be improved [4].
References
| [1] | S.N. Bernshtein, "Sur l'ordre de la meilleure approximation des fonctions continués par de polynomes de degré donné" Mem. Publ. Classe Sci. Acad. Belgique (2) , 4 (1912) pp. 1–103 |
| [2] | A. Marchaud, "Sur les dérivées et sur les différences des fonctions de variables réelles" J. Math. Pures Appl. , 6 (1927) pp. 337–425 |
| [3] | A. Zygmund, "Smooth functions" Duke Math. J. , 12 (1945) pp. 47–76 |
| [4] | A.V. Efimov, "Estimate of the modules of continuity of a function in the class 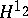 " Izv. Akad. Nauk SSSR Ser Mat. , 21 (1957) pp. 283–288 (In Russian) " Izv. Akad. Nauk SSSR Ser Mat. , 21 (1957) pp. 283–288 (In Russian) |
Comments
The modulus of smoothness $ \omega _ {m} ( f, \delta ) $ is also written in terms of symmetric differences, as $ \omega _ {m} ( f , \delta ) = \sup _ {0 < h \leq \delta } \| \Delta _ {h} ^ {m} f \| $, where
$$ \Delta _ {h} ^ {1} f ( x) = \ f \left ( x + \frac{h}{2} \right ) - f \left ( x - \frac{h}{2} \right ) $$
and
$$ \Delta _ {h} ^ {m} f ( x) = \ \Delta _ {n} ( \Delta _ {n} ^ {m-1} f( x)) = \ \sum_{i=0}^ { m } (- 1) ^ {m-i} \left ( \begin{array}{c} m \\ i \end{array} \right ) f \left ( x + ( m- 2i) \frac{h}{2} \right ) . $$
This gives a recurrent procedure for computing (approximations of) it.
To overcome certain shortcomings of this (classical) modulus of smoothness (especially its ability to characterize the order of the best polynomial approximation $ E _ {n} ( f ) $ to a function $ f \in L _ {p} [- 1 , 1] $), new moduli of smoothness have been introduced. They use so-called step-weight functions $ \varphi ( x) $, and are defined by
$$ \omega _ \varphi ^ {n} ( f , \delta ) _ {p} = \ \sup _ {0 < h \leq \delta } \ \| \Delta _ {h\varphi } ^ {m} f \| _ {L _ {p} } . $$
The function $ \varphi ( x) $ is chosen for the problem at hand. Note that here the increment $ h \varphi ( x) $ varies with $ x $. A basic result is that $ E _ {n} ( f ) _ {p} = O( n ^ {- \alpha } ) $ if and only if $ \omega _ \varphi ^ {m} ( f , \delta ) _ {p} = O( t ^ \alpha ) $. (Here $ 0< \alpha < m $, $ 1 \leq p \leq \infty $, $ \varphi ( x) = ( 1- x ^ {2} ) ^ {1/2} $, $ f \in L _ {p} [- 1, 1] $, and approximation is in $ L _ {p} [- 1, 1] $.) For more on such moduli, their use in $ L _ {p} $ approximation problems and in the interpolation of spaces, see [a1].
References
| [a1] | Z. Ditzian, V. Totik, "Moduli of smoothness" , Springer (1987) |
| [a2] | G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966) |
Smoothness, modulus of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Smoothness,_modulus_of&oldid=12777