Difference between revisions of "Watson transform"
(Importing text file) |
(latex details) |
||
| (One intermediate revision by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | w0971201.png | ||
| + | $#A+1 = 28 n = 1 | ||
| + | $#C+1 = 28 : ~/encyclopedia/old_files/data/W097/W.0907120 Watson transform | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | + | An integral transform $ g $ | |
| + | of a function $ f \in {L _ {2} } ( 0, \infty ) $, | ||
| + | defined as follows: | ||
| − | + | $$ \tag{1 } | |
| + | g( x) = | ||
| + | \frac{d}{dx} | ||
| + | \int\limits _ { 0 } ^ \infty \widetilde \omega ( xu) f( u) | ||
| + | \frac{du}{u} | ||
| + | . | ||
| + | $$ | ||
| − | ( | + | Here $ x $ |
| + | is a real variable, the kernel $ {\widetilde \omega } ( x) $ | ||
| + | has the form | ||
| − | + | $$ \tag{2 } | |
| + | \widetilde \omega ( x) = | ||
| + | \frac{x}{2 \pi } | ||
| − | + | \mathop{\rm l}.i.m. _ {T \rightarrow \infty } \ | |
| + | \int\limits _ { -T}^ { T } | ||
| + | \frac{\Omega \left ( | ||
| + | \frac{1}{2} | ||
| + | + it \right ) }{ | ||
| + | \frac{1}{2} | ||
| + | - it } | ||
| + | x ^ {- ( t+ 1/2) } dt | ||
| + | $$ | ||
| − | + | (l.i.m. denotes the limit in the mean in $ L _ {2} $) | |
| + | and the function $ \Omega ( it + 1 / 2) $ | ||
| + | satisfies the condition | ||
| + | |||
| + | $$ | ||
| + | \Omega ( s) \Omega ( 1- s) = 1. | ||
| + | $$ | ||
| + | |||
| + | The following conditions are sufficient for the existence of the kernel $ {\widetilde \omega } ( x) $ | ||
| + | and the inclusion $ {\widetilde \omega ( x) } / x \in {L _ {2} } ( 0, \infty ) $: | ||
| + | |||
| + | $$ | ||
| + | \Omega \left ( | ||
| + | \frac{1}{2} | ||
| + | - it \right ) = \Omega \left ( | ||
| + | \frac{1}{2} | ||
| + | + it \right ) | ||
| + | $$ | ||
and | and | ||
| − | + | $$ | |
| − | + | \frac{\Omega \left ( | |
| + | \frac{1}{2} | ||
| + | + it \right ) }{ | ||
| + | \frac{1}{2} | ||
| + | - it } | ||
| + | \in \ | ||
| + | L _ {2} (- \infty , \infty ). | ||
| + | $$ | ||
| − | + | For a function $ f \in L _ {2} ( 0, \infty ) $, | |
| + | formula (1) defines the function $ g \in L _ {2} ( 0, \infty ) $ | ||
| + | almost-everywhere. The inversion formula for the Watson transform (1) has the form | ||
| + | |||
| + | $$ | ||
| + | f( x) = | ||
| + | \frac{d}{dx} | ||
| + | \int\limits _ { 0 } ^ \infty \widetilde \omega ( xu ) | ||
| + | g( u) | ||
| + | \frac{du}{u} | ||
| + | . | ||
| + | $$ | ||
Named after G.N. Watson [[#References|[1]]], who was the first to study this transform. | Named after G.N. Watson [[#References|[1]]], who was the first to study this transform. | ||
| Line 28: | Line 91: | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> G.N. Watson, "General transforms" ''Proc. London Math. Soc. (2)'' , '''35''' (1933) pp. 156–199</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> G.N. Watson, "General transforms" ''Proc. London Math. Soc. (2)'' , '''35''' (1933) pp. 156–199</TD></TR></table> | ||
| + | ====Comments==== | ||
| + | Quite generally, let $ \psi $ | ||
| + | be a Lebesgue-measure function in $ \mathbf R _ {>0} $ | ||
| + | and let | ||
| + | $$ | ||
| + | \phi = \int\limits _ { 0 } ^ { x } \psi ( x) dt . | ||
| + | $$ | ||
| − | + | The kernel $ \psi $( | |
| − | + | or $ \phi $) | |
| − | + | is called a generalized kernel, or kernel of a generalized transform, if | |
| − | |||
| − | + | a) $ \psi ( x) $ | |
| + | is real valued on $ \mathbf R _ {>0} $; | ||
| − | + | b) $ x ^ {-1} \phi ( x) \in L _ {2} ( \mathbf R _ {>0 }) $; | |
| − | + | c) $ \int _ {0} ^ \infty \phi ( xu ) \phi ( yu ) u ^ {-2} du = \min ( x, y) $. | |
| − | + | The operator $ \Phi $ | |
| + | defined on $ L _ {2} ( \mathbf R _ {>0} ) $ | ||
| + | by | ||
| − | + | $$ | |
| + | \Phi ( f )( x) = | ||
| + | \frac{d}{dx} | ||
| + | \int\limits _ { 0 } ^ \infty | ||
| − | + | \frac{\phi ( xt) f( t) }{t} | |
| + | dt | ||
| + | $$ | ||
is called a generalized transform or Watson transform. | is called a generalized transform or Watson transform. | ||
Latest revision as of 19:40, 19 January 2024
An integral transform $ g $
of a function $ f \in {L _ {2} } ( 0, \infty ) $,
defined as follows:
$$ \tag{1 } g( x) = \frac{d}{dx} \int\limits _ { 0 } ^ \infty \widetilde \omega ( xu) f( u) \frac{du}{u} . $$
Here $ x $ is a real variable, the kernel $ {\widetilde \omega } ( x) $ has the form
$$ \tag{2 } \widetilde \omega ( x) = \frac{x}{2 \pi } \mathop{\rm l}.i.m. _ {T \rightarrow \infty } \ \int\limits _ { -T}^ { T } \frac{\Omega \left ( \frac{1}{2} + it \right ) }{ \frac{1}{2} - it } x ^ {- ( t+ 1/2) } dt $$
(l.i.m. denotes the limit in the mean in $ L _ {2} $) and the function $ \Omega ( it + 1 / 2) $ satisfies the condition
$$ \Omega ( s) \Omega ( 1- s) = 1. $$
The following conditions are sufficient for the existence of the kernel $ {\widetilde \omega } ( x) $ and the inclusion $ {\widetilde \omega ( x) } / x \in {L _ {2} } ( 0, \infty ) $:
$$ \Omega \left ( \frac{1}{2} - it \right ) = \Omega \left ( \frac{1}{2} + it \right ) $$
and
$$ \frac{\Omega \left ( \frac{1}{2} + it \right ) }{ \frac{1}{2} - it } \in \ L _ {2} (- \infty , \infty ). $$
For a function $ f \in L _ {2} ( 0, \infty ) $, formula (1) defines the function $ g \in L _ {2} ( 0, \infty ) $ almost-everywhere. The inversion formula for the Watson transform (1) has the form
$$ f( x) = \frac{d}{dx} \int\limits _ { 0 } ^ \infty \widetilde \omega ( xu ) g( u) \frac{du}{u} . $$
Named after G.N. Watson [1], who was the first to study this transform.
References
| [1] | G.N. Watson, "General transforms" Proc. London Math. Soc. (2) , 35 (1933) pp. 156–199 |
Comments
Quite generally, let $ \psi $ be a Lebesgue-measure function in $ \mathbf R _ {>0} $ and let
$$ \phi = \int\limits _ { 0 } ^ { x } \psi ( x) dt . $$
The kernel $ \psi $( or $ \phi $) is called a generalized kernel, or kernel of a generalized transform, if
a) $ \psi ( x) $ is real valued on $ \mathbf R _ {>0} $;
b) $ x ^ {-1} \phi ( x) \in L _ {2} ( \mathbf R _ {>0 }) $;
c) $ \int _ {0} ^ \infty \phi ( xu ) \phi ( yu ) u ^ {-2} du = \min ( x, y) $.
The operator $ \Phi $ defined on $ L _ {2} ( \mathbf R _ {>0} ) $ by
$$ \Phi ( f )( x) = \frac{d}{dx} \int\limits _ { 0 } ^ \infty \frac{\phi ( xt) f( t) }{t} dt $$
is called a generalized transform or Watson transform.
References
| [a1] | G.O. Okikiolu, "Aspects of the theory of bounded operators in 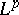 -spaces" , Acad. Press (1971) pp. §6.7 -spaces" , Acad. Press (1971) pp. §6.7 |
Watson transform. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Watson_transform&oldid=12261