Difference between revisions of "Galois geometry"
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
(Category:Algebraic geometry, Category:Combinatorics) |
||
| (2 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | Briefly speaking, Galois geometry is analytical and algebraic geometry over a [[Galois field|Galois field]], that is, geometry over a [[Finite field|finite field]] (cf. also [[Analytic geometry|Analytic geometry]]; [[Algebraic geometry|Algebraic geometry]]). Its beginning may be traced back to a result of B. Segre (1954), saying that every | + | {{TEX|done}} |
| + | Briefly speaking, Galois geometry is analytical and algebraic geometry over a [[Galois field|Galois field]], that is, geometry over a [[Finite field|finite field]] (cf. also [[Analytic geometry|Analytic geometry]]; [[Algebraic geometry|Algebraic geometry]]). Its beginning may be traced back to a result of B. Segre (1954), saying that every $(q+1)$-arc, i.e. set of $q+1$ three-by-three non-collinear points, of a projective Galois plane $PG(2, q), q = p^h, p$ an odd prime number, is an irreducible [[Conic|conic]] [[#References|[a7]]]. | ||
The connections between Galois geometry and other branches of mathematics are numerous: classical algebraic geometry and algebra [[#References|[a18]]], information theory [[#References|[a11]]], physics [[#References|[a14]]], coding theory (cf. also [[Coding and decoding|Coding and decoding]]) [[#References|[a12]]], [[Cryptography|cryptography]] [[#References|[a17]]] and [[Mathematical statistics|mathematical statistics]] [[#References|[a11]]], [[#References|[a13]]]. | The connections between Galois geometry and other branches of mathematics are numerous: classical algebraic geometry and algebra [[#References|[a18]]], information theory [[#References|[a11]]], physics [[#References|[a14]]], coding theory (cf. also [[Coding and decoding|Coding and decoding]]) [[#References|[a12]]], [[Cryptography|cryptography]] [[#References|[a17]]] and [[Mathematical statistics|mathematical statistics]] [[#References|[a11]]], [[#References|[a13]]]. | ||
| − | There are graphic characterizations of remarkable algebraic varieties [[#References|[a15]]] like quadrics [[#References|[a10]]], Veronese varieties, Grassmann varieties, unitals [[#References|[a8]]], etc. (cf. also [[Algebraic variety|Algebraic variety]]). Further, there are links between caps and codes [[#References|[a12]]], [[#References|[a13]]] (cf. also [[Goppa code|Goppa code]]), with the classification of | + | There are graphic characterizations of remarkable algebraic varieties [[#References|[a15]]] like quadrics [[#References|[a10]]], Veronese varieties, Grassmann varieties, unitals [[#References|[a8]]], etc. (cf. also [[Algebraic variety|Algebraic variety]]). Further, there are links between caps and codes [[#References|[a12]]], [[#References|[a13]]] (cf. also [[Goppa code|Goppa code]]), with the classification of $k$-sets from the point of view of characters [[#References|[a9]]], and with the study of ovals and hyperovals, the theory of spreads and blocking sets and the theory of combinatorial designs [[#References|[a16]]] (cf. also [[Block design|Block design]]). |
General references are: [[#References|[a1]]], [[#References|[a2]]], [[#References|[a3]]], [[#References|[a4]]], [[#References|[a5]]], [[#References|[a6]]]. The other references deal with specific topics related with Galois geometry. | General references are: [[#References|[a1]]], [[#References|[a2]]], [[#References|[a3]]], [[#References|[a4]]], [[#References|[a5]]], [[#References|[a6]]]. The other references deal with specific topics related with Galois geometry. | ||
| Line 9: | Line 10: | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> T. Beth, D. Jungnickel, H. Lenz, "Design theory" , BI Wissenschaftsverlag & Cambridge Univ. Press (1984) {{MR|1742365}} {{MR|1729456}} {{MR|0890103}} {{MR|0779284}} {{ZBL|0945.05005}} {{ZBL|0945.05004}} {{ZBL|0602.05001}} {{ZBL|0569.05002}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.W.P. Hirschfeld, "Projective geometries over finite fields" , Oxford Univ. Press (1979) {{MR|0554919}} {{ZBL|0418.51002}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J.W.P. Hirschfeld, "Finite projective spaces in three dimensions" , Oxford Univ. Press (1985) {{MR|840877}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> J.W.P. Hirschfeld, J.A. Thas, "General Galois geometries" , ''Oxford Sci. Publ.'' , Clarendon Press (1991) {{MR|1363259}} {{ZBL|0789.51001}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> B. Segre, "Introduction to Galois geometries" J.W.P. Hirschfeld (ed.) ''Atti Accad. Naz. Lincei, Mem.'' , '''8''' (1967) pp. 133–236 {{MR|0238846}} {{ZBL|0194.21503}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M. Scafati, G. Tallini, "Geometrie di Galois e teoria dei codici" , CISU , Roma (1995)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> B. Segre, "Ovals in a finite projective plane" ''Canad. J. Math.'' , '''7''' (1955) pp. 414–416 {{MR|0071034}} {{ZBL|0065.13402}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> M. Scafati Tallini, "Caratterizzazione grafica delle forme hermitiane di un <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g1100308.png" />" ''Rend. Mat. Roma'' , '''26''' (1967) pp. 273–303 {{MR|}} {{ZBL|0162.24201}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> M. Scafati Tallini, "On <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g1100309.png" />-sets of kind <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003010.png" /> of a finite projective or affine space" ''Ann. Discrete Math.'' , '''14''' (1982) pp. 39–56</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> G. Tallini, "Sulle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003011.png" />-calotte di uno spazio lineare finito" ''Ann. di Mat. (4)'' , '''42''' (1956) pp. 119–164 {{MR|85531}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> G. Tallini, "Le geometrie di Galois e le loro applicazioni alla statistica e alla teoria dell'informazione" ''Rend. Mat. Roma'' , '''19''' (1960) pp. 379–400 {{MR|0125484}} {{ZBL|0211.51403}} </TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> G. Tallini, "On caps of kind <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003012.png" /> in a Galois <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003013.png" />-dimensional space" ''Acta Arithmetica'' , '''VII''' (1961) pp. 19–28 {{MR|150647}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> G. Tallini, "Un'applicazione delle geometrie di Galois a questioni di statistica" ''Rend. Accad. Naz. Lincei'' , '''8''' (1963) pp. 479–485 {{MR|0167432}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> G. Tallini, "Geometrie di Galois e loro applicazioni alla fisica" , ''Lecture Notes'' ''Sem. Lab. Naz. CNEN'' , '''F–70–63''' (1970)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> G. Tallini, "Graphic characterization of algebraic varieties in Galois space" , ''Atti Conv. Int. Teorie Combinatorie'' , '''II''' , Accad. Naz. Lincei (1976) pp. 153–165 {{MR|462986}} {{ZBL|0364.50010}} </TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> G. Tallini, "Lectures on Galois geometries and Steiner systems" , ''Geometries, Codes and Cryptography: CISM courses and lectures'' , '''313''' , Springer (1990) pp. 1–23 {{MR|1140926}} {{ZBL|0714.51002}} </TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> G. Tallini, A. Beutelspacher, "Examples of essentially <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003014.png" />-fold secure geometric authentication systems with large <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003015.png" />" ''Rend. Mat. Roma (VII)'' , '''10''' (1990) pp. 321–326 {{MR|1076161}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> G. Tallini, "General multivalued algebraic structures and geometric spaces" , ''Proc. 4th Int. Congress on Algebraic Hyperstructures and Applications, Xanthi, Greece'' (1990) pp. 197–202 {{MR|1125331}} {{ZBL|}} </TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> T. Beth, D. Jungnickel, H. Lenz, "Design theory" , BI Wissenschaftsverlag & Cambridge Univ. Press (1984) {{MR|1742365}} {{MR|1729456}} {{MR|0890103}} {{MR|0779284}} {{ZBL|0945.05005}} {{ZBL|0945.05004}} {{ZBL|0602.05001}} {{ZBL|0569.05002}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.W.P. Hirschfeld, "Projective geometries over finite fields" , Oxford Univ. Press (1979) {{MR|0554919}} {{ZBL|0418.51002}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J.W.P. Hirschfeld, "Finite projective spaces in three dimensions" , Oxford Univ. Press (1985) {{MR|840877}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> J.W.P. Hirschfeld, J.A. Thas, "General Galois geometries" , ''Oxford Sci. Publ.'' , Clarendon Press (1991) {{MR|1363259}} {{ZBL|0789.51001}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> B. Segre, "Introduction to Galois geometries" J.W.P. Hirschfeld (ed.) ''Atti Accad. Naz. Lincei, Mem.'' , '''8''' (1967) pp. 133–236 {{MR|0238846}} {{ZBL|0194.21503}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M. Scafati, G. Tallini, "Geometrie di Galois e teoria dei codici" , CISU , Roma (1995)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> B. Segre, "Ovals in a finite projective plane" ''Canad. J. Math.'' , '''7''' (1955) pp. 414–416 {{MR|0071034}} {{ZBL|0065.13402}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> M. Scafati Tallini, "Caratterizzazione grafica delle forme hermitiane di un <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g1100308.png" />" ''Rend. Mat. Roma'' , '''26''' (1967) pp. 273–303 {{MR|}} {{ZBL|0162.24201}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> M. Scafati Tallini, "On <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g1100309.png" />-sets of kind <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003010.png" /> of a finite projective or affine space" ''Ann. Discrete Math.'' , '''14''' (1982) pp. 39–56</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> G. Tallini, "Sulle <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003011.png" />-calotte di uno spazio lineare finito" ''Ann. di Mat. (4)'' , '''42''' (1956) pp. 119–164 {{MR|85531}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> G. Tallini, "Le geometrie di Galois e le loro applicazioni alla statistica e alla teoria dell'informazione" ''Rend. Mat. Roma'' , '''19''' (1960) pp. 379–400 {{MR|0125484}} {{ZBL|0211.51403}} </TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> G. Tallini, "On caps of kind <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003012.png" /> in a Galois <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003013.png" />-dimensional space" ''Acta Arithmetica'' , '''VII''' (1961) pp. 19–28 {{MR|150647}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> G. Tallini, "Un'applicazione delle geometrie di Galois a questioni di statistica" ''Rend. Accad. Naz. Lincei'' , '''8''' (1963) pp. 479–485 {{MR|0167432}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> G. Tallini, "Geometrie di Galois e loro applicazioni alla fisica" , ''Lecture Notes'' ''Sem. Lab. Naz. CNEN'' , '''F–70–63''' (1970)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> G. Tallini, "Graphic characterization of algebraic varieties in Galois space" , ''Atti Conv. Int. Teorie Combinatorie'' , '''II''' , Accad. Naz. Lincei (1976) pp. 153–165 {{MR|462986}} {{ZBL|0364.50010}} </TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> G. Tallini, "Lectures on Galois geometries and Steiner systems" , ''Geometries, Codes and Cryptography: CISM courses and lectures'' , '''313''' , Springer (1990) pp. 1–23 {{MR|1140926}} {{ZBL|0714.51002}} </TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> G. Tallini, A. Beutelspacher, "Examples of essentially <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003014.png" />-fold secure geometric authentication systems with large <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/g/g110/g110030/g11003015.png" />" ''Rend. Mat. Roma (VII)'' , '''10''' (1990) pp. 321–326 {{MR|1076161}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> G. Tallini, "General multivalued algebraic structures and geometric spaces" , ''Proc. 4th Int. Congress on Algebraic Hyperstructures and Applications, Xanthi, Greece'' (1990) pp. 197–202 {{MR|1125331}} {{ZBL|}} </TD></TR></table> | ||
| + | |||
| + | [[Category:Algebraic geometry]] | ||
| + | [[Category:Combinatorics]] | ||
Latest revision as of 19:43, 9 November 2014
Briefly speaking, Galois geometry is analytical and algebraic geometry over a Galois field, that is, geometry over a finite field (cf. also Analytic geometry; Algebraic geometry). Its beginning may be traced back to a result of B. Segre (1954), saying that every $(q+1)$-arc, i.e. set of $q+1$ three-by-three non-collinear points, of a projective Galois plane $PG(2, q), q = p^h, p$ an odd prime number, is an irreducible conic [a7].
The connections between Galois geometry and other branches of mathematics are numerous: classical algebraic geometry and algebra [a18], information theory [a11], physics [a14], coding theory (cf. also Coding and decoding) [a12], cryptography [a17] and mathematical statistics [a11], [a13].
There are graphic characterizations of remarkable algebraic varieties [a15] like quadrics [a10], Veronese varieties, Grassmann varieties, unitals [a8], etc. (cf. also Algebraic variety). Further, there are links between caps and codes [a12], [a13] (cf. also Goppa code), with the classification of $k$-sets from the point of view of characters [a9], and with the study of ovals and hyperovals, the theory of spreads and blocking sets and the theory of combinatorial designs [a16] (cf. also Block design).
General references are: [a1], [a2], [a3], [a4], [a5], [a6]. The other references deal with specific topics related with Galois geometry.
References
| [a1] | T. Beth, D. Jungnickel, H. Lenz, "Design theory" , BI Wissenschaftsverlag & Cambridge Univ. Press (1984) MR1742365 MR1729456 MR0890103 MR0779284 Zbl 0945.05005 Zbl 0945.05004 Zbl 0602.05001 Zbl 0569.05002 |
| [a2] | J.W.P. Hirschfeld, "Projective geometries over finite fields" , Oxford Univ. Press (1979) MR0554919 Zbl 0418.51002 |
| [a3] | J.W.P. Hirschfeld, "Finite projective spaces in three dimensions" , Oxford Univ. Press (1985) MR840877 |
| [a4] | J.W.P. Hirschfeld, J.A. Thas, "General Galois geometries" , Oxford Sci. Publ. , Clarendon Press (1991) MR1363259 Zbl 0789.51001 |
| [a5] | B. Segre, "Introduction to Galois geometries" J.W.P. Hirschfeld (ed.) Atti Accad. Naz. Lincei, Mem. , 8 (1967) pp. 133–236 MR0238846 Zbl 0194.21503 |
| [a6] | M. Scafati, G. Tallini, "Geometrie di Galois e teoria dei codici" , CISU , Roma (1995) |
| [a7] | B. Segre, "Ovals in a finite projective plane" Canad. J. Math. , 7 (1955) pp. 414–416 MR0071034 Zbl 0065.13402 |
| [a8] | M. Scafati Tallini, "Caratterizzazione grafica delle forme hermitiane di un  " Rend. Mat. Roma , 26 (1967) pp. 273–303 Zbl 0162.24201 " Rend. Mat. Roma , 26 (1967) pp. 273–303 Zbl 0162.24201 |
| [a9] | M. Scafati Tallini, "On 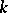 -sets of kind -sets of kind 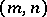 of a finite projective or affine space" Ann. Discrete Math. , 14 (1982) pp. 39–56 of a finite projective or affine space" Ann. Discrete Math. , 14 (1982) pp. 39–56 |
| [a10] | G. Tallini, "Sulle 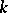 -calotte di uno spazio lineare finito" Ann. di Mat. (4) , 42 (1956) pp. 119–164 MR85531 -calotte di uno spazio lineare finito" Ann. di Mat. (4) , 42 (1956) pp. 119–164 MR85531 |
| [a11] | G. Tallini, "Le geometrie di Galois e le loro applicazioni alla statistica e alla teoria dell'informazione" Rend. Mat. Roma , 19 (1960) pp. 379–400 MR0125484 Zbl 0211.51403 |
| [a12] | G. Tallini, "On caps of kind  in a Galois in a Galois  -dimensional space" Acta Arithmetica , VII (1961) pp. 19–28 MR150647 -dimensional space" Acta Arithmetica , VII (1961) pp. 19–28 MR150647 |
| [a13] | G. Tallini, "Un'applicazione delle geometrie di Galois a questioni di statistica" Rend. Accad. Naz. Lincei , 8 (1963) pp. 479–485 MR0167432 |
| [a14] | G. Tallini, "Geometrie di Galois e loro applicazioni alla fisica" , Lecture Notes Sem. Lab. Naz. CNEN , F–70–63 (1970) |
| [a15] | G. Tallini, "Graphic characterization of algebraic varieties in Galois space" , Atti Conv. Int. Teorie Combinatorie , II , Accad. Naz. Lincei (1976) pp. 153–165 MR462986 Zbl 0364.50010 |
| [a16] | G. Tallini, "Lectures on Galois geometries and Steiner systems" , Geometries, Codes and Cryptography: CISM courses and lectures , 313 , Springer (1990) pp. 1–23 MR1140926 Zbl 0714.51002 |
| [a17] | G. Tallini, A. Beutelspacher, "Examples of essentially  -fold secure geometric authentication systems with large -fold secure geometric authentication systems with large  " Rend. Mat. Roma (VII) , 10 (1990) pp. 321–326 MR1076161 " Rend. Mat. Roma (VII) , 10 (1990) pp. 321–326 MR1076161 |
| [a18] | G. Tallini, "General multivalued algebraic structures and geometric spaces" , Proc. 4th Int. Congress on Algebraic Hyperstructures and Applications, Xanthi, Greece (1990) pp. 197–202 MR1125331 |
Galois geometry. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Galois_geometry&oldid=23835