User:Maximilian Janisch/latexlist/Algebraic Groups/Homogeneous space

This page is a copy of the article Homogeneous space in order to test automatic LaTeXification. This article is not my work.

A set together with a given transitive group action. More precisely, $N$ is a homogeneous space with group $k$ if a mapping

\begin{equation} ( g _ { 0 } x ) \rightarrow g x \end{equation}

of the set $G \times M$ into $N$ is given, such that

1) $( g h ) x = g ( h x )$;

2) $e x = x$;

3) for any $x , y \in M$ there exists a $g \in G$ such that $g x = y$.

The elements of the set $N$ are called the points of the homogeneous space, and the group $k$ is called the group of motions, or the basic (fundamental) group of the homogeneous space.

Any point $\pi$ in $N$ determines a subgroup

\begin{equation} G _ { X } = \{ g \in G : g x = x \} \end{equation}

of $k$. It is called the isotropy group, or stationary subgroup, or stabilizer of the point $\pi$. The stabilizers of different points are conjugate in $k$ by inner automorphisms.

With an arbitrary subgroup of $k$ is associated a certain homogeneous space for $k$, namely, the set $M = G / H$ of left cosets of $H$ in $k$, on which $k$ acts by the formula

\begin{equation} g ( \alpha H ) = ( g a ) H , \quad g , \alpha \in G \end{equation}

This homogeneous space is called the quotient space of $k$ by $H$, and the subgroup $H$ turns out to be the stabilizer of the point $e H = H$ of this space ($E$ is the identity of $k$). Any homogeneous space $N$ with group $k$ can be identified with the quotient space of $k$ by the subgroup $H = G _ { X }$, the stabilizer of a fixed point $x \in M$, by means of the bijection

\begin{equation} M \supset y \Leftrightarrow g H \in G / H \end{equation}

where 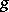is any element of $k$ such that $g x = y$.

If $k$ is a topological group and $H$ is a subgroup of it (respectively, $k$ is a Lie group and $H$ is a closed subgroup of $k$), then $M = G / H$ is endowed with the structure of a topological space (respectively, of a differentiable manifold) in a canonical way, relative to which the action of $k$ on $N$ is continuous (respectively, differentiable). If a Lie group $k$ acts transitively and differentiably on a differentiable manifold $N$, then, for any point $x _ { 0 } \in M$, the subgroup $H = G _ { X _ { 0 } }$ is closed and the bijection $g H \rightarrow g x 0$ above is differentiable; if the number of connected components of $k$ is at most countable, then this bijection is a diffeomorphism.

Other cases which have been studied are when $k$ is an algebraic group and $N$ an algebraic variety (see Homogeneous space of an algebraic group), and when $N$ is a complex manifold and $k$ is a real (or complex) Lie group (see Homogeneous complex manifold).

In what follows $N$ is always a differentiable manifold and $k$ is a Lie group.

Geometry of homogeneous spaces.

According to F. Klein's Erlangen program, the subject of the geometry of a homogeneous space is the study of invariants of the group of motions of a homogeneous space. The classical area of research here is the classification of the various subsets of a homogeneous space, in particular submanifolds and their unions, families of submanifolds, etc., up to motions of the group $k$. Such a classification can be obtained by constructing a complete system of invariants of subsets of given type (examples of such systems of invariants are the length of the sides of a triangle, or the curvature and torsion of a smooth curve in the three-dimensional Euclidean space). A general method for constructing a complete system of local invariants (the moving-frame method) for a smooth submanifold in an arbitrary homogeneous space of a Lie group was developed by E. Cartan (see [6], [16]).

Another direction of research is the discovery and study of invariant geometric objects on a homogeneous space (see Invariant object on a homogeneous space). The action of the basic Lie group $k$ on a homogeneous space $N$ induces an action of $k$ on the space of various geometric objects on $N$ (functions, vector and tensor fields, connections, differential operators, etc.). Geometric objects that are fixed under this action are called invariant objects. Examples of such objects are the Euclidean metric on a Euclidean space regarded as a homogeneous space of the group of Euclidean motions, and the conformal metric giving the angle between curves in a conformal space. Closely related to this area is the problem of describing and studying homogeneous spaces having a particular invariant. For example, one can consider Riemannian and pseudo-Riemannian spaces, spaces with an affine connection, symplectic homogeneous spaces, homogeneous complex manifolds, that is, homogeneous spaces having an invariant metric (Riemannian or pseudo-Riemannian), an affine connection, a symplectic structure, or a complex structure, respectively. See also Riemannian space, homogeneous; Symplectic homogeneous space; Homogeneous complex manifold.

An important class of homogeneous spaces is the class of reductive homogeneous spaces, that is, homogeneous spaces $G / H$ such that the Lie algebra $8$ of the Lie group $k$ has the decomposition

\begin{equation} \mathfrak { g } = \mathfrak { f } + \mathfrak { m } , \quad \mathfrak { f } \cap \mathfrak { m } = \{ 0 \} \end{equation}

where $f$ is the Lie algebra of $H$ and $11$ is a subspace invariant under the adjoint representation of $H$ in $8$ (cf. Adjoint representation of a Lie group). Such a decomposition defines a geodesically-complete linear connection on $G / H$ with parallel curvature and torsion tensors. Conversely, a simply-connected manifold with a complete linear connection having parallel curvature and torsion tensors is a reductive homogeneous space with respect to the automorphism group of this connection (see [5]). A special case of a reductive homogeneous space is a symmetric space, for which the decomposition (*) satisfies the additional condition $[ \mathfrak { m } , \mathfrak { m } ] \subseteq \mathfrak { f }$. Geometrically this condition means that the corresponding connection has zero torsion. Examples of symmetric spaces are the globally symmetric Riemannian spaces (cf. Globally symmetric Riemannian space), as well as the space of an arbitrary Lie group, on which the group of motions is generated by left or right translations.

Homogeneous bundles and representation theory.

The action of $k$ can be extended not only to bundles of geometric objects, but also to the larger class of so-called homogeneous bundles. A homogeneous bundle $31$ over the homogeneous space $G / H$ is given by the left action of the subgroup $H$ on an arbitrary manifold $H ^ { \prime }$ (a typical fibre) and is defined as the natural projection

\begin{equation} \pi : G \times _ { H } F \rightarrow G / H \end{equation}

where $G \times _ { H } F$ is the fibre product as the quotient of the direct product $G \times F$ by the equivalence relation

\begin{equation} ( g , f ) \sim ( g h ^ { - 1 } , h f ) , \quad g \in G , \quad k \in H , \quad f \in F \end{equation}

If $P$ is a vector space on which $H$ acts linearly, then the corresponding homogeneous bundle $31$ is a vector bundle, and in the space of its sections $\Gamma ( \pi )$ there is a linear representation of $k$, induced by the representation of the subgroup $H$ in $H ^ { \prime }$. The study of induced representations (cf. Induced representation) (the properties of which turn out to be closely related to the geometry of the corresponding homogeneous space) and their generalizations plays an important role in the representation theory of Lie groups (see [7]).

Analysis on homogeneous spaces.

Among the most developed areas are: 1) the study of various function spaces on a homogeneous space (spaces of functions, spaces of sections of homogeneous vector bundles, cohomology spaces with values in appropriate sheaves); 2) the study of invariant differential operators acting on these spaces; and 3) the study of various dynamical systems related to homogeneous spaces.

The first area includes the theory of spherical functions (and, more generally, spherical sections), which studies finite-dimensional spaces of functions on a homogeneous space which are invariant with respect to the basic group (see Representation function), many special functions of mathematical physics can be interpreted as spherical functions on some homogeneous space, and the study of representations of the basic group in such function spaces enables one to obtain in a unified way the basic results of the theory of special functions (integral representations, recurrence formulas, addition theorems, etc., see [2]). A natural generalization of the theory of Fourier series and integrals is abstract harmonic analysis (cf. Harmonic analysis, abstract) on homogeneous spaces, one of the basic problems in which consists of the description of the decomposition of the space of square-integrable functions on a homogeneous space as the sum of subspaces irreducible under the action of the basic group. The majority of results obtained here are connected with the case when the homogeneous space is the space of a semi-simple Lie group (see [4]).

The theory of automorphic functions leads to the more general problem of the decomposition into irreducible components of the space of square-integrable sections of a homogeneous vector bundle over a homogeneous space $G / H$ which are invariant relative to a discrete subgroup $\Gamma \subset G$.

As well as function spaces, various measure spaces on homogeneous spaces are also studied, for example in connection with applications to probability theory (see [3], [9]).

The second area includes problems of the description of invariant differential operators (cf. Invariant differential operator) on homogeneous spaces, the study of their properties, finding their spectrum and fundamental solution, and the investigation of the solutions of the corresponding partial differential equations (see [8], [15]).

The third area includes the study of various dynamical systems (cf. Dynamical system) related to the homogeneous space, for example, the flow generated by a one-parameter subgroup of the basic group, the flow generated by the canonical connection of a Lie group, the geodesic flow of a homogeneous Riemannian space, etc. Conditions for the ergodicity of flows have been investigated, and a description of their first integrals have been given (see [1]).

Integral geometry is also related to analysis on homogeneous spaces, being connected with the theory of invariant measures on homogeneous spaces and on manifolds related to these, with as points submanifolds of one sort or another.

The topology of homogeneous spaces.

The methods of algebraic topology in many cases allow one to reduce the problem of computing basic topological invariants of a homogeneous space (the cohomology ring, characteristic classes, $K$-functor, homotopy groups, etc.) to certain algebraic problems concerning the algebraic structure of the basic group and the isotropy group of the homogeneous space. Explicit results of this kind have been obtained for several classes of homogeneous spaces. For example, a theorem of H. Cartan gives an algorithm for computing the real cohomology algebra $H ^ { * } ( G / H ; R )$, where $k$ and $H$ are connected compact Lie groups, in terms of invariants of the Weyl groups (cf. Weyl group) of $k$ and $H$ (see [10]). In particular, if $G / H$ has non-zero Euler characteristic (this is equivalent to $k$ and $H$ having the same rank), then the Poincaré polynomial (cf. Künneth formula) of the manifold $G / H$ has the form

\begin{equation} P ( G / H , t ) = \prod _ { i = 1 } ^ { r } \frac { 1 - t ^ { 2 k } i } { 1 - t ^ { 2 l _ { i } } } \end{equation}

where $k _ { 1 } , \ldots , k$ and $l _ { 1 } , \dots , l$ are the degrees of the basis invariant polynomials of the Weyl groups for $k$ and $H$, respectively (Hirsch's formula).

A very detailed study has been made of the topological structure of homogeneous spaces of compact Lie groups, symmetric spaces and solv manifolds (homogeneous spaces of solvable Lie group, cf. Solv manifold). The Mostow–Karpelevich theorem, which states that any homogeneous space of a Lie group having a finite basic group is diffeomorphic to a vector bundle over the homogeneous space of a compact Lie group, reduces the study of the topology of homogeneous spaces to a considerable extent to the case when the basic group is compact.

The classification of homogeneous spaces.

The basic problems in this area consist in the determination of those manifolds which are homogeneous spaces of connected Lie groups and in the enumeration of all transitive actions of connected Lie groups on these manifolds. For example, the only homogeneous spaces of dimension 2 are the plane, the cylinder, the sphere, the torus, the Möbius strip, the projective plane, and the Klein bottle. At present (1982), the classification of three-dimensional homogeneous spaces has also been carried out, as well as the classification (up to finite-sheeted coverings) of all compact homogeneous spaces of dimensions $< 6$ (see [11]).

For a number of important classes of homogeneous spaces $N$ of high dimension, a classification of all transitive actions of Lie groups on $N$ is known (see ). For example, the classification of all transitive actions of compact Lie groups on spheres has the following form. Any continuous, transitive and effective action of a connected compact Lie group on $S ^ { n }$ can be transformed by a homeomorphism of the sphere $S ^ { n }$ to the standard linear action of the group $SO ( n + 1 )$ or one of the following subgroups of it:

$G = SU ( k )$ or $U ( k )$ if $n = 2 k - 1$;

$G = \operatorname { Sp } ( k )$, $\operatorname { Sp } ( k ) \times U ( 1 )$ or $\operatorname { Sp } ( k ) \times \operatorname { Sp } ( 1 )$ if $n = 4 k - 1$;

$G = \operatorname { Spin } ( 7 )$ or $\operatorname { pin } ( 9 )$ if $n = 7,15$;

$G = G$ if $n = 6$ (the Montgomery–Samelson–Borel theorem, see [10]). As for transitive actions of non-compact Lie groups on the sphere $S ^ { n }$, for even $12$ the only such actions are essentially the projective action of $SL ( n + 1 )$ and the conformal action of $SO ( 1 , n + 1 )$. For odd $12$, the result is more complicated: Transitive and effective actions can exist of a Lie group with a radical of arbitrarily large dimension.

References

[1]	L. Auslander, L. Green, F. Hahn, "Flows on homogeneous spaces" , Princeton Univ. Press (1963) MR0167569 Zbl 0106.36802
[2]	N.Ya. Vilenkin, "Special functions and the theory of group representations" , Amer. Math. Soc. (1968) (Translated from Russian) MR0229863 Zbl 0172.18404
[3]	U. Grenander, "Probabilities on algebraic structures" , Wiley (1963) MR0206994 Zbl 0131.34804
[4]	D.P. Zhelobenko, "Harmonic analysis of functions on semi-simple complex Lie groups" , Moscow (1974) (In Russian)
[5]	E. Cartan, "Groupes de Lie" , Oeuvres complétes. Partie I , 1–2 , Gauthier-Villars (1952) MR0050516 Zbl 0049.30303 Zbl 0049.30302
[6]	E. Cartan, "La théorie des groupes finis et continus et la géométrie différentielle traitées par la méthode du repère mobile" , Gauthier-Villars (1951) MR1190006 Zbl 0054.01401 Zbl 0043.36601
[7]	A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) (Translated from Russian) MR0412321 Zbl 0342.22001
[8]	S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) MR0514561 Zbl 0451.53038
[9]	E.J. Hannan, "Group representations and applied probability" , Methuen (1965) MR0211427 MR0211426 Zbl 0134.33802
[10]	A. Borel, "Sur la cohomologie des espaces fibrés principaux et des espaces homogènes de groupes de Lie compacts" Ann. of Math. , 57 (1953) pp. 115–207 MR0051508 Zbl 0052.40001
[11]	V.V. Gorbatsevich, "Compact homogeneous manifolds of small dimension" , Geometric methods in problems of algebra and analysis , Yaroslavl' (1980) pp. 37–60 (In Russian) MR0617386
[12a]	L. Onishchik, "Transitive compact transformation groups" Transl. Amer. Math. Soc. (2) , 55 (1966) pp. 153–194 Mat. Sb. , 60 : 4 (1963) pp. 447–485 Zbl 0207.33604
[12b]	A.L. Onishchik, "On Lie groups, transitive on compact manifolds, III" Math. USSR Sb. , 4 (1968) pp. 233–240 Mat. Sb. , 75 (1968) pp. 255–263 Zbl 0198.29001
[13]	Itogi Nauk. Algebra Topol. 1963 (1964)
[14]	D.V. Alekseevskii, "Lie groups and homogeneous spaces" J. Soviet Math. , 11 (1974) pp. 483–539 Itogi Nauk. Algebra Topol. Geom. , 11 (1974) pp. 38–124 MR0427536
[15]	S. Helgason, "Groups and geometric analysis" , Acad. Press (1984) MR0754767 Zbl 0543.58001
[16]	G. Jensen, "Higher order contact of submanifolds of homogeneous spaces" , Lect. notes in math. , 610 , Springer (1977) MR0500648 Zbl 0356.53005
[17]	S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 2 , Interscience (1969) MR0238225 Zbl 0175.48504
[18]	J.A. Wolf, "Spaces of constant curvature" , Publish or Perish (1974) MR0343214 Zbl 0281.53034
[19]	E.B. Vinberg, A.L. Onishchik, "Fundamentals of the theory of Lie groups" , Fundamental Directions , 20. Lie groups and Lie algebras 1 , VINITI (1988) pp. 5–101 (In Russian) Zbl 0656.22002
[20]	V.V. Gorbatsevich, A.L. Onishchik, "Lie groups of transformations" , Fundamental Directions , 20. Lie groups and Lie algebras 1 , VINITI (1988) pp. 103–240 (In Russian) MR0950863 Zbl 0656.22003
[21]	A.A. Kirillov, "Introduction to representation theory and noncommutative harmonics" , Fundamental Directions , 22. Noncommutative harmonic analysis 1 , VINITI (1988) pp. 5–162 (In Russian) MR0942947
[22]	Yu.A. Neretin, "Representations of the Virasoro algebra and of affine algebras" , Fundamental Directions , 22. Noncommutative harmonic analysis 1 , VINITI (1988) pp. 163–224 (In Russian) MR942948 Zbl 0656.17011