Rank of a module
The rank of a left module $ M $
over a ring $ R $
imbeddable in a skew-field $ k $
is the dimension of the tensor product $ k \otimes _ {R} M $,
regarded as a vector space over $ k $.
If $ R = \mathbf Z $,
the ring of integers, the definition coincides with the usual definition of the rank of an Abelian group (cf. Rank of a group). If $ k $
is a flat $ R $-
module (say, $ k $
is the skew-field of fractions of $ R $,
cf. Flat module), then the ranks of the modules in an exact sequence
$$ 0 \rightarrow M ^ \prime \rightarrow M \rightarrow M ^ {\prime\prime} \rightarrow 0 $$
satisfy the equality
$$ \mathop{\rm rk} M = \mathop{\rm rk} M ^ \prime + \mathop{\rm rk} M ^ {\prime\prime} . $$
The rank of a free module $ M $ over an arbitrary ring $ R $( cf. Free module) is defined as the number of its free generators. For rings that can be imbedded into skew-fields this definition coincides with that in 1). In general, the rank of a free module is not uniquely defined. There are rings (called $ n $- FI-rings) such that any free module over such a ring with at most $ n $ free generators has a uniquely-defined rank, while for free modules with more than $ n $ generators this property does not hold. A sufficient condition for the rank of a free module over a ring $ R $ to be uniquely defined is the existence of a homomorphism $ \phi : R \rightarrow k $ into a skew-field $ k $. In this case the concept of the rank of a module can be extended to projective modules as follows. The homomorphism $ \phi $ induces a homomorphism of the groups of projective classes $ \phi ^ {*} : K _ {0} R \rightarrow K _ {0} k \approx \mathbf Z $, and the rank of a projective module $ P $ is by definition the image of a representative of $ P $ in $ \mathbf Z $. Such a homomorphism $ \phi $ exists for any commutative ring $ R $.
References
| [1] | P.M. Cohn, "Free rings and their relations" , Acad. Press (1971) |
| [2] | J.W. Milnor, "Introduction to algebraic 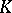 -theory" , Princeton Univ. Press (1971) -theory" , Princeton Univ. Press (1971) |
Comments
The rank of a projective module $ P $, as defined here, depends on the choice of $ \phi $.
Rank of a module. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Rank_of_a_module&oldid=48433