Auto-regressive process

A stochastic process whose values satisfy an auto-regression equation with certain constants :

(*)

where is some positive number and where the variables are usually assumed to be uncorrelated and identically distributed around their average value 0 with a variance . If all the zeros of the function of a complex variable lie inside the unit circle, then equation (*) has the solution

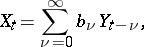where the are connected with the 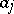by the relation

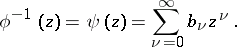For example, let be a white noise process with spectral density 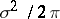; in such a case the only kind of auto-regressive process satisfying equation (*) will be a process 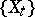with spectral density

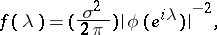which is stationary in the wide sense if has no real zeros. The autocovariances (cf. Autocovariance) of the process satisfy the recurrence relation

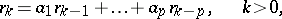and, in terms of the 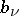, have the form

The parameters 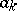of the auto-regression are connected with the auto-correlation coefficients 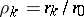of the process by the matrix relation

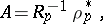where , 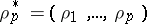and 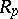is the matrix of auto-correlation coefficients (the Yule–Walker equation).

References

[1]	U. Grenander, M. Rosenblatt, "Statistical analysis of stationary time series" , Wiley (1957)
[2]	E.J. Hannan, "Time series analysis" , Methuen , London (1960)