Wiener space, abstract

The probability distribution of a Brownian motion 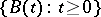is a Gaussian measure (cf. also Constructive quantum field theory) that can be supported by the space 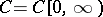of continuous functions. For this reason, 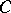is also called the classical Wiener space. This notion can be generalized to a Banach space on which a Gaussian measure can be introduced.

Advanced stochastic analysis can be carried out on a Wiener space. The analysis was initiated by P. Lévy and N. Wiener, and a systematic development was made by R.H. Cameron and W.T. Martin, I.E. Segal, L. Gross, K. Itô, and others.

Following [a2], one can introduce the notion of an abstract Wiener space, which is a generalization of the classical Wiener space.

Let 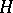be a real separable Hilbert space with norm . On on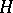e introduces the weak Gaussian distribution in such a way that on any finite-, say -, dimensional subspace 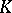of 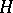the restriction of to 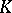is the -dimensional standard Gaussian distribution. In fact, may be called the weak white noise measure. A semi-norm (or norm) 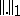on 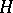is called a measurable norm if for any positive there exists a finite-dimensional projection operator 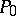such that for any finite-dimensional projection operator 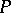orthogonal to 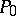the inequality holds.

Now, let 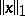be a measurable norm on and let 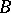be the completion of 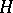with respect to this norm (cf. Complete space). Then 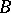is a Banach space. Let be the 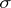-ring generated by the cylinder subsets of 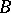(cf. Cylinder set). For a cylinder set measure 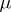on 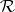induced by the Gaussian distribution on 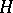, the measure 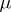is countably additive on . Therefore, taking the 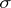-field 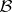generated by 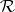, a measure space 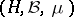is obtained.

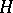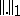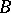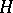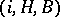The classical definition of an abstract Wiener space is given as follows. First, take a Hilbert space with norm and take a measurable norm , to obtain a Banach space . The injection mapping from into is denoted by . Then the triple is called an abstract Wiener space. This means that a weak measure on 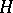can be extended to a completely additive measure supported by 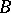. A stochastic analysis can be developed for this latter measure (see [a4]).

One of the developments of the notion of an abstract Wiener space is that of a rigged Hilbert space, due to I.M. Gel'fand and N.Ya. Vilenkin (see [a1]). Let 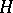be a real Hilbert space and let be a countably Hilbert nuclear space that is continuously imbedded in 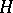. The dual space of gives rise to the rigged Hilbert space

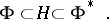Given a characteristic functional 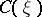, , 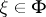that is, is continuous in , positive definite and 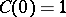, there exists a countably additive probability measure 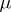in 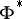such that

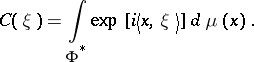A typical example of a rigged Hilbert space is the triple consisting of the Hilbert space 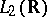, the Schwartz space and the space 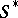of tempered distributions (cf. Generalized function). White noise is also an important example of 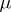on ; it has characteristic functional 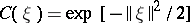. The analysis on the function space with the white noise measure is well developed (see [a3]).

References

[a1]	I.M. Gel'fand, N.Ya. Vilenkin, "Generalized functions 4: applications of harmonic analysis" , Acad. Press (1964) (In Russian)
[a2]	L. Gross, "Abstract Wiener spaces" , Proc. 5th Berkeley Symp. Math. Stat. Probab. , 2 (1965) pp. 31–42
[a3]	T. Hida, H.H. Kuo, J. Potthoff, L. Streit, "White noise. An infinite dimensional calculus" , Kluwer Acad. Publ. (1993)
[a4]	H.H. Kuo, "Gaussian measures in Banach spaces" , Lecture Notes in Mathematics , 463 , Springer (1975)