Trigonometric functions
The class of elementary functions sine, cosine, tangent, cotangent, secant, cosecant. These are denoted, respectively, by: $ \sin x $,
$ \cos x $,
$ \mathop{\rm tan} x $(
or $ \mathop{\rm tg} x $),
$ \mathop{\rm cot} x $(
or $ \mathop{\rm cotan} x $),
$ \mathop{\rm sec} x $,
$ \mathop{\rm csc} x $(
or $ \cosec x $).
Trigonometric functions of a real argument.
Let $ \alpha $ be a real number. Let $ A = ( x _ \alpha , y _ \alpha ) $ be the end point of the arc on the unit circle $ x ^ {2} + y ^ {2} = 1 $( see Fig. a) having initial point $ B = ( 1, 0) $ and length $ | \alpha | $. The arc from $ B $ to $ A $ is taken in the counter-clockwise direction if $ \alpha \geq 0 $, and in the clockwise direction if $ \alpha < 0 $. If $ \alpha = 0 $, then $ A = B $; if, e.g., $ \alpha = (- 7 \pi )/ ( 2) $, then $ A = ( 0, 1) $. $ B $, $ | BA | = \alpha > 0 $

Figure: t094210a
The basic trigonometric functions sine and cosine are defined at $ \alpha $ by the formulas
$$ \sin \alpha = \ y _ \alpha ,\ \ \cos \alpha = \ x _ \alpha . $$
The remaining trigonometric functions can be defined by the formulas
$$ \mathop{\rm tan} \alpha = \ \frac{\sin \alpha }{\cos \alpha } ,\ \ \mathop{\rm cot} \alpha = \ \frac{\cos \alpha }{\sin \alpha } , $$
$$ \mathop{\rm sec} \alpha = { \frac{1}{\cos \alpha } } ,\ \ \mathop{\rm csc} \alpha = { \frac{1}{\sin \alpha } } . $$
All trigonometric functions are periodic. The graphs of the trigonometric functions are given in Fig. b.
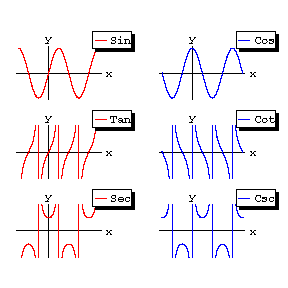
Figure: t094210b
The main properties of the trigonometric functions — the domain of definition, the range, the parity, and sections of monotonicity — are given in the table below.
<tbody> </tbody>
|
Each trigonometric function is continuous and infinitely differentiable at each point of its domain of definition; the derivatives of the trigonometric functions are:
$$ ( \sin x) ^ \prime = \cos x,\ \ ( \cos x) ^ \prime = - \sin x, $$
$$ ( \mathop{\rm tan} x) ^ \prime = { \frac{1}{\cos ^ {2} x } } ,\ ( \mathop{\rm cot} x) ^ \prime = - { \frac{1}{\sin ^ {2} x } } . $$
The integrals of the trigonometric functions are:
$$ \int\limits \sin x dx = - \cos x + C,\ \ \int\limits \cos x dx = \sin x + C, $$
$$ \int\limits \mathop{\rm tan} x dx = - \mathop{\rm ln} | \cos x | + C, \int\limits \mathop{\rm cot} x dx = \mathop{\rm ln} | \sin x | + C. $$
All trigonometric functions have a power series expansion:
$$ \sin x = x - \frac{x ^ {3} }{3! } + \frac{x ^ {5} }{5! } - \dots + (- 1) ^ {n} \frac{x ^ {2n + 1 } }{( 2n + 1)! } + \dots $$
for $ | x | < \infty $;
$$ \cos x = 1 - \frac{x ^ {2} }{2! } + \frac{x ^ {4} }{4! } - \frac{x ^ {6} }{6! } + \dots + (- 1) ^ {n} \frac{x ^ {2n} }{( 2n)! } + \dots $$
for $ | x | < \infty $;
$$ \mathop{\rm tan} x = \ x + { \frac{1}{3} } x ^ {3} + { \frac{2}{15} } x ^ {5} + { \frac{17}{315} } x ^ {7} + \dots $$
$$ \dots + \frac{2 ^ {2n} ( 2 ^ {2n} - 1) | B _ {n} | }{( 2n)! } x ^ {2n - 1 } + \dots $$
for $ | x | < {\pi / 2 } $;
$$ \mathop{\rm cot} x = { \frac{1}{x} } - $$
$$ - \left [ { \frac{x}{3} } + \frac{x ^ {3} }{45 } + \frac{2x ^ {5} }{945 } + \frac{x ^ {7} }{4725 } + \dots + \frac{2 ^ {2n} | B _ {n} | }{( 2n)! } x ^ {2n - 1 } + \dots \right ] $$
for $ 0 < | x | < \pi $( the $ B _ {n} $ are the Bernoulli numbers).
The function inverse to the function $ x = \sin y $ defines $ y $ as a many-valued function of $ x $, it is denoted by $ y = \mathop{\rm arc} \sin x $. The inverse functions of the other trigonometric functions are defined similarly; they are all called inverse trigonometric functions.
Trigonometric functions of a complex variable.
The trigonometric functions for complex values of the variable $ z = x + iy $ are defined as analytic continuations (cf. Analytic continuation) of the corresponding trigonometric functions of the real variable in the complex plane.
Thus, $ \sin z $ and $ \cos z $ can be defined by means of the power series for $ \sin x $ and $ \cos x $ given above. These series converge in the entire complex plane, therefore $ \sin z $ and $ \cos z $ are entire functions (cf. Entire function).
The trigonometric functions tangent and cotangent are defined by the formulas
$$ \mathop{\rm tan} z = \ \frac{\sin z }{\cos z } ,\ \ \mathop{\rm cot} z = \ \frac{\cos z }{\sin z } . $$
The trigonometric functions $ \mathop{\rm tan} z $ and $ \mathop{\rm cot} z $ are meromorphic functions (cf. Meromorphic function). The poles of $ \mathop{\rm tan} z $ are simple (of order one) and are situated at the points $ z = \pi / 2 + \pi n $, $ n = 0, \pm 1 ,\dots $.
All formulas for the trigonometric functions of a real argument remain true for a complex argument as well.
In contrast to the trigonometric functions of a real variable, the functions $ \sin z $ and $ \cos z $ take all complex values: The equations $ \sin z = a $ and $ \cos z = a $ each have infinitely many solutions for any complex $ a $:
$$ z = \mathop{\rm arc} \sin a = \ - i \mathop{\rm ln} ( ia \pm \sqrt {1 - a ^ {2} } ), $$
$$ z = \mathop{\rm arc} \cos a = - i \mathop{\rm ln} ( a \pm \sqrt {a ^ {2} - 1 } ). $$
The trigonometric functions $ \mathop{\rm tan} z $ and $ \mathop{\rm cot} z $ take all complex values except $ \pm i $: The equations $ \mathop{\rm tan} z = a $, $ \mathop{\rm cot} z = a $ each have infinitely many solutions for any complex number $ a \neq \pm i $:
$$ z = \mathop{\rm arc} \mathop{\rm tan} a = \ { \frac{i}{2} } \mathop{\rm ln} \frac{1 - ia }{1 + ia } , $$
$$ z = \mathop{\rm arc} \mathop{\rm cot} a = { \frac{i}{2} } \mathop{\rm ln} \frac{ia + 1 }{ia - 1 } . $$
The trigonometric functions can be expressed in terms of the exponential function:
$$ \sin z = { \frac{1}{2i} } ( e ^ {iz} - e ^ {-} iz ), $$
$$ \cos z = { \frac{1}{2} } ( e ^ {iz} + e ^ {-} iz ),\ \ \mathop{\rm tan} z = { \frac{1}{i} } \frac{e ^ {iz} - e ^ {-} iz }{e ^ {iz} + e ^ {-} iz } , $$
and the hyperbolic functions:
$$ \sin z = - i \sinh iz,\ \ \cos z = \cosh iz,\ \ \mathop{\rm tan} z = - i \mathop{\rm tanh} iz. $$
Comments
The trigonometric functions are also called circular functions.
A formal definition of $ \sin z $ and $ \cos z $( independent of a picture) can be given by power series and as follows. First of all it can easily be proved that from the previous, visual definition of $ \sin x $ and $ \cos x $ follows:
$$ \mathop{\rm arc} \sin x = \int\limits _ { 0 } ^ { x } \frac{dx}{\sqrt {1- t ^ {2} } } . $$
This can be taken as a formal definition of $ \mathop{\rm arc} \sin x $ and the inverse function of $ \mathop{\rm arc} \sin x $ can be taken as a formal definition of $ \sin x $.
If $ z $ is a complex number $ x + iy $, with real $ x $ and $ y $, one can define $ e ^ {z} = e ^ {x} ( \cos y + i \sin y ) $, and then for complex $ z $ define:
$$ \sin z = \frac{e ^ {iz} - e ^ {-} iz }{2i} ,\ \ \cos z = \frac{e ^ {iz} + e ^ {-} iz }{2} . $$
References
| [a1] | T.M. Apostol, "Calculus" , I , Blaisdell (1967) |
| [a2] | A.R.F. Verhey, "Complex variables and applications" , McGraw-Hill (1974) |
| [a3] | M. Abramowitz, I.A. Stegun, "Handbook of mathematical functions" , Dover, reprint (1972) |
Trigonometric functions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Trigonometric_functions&oldid=49035