Topology of imbeddings
The branch of topology in which one studies local topological properties of dispositions of closed subsets of a Euclidean space or manifold.
The topology of imbeddings arose in the work of A. Schoenflies, L. Antoine, P.S. Urysohn, and J. Alexander. Imbeddings in 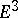 were studied in the 1950s. In particular, it was proved that an imbedding of a surface in
were studied in the 1950s. In particular, it was proved that an imbedding of a surface in 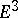 can be topologically approximated by a polyhedral imbedding. The systematic study of the topology of imbeddings in
can be topologically approximated by a polyhedral imbedding. The systematic study of the topology of imbeddings in 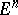 for
for  began after the solution of the Schoenflies conjecture. Basically it came about in an environment of accumulation of facts and solutions to a large number of problems of a special character. Relations between methods of the theory of the topology of imbeddings and the geometric topology of manifolds were also clarified. At approximately the middle of the 1970s, the topology of imbeddings was formulated as an independent branch with its own themes, methods and problems. It was used to solve a number of basic problems in the geometric topology of manifolds: the existence was proved of a non-combinatorial triangulation of spheres of dimension
began after the solution of the Schoenflies conjecture. Basically it came about in an environment of accumulation of facts and solutions to a large number of problems of a special character. Relations between methods of the theory of the topology of imbeddings and the geometric topology of manifolds were also clarified. At approximately the middle of the 1970s, the topology of imbeddings was formulated as an independent branch with its own themes, methods and problems. It was used to solve a number of basic problems in the geometric topology of manifolds: the existence was proved of a non-combinatorial triangulation of spheres of dimension 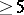 , the characterization of topological manifolds was obtained and the simply-connected four-dimensional manifolds were classified.
, the characterization of topological manifolds was obtained and the simply-connected four-dimensional manifolds were classified.
A topological imbedding of a space  (as a rule, a manifold, a polyhedron or a compact set) in a Euclidean space
(as a rule, a manifold, a polyhedron or a compact set) in a Euclidean space 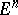 is an arbitrary homeomorphism
is an arbitrary homeomorphism 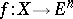 from
from 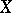 onto a space
onto a space 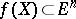 . Sometimes, a topological imbedding is simply understood to be an inclusion
. Sometimes, a topological imbedding is simply understood to be an inclusion  . Two imbeddings
. Two imbeddings 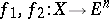 are said to be equivalent if there exists a homeomorphism
are said to be equivalent if there exists a homeomorphism  such that
such that 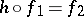 . If
. If  is an isotopy, then the imbeddings are said to be isotopic.
is an isotopy, then the imbeddings are said to be isotopic.
The simplest examples of non-equivalent imbeddings are obtained using knots (see Knot theory); it is much more difficult to construct non-equivalent imbeddings of zero-dimensional compacta or segments in 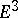 (see Wild knot).
(see Wild knot).
A Cantor set on a rectilinear segment lying in 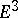 and a wild zero-dimensional Antoine compactum in
and a wild zero-dimensional Antoine compactum in 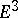 are non-equivalent.
are non-equivalent.
The fact that the basic problems of the theory of topological imbeddings concentrate on local properties is explained by the existence of so-called wild imbeddings, for which the regularity of the local structure is destroyed. The study of global properties of tame (locally flat) imbeddings is, as a rule, not included in the topology of imbeddings (cf. also Tame imbedding).
The following four theorems may be considered as fundamental in the theory of topological imbeddings.
Theorem 1 (characterization).
An imbedding  is tame if and only if the complement
is tame if and only if the complement 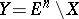 has the property 1-ULC (for an arbitrary
has the property 1-ULC (for an arbitrary 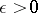 there exists a
there exists a 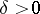 such that each
such that each 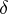 -mapping
-mapping 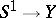 is
is  -homotopically zero in
-homotopically zero in  ).
).
Theorem 2 (on close imbeddings).
Any two close tame imbeddings are isotopic by a small isotopy.
Theorem 3 (on imbeddings in a trivial-dimensional domain).
If 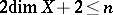 , then any two tame imbeddings are isotopic.
, then any two tame imbeddings are isotopic.
Theorem 4 (on approximation).
Any imbedding can be approximated by a tame imbedding. With the exception of theorem 3, all these theorems have been proved only under certain restrictions on the dimensions; these restrictions are different for manifolds, polyhedra and compacta.
An imbedding of a manifold  in
in  is said to be tame (or locally flat) if for an arbitrary point
is said to be tame (or locally flat) if for an arbitrary point 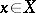 there exists a neighbourhood
there exists a neighbourhood 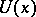 in
in 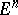 such that the pair
such that the pair  is homeomorphic to the standard pair
is homeomorphic to the standard pair 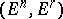 under a homeomorphism transferring the point to the origin of
under a homeomorphism transferring the point to the origin of 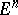 .
.
Theorem 1 holds if  and
and 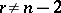 (if
(if 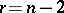 and
and 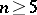 the answer is also known: It is necessary for the complement
the answer is also known: It is necessary for the complement 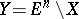 to be, roughly speaking, homotopically equivalent to a circle).
to be, roughly speaking, homotopically equivalent to a circle).
Theorem 2 holds if  and
and 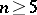 (the addition of a small knot shows that for
(the addition of a small knot shows that for 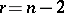 , theorem 2 is obviously false; a condition for two imbeddings to be isotopically close is known when
, theorem 2 is obviously false; a condition for two imbeddings to be isotopically close is known when 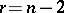 ). In addition, if
). In addition, if 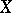 is the sphere
is the sphere 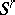 , it has been proved that an arbitrary tame imbedding
, it has been proved that an arbitrary tame imbedding  is isotopically standard if
is isotopically standard if  (if
(if  and
and 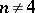 this is true if and only if the complement
this is true if and only if the complement 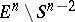 is homotopically equivalent to a circle).
is homotopically equivalent to a circle).
Theorem 4 holds if 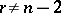 and
and 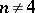 (moreover, if
(moreover, if 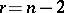 and
and 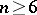 this theorem — as corresponding counterexamples show — is obviously false).
this theorem — as corresponding counterexamples show — is obviously false).
An imbedding of a polyhedron  in
in 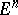 is said to be tame if it is equivalent to a piecewise-linear imbedding. Theorems 1, 2 and 3 hold if
is said to be tame if it is equivalent to a piecewise-linear imbedding. Theorems 1, 2 and 3 hold if  .
.
An imbedding of an  -dimensional compactum
-dimensional compactum 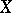 in
in 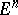 is said to be tame if it is possible to remove it by an isotopy from an arbitrary rectilinear polyhedron
is said to be tame if it is possible to remove it by an isotopy from an arbitrary rectilinear polyhedron 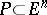 of dimension
of dimension 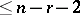 . Theorem 1 holds if
. Theorem 1 holds if  and
and 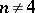 , theorem 2 is, generally speaking, false (if
, theorem 2 is, generally speaking, false (if  ), while theorem 4 holds for arbitrary
), while theorem 4 holds for arbitrary  .
.
References
| [1] | L.V. Keldysh, "Topological imbeddings in a Euclidean space" , Amer. Math. Soc. (1968) (Translated from Russian) |
| [2] | A.V. Chernavskii, "Locally homotopic unknotted imbeddings of manifolds" Soviet Math. Dokl. , 9 : 4 (1968) pp. 835–839 Dokl. Akad. Nauk SSSR , 181 : 2 (1968) pp. 290–293 |
| [3] | A.V. Chernavskii, "Coincidence of local flatness and local simple-connectedness for embeddings of (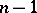 )-dimensional manifolds in )-dimensional manifolds in  -dimensional manifolds when -dimensional manifolds when 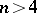 " Math. USSR Sb. , 20 (1973) pp. 297–304 Mat. Sb. , 91 : 2 (1973) pp. 279–286 " Math. USSR Sb. , 20 (1973) pp. 297–304 Mat. Sb. , 91 : 2 (1973) pp. 279–286 |
| [4] | R. Daverman, "Locally nice codimension one manifolds are locally flat" Bull. Amer. Math. Soc. , 79 : 2 (1973) pp. 410–413 |
| [5] | T. Chapman, "Locally homotopically unknotted embeddings of manifolds in codimension two are locally flat" Topology , 18 (1979) pp. 339–348 |
| [6] | F. Ancel, J. Cannon, "The locally flat approximation of cell-like embeddings" Ann. of Math. , 109 (1979) pp. 61–86 |
| [7] | J. Bryant, C.L. Seebeck, "Locally nice embeddings of polyhedra" Quart. J. Math. , 19 (1968) pp. 257–274 |
| [8] | R.D. Edwards, "The equivalence of close piecewise linear embeddings" General Topol. Appl. , 5 : 2 (1975) pp. 147–180 |
| [9] | R.T. Miller, "Approximating codimension three embeddings" Ann. of Math. , 95 : 3 (1972) pp. 406–416 |
| [10] | J.L. Bryant, "Approximating embeddings of polyhedra in codimension three" Trans. Amer. Math. Soc. , 170 (1972) pp. 85–95 |
| [11] | R.D. Edwards, "Dimension theory I" L.C. Glase (ed.) T.B. Rushing (ed.) , Geometric Topology (Utah, 1974) , Lect. notes in math. , 438 , Springer (1975) pp. 195–211 |
| [12] | M.A. Shtan'ko, "Approximation of compacta in codimension greater than two" Math. USSR Sb. , 19 : 4 (1973) pp. 615–626 Mat. Sb. , 90 : 4 (1973) pp. 625–636 |
Comments
The question of whether a given space admits an imbedding in a particular 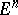 and the classification of such imbeddings have been the subject of much research in algebraic topology. H. Whitney [a1] proved that every smooth
and the classification of such imbeddings have been the subject of much research in algebraic topology. H. Whitney [a1] proved that every smooth  -dimensional manifold can be imbedded in
-dimensional manifold can be imbedded in  (
(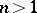 ). The theory of characteristic classes (cf. Characteristic class) yields necessary conditions for a given
). The theory of characteristic classes (cf. Characteristic class) yields necessary conditions for a given  -manifold to imbed in lower dimensions. For example, real projective space of dimension
-manifold to imbed in lower dimensions. For example, real projective space of dimension  cannot be imbedded in
cannot be imbedded in  . A. Haeflinger [a2] gave sufficient conditions for certain ranges of dimension. W.-T. Wu [a3], [a4] has developed algebraic invariants for imbeddings of simplicial complexes.
. A. Haeflinger [a2] gave sufficient conditions for certain ranges of dimension. W.-T. Wu [a3], [a4] has developed algebraic invariants for imbeddings of simplicial complexes.
Cf. also Tight and taut immersions.
References
| [a1] | H. Whitney, "The self-intersections of a smooth  -manifold in -manifold in 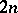 -space" Ann. of Math. , 45 (1944) pp. 220–246 -space" Ann. of Math. , 45 (1944) pp. 220–246 |
| [a2] | A. Haeflinger, "Plongements différentiables dans la domaine stable" Comm. Math. Helvetici , 37 (1963) pp. 155–176 |
| [a3] | W.-T. Wu, "On the realization of complexes in Euclidean spaces I" Scientia Sinica , 7 (1958) pp. 251–297 ((In Chinese; English abstract.)) |
| [a4] | W.-T. Wu, "On the realization of complexes in Euclidean spaces II" Scientia Sinica , 8 (1959) pp. 79–94 ((In Chinese; English abstract.)) |
| [a5] | E.E. Moise, "Geometric topology in dimensions 2 and 3" , Springer (1977) |
Topology of imbeddings. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Topology_of_imbeddings&oldid=16116