Topological vector space
over a topological field 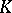
A vector space  over
over 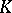 equipped with a topology (cf. Topological structure (topology)) that is compatible with the vector space structure, that is, the following axioms are satisfied: 1) the mapping
equipped with a topology (cf. Topological structure (topology)) that is compatible with the vector space structure, that is, the following axioms are satisfied: 1) the mapping 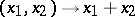 ,
,  , is continuous; and 2) the mapping
, is continuous; and 2) the mapping 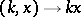 ,
, 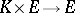 , is continuous (here the products
, is continuous (here the products  and
and 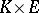 are thought of as having the product topologies). Entirely analogously, one can define topological left and right vector spaces over a (not necessarily commutative) topological division ring. Sometimes a topological vector space
are thought of as having the product topologies). Entirely analogously, one can define topological left and right vector spaces over a (not necessarily commutative) topological division ring. Sometimes a topological vector space 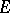 with a topology
with a topology  is denoted by
is denoted by 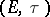 . On the other hand, reference to the field
. On the other hand, reference to the field 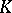 is often not made.
is often not made.
Two topological vector spaces 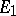 and
and 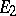 over the same topological field are said to be isomorphic if there exists a continuous bijective linear transformation from
over the same topological field are said to be isomorphic if there exists a continuous bijective linear transformation from  onto
onto  whose inverse is also continuous. The dimension of a topological vector space
whose inverse is also continuous. The dimension of a topological vector space 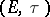 is the dimension of the vector space
is the dimension of the vector space 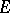 .
.
Methods for specifying a topology in a topological vector space, and properties of the topology.
Let 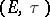 be a topological vector space over a topological field
be a topological vector space over a topological field  . The topology
. The topology  is invariant under translations (that is, for any
is invariant under translations (that is, for any  the mapping
the mapping 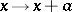 is a homeomorphism from
is a homeomorphism from 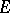 onto itself). Hence the topology
onto itself). Hence the topology  is uniquely determined by a base (basis, fundamental system) of neighbourhoods of any fixed point (in particular, of zero). The topology
is uniquely determined by a base (basis, fundamental system) of neighbourhoods of any fixed point (in particular, of zero). The topology  is compatible with the additive group structure of
is compatible with the additive group structure of  , and the following propositions hold. 1)
, and the following propositions hold. 1) 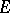 is Hausdorff (separated) if and only if, for every point
is Hausdorff (separated) if and only if, for every point 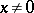 of
of 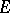 , there exists a neighbourhood of zero which does not contain
, there exists a neighbourhood of zero which does not contain  . 2) If
. 2) If 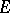 is Hausdorff, then it is completely regular. 3) There exists a unique uniform structure (cf. also Uniform space) on
is Hausdorff, then it is completely regular. 3) There exists a unique uniform structure (cf. also Uniform space) on  with the following properties: a) it is invariant under translations (that is, all translations are uniformly continuous); and b) the topology associated with it coincides with the original topology of the space
with the following properties: a) it is invariant under translations (that is, all translations are uniformly continuous); and b) the topology associated with it coincides with the original topology of the space 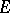 . A set in a topological vector space is called complete if it is complete with respect to the above-mentioned uniform structure. Thus, a topological vector space
. A set in a topological vector space is called complete if it is complete with respect to the above-mentioned uniform structure. Thus, a topological vector space 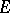 is complete if every Cauchy filter in
is complete if every Cauchy filter in 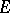 converges. For every topological vector space
converges. For every topological vector space 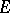 there exists a complete topological vector space, over the same field, containing
there exists a complete topological vector space, over the same field, containing 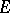 as an everywhere-dense subset and inducing the original topology and linear structure on
as an everywhere-dense subset and inducing the original topology and linear structure on 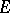 . It is called the completion of
. It is called the completion of 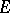 . Every Hausdorff topological vector space has a Hausdorff completion, unique up to an isomorphism fixing
. Every Hausdorff topological vector space has a Hausdorff completion, unique up to an isomorphism fixing 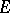 pointwise.
pointwise.
From now on, unless explicitly stated otherwise,  will be assumed to be a non-discretely normed field equipped with the topology induced by the norm (cf. Norm on a field). If
will be assumed to be a non-discretely normed field equipped with the topology induced by the norm (cf. Norm on a field). If 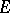 is a vector space over
is a vector space over 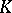 , then a set
, then a set 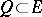 is called circled (or balanced) if
is called circled (or balanced) if 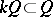 for all
for all 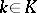 with
with 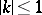 . If
. If 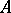 and
and 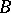 are two subsets of
are two subsets of 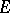 , then one says that
, then one says that 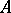 absorbs
absorbs 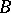 if there exists a positive number
if there exists a positive number  such that
such that  for all
for all 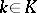 with
with  . A subset of
. A subset of 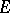 is called absorbing (or radial) if it absorbs every one point set. In every topological vector space
is called absorbing (or radial) if it absorbs every one point set. In every topological vector space 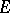 over
over  there exists a base
there exists a base  of closed neighbourhoods of zero with the following properties:
of closed neighbourhoods of zero with the following properties: 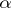 ) for every set
) for every set 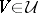 there exists a
there exists a 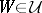 such that
such that 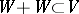 ;
; 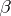 ) every
) every 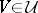 is a balanced absorbing set; and
is a balanced absorbing set; and 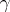 ) if
) if 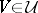 , then
, then 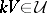 for every
for every 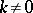 in
in  . On the other hand, let
. On the other hand, let  be a topology on a vector space
be a topology on a vector space 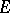 over
over 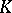 which is invariant under translations and has a base of neighbourhoods of zero satisfying properties
which is invariant under translations and has a base of neighbourhoods of zero satisfying properties 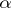 ) and
) and 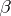 ), and also the following property:
), and also the following property: 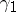 ) there exists a
) there exists a 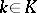 ,
, 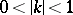 , such that if
, such that if 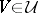 , then
, then 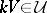 . Then
. Then 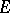 , together with the topology
, together with the topology  , is a topological vector space over
, is a topological vector space over  (in case the norm on
(in case the norm on 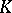 is Archimedean (cf. Archimedean axiom),
is Archimedean (cf. Archimedean axiom), 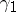 ) is a consequence of the other properties imposed on
) is a consequence of the other properties imposed on 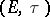 ). Every filter base
). Every filter base 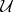 in a vector space
in a vector space 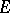 over
over 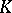 which satisfies properties
which satisfies properties 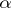 ),
), 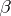 ) and
) and  ), or at least
), or at least 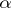 ) and
) and 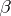 ) in the case of a field with an Archimedean norm, is a fundamental system of neighbourhoods of zero (not necessarily closed) for some uniquely determined topology
) in the case of a field with an Archimedean norm, is a fundamental system of neighbourhoods of zero (not necessarily closed) for some uniquely determined topology  on
on 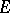 that is compatible with the vector-space structure on
that is compatible with the vector-space structure on 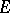 . A topological vector space
. A topological vector space 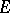 over the field
over the field 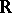 of real numbers or the field
of real numbers or the field 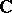 of complex numbers, and its topology, are called locally convex if
of complex numbers, and its topology, are called locally convex if 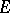 has a base of neighbourhoods of zero consisting of convex sets (the definition of a locally convex space sometimes requires also that the space be Hausdorff).
has a base of neighbourhoods of zero consisting of convex sets (the definition of a locally convex space sometimes requires also that the space be Hausdorff).
Examples.
1) Every topological field  can be thought of as a (one-dimensional) topological vector space over itself. Considered in this way, it will be denoted by
can be thought of as a (one-dimensional) topological vector space over itself. Considered in this way, it will be denoted by 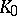 .
.
2) Let  be a non-empty set, and let
be a non-empty set, and let 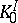 be the vector space over
be the vector space over  which is the product of
which is the product of 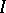 copies of
copies of 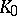 , equipped with the product topology. Then
, equipped with the product topology. Then 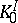 is a topological vector space.
is a topological vector space.
3) If the topology of the topological field 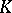 is discrete, then every vector space
is discrete, then every vector space 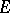 over
over 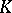 , with a topology that is compatible with its additive group structure and that is invariant under multiplication by any non-zero element of
, with a topology that is compatible with its additive group structure and that is invariant under multiplication by any non-zero element of  , is a topological vector space (these conditions are satisfied, in particular, by the discrete topology on
, is a topological vector space (these conditions are satisfied, in particular, by the discrete topology on 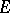 ). A topological vector space over a field with the discrete topology is called a topological vector group.
). A topological vector space over a field with the discrete topology is called a topological vector group.
4) Let 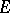 be a vector space over the topological field
be a vector space over the topological field  , and let
, and let 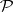 be a set of semi-norms on
be a set of semi-norms on 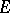 . The ball of radius
. The ball of radius 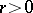 in the semi-norm
in the semi-norm 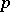 on
on 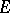 is the set
is the set 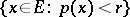 . The set of all intersections of finite collections of balls (of all (positive) radii) in (all) semi-norms belonging to
. The set of all intersections of finite collections of balls (of all (positive) radii) in (all) semi-norms belonging to 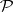 , forms a base of neighbourhoods of zero for a certain topology
, forms a base of neighbourhoods of zero for a certain topology 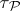 on
on 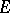 that is compatible with the vector-space structure. One says that this topology is given, or defined, by
that is compatible with the vector-space structure. One says that this topology is given, or defined, by 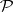 . If
. If 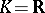 or
or 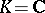 , then
, then 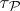 is locally convex. Conversely, the topology of any locally convex space can be defined by some set of semi-norms — for example, by the set of gauge functions (Minkowski functionals) of an arbitrary subbase of neighbourhoods of zero consisting of balanced convex sets.
is locally convex. Conversely, the topology of any locally convex space can be defined by some set of semi-norms — for example, by the set of gauge functions (Minkowski functionals) of an arbitrary subbase of neighbourhoods of zero consisting of balanced convex sets.
A subset of a topological vector space is called bounded if it is absorbed by every neighbourhood of zero.
A topological vector space is called normable if its topology can be defined by a single norm. A topological vector space over 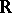 or
or  is normable if and only if it is Hausdorff and has a convex bounded neighbourhood of zero (Kolmogorov's theorem).
is normable if and only if it is Hausdorff and has a convex bounded neighbourhood of zero (Kolmogorov's theorem).
5) Let  be a natural number, let
be a natural number, let 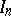 be a set containing
be a set containing  elements and let
elements and let 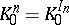 . The topology of
. The topology of 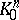 is defined by the norm
is defined by the norm 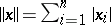 , where the symbol
, where the symbol  denotes the norm in
denotes the norm in 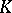 . If the field
. If the field 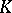 is complete, then every
is complete, then every  -dimensional topological vector space over
-dimensional topological vector space over  is isomorphic to
is isomorphic to 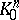 (for
(for 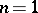 this holds even without the completeness assumption on
this holds even without the completeness assumption on  ). If the field
). If the field  is locally compact, then a Hausdorff topological vector space over
is locally compact, then a Hausdorff topological vector space over  is finite dimensional if and only if it has a pre-compact neighbourhood of zero (Tikhonov's theorem).
is finite dimensional if and only if it has a pre-compact neighbourhood of zero (Tikhonov's theorem).
A topological vector space is called metrizable if its topology can be defined by some metric (among all such metrics, there always exists one that is invariant under translations). A topological vector space is metrizable if and only if it is Hausdorff and has a countable base of neighbourhoods of zero.
6) Let 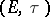 be a topological vector space, let
be a topological vector space, let  be a vector subspace of
be a vector subspace of 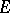 and let
and let 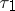 be the subspace topology on
be the subspace topology on 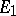 . The topology
. The topology 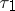 is compatible with the vector-space structure of
is compatible with the vector-space structure of 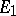 . The topological vector space
. The topological vector space 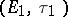 is called a topological vector subspace of the topological vector space
is called a topological vector subspace of the topological vector space 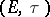 . If
. If 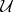 is a base (respectively, subbase) of neighbourhoods of zero in
is a base (respectively, subbase) of neighbourhoods of zero in 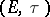 , then the set
, then the set 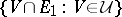 forms a base (respectively, subbase) of neighbourhoods of zero in
forms a base (respectively, subbase) of neighbourhoods of zero in 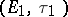 . If
. If 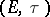 is Hausdorff (respectively, metrizable, locally convex), then so is
is Hausdorff (respectively, metrizable, locally convex), then so is  . If the topology
. If the topology  is given by some set of semi-norms, then the topology
is given by some set of semi-norms, then the topology  is given by the restrictions of these semi-norms to
is given by the restrictions of these semi-norms to 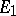 .
.
7) Let 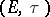 and
and 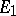 be as in 6) above, and let
be as in 6) above, and let 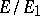 be the quotient vector space of
be the quotient vector space of 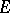 by the subspace
by the subspace 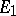 . The quotient topology
. The quotient topology  on
on 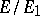 is compatible with the vector-space structure on
is compatible with the vector-space structure on 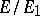 . The topological vector space
. The topological vector space  is called the topological vector quotient space of
is called the topological vector quotient space of 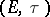 over
over 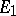 . (By the definition of a quotient space, a set
. (By the definition of a quotient space, a set 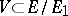 is closed in
is closed in 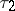 if and only if its inverse image under the canonical mapping
if and only if its inverse image under the canonical mapping  is closed in
is closed in 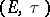 .) If
.) If 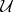 is a base of neighbourhoods of zero in
is a base of neighbourhoods of zero in 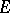 , then the set of images of its elements under the canonical mapping
, then the set of images of its elements under the canonical mapping 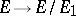 forms a base of neighbourhoods of zero in
forms a base of neighbourhoods of zero in 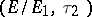 (for a subbase, this does not hold in general). The topological vector space
(for a subbase, this does not hold in general). The topological vector space  is Hausdorff if and only if the subspace
is Hausdorff if and only if the subspace 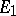 is closed in
is closed in 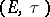 . If
. If 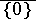 denotes the closure of the singleton set
denotes the closure of the singleton set 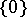 in
in 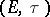 , then the (Hausdorff) topological vector space
, then the (Hausdorff) topological vector space  is called the Hausdorff topological vector space associated with
is called the Hausdorff topological vector space associated with 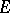 . Of course, if
. Of course, if 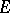 itself is Hausdorff, then the associated Hausdorff topological vector space is isomorphic to it. If
itself is Hausdorff, then the associated Hausdorff topological vector space is isomorphic to it. If 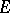 is locally convex (respectively, if
is locally convex (respectively, if 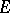 is metrizable and
is metrizable and  is closed; or if
is closed; or if 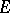 is metrizable and complete), then
is metrizable and complete), then 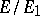 is locally convex (respectively, metrizable, complete). However,
is locally convex (respectively, metrizable, complete). However, 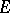 can be complete (non-metrizable) and have a non-complete topological vector quotient (even a separable metrizable one) (see below).
can be complete (non-metrizable) and have a non-complete topological vector quotient (even a separable metrizable one) (see below).
8) Let 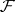 be the vector space of all Lebesgue-measurable real-valued functions on
be the vector space of all Lebesgue-measurable real-valued functions on  , let
, let  be the Lebesgue measure on this interval and, for each
be the Lebesgue measure on this interval and, for each 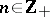 , let
, let
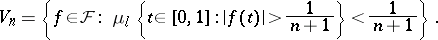 |
The set 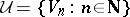 forms a filter base in
forms a filter base in 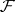 with the properties
with the properties 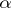 ) and
) and 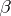 ). Let
). Let  be the topology compatible with the vector-space structure on
be the topology compatible with the vector-space structure on  with base of neighbourhoods of zero
with base of neighbourhoods of zero 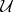 , and let
, and let 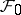 be the Hausdorff topological vector space associated with
be the Hausdorff topological vector space associated with 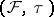 (
( itself is not Hausdorff). The topological vector space
itself is not Hausdorff). The topological vector space 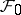 is metrizable, but not locally convex. As a vector space, it can be identified with the space of
is metrizable, but not locally convex. As a vector space, it can be identified with the space of 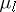 -equivalence classes of
-equivalence classes of 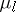 -measurable real-valued functions on
-measurable real-valued functions on  . Convergence of a sequence in
. Convergence of a sequence in  (respectively,
(respectively, 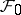 ) is the same as convergence in measure (of individual functions in the first case, and of
) is the same as convergence in measure (of individual functions in the first case, and of 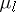 -equivalence classes in the second).
-equivalence classes in the second).
From now on 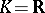 or
or 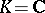 .
.
9) Let 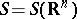 be the vector space of all infinitely-differentiable functions
be the vector space of all infinitely-differentiable functions 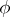 on
on 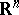 , with values in
, with values in  , for which
, for which  : For all
: For all 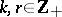 ,
,
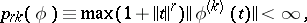 |
where
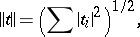 |
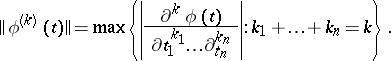 |
Endowed with the topology 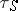 given by the collection of norms
given by the collection of norms 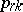 defined by the above equations,
defined by the above equations, 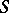 is a complete, metrizable, locally convex space (such spaces are called Fréchet spaces). The space
is a complete, metrizable, locally convex space (such spaces are called Fréchet spaces). The space 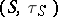 plays an important part in the theory of generalized functions (cf. Generalized function). It is interesting that there is no norm on
plays an important part in the theory of generalized functions (cf. Generalized function). It is interesting that there is no norm on 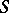 for which
for which  is a Banach space and in which the functions
is a Banach space and in which the functions 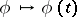 ,
,  (
(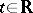 ), are all continuous (in particular,
), are all continuous (in particular, 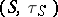 is not normable).
is not normable).
Some methods for constructing topological vector spaces.
1) Projective topologies. Let 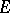 be a vector space, and for each
be a vector space, and for each 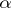 in some index set
in some index set 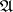 , let
, let 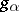 be a linear transformation from
be a linear transformation from 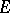 into a topological vector space
into a topological vector space 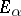 . Then among all the topologies on
. Then among all the topologies on 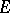 for which all mappings
for which all mappings 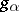 are continuous, there is a weakest one
are continuous, there is a weakest one  (the supremum of the set of topologies
(the supremum of the set of topologies  , where
, where 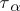 is the topology on
is the topology on 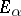 for each
for each 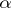 ). The topology
). The topology  is called a projective topology, and the space
is called a projective topology, and the space 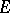 endowed with
endowed with  is called the projective limit of the collection of spaces
is called the projective limit of the collection of spaces 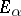 with respect to the mappings
with respect to the mappings 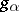 . The topology
. The topology  is compatible with the vector-space structure on
is compatible with the vector-space structure on 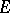 , and if all spaces
, and if all spaces 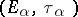 are locally convex, then so is
are locally convex, then so is 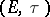 . (Sometimes the term "projective limit" is used to denote a more special construction, and not as a synonym for "space with a projective topology" , cf. Locally convex space.)
. (Sometimes the term "projective limit" is used to denote a more special construction, and not as a synonym for "space with a projective topology" , cf. Locally convex space.)
Examples of projective limits.
a) The product of a collection of spaces 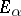 endowed with the projective topology with respect to the projections
endowed with the projective topology with respect to the projections 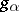 — hence the term "projective limit" .
— hence the term "projective limit" .
b) Let  be a vector space and let
be a vector space and let 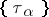 be a collection of topologies on
be a collection of topologies on 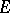 compatible with its vector-space structure. The space
compatible with its vector-space structure. The space 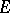 endowed with the supremum of the topologies
endowed with the supremum of the topologies 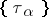 is the projective limit of the collection of topological vector spaces
is the projective limit of the collection of topological vector spaces 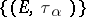 with respect to the collection of identity mappings
with respect to the collection of identity mappings 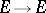 .
.
c) A topological vector subspace  of
of 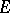 is the projective limit of the singleton collection
is the projective limit of the singleton collection 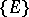 with respect to the inclusion mapping
with respect to the inclusion mapping 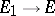 .
.
d) Any locally convex space is the projective limit of a collection of Banach spaces (cf. Banach space).
2) Inductive topologies. Let 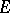 be a vector space, and for each
be a vector space, and for each  in some set
in some set 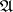 , let
, let  be a linear transformation from a topological vector space
be a linear transformation from a topological vector space 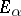 into
into 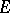 . Then in
. Then in 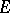 there exist: a) a strongest topology in which all
there exist: a) a strongest topology in which all 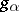 are continuous; b) a strongest topology compatible with the vector-space structure in which all these mappings are continuous; c) a strongest locally convex topology in which all
are continuous; b) a strongest topology compatible with the vector-space structure in which all these mappings are continuous; c) a strongest locally convex topology in which all 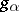 are continuous (even in the case that all
are continuous (even in the case that all 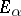 are locally convex spaces, these three topologies may be distinct). If all
are locally convex spaces, these three topologies may be distinct). If all 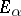 are locally convex spaces, then the space
are locally convex spaces, then the space 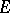 endowed with the topology defined in c) is called the inductive limit of the collection
endowed with the topology defined in c) is called the inductive limit of the collection 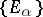 with respect to the mappings
with respect to the mappings 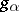 , and the topology is an inductive topology (of the same collection with respect to the same mappings). The term "inductive limit" is sometimes used in different senses. That introduced here is the widest which occurs in the literature. An inductive topology is a projective topology, being the supremum of a collection of topologies.
, and the topology is an inductive topology (of the same collection with respect to the same mappings). The term "inductive limit" is sometimes used in different senses. That introduced here is the widest which occurs in the literature. An inductive topology is a projective topology, being the supremum of a collection of topologies.
Examples of inductive limits: a) The locally convex direct sum of a collection 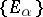 of locally convex spaces. This is the algebraic direct sum
of locally convex spaces. This is the algebraic direct sum 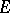 of the collection of vector spaces
of the collection of vector spaces 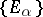 , endowed with the inductive topology of the collection of locally convex spaces
, endowed with the inductive topology of the collection of locally convex spaces 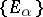 with respect to the collection of canonical inclusions
with respect to the collection of canonical inclusions 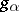 of
of 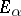 into
into 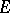 . b) Let
. b) Let 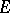 be a vector space and let
be a vector space and let 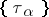 be a collection of locally convex topologies on
be a collection of locally convex topologies on  that are compatible with the vector-space structure. Let
that are compatible with the vector-space structure. Let  be its infimum in the class of all locally convex topologies. Then the locally convex space
be its infimum in the class of all locally convex topologies. Then the locally convex space 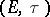 is the inductive limit of the collection of locally convex spaces
is the inductive limit of the collection of locally convex spaces 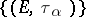 with respect to the collection of identity mappings on
with respect to the collection of identity mappings on 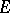 . c) Let
. c) Let 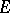 be a locally convex space and let
be a locally convex space and let 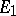 be a vector subspace. The topological vector quotient space
be a vector subspace. The topological vector quotient space  is the inductive limit of the singleton collection
is the inductive limit of the singleton collection 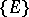 with respect to the canonical mapping
with respect to the canonical mapping 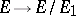 . d) A locally convex space is called bornological if any linear transformation from it into any Banach space which maps every bounded set to a bounded set, is continuous. A locally convex space is bornological if and only if it is the inductive limit of a collection of normable, locally convex spaces. e) Let
. d) A locally convex space is called bornological if any linear transformation from it into any Banach space which maps every bounded set to a bounded set, is continuous. A locally convex space is bornological if and only if it is the inductive limit of a collection of normable, locally convex spaces. e) Let  be a non-empty open subset of the space
be a non-empty open subset of the space 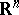 , and for every compactum
, and for every compactum 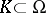 , let
, let 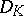 be the topological vector subspace of
be the topological vector subspace of 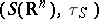 consisting of all functions in
consisting of all functions in 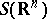 that vanish on
that vanish on 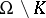 . Let
. Let 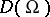 be the vector subspace
be the vector subspace 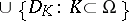 endowed with the inductive topology of the collection of locally convex spaces
endowed with the inductive topology of the collection of locally convex spaces 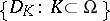 with respect to the canonical inclusions
with respect to the canonical inclusions 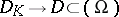 . The locally convex space
. The locally convex space 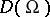 (which also plays an important part in the theory of generalized functions) is complete, Hausdorff and non-metrizable. It is a Montel space, hence paracompact, and so normal. The space
(which also plays an important part in the theory of generalized functions) is complete, Hausdorff and non-metrizable. It is a Montel space, hence paracompact, and so normal. The space 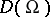 has a non-complete metrizable quotient space [11] which is a proper dense subspace of some
has a non-complete metrizable quotient space [11] which is a proper dense subspace of some 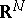 .
.
3) Spaces of mappings. Let  be a topological vector space,
be a topological vector space, 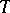 a set and
a set and 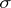 a set of subsets of
a set of subsets of 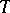 ordered by inclusion, so that the following property holds: for all
ordered by inclusion, so that the following property holds: for all  there exists a
there exists a 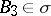 with
with  . Let
. Let 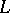 be some vector space of mappings from
be some vector space of mappings from 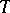 into
into 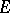 (with the natural algebraic operations), and let
(with the natural algebraic operations), and let 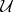 be a base of neighbourhoods of zero in
be a base of neighbourhoods of zero in  . For
. For  and
and 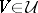 , let
, let  . Then the set
. Then the set 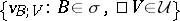 is a base of neighbourhoods of zero (the mapping
is a base of neighbourhoods of zero (the mapping 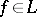 that maps the whole of
that maps the whole of 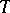 to the zero element of
to the zero element of 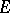 ) for a unique topology on
) for a unique topology on 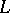 that is invariant under translations. This topology is called the topology of uniform convergence on sets in
that is invariant under translations. This topology is called the topology of uniform convergence on sets in 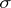 , or the
, or the 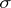 -topology for short. This topology is compatible with the vector-space structure on
-topology for short. This topology is compatible with the vector-space structure on 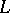 if and only if the set
if and only if the set 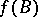 is bounded in
is bounded in 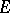 for all
for all 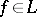 and all
and all 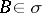 . This holds, e.g., if
. This holds, e.g., if 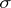 is the set of all finite subsets of
is the set of all finite subsets of  . In this case the
. In this case the 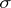 -topology on
-topology on 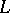 is called the topology of pointwise convergence. This topology is the projective topology (in
is called the topology of pointwise convergence. This topology is the projective topology (in 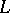 ) of a collection
) of a collection 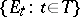 consisting of copies of the space
consisting of copies of the space 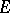 , with respect to the mappings
, with respect to the mappings 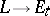 ,
, 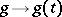 . The space
. The space 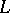 endowed with the
endowed with the  -topology will be denoted by
-topology will be denoted by 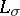 . If
. If 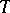 is a topological vector space and if all the elements of the space
is a topological vector space and if all the elements of the space 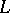 are continuous linear mappings and all the elements of
are continuous linear mappings and all the elements of 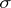 are bounded sets in
are bounded sets in 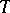 , then
, then 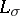 is also a topological vector space. If
is also a topological vector space. If 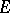 is a locally convex space, then so is
is a locally convex space, then so is 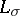 . The vector space of all continuous linear mappings from a topological vector space
. The vector space of all continuous linear mappings from a topological vector space 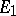 into
into  is denoted by
is denoted by 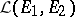 . In particular, suppose that
. In particular, suppose that 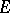 is a locally convex space. The (topologically) dual space to
is a locally convex space. The (topologically) dual space to 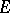 is the vector space
is the vector space  of all continuous linear functionals on
of all continuous linear functionals on  . Thus
. Thus 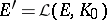 . Endowed with the topology of uniform convergence on the set
. Endowed with the topology of uniform convergence on the set  of all bounded subsets of
of all bounded subsets of  , it is called the strong dual (and its topology is called the strong topology), and is denoted by
, it is called the strong dual (and its topology is called the strong topology), and is denoted by  . The topology of pointwise convergence on
. The topology of pointwise convergence on  is also called the weak topology, or, more often, the weak-
is also called the weak topology, or, more often, the weak- topology. The generally accepted notation for the weak-
topology. The generally accepted notation for the weak- topology is
topology is 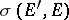 . It is known that the topological dual space of
. It is known that the topological dual space of  can be canonically identified with
can be canonically identified with 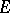 in the obvious manner
in the obvious manner 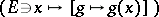 . Hence
. Hence 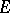 can be given the topology
can be given the topology  of pointwise convergence on
of pointwise convergence on 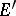 (called the weak topology).
(called the weak topology). 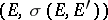 is often denoted by
is often denoted by  . Its dual is
. Its dual is  and, moreover,
and, moreover, 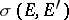 is the weakest locally convex topology
is the weakest locally convex topology  on
on 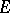 such that
such that 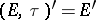 . There is also a strongest locally convex topology with this property, the so-called Mackey topology
. There is also a strongest locally convex topology with this property, the so-called Mackey topology 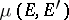 . The Mackey topology is the topology of uniform convergence on the absolutely convex
. The Mackey topology is the topology of uniform convergence on the absolutely convex 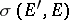 -compact subsets of
-compact subsets of 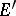 . When
. When 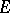 is a normed space,
is a normed space, 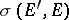 is called the weak
is called the weak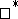 topology on
topology on 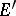 . The topology of any locally convex space can be considered as the topology of convergence on some set of subsets of the dual space.
. The topology of any locally convex space can be considered as the topology of convergence on some set of subsets of the dual space.
4) Tensor products. Let 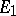 and
and 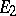 be locally convex spaces, let
be locally convex spaces, let  be their algebraic tensor product, and let
be their algebraic tensor product, and let 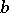 be the canonical bilinear mapping from the topological space
be the canonical bilinear mapping from the topological space  into
into 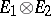 . The projective (respectively, inductive) topology on
. The projective (respectively, inductive) topology on 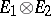 is the strongest of all locally convex topologies on
is the strongest of all locally convex topologies on 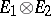 such that
such that 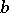 is continuous (respectively, separately continuous). Although this terminology is not completely consistent, it is generally accepted. The locally convex space obtained by putting the projective (respectively, injective) topology on the vector space
is continuous (respectively, separately continuous). Although this terminology is not completely consistent, it is generally accepted. The locally convex space obtained by putting the projective (respectively, injective) topology on the vector space 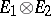 is denoted by
is denoted by  (
(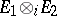 ) and its completion by
) and its completion by  (
( ). The spaces
). The spaces 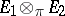 and
and 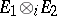 are called the locally convex tensor products of the corresponding locally convex spaces, and their completions are called the complete locally convex tensor products. There exist other locally convex tensor products besides those introduced here. These arise by introducing on the algebraic tensor product topologies other than those described above. Many of the properties of tensor products are simpler if one of the factors is a nuclear space.
are called the locally convex tensor products of the corresponding locally convex spaces, and their completions are called the complete locally convex tensor products. There exist other locally convex tensor products besides those introduced here. These arise by introducing on the algebraic tensor product topologies other than those described above. Many of the properties of tensor products are simpler if one of the factors is a nuclear space.
Examples. The locally convex spaces 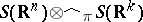 ,
, 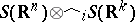 and
and  are canonically isomorphic (the isomorphism between the first two is a consequence of the fact that every separately-continuous bilinear mapping from a product of Fréchet spaces into an arbitrary locally convex space is continuous). The locally convex spaces
are canonically isomorphic (the isomorphism between the first two is a consequence of the fact that every separately-continuous bilinear mapping from a product of Fréchet spaces into an arbitrary locally convex space is continuous). The locally convex spaces 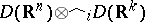 and
and 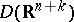 are also canonically isomorphic. The vector spaces
are also canonically isomorphic. The vector spaces  and
and  are canonically isomorphic, but their topologies do not coincide , [9].
are canonically isomorphic, but their topologies do not coincide , [9].
Duality.
An important part in the study of locally convex spaces is played by the relation between a locally convex space and its dual. In particular, certain properties of a locally convex space depend only on the size of the dual space. Thus, if  is a locally convex space and
is a locally convex space and 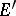 is its dual, then for all locally convex topologies on
is its dual, then for all locally convex topologies on 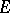 that are compatible with the duality between
that are compatible with the duality between 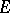 and
and 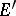 , the bounded sets are precisely the same, and the closed convex sets are also precisely the same.
, the bounded sets are precisely the same, and the closed convex sets are also precisely the same.
Duality theory turns out to be useful in the study of complete spaces. Thus, a locally convex space (respectively, metrizable locally convex space) 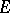 is complete if and only if every hyperplane (respectively, convex subset) of its dual
is complete if and only if every hyperplane (respectively, convex subset) of its dual 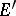 whose intersections with the polars of all neighbourhoods of zero in
whose intersections with the polars of all neighbourhoods of zero in 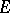 are all closed in the topology
are all closed in the topology  , is itself closed in that topology (Banach–Grothendieck and Krein–Shmul'yan theorems).
, is itself closed in that topology (Banach–Grothendieck and Krein–Shmul'yan theorems).
In this context one can make the following definition. A locally convex space is called 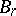 -complete (respectively,
-complete (respectively, 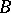 -complete or fully complete, hypercomplete, a Krein–Shmul'yan space) if any everywhere-dense linear subspace (respectively, linear subspace, absolutely-convex subset, convex subset) of
-complete or fully complete, hypercomplete, a Krein–Shmul'yan space) if any everywhere-dense linear subspace (respectively, linear subspace, absolutely-convex subset, convex subset) of 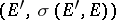 whose intersections with the polars of all neighbourhoods of zero in
whose intersections with the polars of all neighbourhoods of zero in  are closed, is itself closed. These classes of spaces play an important part in generalizations of Banach's closed-graph and open-mapping theorems (cf. below). Complete, metrizable, locally convex spaces, and strong duals of reflexive (cf. below), metrizable, locally convex spaces, belong to each of these classes. At the same time the spaces
are closed, is itself closed. These classes of spaces play an important part in generalizations of Banach's closed-graph and open-mapping theorems (cf. below). Complete, metrizable, locally convex spaces, and strong duals of reflexive (cf. below), metrizable, locally convex spaces, belong to each of these classes. At the same time the spaces 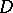 and
and  do not belong to any of them. The classes of hypercomplete spaces and Krein–Shmul'yan spaces do not coincide. However, it is not yet known (1992) whether or not the classes of
do not belong to any of them. The classes of hypercomplete spaces and Krein–Shmul'yan spaces do not coincide. However, it is not yet known (1992) whether or not the classes of 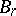 -complete spaces and hypercomplete spaces coincide.
-complete spaces and hypercomplete spaces coincide.
Using duality methods, one can also prove the following propositions about the compact subsets of locally convex spaces. 1) Let 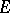 be a locally convex space and let
be a locally convex space and let 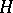 be a subset of
be a subset of 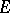 with a complete closed convex hull in the Mackey topology. If every sequence of elements of
with a complete closed convex hull in the Mackey topology. If every sequence of elements of 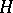 has a limit point in
has a limit point in  , then
, then 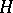 is relatively compact (Eberlein's theorem). 2) Let
is relatively compact (Eberlein's theorem). 2) Let 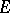 be a metrizable locally convex space and let
be a metrizable locally convex space and let 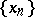 be a sequence in
be a sequence in  for which every subsequence has a limit point in
for which every subsequence has a limit point in 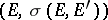 . Then one can extract a convergent subsequence from
. Then one can extract a convergent subsequence from 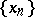 (Shmul'yan's theorem). 3) Let
(Shmul'yan's theorem). 3) Let  be a compact subset of a Hausdorff locally convex space
be a compact subset of a Hausdorff locally convex space 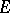 and let
and let 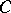 be the closed convex hull of
be the closed convex hull of 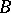 . Then
. Then 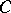 is compact if and only if it is complete in the Mackey topology (Krein's theorem).
is compact if and only if it is complete in the Mackey topology (Krein's theorem).
A locally convex space 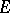 is called semi-reflexive (respectively, reflexive) if the canonical inclusion
is called semi-reflexive (respectively, reflexive) if the canonical inclusion 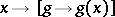 ,
,  , is an isomorphism of vector spaces (respectively, an isomorphism of topological vector spaces). A locally convex space
, is an isomorphism of vector spaces (respectively, an isomorphism of topological vector spaces). A locally convex space 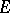 is semi-reflexive if and only if every bounded subset in it is relatively compact in the topology
is semi-reflexive if and only if every bounded subset in it is relatively compact in the topology  . It is reflexive if and only if it is a semi-reflexive barrelled space.
. It is reflexive if and only if it is a semi-reflexive barrelled space.
Mappings between topological vector spaces.
1) Closed-graph and open-mapping theorems. A linear mapping  from a topological vector space
from a topological vector space 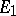 into a topological vector space
into a topological vector space 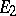 is called a topological homomorphism if it maps every open set in
is called a topological homomorphism if it maps every open set in 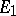 to an open set in
to an open set in  (in the topology induced from that of
(in the topology induced from that of 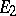 ). The graph of
). The graph of 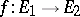 is the set
is the set 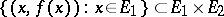 .
.
Let 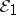 and
and 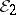 be two classes of topological vector spaces. One says that the closed-graph theorem (respectively, the homomorphism or open-mapping theorem) holds for the pair
be two classes of topological vector spaces. One says that the closed-graph theorem (respectively, the homomorphism or open-mapping theorem) holds for the pair  if for all
if for all 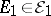 and
and  , every linear mapping
, every linear mapping 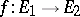 whose graph is closed in the topological vector space
whose graph is closed in the topological vector space 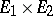 is continuous (respectively, if every surjective continuous linear mapping from
is continuous (respectively, if every surjective continuous linear mapping from 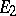 onto
onto 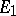 is a topological homomorphism). If
is a topological homomorphism). If 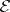 is the class of all complete, metrizable, topological vector spaces, then both the closed-graph theorem and the open-mapping theorem hold for
is the class of all complete, metrizable, topological vector spaces, then both the closed-graph theorem and the open-mapping theorem hold for 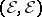 (Banach's theorem). This result can be strengthened: Let
(Banach's theorem). This result can be strengthened: Let 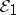 be the class of all Hausdorff locally convex spaces which are inductive limits of families of Banach spaces (such spaces are called ultrabornological), and let
be the class of all Hausdorff locally convex spaces which are inductive limits of families of Banach spaces (such spaces are called ultrabornological), and let 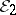 be the smallest class of locally convex spaces that contains all complete, metrizable, locally convex spaces and that is closed with respect to projective and inductive limits of countable collections of spaces in it. Then the closed-graph and open-mapping theorems hold for the pair
be the smallest class of locally convex spaces that contains all complete, metrizable, locally convex spaces and that is closed with respect to projective and inductive limits of countable collections of spaces in it. Then the closed-graph and open-mapping theorems hold for the pair 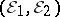 (Raikov's theorem). (All the complete locally convex spaces arising in functional analysis, with their usual topologies, belong to both of these classes.) In fact, the assertion formulated can be proved for a somewhat wider class
(Raikov's theorem). (All the complete locally convex spaces arising in functional analysis, with their usual topologies, belong to both of these classes.) In fact, the assertion formulated can be proved for a somewhat wider class 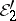 of topological vector spaces than
of topological vector spaces than 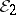 , and for multi-valued linear mappings. In [7] yet another class
, and for multi-valued linear mappings. In [7] yet another class 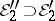 of topological vector spaces has been described that can play the part of
of topological vector spaces has been described that can play the part of  in the assertion — the so-called spaces with a web.
in the assertion — the so-called spaces with a web.
Let  ,
, 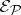 ,
, 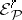 be, respectively, the class of all Hausdorff barrelled, totally complete, and
be, respectively, the class of all Hausdorff barrelled, totally complete, and 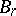 -complete, locally convex spaces. Then the closed-graph theorem holds for
-complete, locally convex spaces. Then the closed-graph theorem holds for 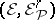 , and the open-mapping theorem holds for
, and the open-mapping theorem holds for  .
.
2) Fixed-point theorems. a) Let 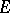 be a Hausdorff locally convex space, let
be a Hausdorff locally convex space, let 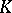 be a non-empty convex compact subset of it and let
be a non-empty convex compact subset of it and let  be a mapping from
be a mapping from 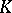 into the set of non-empty convex closed subsets of
into the set of non-empty convex closed subsets of 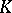 . Suppose that for each
. Suppose that for each 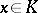 and each neighbourhood
and each neighbourhood  of the set
of the set 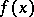 , there exists a neighbourhood
, there exists a neighbourhood 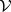 of
of  such that
such that  (this property of
(this property of 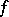 is called upper semicontinuity). Then there exists a point
is called upper semicontinuity). Then there exists a point 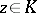 such that
such that 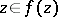 — a "fixed point" of
— a "fixed point" of 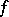 (Fan's theorem — a generalization of the Schauder–Tikhonov theorem). b) Let
(Fan's theorem — a generalization of the Schauder–Tikhonov theorem). b) Let 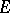 be a Hausdorff topological vector space, let
be a Hausdorff topological vector space, let 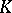 be a non-empty, compact, convex subset of it, and let
be a non-empty, compact, convex subset of it, and let 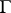 be a set of pairwise-commuting continuous mappings
be a set of pairwise-commuting continuous mappings 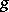 from
from 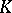 into
into  with the following property: If
with the following property: If 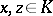 and
and 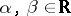 with
with 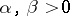 and
and 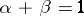 , then
, then 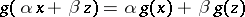 . Then there exists a point
. Then there exists a point 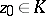 such that
such that 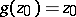 for all
for all 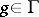 (the Markov–Kakutani theorem).
(the Markov–Kakutani theorem).
3) Also of great importance in the theory of locally convex spaces are the Hahn–Banach theorem and the Banach–Steinhaus theorem.
A number of interesting results in the theory of measures taking values in locally convex spaces and, (especially) in connection with the theory of random processes, in the theory of numerical cylindrical measures on locally convex spaces, have been obtained.
Mathematical analysis on topological vector spaces has arisen, and continues to develop — so-called infinite-dimensional analysis. Although a generalization of classical analysis, it also differs from it, both in terms of the problems and results which arise and in terms of the methods. Infinite-dimensional analysis includes the theory of differentiable mappings on topological vector spaces and differentiable measures on topological vector spaces; the theory of generalized functions and measures (distributions) on topological vector spaces; and the theory of differential equations — both with respect to functions of a real argument and taking values in a topological vector space, and with respect to numerical functions and measures (possibly generalized) defined on a topological vector space. The language of infinite-dimensional analysis is very natural for the formulation of fundamental problems in the physics of infinite-dimensional systems — quantum field theory, statistical mechanics and hydrodynamics — as well as of certain mathematical problems originating outside infinite-dimensional analysis.
References
| [1] | N. Bourbaki, "Elements of mathematics. Topological vector spaces" , Addison-Wesley (1977) (Translated from French) |
| [2] | A.P. Robertson, W.S. Robertson, "Topological vector spaces" , Cambridge Univ. Press (1964) |
| [3] | H.H. Schaefer, "Topological vector spaces" , Macmillan (1966) |
| [4] | R.E. Edwards, "Functional analysis: theory and applications" , Holt, Rinehart & Winston (1965) |
| [5] | A. Pietsch, "Nuclear locally convex spaces" , Springer (1972) (Translated from German) |
| [6] | A. Pietsch, "Operator ideals" , Deutsch. Verlag Wissenschaft. (1978) |
| [7] | M. de Wilde, "Closed graph theorems and webbed spaces" , Pitman (1978) |
| [8a] | L. Schwartz, "Théorie des distributions" , 1–2 , Hermann (1966) |
| [8b] | L. Schwartz, "Théorie des distributions à valeurs vectorielles I" Ann. Inst. Fourier (Grenoble) , 7 (1957) pp. 1–141 |
| [8c] | L. Schwartz, "Théorie des distributions à valeurs vectorielles II" Ann. Inst. Fourier (Grenoble) , 8 (1958) pp. 1–209 |
| [9] | E.T. Shavgulidze, "Conditions for certain forms of completeness in the class of projective limits of sequences of inductive limits of sequences of Fréchet spaces" Funct. Anal. Appl. , 11 : 1 (1972) pp. 82–83 Funkts. Anal. i Prilozhen. , 11 : 1 (1977) pp. 91–92 |
| [10] | O.G. Smolyanov, "The space 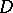 is not hereditarily complete" Math. USSR Izv. , 5 : 3 (1971) pp. 696710 Izv. Akad. Nauk SSSR, Ser. Mat. , 35 : 3 (1971) pp. 682–696 is not hereditarily complete" Math. USSR Izv. , 5 : 3 (1971) pp. 696710 Izv. Akad. Nauk SSSR, Ser. Mat. , 35 : 3 (1971) pp. 682–696 |
| [11] | O.G. Smolyanov, "Analysis on topological linear spaces and applications" , Moscow (1979) (In Russian) |
| [12] | H. Jarchow, "Locally convex spaces" , Teubner (1981) (Translated from German) |
| [13] | A. Grothendieck, "Produits tensoriels topologiques et espaces nucléaires" Mem. Amer. Math. Soc. , 16 (1955) pp. 1–140 |
| [14] | M. Valdivia, "Topics in locally convex spaces" , North-Holland (1982) |
| [15] | G. Köthe, "Topological vector spaces" , 1–2 , Springer (1969–1979) |
| [16] | P. Pérez Carrera, J. Bonet, "Barrelled locally convex spaces" , North-Holland (1987) |
| [17] | A. Fröhlicher, A. Kriegl, "Linear spaces and differentiation theory" , Wiley (1988) |
| [18] | O.G. Smolyanov, E.T. Shavgulidze, "Continual integrals" , Moscow (1990) (In Russian) |
Comments
For (aspects of) infinite-dimensional analysis see e.g. Differential equation, abstract; Qualitative theory of differential equations in Banach spaces; Vector measure; and [a3].
A locally convex topological vector space is quasi-complete if every bounded closed set is complete. Every quasi-complete topological vector space is sequentially complete (every Cauchy sequence has a limit). There are examples of sequentially-complete spaces that are not quasi-complete, and of quasi-complete spaces that are not complete [15]. There is a natural quasi-completion operation, [15], Vol. 1, p. 295.
References
| [a1] | J. Horvath, "Topological vector spaces and distributions" , 1 , Addison-Wesley (1968) |
| [a2] | J. Dugundji, A. Granas, "Fixed point theory" , I , PWN (1982) |
| [a3] | Yu.L. Daleckii, S.V. Fomin, "Measures and differential equations in infinite-dimensional spaces" , Kluwer (1992) (Translated from Russian) |
Topological vector space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Topological_vector_space&oldid=16374