Periodic semi-group
A semi-group in which each monogenic sub-semi-group (cf. Monogenic semi-group) is finite (in other words, each element has finite order). Every periodic semi-group has idempotents. The set  of all elements in a periodic semi-group some power (depending on the element) of which is equal to a given idempotent
of all elements in a periodic semi-group some power (depending on the element) of which is equal to a given idempotent  is called the torsion class corresponding to that idempotent. The set
is called the torsion class corresponding to that idempotent. The set  of all elements from
of all elements from 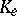 for which
for which  serves as the unit is an
serves as the unit is an  -class (see Green equivalence relations). It is the largest subgroup in
-class (see Green equivalence relations). It is the largest subgroup in  and an ideal in the sub-semi-group
and an ideal in the sub-semi-group  generated by
generated by  ; therefore,
; therefore, 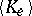 is a homogroup (see Minimal ideal). A periodic semi-group containing a unique idempotent is called unipotent. The unipotency of a periodic semi-group
is a homogroup (see Minimal ideal). A periodic semi-group containing a unique idempotent is called unipotent. The unipotency of a periodic semi-group  is equivalent to each of the following conditions:
is equivalent to each of the following conditions:  is an ideal extension of a group by a nil semi-group, or
is an ideal extension of a group by a nil semi-group, or  is a subdirect product of a group and a nil semi-group.
is a subdirect product of a group and a nil semi-group.
The decomposition of a periodic semi-group into torsion classes plays a decisive part in the study of many aspects of periodic semi-groups. An arbitrary torsion class is not necessarily a sub-semi-group: A minimal counterexample is the five-element Brandt semi-group 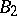 , which is isomorphic to a Rees semi-group of matrix type over the unit group having as unit the sandwich matrix of order two. In a periodic semi-group
, which is isomorphic to a Rees semi-group of matrix type over the unit group having as unit the sandwich matrix of order two. In a periodic semi-group  , all torsion classes are sub-semi-groups if and only if
, all torsion classes are sub-semi-groups if and only if  does not contain sub-semi-groups that are ideal extensions of a unipotent semi-group by
does not contain sub-semi-groups that are ideal extensions of a unipotent semi-group by  ; in this case, the decomposition of
; in this case, the decomposition of 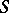 into torsion classes is not necessarily a band of semi-groups. Various conditions are known (including necessary and sufficient ones) under which a periodic semi-group is a band of torsion classes; this clearly occurs for commutative semi-groups, and it is true for periodic semi-groups having two idempotents [3].
into torsion classes is not necessarily a band of semi-groups. Various conditions are known (including necessary and sufficient ones) under which a periodic semi-group is a band of torsion classes; this clearly occurs for commutative semi-groups, and it is true for periodic semi-groups having two idempotents [3].
The Green relations  and
and  coincide in any periodic semi-group; a
coincide in any periodic semi-group; a  -simple periodic semi-group is completely
-simple periodic semi-group is completely  -simple. The following conditions are equivalent for a periodic semi-group
-simple. The following conditions are equivalent for a periodic semi-group  : 1)
: 1) 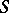 is an Archimedean semi-group; 2) all idempotents in
is an Archimedean semi-group; 2) all idempotents in  are pairwise incomparable with respect to the natural partial order (see Idempotent); and 3)
are pairwise incomparable with respect to the natural partial order (see Idempotent); and 3)  is an ideal extension of a completely-simple semi-group by a nil semi-group. Many conditions equivalent to the fact that a periodic semi-group
is an ideal extension of a completely-simple semi-group by a nil semi-group. Many conditions equivalent to the fact that a periodic semi-group  decomposes into a band (and then also into a semi-lattice) of Archimedean semi-groups are known; they include the following: a) for any
decomposes into a band (and then also into a semi-lattice) of Archimedean semi-groups are known; they include the following: a) for any  and for any idempotent
and for any idempotent  , if
, if 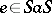 , then
, then  (cf. [5]); b) in
(cf. [5]); b) in  , each regular
, each regular  -class is a sub-semi-group; and c) each regular element of
-class is a sub-semi-group; and c) each regular element of 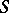 is a group element.
is a group element.
Let 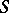 be an infinite periodic semi-group and let
be an infinite periodic semi-group and let  be the set of all its idempotents. If
be the set of all its idempotents. If  is finite,
is finite, 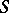 contains an infinite unipotent sub-semi-group, while if
contains an infinite unipotent sub-semi-group, while if  is infinite,
is infinite, 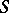 contains an infinite sub-semi-group that is a nilpotent semi-group or a semi-group of idempotents (cf. Idempotents, semi-group of) [4].
contains an infinite sub-semi-group that is a nilpotent semi-group or a semi-group of idempotents (cf. Idempotents, semi-group of) [4].
An important subclass of periodic semi-groups is constituted by the locally finite semi-groups (cf. Locally finite semi-group). A more extensive class is constituted by the quasi-periodic semi-groups ( is called quasi-periodic if some power of each of its elements lies in a subgroup
is called quasi-periodic if some power of each of its elements lies in a subgroup 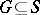 ). Many properties of periodic semi-groups can be transferred to quasi-periodic ones. Quasi-periodic groups are also called epigroups.
). Many properties of periodic semi-groups can be transferred to quasi-periodic ones. Quasi-periodic groups are also called epigroups.
References
| [1] | A.H. Clifford, G.B. Preston, "The algebraic theory of semi-groups" , 1 , Amer. Math. Soc. (1961) |
| [2] | E.S. Lyapin, "Semigroups" , Amer. Math. Soc. (1974) (Translated from Russian) |
| [3] | A.S. Prosvirov, "Periodic semigroups" Mat. Zap. Uralsk. Univ. , 8 : 1 (1971) pp. 77–94 (In Russian) |
| [4] | L.N. Shevrin, "On the theory of periodic semigroups" Soviet Math. Izv. Vyz. , 18 : 5 (1974) pp. 172–181 Izv. Vyzov. Mat. , 18 : 5 (1974) pp. 205–215 |
| [5] | M. Putcha, "Semilattice decompositions of semigroups" Semigroup Forum , 6 : 1 (1973) pp. 12–34 |
| [6] | S. Schwarz, "Contribution to the theory of torsion semigroups" Chekhoslov. Mat. Zh. , 3 (1953) pp. 7–21 (In Russian) (English abstract) |
Periodic semi-group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Periodic_semi-group&oldid=17355