Cardioid
From Encyclopedia of Mathematics
A plane algebraic curve of order four which is described by a point 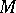 of a circle of radius
of a circle of radius  rolling on a circle with the same radius
rolling on a circle with the same radius  ; an epicycloid with modulus
; an epicycloid with modulus 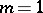 . The equation of the cardioid in polar coordinates is:
. The equation of the cardioid in polar coordinates is:
 |
In Cartesian coordinates it is:
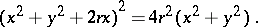 |
The arc length from the cusp is:
 |
The radius of curvature is:
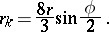 |
The area bounded by the curve equals 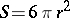 . The length of the curve is
. The length of the curve is 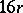 . The cardioid is a conchoid of the circle, a special case of a Pascal limaçon and a sinusoidal spiral.
. The cardioid is a conchoid of the circle, a special case of a Pascal limaçon and a sinusoidal spiral.

Figure: c020390a
References
| [1] | A.A. Savelov, "Planar curves" , Moscow (1960) (In Russian) |
Comments
References
| [a1] | J.D. Lawrence, "A catalog of special plane curves" , Dover, reprint (1972) |
How to Cite This Entry:
Cardioid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cardioid&oldid=13869
Cardioid. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cardioid&oldid=13869
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article