Existentially closed
existentially complete
Let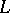 
be a first-order language (cf. Model (in logic)). A substructure 
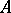of an 
-structure 
(cf. Structure) is called existentially closed (or existentially complete) in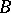 
if every existential sentence with parameters from 
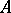is true in 
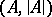if it is true in 
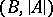. An existential sentence with parameters from 
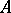is a closed formula 
, where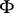 
is a formula without quantifiers in the first-order language of signature 
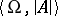, with 
the signature of 
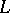(cf. Model theory).
If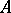 
is a substructure of 
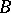and 
admits an embedding, fixing the elements of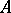 
, in some elementary extension of 
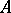(cf. Elementary theory), then 
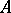is existentially closed in 
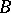. Conversely, if 
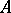is existentially closed in 
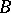and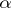 
is a cardinal number greater than the cardinality of 
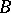, then 
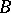admits an embedding, fixing the elements of 
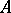, in every 
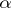-saturated extension of 
(cf. also Model theory).
A member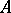 
of a class 
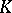of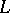 
-structures is called existentially closed (or existentially complete) with respect to 
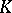if 
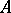is existentially closed in every member 
of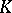 
, provided that 
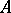is a substructure of 
.
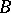If a field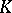 
is existentially closed in an extension field 
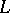, then 
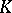is (relatively) algebraically closed in 
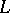(cf. Algebraically closed field). Hence, a field that is existentially closed with respect to all fields must be algebraically closed, and a formally real field that is existentially closed with respect to all formally real fields must be a real closed field. Existentially closed fields or rings (with respect to suitable classes) give rise to a corresponding Nullstellensatz. This is a theorem describing the form of a polynomial 
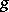depending on finitely many other polynomials 
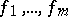, provided that there is an existentially closed member 
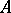of the class containing the coefficients of the polynomials and such that every common root of the 
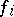in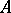 
is also a root of 
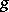. For the class of fields, the corresponding theorem is Hilbert's Nullstellensatz (cf. Hilbert theorem). There are corresponding theorems for formally real fields (see Real closed field), 
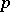-valued fields (see 
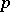-adically closed field), differential fields, division rings, commutative rings, and commutative regular rings. The general model-theoretic framework was considered by V. Weispfenning in 1977.
References
| [a1] | G. Cherlin, "Model theoretic algebra" , Lecture Notes in Mathematics , 521 , Springer (1976) |
Existentially closed. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Existentially_closed&oldid=35477