Interval and segment

The simplest sets of points on the line. An interval (open interval) is a set of points on a line lying between two fixed points and , where and themselves are considered not to belong to the interval. A segment (closed interval) is a set of points between two points and , where and are included. The terms "interval" and "segment" are also used for the corresponding sets of real numbers: an interval consists of numbers satisfying , while a segment consists of those for which . An interval is denoted by 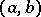, or , and a segment by .

The term "interval" is also used in a wider sense for arbitrary connected sets on the line. In this case one considers not only 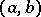but also the infinite, or improper, intervals , , 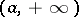, 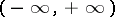the segment 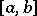, and the half-open intervals 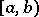, , 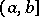, 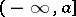. Here, a round bracket indicates that the appropriate end of the interval is not included, while a square bracket indicates that the end is included.

BSE-3

The notion of an interval in a partially ordered set is more general. An interval 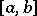consists in this setting of all elements of the partially ordered set that satisfy . An interval in a partially ordered set that consists of precisely two elements is called a simple or an elementary interval.

L.A. Skornyakov

Comments

In English the term "segment" is not often used, except in specifically geometrical contexts; the normal terms are "open interval" and "closed interval" , cf. also Interval, open; Interval, closed.