Elliptic genera

The name elliptic genus has been given to various multiplicative cobordism invariants taking values in a ring of modular forms. The following is an attempt to present the simplest case — level- genera in characteristic — in a unified way. It is convenient to use N. Katz's approach to modular forms (cf. [a7]) and view a modular form as a function of elliptic curves with a chosen invariant differential (cf. also Elliptic curve). A similar approach to elliptic genera was used by J. Franke [a3].

Jacobi functions.

Let be any perfect field of characteristic and fix an algebraic closure of (cf. Algebraically closed field). Consider a triple consisting of:

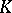i) an elliptic curve over , i.e. a smooth curve of genus with a specified -rational base-point ;

ii) an invariant 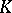-rational differential ;

iii) a -rational primitive -division point 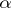. Following J.I. Igusa [a6] (up to a point), one can associate to these data two functions, and 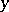, as follows.

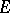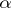The set of -division points on can be described as follows. There are four -division points ( is one of them), four primitive 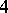-division points such that 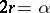, and eight primitive 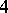-division points such that . Consider the degree- divisor 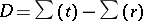. Since in and since Galois symmetries transform into itself, Abel's theorem (cf., for example, [a11], III.3.5.1, or Abel theorem) implies that there is a function , uniquely defined up to a multiplicative constant, such that .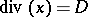

The function is odd, satisfies , and undergoes sign changes under the two other translations of exact order . Moreover, if 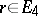satisfies 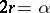, then translation by transforms into for some non-zero constant . This constant depends on the choice of but only up to sign. It follows that 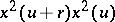does not depend on the choice of . This constant is written as , i.e.

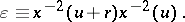One also defines

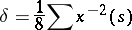(the summation is over the primitive 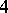-division points such that 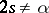). If is one of the values of , the other values are , each taken twice. It follows that

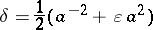and

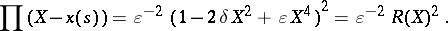It is now easy to see that

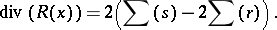Using once more Abel's theorem, one sees that there is a unique 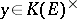such that , and 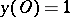. Since , one has .

The differential has four double poles . Also, it is easy to see that is a double zero of , hence a simple zero of . One concludes that

and that is an invariant differential on .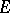

A slight modification of the argument given in [a6] shows that the Jacobi elliptic functions satisfy the Euler addition formula

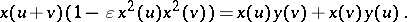Accordingly, one defines the Euler formal group law by

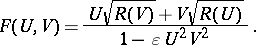Notice that since 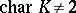, is defined over .

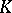The elliptic genus.

At this point, one normalizes over by requiring that (the given invariant differential). All the objects , and 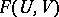are now completely determined by the initial data. Replacing by 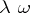(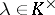) yields:

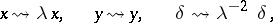(a1)

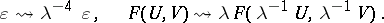As any formal group law, is classified by a unique ring homomorphism

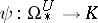from the complex cobordism ring. Since 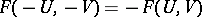, it is easy to see that uniquely factors through a ring homomorphism

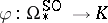from the oriented cobordism ring. By definition, 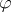is the level- elliptic genus. Suppose now that 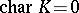. Define a local parameter near so that and . Then can be expanded into a formal power series 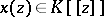which clearly satisfies 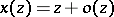and . In this case, the elliptic genus can be defined as the Hirzebruch genus (cf. [a4] or [a5]) corresponding to the series 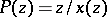. Since 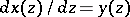, the logarithm 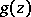of this elliptic genus is given by the elliptic integral

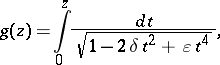(a2)

which gives the original definition in [a9].

Modularity.

For any closed oriented manifold of dimension , 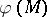is a function of the triple 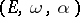. As easily follows from (a1), multiplying 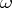by 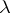results in multiplying by 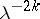. Also, depends only on the isomorphism class of the triple and commutes with arbitrary extensions of the scalar field 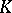. In the terminology of Katz ([a7]; adapted here to modular forms over fields), 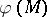is a modular form of level and weight . Let 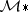be the graded ring of all such modular forms. Then , , 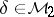. Moreover, one can prove that 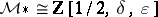. If one identifies these two isomorphic rings, the elliptic genus becomes the Hirzebruch genus

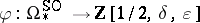with logarithm given by the formal integral (a2).

Integrality.

Consider

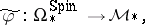i.e., the composition of with the forgetful homomorphism . As is shown in [a2],

The ring agrees with the ring 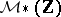of modular forms over 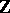. Thus: If 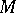is a 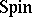-manifold of dimension 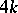, then .

Example: the Tate curve.

Let 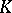be a local field, complete with respect to a discrete valuation , and let 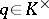be any element satisfying 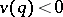. Consider 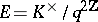. It is well-known (cf. [a11], § C.14) that can be identified with the elliptic curve (known as the Tate curve)

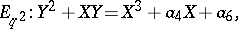where

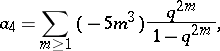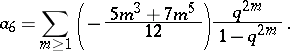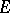can be treated as an elliptic curve over with 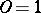. Fix the invariant differential () on ( 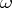corresponds to the differential 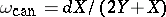on the Tate curve). 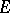has three -rational primitive -division points: , 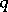and 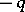. To describe the corresponding Jacobi function , consider the theta-function

This is a "holomorphic" function on with simple zeros at points of 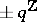(cf. [a10] for a justification of this terminology), satisfying

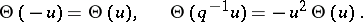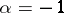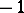Consider the case where . Let be any square root of , and let

(a3)

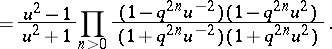is a meromorphic function on satisfying 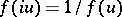and

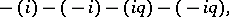i.e., 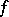is a multiple of the Jacobi function of .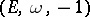

Notice now that the normalization condition 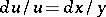can be written as , where is the derivative with respect to . Since , one has . Differentiating (a3), one obtains

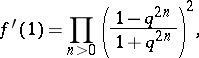and

Finally, if , the function satisfies . It follows that the generating series 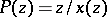is given by

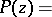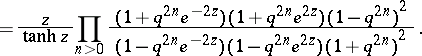The cases where or 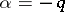are treated similarly, with

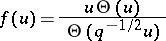and

respectively.

Strict multiplicativity.

The following theorem, also known (in an equivalent form) as the Witten conjecture, was proven first by C. Taubes [a12], then by R. Bott and Taubes [a1]. Let 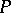be a principal 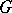-bundle (cf. also Principal 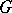-object) over an oriented manifold 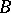, where 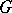is a compact connected Lie group, and suppose 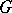acts on a compact -manifold 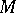. Then

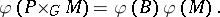For the history of this conjecture, cf. [a8].

References

[a1]	R. Bott, C. Taubes, "On the rigidity theorems of Witten" J. Amer. Math. Soc. , 2 (1989) pp. 137–186 MR0954493 Zbl 0667.57009
[a2]	D.V. Chudnovsky, G.V. Chudnovsky, P.S. Landweber, S. Ochanine, R.E. Stong, "Integrality and divisibility of the elliptic genus" Preprint (1988)
[a3]	J. Franke, "On the construction of elliptic cohomology" Math. Nachr. , 158 (1992) pp. 43–65 MR1235295 Zbl 0777.55003
[a4]	F. Hirzebruch, "Topological methods in algebraic geometry" , Grundlehren math. Wiss. , Springer (1966) (Edition: Third) MR0202713 Zbl 0138.42001
[a5]	F. Hirzebruch, Th. Berger, R. Jung, "Manifolds and modular forms" , Aspects of Mathematics , E20 , Vieweg (1992) (Appendices by Nils-Peter Skoruppa and by Paul Baum) MR1189136 Zbl 0752.57013 Zbl 0767.57014
[a6]	J.-I. Igusa, "On the transformation theory of elliptic functions" Amer. J. Math. , 81 (1959) pp. 436–452 MR0104668 Zbl 0131.28102
[a7]	N.M. Katz, "-adic properties of modular schemes and modular forms" W. Kuyk (ed.) J.-P. Serre (ed.) , Modular Functions in One Variable III. Proc. Internat. Summer School, Univ. of Antwerp, RUCA, July 17--August 3, 1972 , Lecture Notes in Mathematics , 350 (1973) pp. 69–190 MR0447119 Zbl 0271.10033
[a8]	P.S. Landweber, "Elliptic genera: An introductory overview" P.S. Landweber (ed.) , Elliptic Curves and Modular Forms in Algebraic Topology (Proc., Princeton 1986) , Lecture Notes in Mathematics , 1326 , Springer (1988) pp. 1–10 MR0970279 Zbl 0649.57021
[a9]	S. Ochanine, "Sur les genres multiplicatifs définis par des intégrales elliptiques" Topology , 26 (1987) pp. 143–151 MR0895567 Zbl 0626.57014
[a10]	P. Roquette, "Analytic theory of elliptic functions over local fields" , Hamburger Math. Einzelschrift. , 1 , Vandenhoeck and Ruprecht (1970) MR0260753 Zbl 0194.52002
[a11]	J.H. Silverman, "The arithmetic of elliptic curves" , GTM , 106 , Springer (1986) MR0817210 Zbl 0585.14026
[a12]	C. Taubes, "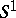 actions and elliptic genera" Comm. Math. Phys. , 122 (1989) pp. 455–526 MR0998662 Zbl 0683.58043