Packing

A packing of a (finite or infinite) family of sets $M_i$ in a set $A$ is, in its strict sense, any pairwise disjoint family of subsets $M_i\subset A$.

However, in geometry, the sets $M_i$ are often closed domains, and then this condition is relaxed to requiring only that the interiors of the sets are pairwise disjoint.

Lattice packings

As a special case, in vector spaces $V$, such as $\R^d$, packings of translates $ \{ M+v \mid v \in Z \} $, of a set $ M \subset V $ are considered. If the set $Z \subset V $ of translation vectors is a lattice, then the packing is called a lattice packing. In particular, such packings are investigated in the geometry of numbers and in discrete geometry.

Sphere packings

Packings of congruent spheres are considered both in the geometry of numbers and in discrete geometry, and have applications in coding theory. A central problem is finding the densest packing, and the densest lattice packing, of congruent spheres in $\R^d$. For $d=3$, the problem (known as Kepler conjecture or Kepler problem) to decide whether there is a better packing than the densest lattice packing was a famous open problem that was recently solved by Hales (1998). With the help of massive computer calculations he showed that the densest lattice packing of spheres is optimal. This result is generally considered as correct but because of its size it has not yet been verified independently. However, Hales and his team are working on a computer-verifyable version of the proof.

Tilings

A tiling is a packing without gaps, i.e., such that the $M_i$ are also a covering of $A$.

---

---

(text below not yet revised)

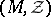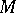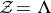in this case one speaks of the packing of the set by the system of vectors . If is a point lattice in , one speaks of a lattice packing .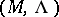

One also considers packing of sets 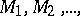not only in but also in other manifolds, on an -dimensional sphere, in a given domain, etc. (cf. [1], [2]).

Comments

Sphere packing has various applications in error-correcting codes (cf. Error-correcting code), the channel coding problem, Steiner systems (cf. Steiner system), -designs, and in the theory of finite groups. The most important special case is the sphere packing in 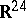via the Leech lattice. Finite and infinite sphere packing in 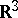has applications in classical and modern crystallography (cf. Crystallography, mathematical).

References

[1]	E.P. Baranovskii, "Packings, coverings, partitions, and certain other distributions in spaces of constant curvature" Progress in Math. , 9 (1971) pp. 209–253 Itogi Nauk. Algebra. Topol. Geom. 1967 (1969) pp. 181–225
[2]	L. Fejes Toth, "Lagerungen in der Ebene, auf der Kugel und im Raum" , Springer (1972)
[3]	C.A. Rogers, "Packing and covering" , Cambridge Univ. Press (1964)
[a1]	P. Erdös, P.M. Gruber, J. Hammer, "Lattice points" , Longman (1989)
[a2]	P.M. Gruber, C.G. Lekkerkerker, "Geometry of numbers" , North-Holland (1987) pp. Sect. (iv) (Updated reprint)
[a3]	B. Grünbaum, G.C. Shephard, "Tilings and patterns" , Freeman (1986)
[a4]	J.H. Conway, N.J.A. Sloane, "Sphere packing, lattices and groups" , Springer (1988)

2020 Mathematics Subject Classification: Primary: 52C20,52C22 [MSN][ZBL]