Booth lemniscate

A plane algebraic curve of order four whose equation in orthogonal Cartesian coordinates is

$$ (x ^ {2} + y ^ {2} ) ^ {2} - (2m ^ {2} + n) x ^ {2} - (2m ^ {2} - n) y ^ {2} = 0. $$

If $ | n | < 2 m ^ {2} $, the Booth lemniscate is called elliptic (it has singular point $ O $( Fig. a), where $ 0 < n < 2 m ^ {2} $). If $ | n | > 2 m ^ {2} $, the Booth lemniscate is called hyperbolic (it has a nodal point at the coordinate origin, cf. Fig. b, where $ n > 2 m ^ {2} $).

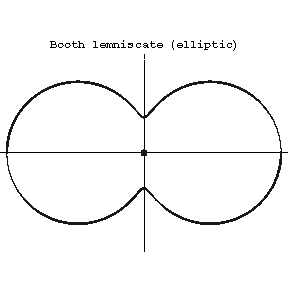
Figure: b017000a

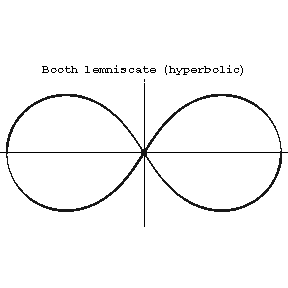
Figure: b017000b

The equation of an elliptic Booth lemniscate in polar coordinates is

$$ \rho ^ {2} = a ^ {2} \cos ^ {2} \phi + b ^ {2} \sin ^ {2} \phi \ \ \textrm{ or } \ \rho \equiv 0. $$

If $ n > 2 m ^ {2} $, the equation of a hyperbolic Booth lemniscate has the form

$$ \rho ^ {2} = \ a ^ {2} \cos ^ {2} \phi - b ^ {2} \sin ^ {2} \phi ; $$

and if $ n < -2 m ^ {2} $

$$ \rho ^ {2} = \ - a ^ {2} \cos ^ {2} \phi + b ^ {2} \sin ^ {2} \phi $$

$$ (a ^ {2} = | 2m ^ {2} + n | ,\ b ^ {2} = | 2m ^ {2} - n | ). $$

The arc length of a Booth lemniscate is expressed by elliptic integrals. The area bounded by an elliptic Booth lemniscate is

$$ S = { \frac \pi {2} } (a ^ {2} + b ^ {2} ), $$

while that bounded by a hyperbolic Booth lemniscate is

$$ S = \frac{a ^ {2} - b ^ {2} }{2} \ \mathop{\rm arctg} { \frac{a}{b} } + { \frac{ab}{2} } . $$

The Booth lemniscate is a special case of a Persian curve.

Named after J. Booth [1].

References

[1]	J. Booth, "A treatise on some new geometrical methods" , 1–2 , London pp. 1873–1877
[2]	A.A. Savelov, "Planar curves" , Moscow (1960) pp. 144–146 (In Russian)