Approximation of functions of a complex variable

The branch of complex analysis studying problems regarding the approximate representation (approximation) of functions of a complex variable by means of analytic functions of a specific class. The fundamental problems in the theory of approximation of functions of a complex variable are: the possibility of approximating; the rate of approximation; and the approximation properties of various methods of representing functions (interpolation sequences and series, series in orthogonal polynomials or Faber polynomials, expansion in continued fractions and Padé approximation, sequences of polynomials in exponential functions, Dirichlet series, etc.). The theory of approximation of functions of a complex variable is intimately connected with other branches of complex analysis, and with mathematics in general. Methods and results on conformal mapping, integral representation, potential theory, the theory of function algebras, etc., play an important role in approximation theory.

The central problems in the theory of approximation of functions of a complex variable relate to approximation by polynomials or rational functions, in particular by polynomials and rational functions of best approximation (existence, characteristic properties, uniqueness), as well as to extremal problems and various estimates for polynomials and rational functions (growth estimates, inequalities for derivatives, polynomials and rational functions, least deviation from zero, etc.).

A.A. Gonchar

The approximation of functions of a complex variable by polynomials and rational functions.

In this branch of approximation theory one may distinguish several directions.

1) The study of the possibility of approximating a function 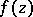of a complex variable with given accuracy by polynomials or rational functions in , in dependence on the properties of the set on which 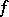is given and on which 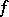is to be approximated, on the properties of the metric 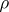of deviation and on the properties of 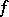itself.

2) The study of the properties of polynomials and rational functions of best approximation, i.e. polynomials 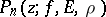and rational functions 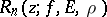of degree not exceeding , for which

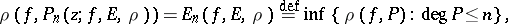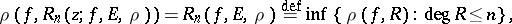where the infima are over the set of polynomials 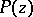of degree 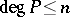or rational functions 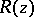of degree 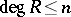, respectively (or over parts of these sets, distinguished by addition requirements). In fact, one deals here with properties of solutions for a certain class of extremal problems. In this context one may also consider the study of other extremal problems on sets of polynomials, rational functions and on certain classes of analytic functions, as well as the study of analytic properties of polynomials and rational functions (in particular, obtaining inequalities between various norms of these functions and their derivatives).

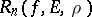3) The study of the dependence of the rate of decrease (to zero) of and , as 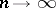, on the properties of 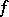, 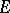and 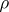(the so-called direct theorems of approximation theory) and the dependence of the properties of on the rate of decrease of and 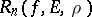to zero as 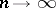and on the properties of 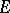and 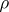(inverse theorems). The study of approximation properties of well-known methods in approximation theory (e.g., series in Faber polynomials, various interpolation processes (cf. Interpolation process)), as well as the search for new effective approximation methods are related to this direction.

4) The approximation of functions of several complex variables. Here, basically, the same problems are solved as in the case of one complex variable, but the results and the methods for obtaining them differ, as a rule, sharply from those used in the case of one variable.

In the following some basic results have been listed.

1) The problem of the existence of a uniform approximation by polynomials that is as good as one pleases is solved by the Runge theorem (if 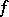is analytic on 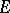), the Lavrent'ev theorem (if 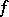is continuous on ), the Keldysh theorem (if 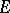is a closed domain, 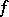is continuous on 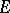and analytic within 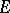) and the Mergelyan theorem (in the general case: 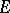is a compact set, 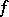is continuous on 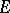and analytic at interior points of ).

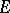2) The problem whether it is possible to approximate holomorphic functions on closed sets 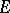in the extended complex plane 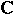is solved by Runge's theorem. In the study of approximating a function 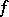in various spaces, using the metric of these spaces, by rational functions, an important role is played by characteristics of the set 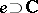analogous to the analytic capacity 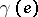. In terms of 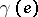the problem of the description of all compact sets 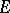on which any continuous function can be approximated with arbitrary accuracy by rational functions is solved as follows: It is necessary and sufficient that either

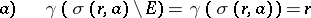for any disc 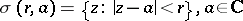, 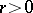; or that

for any 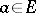(the equivalence of a) and b) expresses the so-called "instability" of the analytic capacity).

3) If 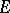is bounded and Lebesgue-measurable and if 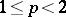, then the set of all rational functions is dense in .

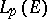4) If 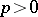and if 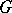is a simply-connected domain with a rectifiable Jordan boundary, then the family of all polynomials in is dense in the Smirnov class 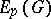if and only if 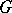is a Smirnov domain.

5) Let the complex-valued functions 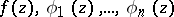, , 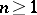be continuous on a compact set 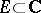. Among all generalized polynomials

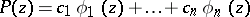( are arbitrary complex numbers) a generalized polynomial 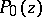deviates least from 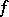in the metric

if and only if

for each .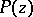

6) If 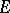is a compact set with connected complement 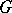and if 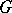has a Green function (for the first boundary value problem for the Laplace equation) 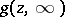with a pole at 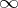, then for each 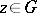and each polynomial 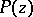of degree , the inequality

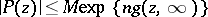with 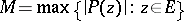holds.

7) If 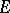is a bounded non-degenerate continuum with connected complement 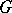and if 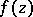is analytic at interior points of 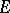and continuous on 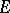with modulus of continuity 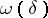, then

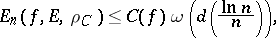where

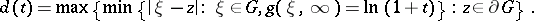If the closed domain 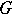is bounded by an analytic curve 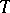, then the condition

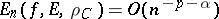is equivalent to the condition that 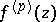is Hölder continuous of order , , 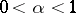in . The case when 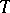is a piecewise-smooth curve with corners has been studied.

8) In a number of cases for the approximation of analytic functions various interpolation processes prove effective, including Padé approximation and its generalizations.

9) For , in 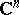there exist both non-closed Jordan curves on which not every continuous function can be uniformly approximated by polynomials in to any degree of accuracy, and closed Jordan curves on which polynomials uniformly approximate any continuous function. In 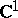this is impossible.

10) Up till now (1983) there are comparatively few direct theorems on the approximation by rational functions with free poles (i.e. without any condition on the position of the poles of the approximating functions) and a considerable amount of inverse theorems.

References

[1]	V.L. Goncharov, "The theory of interpolation and approximation of functions" , Moscow (1954) (In Russian)
[2]	J.L. Walsh, "Interpolation and approximation by rational functions in the complex domain" , Amer. Math. Soc. (1969)
[3]	V.I. Smirnov, A.N. Lebedev, "Functions of a complex variable: constructive theory" , M.I.T. (1968) (Translated from Russian)
[4]	V.K. Dzyadyk, "Introduction to the theory of uniform approximation of functions by polynomials" , Moscow (1977) (In Russian)
[5]	V.N. Rusak, "Rational functions as approximating tool" , Minsk (1979) (In Russian)
[6]	T.W. Gamelin, "Uniform algebras" , Prentice-Hall (1969)
[7]	A.F. Leont'ev, "Exponential series" , Moscow (1976) (In Russian)
[8]	, Some questions in approximation theory , Moscow (1963) (In Russian; translated from English)
[9]	M.V. Keldysh, Dokl. Akad. Nauk SSSR , 4 (1936) pp. 163–166
[10]	M. [M.A. Lavrent'ev] Lafrientieff, "Zur Theorie der konformen Abbildung" Trudy Mat. Inst. Steklov. , 5 (1934) pp. 159–245
[11]	A.N. Kolmogorov, "Remark on the polynomials of P.L. Chebyshev deviating least from a given function" Uspekhi Mat. Nauk , 3 : 1 (1948) pp. 216–221 (In Russian)
[12]	S.N. Mergelyan, "Uniform approximation to functions of a complex variable" Series and approximation , 3 , Amer. Math. Soc. (1962) pp. 294–391 Uspekhi Mat. Nauk , 7 : 2 (1952) pp. 31–122
[13]	A.G. Vitushkin, "The analytic capacity of sets in problems of approximation theory" Russian Math. Surveys , 22 (1967) pp. 167–200 Uspekhi Mat. Nauk , 22 : 6 (1967) pp. 141–199
[14]	M.M. Dzhrbasyan, "Some questions of the theory of weighted polynomials in a complex domain" Mat. Sb. , 36 (1955) pp. 353–440 (In Russian)
[15]	A.A. Gonchar, "The rate of approximation of functions by rational numbers and properties of numbers" , Proc. Internat. Congress Mathematicians (Moscow, 1966) , Mir (1968) pp. 329–356 (In Russian)
[16]	E.P. Dolzhenko, P.L. Ul'yanov, Vestn. Moskov. Univ. Ser. Mat. Mekh. , 1 (1980) pp. 3–13
[17]	S.N. Mergelyan, "On the approximation of functions of a complex variable" , Mathematics in the USSR during 40 years: 1917–1957 , 1 , Moscow (1959) pp. 383–398 (In Russian)
[18]	A.A. Gonchar, S.N. Mergelyan, , History of paternal mathematics , 1 , Kiev (1970) pp. 112–193 (In Russian)
[19]	P.M. Tamrazov, "Smoothness and polynomial approximation" , Kiev (1975) (In Russian)
[20]	M.S. Mel'nikov, S.O. Sinanyan, , Contemporary problems in mathematics , 4 , Moscow (1975) pp. 143–250 (In Russian)

E.P. Dolzhenko

Comments

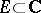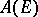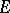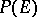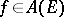Let be a compact set. Let denote the set of functions that are continuous on and analytic at interior points (if any) of . Denote by (respectively, ) the set of functions that can be uniformly approximated on 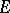by polynomials (respectively, rational functions). Mergelyan's theorem states: 1) 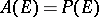if and only if , the complement of 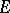, is connected; 2) 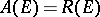if is finitely-connected. Examples of compact sets 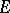for which 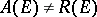are known (e.g. the Schweizer Käse of A. Roth, cf. [a1]). The problem arises of characterizing those 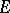for which 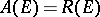. The solution was given by E. Bishop and A.G. Vitushkin (independently) for compact sets without interior points, and by Vitushkin for general compact 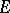. Vitushkin's theorem can be found in [a1].

The sets 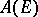, 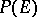and 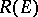are uniform algebras, e.g. function algebras endowed with the sup-norm (cf. Algebra of functions; Uniform algebra). For studies stressing this aspect see [6] and [a4].

Another direction of research is to study not approximation by linear combinations of 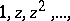or 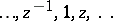(polynomials or rational functions) but by linear combinations of powers 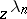, or of exponentials 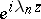, where 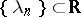, on sets 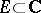, mostly on curves satisfying some "oscillation condition" . Results of this type related to the Müntz theorem; Lacunary power series; the Paley–Wiener theorem, zero sets of analytic functions, etc. (cf. [a5]).

In 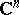approximation problems depend essentially on the geometry of the domain under consideration (this is related to the so-called Levi problem of characterizing domains of holomorphy). One way to approach approximation problems is via Hörmander's 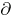mechanism (cf. [a6]). An example of an approximation theorem is Kerzman's theorem: Let be a strongly pseudo-convex domain with sufficiently smooth boundary ( suffices). Then every function 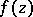holomorphic on and continuous on 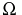can be uniformly approximated on 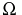by functions 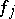that are holomorphic on some (strongly pseudo-convex) domain 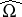containing , [a7].

References

[a1]	D. Gaier, "Vorlesungen über Approximation im Komplexen" , Birkhäuser (1980)
[a2]	R.C. Buck, "Survey of recent Russian literature on approximation" R.E. Langer (ed.) , On numerical approximation , Univ. of Wisconsin Press (1959) pp. 341–359
[a3]	J. Korevaar, "Polynomial and rational approximation in the complex domain" J.G. Clunie (ed.) , Aspects of contemporary complex analysis , Acad. Press (1980) pp. 251–292
[a4]	E.L. Stout, "The theory of uniform algebras" , Bogden & Quigley (1971)
[a5]	R.M. Redheffer, "Completeness of sets of complex exponentials" Adv. in Math. , 24 (1977) pp. 1–62
[a6]	L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973) pp. Sect. 4.5
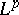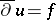[a7]	N. Kerzman, "Hölder and estimates for solutions of on strongly pseudo-convex domains" Commun. Pure Appl. Math. , 24 (1971) pp. 301–380