Cyclic vector

Let be an endomorphism of a finite-dimensional vector space . A cyclic vector for is a vector such that form a basis for , i.e. such that the pair is completely reachable (see also Pole assignment problem; Majorization ordering; System of subvarieties; Frobenius matrix).

A vector in an (infinite-dimensional) Banach space or Hilbert space with an operator on it is said to be cyclic if the linear combinations of the vectors 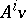, , 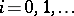form a dense subspace, [a1].

More generally, let 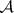be a subalgebra of 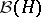, the algebra of bounded operators on a Hilbert space 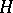. Then 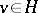is cyclic if 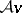is dense in 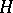, [a2], [a5].

If 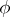is a unitary representation of a (locally compact) group 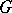in , then is called cyclic if the linear combinations of the , , 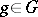form a dense set, [a3], [a4]. For the connection between positive-definite functions on and the cyclic representations (i.e., representations that admit a cyclic vector), see Positive-definite function on a group. An irreducible representation is cyclic with respect to every non-zero vector.

References

[a1]	M. Reed, B. Simon, "Methods of mathematical physics: Functional analysis" , 1 , Acad. Press (1972) pp. 226ff
[a2]	R.V. Kadison, J.R. Ringrose, "Fundamentals of the theory of operator algebras" , 1 , Acad. Press (1983) pp. 276
[a3]	S.A. Gaal, "Linear analysis and representation theory" , Springer (1973) pp. 156
[a4]	A.A. Kirillov, "Elements of the theory of representations" , Springer (1976) pp. 53 (In Russian)
[a5]	M.A. Naimark, "Normed rings" , Noordhoff (1964) pp. 239 (In Russian)