Erdös-Ginzburg-Ziv theorem
EGZ theorem
If $m$ is a positive integer and $a_1,\ldots,a_{2m-1}$ is a sequence of elements from the cyclic group $\mathbb{Z}_m$, then there exists a set $I\subseteq \{1,\ldots,2m-1\}$ of cardinality $m$ such that $\sum_{i\in I}a_i=0$. This theorem was first shown in [a5].
Related theorems.
Looking at a sequence of 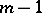 zeros and
zeros and  ones one sees that
ones one sees that 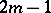 cannot be replaced by a smaller number. This motivates the definition of the Erdös–Ginzburg–Ziv constant for an arbitrary Abelian group, as follows. If
cannot be replaced by a smaller number. This motivates the definition of the Erdös–Ginzburg–Ziv constant for an arbitrary Abelian group, as follows. If 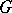 is an Abelian group, then
is an Abelian group, then 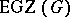 is the minimum integer
is the minimum integer  such that every sequence
such that every sequence  of elements from
of elements from 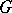 contains a subsequence of cardinality
contains a subsequence of cardinality 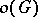 , the order of
, the order of 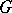 , that adds up to
, that adds up to  . It can be proven that
. It can be proven that 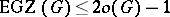 and equality holds if and only if
and equality holds if and only if 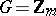 . This result and the observation above led to the following two directions of investigation:
. This result and the observation above led to the following two directions of investigation:
i) To find bounds, or possibly determine, 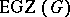 for groups other than
for groups other than 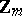 in terms of
in terms of 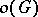 and other group invariants. Recent results in this direction were obtained by Y. Caro and Weidong Gao.
and other group invariants. Recent results in this direction were obtained by Y. Caro and Weidong Gao.
ii) To find or estimate the minimum integer 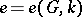 such that every sequence
such that every sequence  of elements from
of elements from 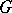 contains a subsequence of cardinality
contains a subsequence of cardinality 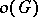 that adds up to
that adds up to  , provided one knows that there are at least
, provided one knows that there are at least  distinct elements in the sequence. Recent results in this direction are due to A. Bialostocki, P. Dierker, Y.O. Hamidoune, and M. Lotspeich. A breakthrough in this direction was achieved after the recent proof of the long standing Erdös–Heilbronn conjecture (cf. also Erdös–Heilbronn problem). Along a different line, J.E. Olson extended the definition of the
distinct elements in the sequence. Recent results in this direction are due to A. Bialostocki, P. Dierker, Y.O. Hamidoune, and M. Lotspeich. A breakthrough in this direction was achieved after the recent proof of the long standing Erdös–Heilbronn conjecture (cf. also Erdös–Heilbronn problem). Along a different line, J.E. Olson extended the definition of the 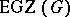 constant to non-Abelian groups and proved that
constant to non-Abelian groups and proved that  still holds.
still holds.
Outline of developments.
There are several reasons why this theorem has recently (1996) drawn much attention.
Quite unexpectedly, N. Alon and M. Dubiner [a1] have shown that the theorem follows from several deeper results in algebra and number theory, establishing interesting links.
The theorem has many possible generalizations, some of which have been proved and others are easy to state as fundamental open problems, see [a4] and the conjectures below.
The theorem motivated the development of what is called a zero-sum Ramsey theory: If the sequence in the theorem consists only of the elements  and
and 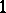 , then its proof follows from the pigeon hole principle (cf. Dirichlet principle), hence the theorem can be viewed as a generalization of this principle. Consequently, since Ramsey theory (cf. also Ramsey theorem) is a development of the pigeon hole principle, there is a clear motivation to develop from the EGZ theorem a zero-sum Ramsey theory along the lines of traditional Ramsey theory. While in Ramsey theory one looks for monochromatic configurations, in zero-sum Ramsey theory the colours are elements of a group and one looks for zero-sum configurations. Zero-sum Ramsey theory generalizes many results of the traditional Ramsey theory and leaves many open problems.
, then its proof follows from the pigeon hole principle (cf. Dirichlet principle), hence the theorem can be viewed as a generalization of this principle. Consequently, since Ramsey theory (cf. also Ramsey theorem) is a development of the pigeon hole principle, there is a clear motivation to develop from the EGZ theorem a zero-sum Ramsey theory along the lines of traditional Ramsey theory. While in Ramsey theory one looks for monochromatic configurations, in zero-sum Ramsey theory the colours are elements of a group and one looks for zero-sum configurations. Zero-sum Ramsey theory generalizes many results of the traditional Ramsey theory and leaves many open problems.
Proofs.
In [a1] five proofs for the EGZ theorem have been given. In all these proofs it is assumed that 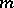 is a prime number, since the transition to a non-prime is a simple induction. The original proof is based on the Cauchy–Davenport theorem from elementary additive number theory; two other proofs use the Fermat little theorem, along with a counting argument and a lemma concerning permanents, respectively. A fourth proof uses the Chevalley–Warning theorem about zeros of polynomials over a finite field. Most interesting is the proof that uses knowledge of the Davenport constant of an Abelian
is a prime number, since the transition to a non-prime is a simple induction. The original proof is based on the Cauchy–Davenport theorem from elementary additive number theory; two other proofs use the Fermat little theorem, along with a counting argument and a lemma concerning permanents, respectively. A fourth proof uses the Chevalley–Warning theorem about zeros of polynomials over a finite field. Most interesting is the proof that uses knowledge of the Davenport constant of an Abelian 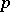 -group, determined by Olson. Let
-group, determined by Olson. Let 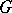 be a finite Abelian group. The Davenport constant of
be a finite Abelian group. The Davenport constant of  , denoted by
, denoted by 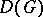 , is the minimal
, is the minimal 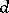 such that every sequence of
such that every sequence of 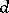 elements from
elements from 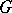 contains a subsequence that adds up to
contains a subsequence that adds up to  .
.
Generalizations and analogues.
Clearly, if  is cyclic of order
is cyclic of order 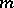 , then
, then 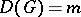 . An interesting relation between
. An interesting relation between 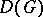 and the EGZ theorem is Weidong's generalization of the EGZ theorem: Let
and the EGZ theorem is Weidong's generalization of the EGZ theorem: Let  be a sequence of elements from an Abelian group
be a sequence of elements from an Abelian group 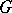 . If
. If 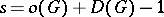 , then there exists a set
, then there exists a set 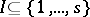 of cardinality
of cardinality 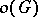 such that
such that 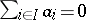 .
.
The following two conjectures are other possible generalizations of the EGZ theorem.
Conjecture 1.
Let 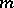 and
and  be positive integers. If
be positive integers. If  is a sequence of elements from the cyclic group
is a sequence of elements from the cyclic group 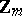 , then it contains at least
, then it contains at least 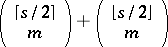 subsequences of
subsequences of 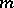 elements that add up to
elements that add up to  .
.
If one takes 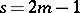 in this conjecture, then the EGZ theorem follows.
in this conjecture, then the EGZ theorem follows.
Conjecture 2.
Let 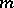 be a positive integer and let
be a positive integer and let 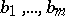 be a sequence of elements from the cyclic group
be a sequence of elements from the cyclic group 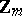 whose sum is
whose sum is  . If
. If 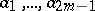 is a sequence of elements from
is a sequence of elements from 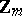 , then it contains a subsequence
, then it contains a subsequence 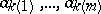 such that the sequence
such that the sequence 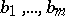 can be reordered
can be reordered 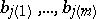 such that
such that 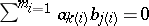 .
.
If one takes 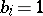 ,
, 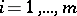 , in this conjecture, then the EGZ theorem follows.
, in this conjecture, then the EGZ theorem follows.
Conjecture 1 was proven by M. Kisin for 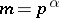 and
and 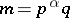 , where
, where 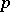 and
and 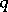 are distinct prime numbers and
are distinct prime numbers and 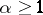 . In [a7], Z. Füredi and D.J. Kleitman confirmed Conjecture 1 asymptotically for every positive integer
. In [a7], Z. Füredi and D.J. Kleitman confirmed Conjecture 1 asymptotically for every positive integer 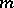 . Conjecture 2 can be easily proven for
. Conjecture 2 can be easily proven for 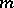 prime. Both conjectures illustrate the general difficulty that exists in this area for handling the non-prime case.
prime. Both conjectures illustrate the general difficulty that exists in this area for handling the non-prime case.
There are many related problems to the EGZ theorem. The following conjecture was raised by H. Harborth and some progress was made by Alon and Dubiner. It illustrates that certain problems are open, even for primes.
Conjecture 3.
If  is a prime and
is a prime and 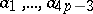 is a sequence of elements from the group
is a sequence of elements from the group 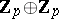 , then there exists a subsequence of
, then there exists a subsequence of 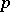 elements whose elements add up to
elements whose elements add up to 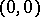 .
.
A sequence containing only the elements 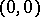 ,
,  ,
, 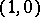 , and
, and 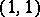 , where each element appears
, where each element appears 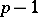 times, implies that
times, implies that 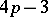 can not be replaced by a smaller number.
can not be replaced by a smaller number.
Zero-sum Ramsey theory.
This theory was first introduced in [a3]. Today it includes many results on zero-sum Ramsey numbers for graphs. Recent developments by Bialostocki, P. Erdös, H. Lefmann, and D. Schaal deal with zero-sum solutions to systems of equations and inequalities over the integers. Surveys of this can be found in [a2] and [a4]. The following theorem from zero-sum Ramsey theory, proved in [a6] and [a8], generalizes in the sense of the EGZ theorem the folkloristic fact that in every  -colouring of the edges of a complete graph there is always a monochromatic spanning tree: If each edge
-colouring of the edges of a complete graph there is always a monochromatic spanning tree: If each edge  of the complete graph
of the complete graph  (cf. also Graph theory) is assigned an element from the cyclic group
(cf. also Graph theory) is assigned an element from the cyclic group 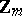 , say
, say 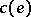 , then there exists a spanning tree
, then there exists a spanning tree 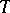 of
of 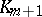 with edges
with edges 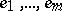 such that
such that 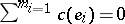 .
.
References
| [a1] | N. Alon, M. Dubiner, "Zero-sum sets of prescribed size" D. Miklós (ed.) V.T. Sós (ed.) T. Szönyi (ed.) , Combinatorics, Paul Erdös is Eighty , Bolyai Society Mathematical Studies , 1 , Keszthely (Hungary) (1993) pp. 33–50 |
| [a2] | A. Bialostocki, "Zero-sum trees: a survey of results and open problems" N.W. Sauer (ed.) R.E. Woodrow (ed.) B. Sands (ed.) , Finite and Infinite Combinatorics in Sets and Logic , Nato ASI Ser. , Kluwer Acad. Publ. (1993) pp. 19–29 |
| [a3] | A. Bialostocki, P. Dierker, "Zero-sum Ramsey theorems" Congressus Numerantium , 70 : 1 (1990) pp. 19–130 |
| [a4] | Y. Caro, "Zero-sum problems: a survey" Discrete Math. , 152 (1996) pp. 93–113 |
| [a5] | P. Erdös, A. Ginzburg, A. Ziv, "A theorem in additive number theory" Israel Research and Development Nat. Council Bull., Sect. F , 10 (1961) pp. 41–43 |
| [a6] | Z. Füredi, D. Kleitman, "On zero trees" J. Graph Th. , 16 (1992) pp. 107–120 |
| [a7] | Z. Füredi, D. Kleitman, "The minimal number of zero sums" D. Miklós (ed.) V.T. Sós (ed.) T. Szönyi (ed.) , Combinatorics, Paul Erdös is Eighty , Bolyai Society Mathematical Studies , 1 , Keszthely (Hungary) (1993) pp. 159–172 |
| [a8] | L. Schrijver, P. Seymour, "A simpler proof and generalization of the zero-trees theorem" J. Combin. Th. A , 58 (1991) pp. 301–305 |
Erdös-Ginzburg-Ziv theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Erd%C3%B6s-Ginzburg-Ziv_theorem&oldid=23258