Siegel disc

Consider a holomorphic vector field with a singularity, i.e. of the form $ {\dot{x} } = Ax + \dots $ with $ x \in \mathbf R ^ {b} $ or $ x \in \mathbf C $. The eigenvalues $ \lambda _ {1} \dots \lambda _ {n} $ are said to be resonant if among the eigenvalues there exists a relation of the form $ \lambda _ {s} = ( m , \lambda ) $, where $ \lambda = ( \lambda _ {1} \dots \lambda _ {n} ) $, $ m = ( m _ {1} \dots m _ {n} ) $ with $ m _ {i} \geq 0 $, $ \sum m _ {i} = | m | \geq 2 $ and $ ( m , \lambda ) $ is the usual inner product. H. Poincaré proved in his dissertation that if the eigenvalues of $ A $ are non-resonant, then $ {\dot{x} } = Ax + \dots $ can be reduced to the linear equation $ {\dot{y} } = Ay $ by a formal change of variable $ x = y + \dots $. The $ | m | $ th order term of this change of variable is given in terms of lower-order terms divided by a term of the form $ ( m , \lambda ) - \lambda _ {s} $. Since, in general, such terms can be either zero (in the resonant case) or arbitrarily close to zero (in which case one says that one has small denominators), the power series $ x = y + \dots $ in general does not converge. An example that divergence can occur was already given by L. Euler. The eigenvalue vector $ \lambda $ belongs to the Poincaré domain if zero is not in the convex hull of the eigenvalues $ \lambda _ {j} \in \mathbf C $; its complement is called the Siegel domain. When the vector of eigenvalues $ \lambda $ is in the Poincaré domain, the vector field can be reduced to a polynomial vector field (called the normal form) by a biholomorphic change of variables in a neighbourhood of the singularity. If, in addition, there are no resonances, then one can choose the polynomial normal form to be linear. If $ \lambda $ is in the Siegel domain, then one says it satisfies a Diophantine condition if there exist $ C > 0 $, $ \nu > 0 $ such that $ | {\lambda _ {s} - ( m , \lambda ) } | \geq {C / {| m | ^ \nu } } $ for all $ m _ {i} \geq 0 $ with $ \sum m _ {i} = | m | \geq 2 $. Siegel's theorem states that, in this case, the vector field can be reduced to its linear part by a holomorphic change of coordinates in a neighbourhood of the singularity. Siegel sketched a proof of this result in 1942, but only in the 1970s complete proofs of this theorem were given (by A.N. Kolmogorov, J. Moser, V.I. Arnol'd, M.R. Herman, J.-C. Yoccoz and many others in various settings, e.g. in the $ C ^ {k} $ category). Many problems concerning invariant tori are based on similar small denominator estimates.

In the discrete case, when one considers a mapping $ F ( x ) = Ax + \dots $, the analogous results also holds. In that case, the vector of eigenvalues $ \lambda $ is in the Poincaré domain if the norm of all the eigenvalues $ \lambda _ {i} $ are all smaller or all greater than one. The complement is again called the Siegel domain. If the dimension $ n = 1 $, then this reduces to the unit circle $ | \lambda | = 1 $.

In fact, in one-dimension the Siegel case is completely understood. So, assume that $ | \lambda | = 1 $ and that $ \lambda $ is not a root of unity. Write $ \lambda = { \mathop{\rm exp} } ( 2 \pi i \alpha ) $ and let $ { {p _ {n} } / {q _ {n} } } $ be the continued fraction expansion of $ \alpha $. If $ \sup _ {n \geq 0 } { {( { \mathop{\rm log} } q _ {n + 1 } ) } / {q _ {n} } } = \infty $, then the formal power series of the linearizing coordinates need not converge (Cremer's theorem). Around 1965, A.D. Bryuno proved that if $ \sum { {( { \mathop{\rm log} } q _ {n + 1 } ) } / {q _ {n} } } < \infty $, then this power series converges. In the late 1980s, Yoccoz proved the converse: if the Bryuno condition is not satisfied, then there exists a holomorphic diffeomorphism with linear part $ z \mapsto \lambda z $ which is not holomorphically linearizable. In 1994, Yoccoz was awarded the Field Medal for this achievement.

If $ f $ is a rational mapping on the Riemann sphere, then Siegel domains appear in the Fatou–Sullivan classification theorem. Let $ J ( f ) $ be the closure of the set of repelling periodic points (cf. also Repelling set) and let $ F ( f ) $ be its complement. $ F ( f ) $ is also the set of points that have a neighbourhood $ U $ such that $ f ^ {n} \mid _ {U} $ forms a normal family. Many properties of these sets were already obtained by G. Julia and P. Fatou in the 1920s and 1930s. Using the measurable Riemann mapping theorem (cf. also Quasi-conformal mapping), D. Sullivan proved in the 1980s that each connected component of $ F ( f ) $ is eventually mapped onto a periodic component, and that the periodic components can be of four types:

i) the basin of an attracting periodic point (where the eigenvalue $ \lambda $ of the linear part satisfies $ | \lambda | < 1 $);

ii) the basin of a parabolic periodic point (when $ \lambda $ is a root of unity);

iii) a Siegel domain (when $ \lambda $ has norm one but is not a root of unity, but still the mapping is holomorphically linearizable on some neighbourhood of the fixed point);

iv) a Herman ring (when the component is an annulus on which the mapping is conjugate to a rotation).

References

[a1]	V.I. Arnol'd, "Geometrical methods in the theory of ordinary differential equations" , Grundlehren math. Wiss. , 250 , Springer (1983) (In Russian)
[a2]	A.D. Bryuno, "Analytical form of differential equations" Trans. Moscow Math. Soc. , 25 (1971) pp. 131–288 (In Russian) (Also: 26 (1972), 199–239)
[a3]	M.R. Herman, "Recent results and some open questions on Siegel's linearisation theorem of germs of complex analytic diffeomorphisms of 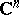near a fixed point" , Proc. VIII Int. Conf. Math. Phys. , World Sci. (1987)
[a4]	C.L. Siegel, "Iteration of analytic functions" Ann. of Math. , 43 (1942) pp. 807–812
[a5]	C.L. Siegel, J. Moser, "Lectures on celestial mechanics" , Springer (1971)
[a6]	J.-C. Yoccoz, "Théorème de Siegel, polynômes quadratiques et nombres de Brjuno" Astérisque , 231 (1995)