Difference between revisions of "Selberg conjecture"
m (convert png to latex) |
|||
| Line 30: | Line 30: | ||
Presently (2000), Selberg's conjecture is part of the "Ramanujan–Petersson conjecture at infinity" . In other words, if interpreting the Ramanujan–Petersson conjecture as a statement about the irreducible representations of -adic groups GL(2,\QQ_p) inside a cuspidal representation of GL(2,\mathcal{A}) (see below), Selberg's conjecture will follow as a statement for GL(2,\RR). | Presently (2000), Selberg's conjecture is part of the "Ramanujan–Petersson conjecture at infinity" . In other words, if interpreting the Ramanujan–Petersson conjecture as a statement about the irreducible representations of p-adic groups GL(2,\QQ_p) inside a cuspidal representation of GL(2,\mathcal{A}) (see below), Selberg's conjecture will follow as a statement for GL(2,\RR). | ||
| − | Indeed, first let \QQ_p denote the completion of the rational field \QQ with respect to the p-adic absolute value |\cdot|_p, p < \infty, and view \RR as the completion with respect to |\cdot|_\infty=|\cdot|. By the adèles, denoted \mathcal{A}, one means the "restricted" direct product \Pi^{\prime}_{p\leq \infty} \QQ_p (restricted so that almost every x_p \in \QQ_p has |x_p|_p \leq 1, ''i.e.'', x_p \in \ZZ_p; cf. also [[Adèle|Adèle]]). Secondly, let L^0_2(Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}) denote the space of measurable functions \phi on Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}, where $G_\mathcal{A} | + | Indeed, first let \QQ_p denote the completion of the rational field \QQ with respect to the p-adic absolute value |\cdot|_p, p < \infty, and view \RR as the completion with respect to |\cdot|_\infty=|\cdot|. By the adèles, denoted \mathcal{A}, one means the "restricted" direct product \Pi^{\prime}_{p\leq \infty} \QQ_p (restricted so that almost every x_p \in \QQ_p has |x_p|_p \leq 1, ''i.e.'', x_p \in \ZZ_p; cf. also [[Adèle|Adèle]]). Secondly, let L^0_2(Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}) denote the space of measurable functions \phi on Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}, where G_\mathcal{A}=GL(2,\mathcal{A})=\Pi^{\prime}_{p\leq\infty}GL(2,\QQ_p) ( "restricted" means g=\prod g_p, with g_p \in GL(2,\ZZ_p) for almost every p), G_\QQ=GL(2,\QQ) imbedded diagonally in G_\mathcal{A}, |
| − | + | $$Z_\mathcal{A} =\left\{ \left(\begin{array}{rr} | |
| + | z & 0 \\ | ||
| + | 0 & z | ||
| + | \end{array}\right): z \in \mathcal{A} \right\}.$$ | ||
is the centre of G_\mathcal{A}, | is the centre of G_\mathcal{A}, | ||
Revision as of 07:14, 29 March 2023
Let \HH denote the upper half-plane, SL(2,\ZZ) the group of integer matrices of determinant one and
\Gamma_0(N)= \left\{ \left(\begin{array}{rr} a & b \\ c & d \end{array}\right) \in SL(2,\ZZ) : c \equiv 0 \,(\operatorname{mod} N) \right\}.
Following H. Maass [a9], let W_s(\Gamma_0(N)) denote the space of bounded functions f on \Gamma_0(N) \backslash \HH that satisfy
\Delta f = \left( \frac{1-s^2}{4} \right) f,
where
\Delta = -y^2 \left( \frac{\partial^2}{\partial^2_x} + \frac{\partial^2}{\partial^2_y} \right)
is the Laplace–Beltrami operator (cf. also Laplace operator). Such eigenfunctions f are called Maass wave forms. Since \Delta in this context is essentially self-adjoint and non-negative (cf. also Self-adjoint operator), it follows that (1-s^2)/4 is real and not zero.
A. Selberg conjectured [a12] that there is a lower bound \ell_1(N) for the smallest (non-zero) eigenvalue: For N \not= 1,
\ell_1(N) \geq 1/4.
This innocent looking conjecture is (cf. [a10]) one of the fundamental unsolved questions in the theory of modular forms (as of 2000; cf. also Modular form). For SL(2,\ZZ) and for small values of N, it has been known for some time (Selberg, W. Roelcke). In general, it has many applications to classical number theory (see [a4] and [a12], for example). To back up his conjecture, Selberg also proved the following assertion:
\ell_1(N) \geq 3/16.
Selberg's approach was to relate this problem to a purely arithmetical question about certain sums of exponentials, called Kloosterman sums (cf. also Exponential sum estimates; Trigonometric sum). This allowed him to invoke results from arithmetic geometry. The key ingredient giving the estimate is a (sharp) bound on Kloosterman sums due to A. Weil [a13]. This bound, in turn, is a consequence of the Riemann hypothesis for the zeta-function of a curve over a finite field, which he had proven earlier (cf. also Riemann hypotheses). On the other hand, to go further than the theorem by this approach one needs to detect cancellations in sums of such Kloosterman sums, and arithmetic geometry offers nothing in this direction. This is the reason that the approach through Kloosterman sums has a natural barrier at 3/16. It is interesting that H. Iwaniec [a5] has given a proof of Selberg's theorem which, while still being along the lines of Kloosterman sums, avoids appealing to Weil's bounds.
Presently (2000), Selberg's conjecture is part of the "Ramanujan–Petersson conjecture at infinity" . In other words, if interpreting the Ramanujan–Petersson conjecture as a statement about the irreducible representations of p-adic groups GL(2,\QQ_p) inside a cuspidal representation of GL(2,\mathcal{A}) (see below), Selberg's conjecture will follow as a statement for GL(2,\RR).
Indeed, first let \QQ_p denote the completion of the rational field \QQ with respect to the p-adic absolute value |\cdot|_p, p < \infty, and view \RR as the completion with respect to |\cdot|_\infty=|\cdot|. By the adèles, denoted \mathcal{A}, one means the "restricted" direct product \Pi^{\prime}_{p\leq \infty} \QQ_p (restricted so that almost every x_p \in \QQ_p has |x_p|_p \leq 1, i.e., x_p \in \ZZ_p; cf. also Adèle). Secondly, let L^0_2(Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}) denote the space of measurable functions \phi on Z_\mathcal{A}G_\QQ \backslash G_\mathcal{A}, where G_\mathcal{A}=GL(2,\mathcal{A})=\Pi^{\prime}_{p\leq\infty}GL(2,\QQ_p) ( "restricted" means g=\prod g_p, with g_p \in GL(2,\ZZ_p) for almost every p), G_\QQ=GL(2,\QQ) imbedded diagonally in G_\mathcal{A},
Z_\mathcal{A} =\left\{ \left(\begin{array}{rr} z & 0 \\ 0 & z \end{array}\right): z \in \mathcal{A} \right\}.
is the centre of G_\mathcal{A},
 |
and
 |
for almost every g. Now, assuming  is an eigenfunction of all Hecke operators T(p), one can define, in a one-to-one way, a function
is an eigenfunction of all Hecke operators T(p), one can define, in a one-to-one way, a function 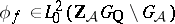 such that the
such that the  -module
-module 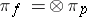 generated by the right
generated by the right 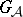 -translates of
-translates of 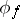 is an irreducible subrepresentation of
is an irreducible subrepresentation of 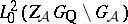 . Then Selberg's conjecture states that the representation
. Then Selberg's conjecture states that the representation  of
of 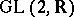 is a principal series
is a principal series  with trivial central character and
with trivial central character and
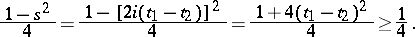 |
In other words, complementary series \pi(s_1,s_2) with s_1-s_2=2s between 0 and 1 (and 1-s^2/4 between 0 and 1/4) should not occur (cf. [a2]; see also Irreducible representation; Principal series). In this context the Ramanujan–Petersson conjecture says that for (almost every)p the same conclusion holds, i.e., for p a class-one representation, that is, p \not| N, \pi_p(s_{1,p},-s_{1,p}) satisfies (cf. [a11])
\operatorname{Re}(s_{1,p}) = 0 .
P. Deligne proved the original Ramanujan conjecture [a1] when \pi_\infty is a holomorphic discrete series of weight k. For example, when f equals Ramanujan's \Delta(z)=\sum_{n=1}^{\infty} \tau(n) e^{2 i \pi n z}, the condition (a1) implies
|\tau(p)| \leq p^{11/2} (p^{s_{1,p}}+p^{s_{2,p}}) \leq 2 p^{11/2}
the famous Ramanujan inequality. In general, Deligne was able to exploit algebraic-geometric interpretations of the classical Ramanujan–Petersson identities. Note that for Selberg's conjecture one again assumes that \pi_\infty is of class-one and \pi_\infty=\pi(s_{1,\infty},s_{2,\infty}) with
\operatorname{Re}(s_{i,\infty})=0.
It was with this modern representation-theoretic point of view that progress was made on Selberg's theorem.
First, consider the mapping
\operatorname{Sym}^m : GL(2,\CC) \longrightarrow GL(m+1,\CC)
defined by action of GL(2,\CC) on symmetric tensors of rank m. It was conjectured by R.P. Langlands [a8] that there should be a corresponding mapping \operatorname{Sym}^m_\star that (roughly) maps cuspidal automorphic representations of GL(2) to those of GL(m+1) ; moreover, whenever \pi corresponds to class-one representations indexed by
\left(\begin{array}{rr} \alpha_p & 0 \\ 0 & \beta_p \end{array}\right)
(including possibly p=\infty), \operatorname{Sym}^m_\star(\pi) should correspond to \operatorname{Sym}^m\left(\begin{array}{rr} \alpha_p & 0 \\ 0 & \beta_p \end{array}\right). This conjecture, for all m, implies both the Selberg and the Ramanujan–Petersson conjecture. In 1978, S. Gelbart and H. Jacquet [a3] proved Langlands' conjecture for m=2; for Selberg's conjecture, this simply replaced the equality in his theorem by an inequality. Then, in 1994 [a7], W. Luo, Z. Rudnick and P. Sarnak used \operatorname{Sym}^2_\star and analytic properties of L-functions to go well beyond Selberg's conjecture:
\ell_1(N) \geq \frac{171}{784} \simeq 0.2181...
And in 2000, H. Kim and F. Shahidi [a6] proved Langlands' conjecture for m=3 and established \ell_1(N) \geq 0.22837..., i.e.,
|\operatorname{Re}(s_{p,i})| \leq \frac{5}{34}
Either Selberg's conjecture will continue to be proved along the lines of Langlands' conjecture, or by entirely new ideas.
There is a far-reaching generalization of Selberg's conjecture to GL(n): If \pi=\otimes_{p\leq\infty} \pi_p is an irreducible cuspidal automorphic representation of GL(n,\mathcal{A}), then every class-one local representation of GL(n,\QQ_p) is "tempered" .
References
| [a1] | P. Deligne, "La conjecture de Weil I" Publ. Math. IHES , 43 (1974) pp. 273–307 MR0340258 Zbl 0314.14007 Zbl 0287.14001 |
| [a2] | I. Gel'fand, M. Graev, I. Piatetski-Shapiro, "Representation theory and automorphic functions" , W.B. Saunders (1969) MR233772 Zbl 0718.11022 Zbl 0138.07201 Zbl 0136.07301 Zbl 0121.30601 |
| [a3] | S. Gelbart, H. Jacquet, "A relation between automorphic representations of GL(2) and GL(3)" Ann. Sci. École Norm. Sup. , 11 (1978) pp. 471–552 MR0533066 Zbl 0406.10022 |
| [a4] | D. Hejhal, "The Selberg trace formula II" , Lecture Notes in Mathematics , 1001 , Springer (1983) Zbl 0543.10020 |
| [a5] | H. Iwaniec, "Selberg's lower bound for the first eigenvalue of congruence groups" , Number Theory, Trace Formula, Discrete Groups , Acad. Press (1989) pp. 371–375 MR993327 |
| [a6] | H. Kim, F. Shahidi, "Functorial products for GL(2) \times GL(3) and functorial symmetric cube for GL(2)" C.R. Acad. Sci. Paris , 331 : 8 (2000) pp. 599–604 MR1799096 Zbl 1002.11044 |
| [a7] | W. Luo, Z. Rudnick, P. Sarnak, "On Selberg's eigenvalue conjecture" Geom. Funct. Anal. , 5 (1995) pp. 387–401 MR1334872 |
| [a8] | R.P. Langlands, "Problems in the theory of automorphic forms" , Lectures in Modern Analysis and Applications , Lecture Notes in Mathematics , 170 , Springer (1970) pp. 18–86 MR0302614 Zbl 0225.14022 |
| [a9] | H. Maass, "Nichtanalytishe Automorphe Funktionen" Math. Ann. , 121 (1949) pp. 141–183 |
| [a10] | P. Sarnak, "Selberg's eigenvalue conjecture" Notices Amer. Math. Soc. , 42 : 4 (1995) pp. 1272–1277 |
| [a11] | I. Satake, "Spherical functions and Ramanujan's conjecture" , Algebraic Groups and Discontinuous Subgroups , Proc. Symp. Pure Math. , IX , Amer. Math. Soc. (1966) pp. 258–264 MR211955 |
| [a12] | A. Selberg, "On the estimation of Fourier coefficients of modular forms" , Proc. Symp. Pure Math. , VIII , Amer. Math. Soc. (1965) pp. 1–15 MR0182610 Zbl 0142.33903 |
| [a13] | A. Weil, "On some exponential sums" Proc. Nat. Acad. Sci. , 34 (1948) pp. 204–207 MR0027006 Zbl 0032.26102 |
Selberg conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Selberg_conjecture&oldid=53528