Difference between revisions of "Acnode"
From Encyclopedia of Mathematics
(TeX) |
m (svg picture) |
||
| Line 2: | Line 2: | ||
An older term, hardly used nowadays (2000), for an isolated point, or hermit point, of a plane algebraic curve (cf. also [[Algebraic curve|Algebraic curve]]). | An older term, hardly used nowadays (2000), for an isolated point, or hermit point, of a plane algebraic curve (cf. also [[Algebraic curve|Algebraic curve]]). | ||
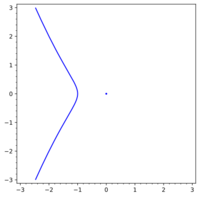
| − | + | [[File:Acnode.svg|center|200px|Acnode]] | |
| − | + | For instance, the point $(0,0)$ is an acnode of the curve $X^3+X^2+Y^2=0$ in $\RR^2$. | |
| − | |||
| − | For instance, the point $(0,0)$ is an acnode of the curve $X^3+X^2+Y^2=0$ in $\ | ||
====References==== | ====References==== | ||
| − | + | * {{Ref|a1}} R.J. Walker, "Algebraic curves", ''Princeton Univ. Press'' (1950) (Reprint: ''Dover'' 1962) | |
Revision as of 19:18, 16 March 2023
An older term, hardly used nowadays (2000), for an isolated point, or hermit point, of a plane algebraic curve (cf. also Algebraic curve).
For instance, the point $(0,0)$ is an acnode of the curve $X^3+X^2+Y^2=0$ in $\RR^2$.
References
- [a1] R.J. Walker, "Algebraic curves", Princeton Univ. Press (1950) (Reprint: Dover 1962)
How to Cite This Entry:
Acnode. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Acnode&oldid=52715
Acnode. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Acnode&oldid=52715
This article was adapted from an original article by M. Hazewinkel (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article