Difference between revisions of "Integral exponential function"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | i0514401.png | ||
| + | $#A+1 = 42 n = 0 | ||
| + | $#C+1 = 42 : ~/encyclopedia/old_files/data/I051/I.0501440 Integral exponential function | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| + | |||
| + | The special function defined for real $ x \neq 0 $ | ||
| + | by the equation | ||
| + | |||
| + | $$ | ||
| + | \mathop{\rm Ei} ( x) = \ | ||
| + | \int\limits _ {- \infty } ^ { x } | ||
| + | |||
| + | \frac{e ^ {t} }{t} | ||
| + | d t = - | ||
| + | \int\limits _ { - } x ^ \infty | ||
| + | |||
| + | \frac{e ^ {-} t }{t} | ||
| + | d t . | ||
| + | $$ | ||
The graph of the integral exponential function is illustrated in Fig.. | The graph of the integral exponential function is illustrated in Fig.. | ||
| Line 9: | Line 32: | ||
Figure: i051440a | Figure: i051440a | ||
| − | Graphs of the functions | + | Graphs of the functions $ y = \mathop{\rm Ei} ( - x ) $, |
| + | $ y = \mathop{\rm Ei} ^ {*} ( x) $ | ||
| + | and $ y = \mathop{\rm Li} ( x) $. | ||
| + | |||
| + | For $ x > 0 $, | ||
| + | the function $ e ^ {t} / t $ | ||
| + | has an infinite discontinuity at $ t = 0 $, | ||
| + | and the integral exponential function is understood in the sense of the principal value of this integral: | ||
| + | |||
| + | $$ | ||
| + | \mathop{\rm Ei} ( x) = \ | ||
| + | \lim\limits _ {\epsilon \rightarrow + 0 } \ | ||
| + | \left \{ | ||
| + | \int\limits _ {- \infty } ^ \epsilon | ||
| − | + | \frac{e ^ {t} }{t} | |
| + | d t + | ||
| + | \int\limits _ \epsilon ^ { x } | ||
| − | + | \frac{e ^ {t} }{t} | |
| + | d t | ||
| + | \right \} . | ||
| + | $$ | ||
The integral exponential function can be represented by the series | The integral exponential function can be represented by the series | ||
| − | + | $$ \tag{1 } | |
| + | \mathop{\rm Ei} ( x) = \ | ||
| + | c + \mathop{\rm ln} ( - x ) + | ||
| + | \sum _ { k= } 1 ^ \infty | ||
| + | |||
| + | \frac{x ^ {k} }{k!k} | ||
| + | ,\ \ | ||
| + | x < 0 , | ||
| + | $$ | ||
and | and | ||
| − | + | $$ \tag{2 } | |
| + | \mathop{\rm Ei} ( x) = c + | ||
| + | \mathop{\rm ln} ( x) + | ||
| + | \sum _ { k= } 1 ^ \infty | ||
| + | |||
| + | \frac{x ^ {k} }{k!k} | ||
| + | ,\ \ | ||
| + | x > 0 , | ||
| + | $$ | ||
| − | where | + | where $ c = 0.5772 \dots $ |
| + | is the [[Euler constant|Euler constant]]. | ||
There is an asymptotic representation: | There is an asymptotic representation: | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ( - x ) \approx \ | ||
| + | |||
| + | \frac{e ^ {-} x }{x} | ||
| + | |||
| + | \left ( | ||
| + | 1 - 1! over {x} + | ||
| + | 2! over {x ^ {2} } - | ||
| + | 3! over {x ^ {3} } + \dots | ||
| + | \right ) ,\ \ | ||
| + | x \rightarrow + \infty . | ||
| + | $$ | ||
| + | |||
| + | As a function of the complex variable $ z $, | ||
| + | the integral exponential function | ||
| + | |||
| + | $$ | ||
| + | \mathop{\rm Ei} ( z) = \ | ||
| + | C + \mathop{\rm ln} ( - z ) + | ||
| + | \sum _ { k= } 1 ^ \infty | ||
| + | |||
| + | \frac{z ^ {k} }{k!k} | ||
| + | ,\ \ | ||
| + | | \mathop{\rm arg} ( - z ) | < \pi , | ||
| + | $$ | ||
| − | + | is a single-valued analytic function in the $ z $- | |
| + | plane slit along the positive real semi-axis $ ( 0 < \mathop{\rm arg} z < 2 \pi ) $; | ||
| + | here the value of $ \mathop{\rm ln} ( - z) $ | ||
| + | is chosen such that $ - \pi < { \mathop{\rm Im} \mathop{\rm ln} } (- z) < \pi $. | ||
| + | The behaviour of $ \mathop{\rm Ei} ( z) $ | ||
| + | close to the slit is described by the limiting relations: | ||
| − | + | $$ | |
| + | \left . | ||
| + | \begin{array}{c} | ||
| + | \lim\limits _ {\eta \downarrow 0 } \ | ||
| + | \mathop{\rm Ei} ( z + i \eta ) = \ | ||
| + | \mathop{\rm Ei} ( z) - i \pi , \\ | ||
| + | \lim\limits _ {\eta \downarrow 0 } \ | ||
| + | \mathop{\rm Ei} ( z - i \eta ) = \ | ||
| + | \mathop{\rm Ei} ( z) + i \pi , \\ | ||
| + | \end{array} | ||
| + | \right \} \ \ | ||
| + | z = x + i y. | ||
| + | $$ | ||
| − | + | The asymptotic representation in the region $ 0 < \mathop{\rm arg} z < 2 \pi $ | |
| + | is: | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ( z) \sim \ | ||
| − | + | \frac{e ^ {z} }{z} | |
| − | + | \left ( | |
| + | 1! over {z} + | ||
| + | 2! over {z ^ {2} } + \dots | ||
| + | + k! over {z ^ {k} } + \dots | ||
| + | \right ) ,\ \ | ||
| + | | z | \rightarrow \infty . | ||
| + | $$ | ||
| − | The integral exponential function is related to the [[Integral logarithm|integral logarithm]] | + | The integral exponential function is related to the [[Integral logarithm|integral logarithm]] $ \mathop{\rm li} ( x) $ |
| + | by the formulas | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ( x) = \ | ||
| + | \mathop{\rm li} ( e ^ {x} ) ,\ \ | ||
| + | x < 0 , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ( \mathop{\rm ln} x ) = \mathop{\rm li} ( x) ,\ x < 1 ; | ||
| + | $$ | ||
| − | and to the [[Integral sine|integral sine]] | + | and to the [[Integral sine|integral sine]] $ \mathop{\rm Si} ( x) $ |
| + | and the [[Integral cosine|integral cosine]] $ \mathop{\rm Ci} ( x) $ | ||
| + | by the formulas: | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ( \pm i x ) = \ | ||
| + | \mathop{\rm Ci} ( x) \pm i \mathop{\rm Si} ( x) \mps | ||
| + | \frac{\pi i }{2} | ||
| + | ,\ \ | ||
| + | x > 0 . | ||
| + | $$ | ||
The differentiation formula is: | The differentiation formula is: | ||
| − | + | $$ | |
| + | |||
| + | \frac{d ^ {n} \mathop{\rm Ei} ( - x ) }{d x ^ {n} } | ||
| + | = \ | ||
| + | ( - 1 ) ^ {n-} 1 | ||
| + | ( n - 1 ) ! x ^ {-} x | ||
| + | e ^ {-} x e _ {n-} 1 ( x) ,\ \ | ||
| + | n = 1 , 2 , . . . . | ||
| + | $$ | ||
The following notations are sometimes used: | The following notations are sometimes used: | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ^ {+} ( z) = \ | ||
| + | \mathop{\rm Ei} ( z + i 0 ) ,\ \ | ||
| + | \mathop{\rm Ei} ^ {-} ( z) = \ | ||
| + | \mathop{\rm Ei} ( z - i 0 ) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | \mathop{\rm Ei} ^ {*} ( z) = { \mathop{\rm Ei} ( z) } bar = \mathop{\rm Ei} ( z) + \pi i . | ||
| + | $$ | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , ''Higher transcendental functions'' , '''2. Bessel functions, parabolic cylinder functions, orthogonal polynomials''' , McGraw-Hill (1953)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , ''Higher transcendental functions'' , '''2. Bessel functions, parabolic cylinder functions, orthogonal polynomials''' , McGraw-Hill (1953)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian)</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | The function | + | The function $ \mathop{\rm Ei} $ |
| + | is usually called the exponential integral. | ||
| − | Instead of by the series representation, for complex values of | + | Instead of by the series representation, for complex values of $ z $( |
| + | $ x $ | ||
| + | not positive real) the function $ \mathop{\rm Ei} ( z) $ | ||
| + | can be defined by the integal (as for real $ x \neq 0 $); | ||
| + | since the integrand is analytic, the integral is path-independent in $ \mathbf C \setminus \{ {x \in \mathbf R } : {x \geq 0 } \} $. | ||
| − | Formula (1) with | + | Formula (1) with $ x $ |
| + | replaced by $ z $ | ||
| + | then holds for $ | \mathop{\rm arg} ( - z ) | < \pi $, | ||
| + | and the function defined by (2) (for $ x > 0 $) | ||
| + | is also known as the modified exponential integral. | ||
Revision as of 22:12, 5 June 2020
The special function defined for real $ x \neq 0 $
by the equation
$$ \mathop{\rm Ei} ( x) = \ \int\limits _ {- \infty } ^ { x } \frac{e ^ {t} }{t} d t = - \int\limits _ { - } x ^ \infty \frac{e ^ {-} t }{t} d t . $$
The graph of the integral exponential function is illustrated in Fig..
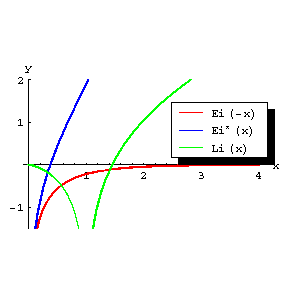
Figure: i051440a
Graphs of the functions $ y = \mathop{\rm Ei} ( - x ) $, $ y = \mathop{\rm Ei} ^ {*} ( x) $ and $ y = \mathop{\rm Li} ( x) $.
For $ x > 0 $, the function $ e ^ {t} / t $ has an infinite discontinuity at $ t = 0 $, and the integral exponential function is understood in the sense of the principal value of this integral:
$$ \mathop{\rm Ei} ( x) = \ \lim\limits _ {\epsilon \rightarrow + 0 } \ \left \{ \int\limits _ {- \infty } ^ \epsilon \frac{e ^ {t} }{t} d t + \int\limits _ \epsilon ^ { x } \frac{e ^ {t} }{t} d t \right \} . $$
The integral exponential function can be represented by the series
$$ \tag{1 } \mathop{\rm Ei} ( x) = \ c + \mathop{\rm ln} ( - x ) + \sum _ { k= } 1 ^ \infty \frac{x ^ {k} }{k!k} ,\ \ x < 0 , $$
and
$$ \tag{2 } \mathop{\rm Ei} ( x) = c + \mathop{\rm ln} ( x) + \sum _ { k= } 1 ^ \infty \frac{x ^ {k} }{k!k} ,\ \ x > 0 , $$
where $ c = 0.5772 \dots $ is the Euler constant.
There is an asymptotic representation:
$$ \mathop{\rm Ei} ( - x ) \approx \ \frac{e ^ {-} x }{x} \left ( 1 - 1! over {x} + 2! over {x ^ {2} } - 3! over {x ^ {3} } + \dots \right ) ,\ \ x \rightarrow + \infty . $$
As a function of the complex variable $ z $, the integral exponential function
$$ \mathop{\rm Ei} ( z) = \ C + \mathop{\rm ln} ( - z ) + \sum _ { k= } 1 ^ \infty \frac{z ^ {k} }{k!k} ,\ \ | \mathop{\rm arg} ( - z ) | < \pi , $$
is a single-valued analytic function in the $ z $- plane slit along the positive real semi-axis $ ( 0 < \mathop{\rm arg} z < 2 \pi ) $; here the value of $ \mathop{\rm ln} ( - z) $ is chosen such that $ - \pi < { \mathop{\rm Im} \mathop{\rm ln} } (- z) < \pi $. The behaviour of $ \mathop{\rm Ei} ( z) $ close to the slit is described by the limiting relations:
$$ \left . \begin{array}{c} \lim\limits _ {\eta \downarrow 0 } \ \mathop{\rm Ei} ( z + i \eta ) = \ \mathop{\rm Ei} ( z) - i \pi , \\ \lim\limits _ {\eta \downarrow 0 } \ \mathop{\rm Ei} ( z - i \eta ) = \ \mathop{\rm Ei} ( z) + i \pi , \\ \end{array} \right \} \ \ z = x + i y. $$
The asymptotic representation in the region $ 0 < \mathop{\rm arg} z < 2 \pi $ is:
$$ \mathop{\rm Ei} ( z) \sim \ \frac{e ^ {z} }{z} \left ( 1! over {z} + 2! over {z ^ {2} } + \dots + k! over {z ^ {k} } + \dots \right ) ,\ \ | z | \rightarrow \infty . $$
The integral exponential function is related to the integral logarithm $ \mathop{\rm li} ( x) $ by the formulas
$$ \mathop{\rm Ei} ( x) = \ \mathop{\rm li} ( e ^ {x} ) ,\ \ x < 0 , $$
$$ \mathop{\rm Ei} ( \mathop{\rm ln} x ) = \mathop{\rm li} ( x) ,\ x < 1 ; $$
and to the integral sine $ \mathop{\rm Si} ( x) $ and the integral cosine $ \mathop{\rm Ci} ( x) $ by the formulas:
$$ \mathop{\rm Ei} ( \pm i x ) = \ \mathop{\rm Ci} ( x) \pm i \mathop{\rm Si} ( x) \mps \frac{\pi i }{2} ,\ \ x > 0 . $$
The differentiation formula is:
$$ \frac{d ^ {n} \mathop{\rm Ei} ( - x ) }{d x ^ {n} } = \ ( - 1 ) ^ {n-} 1 ( n - 1 ) ! x ^ {-} x e ^ {-} x e _ {n-} 1 ( x) ,\ \ n = 1 , 2 , . . . . $$
The following notations are sometimes used:
$$ \mathop{\rm Ei} ^ {+} ( z) = \ \mathop{\rm Ei} ( z + i 0 ) ,\ \ \mathop{\rm Ei} ^ {-} ( z) = \ \mathop{\rm Ei} ( z - i 0 ) , $$
$$ \mathop{\rm Ei} ^ {*} ( z) = { \mathop{\rm Ei} ( z) } bar = \mathop{\rm Ei} ( z) + \pi i . $$
References
| [1] | H. Bateman (ed.) A. Erdélyi (ed.) et al. (ed.) , Higher transcendental functions , 2. Bessel functions, parabolic cylinder functions, orthogonal polynomials , McGraw-Hill (1953) |
| [2] | E. Jahnke, F. Emde, "Tables of functions with formulae and curves" , Dover, reprint (1945) (Translated from German) |
| [3] | A. Krazer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960) |
| [4] | N.N. Lebedev, "Special functions and their applications" , Prentice-Hall (1965) (Translated from Russian) |
Comments
The function $ \mathop{\rm Ei} $ is usually called the exponential integral.
Instead of by the series representation, for complex values of $ z $( $ x $ not positive real) the function $ \mathop{\rm Ei} ( z) $ can be defined by the integal (as for real $ x \neq 0 $); since the integrand is analytic, the integral is path-independent in $ \mathbf C \setminus \{ {x \in \mathbf R } : {x \geq 0 } \} $.
Formula (1) with $ x $ replaced by $ z $ then holds for $ | \mathop{\rm arg} ( - z ) | < \pi $, and the function defined by (2) (for $ x > 0 $) is also known as the modified exponential integral.
Integral exponential function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Integral_exponential_function&oldid=47371