Difference between revisions of "User:Ulf Rehmann/Table of automatically generated TeX code"
Ulf Rehmann (talk | contribs) m (→Formal group) |
Ulf Rehmann (talk | contribs) m (→Formal group) |
||
| Line 296: | Line 296: | ||
png file | png file | ||
|- | |- | ||
| − | | 43.([[User:Maximilian Janisch/latexlist/latex/Algebraic Groups/E|120.]])*|| https://www.encyclopediaofmath.org/legacyimages/f/f040/f040820/f040820118.png || $\operatorname { og } F _ { MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ C ^ { - } P ^ { - 1 } ] X ^ { i }$ || $$ \operatorname { log } F _ {\rm MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ {\rm CP ^ {i - 1 } ] X ^ { i }, $$ || conf 0.098 F | + | | 43.([[User:Maximilian Janisch/latexlist/latex/Algebraic Groups/E|120.]])*|| https://www.encyclopediaofmath.org/legacyimages/f/f040/f040820/f040820118.png || $\operatorname { og } F _ { MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ C ^ { - } P ^ { - 1 } ] X ^ { i }$ || $$ \operatorname { log } F _ {\rm MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ {\rm CP} ^ {i - 1 } ] X ^ { i }, $$ || conf 0.098 F |
f040820118.png (118) | f040820118.png (118) | ||
|- | |- | ||
| − | | 44.([[User:Maximilian Janisch/latexlist/latex/Algebraic Groups/E|147.]])*|| https://www.encyclopediaofmath.org/legacyimages/f/f040/f040820/f04082059.png || $( x _ { 1 } , \ldots , x _ { x } ) \circ ( y _ { 1 } , \ldots , y _ { n } ) = ( z _ { 1 } , \ldots , z _ { x } )$ || $$ | + | | 44.([[User:Maximilian Janisch/latexlist/latex/Algebraic Groups/E|147.]])*|| https://www.encyclopediaofmath.org/legacyimages/f/f040/f040820/f04082059.png || $( x _ { 1 } , \ldots , x _ { x } ) \circ ( y _ { 1 } , \ldots , y _ { n } ) = ( z _ { 1 } , \ldots , z _ { x } )$ || $$ ( x _ { 1 } , \ldots , x _ { n } ) \circ ( y _ { 1 } , \ldots , y _ { n } ) = ( z _ { 1 } , \ldots , z _ { n } ), $$ || conf 0.553 F |
f04082059.png (59) | f04082059.png (59) | ||
Revision as of 18:34, 4 November 2019
Algebraic curve
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 1.(23.) | 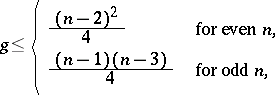 |
$g \leq \left\{ \begin{array} { l l } { \frac { ( n - 2 ) ^ { 2 } } { 4 } } & { \text { for even } n } \\ { \frac { ( n - 1 ) ( n - 3 ) } { 4 } } & { \text { for odd } n } \end{array} \right.$ | $$ g \leq \left\{ \begin{array} { l l } { \frac { ( n - 2 ) ^ { 2 } } { 4 } } & { \text { for even } n, } \\ { \frac { ( n - 1 ) ( n - 3 ) } { 4 } } & { \text { for odd } n, } \end{array} \right.$$ | conf 0.698
a01145065.png (65) |
Algebraic geometry
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 2.(116.) |  |
$\theta = \int _ { 0 } ^ { \lambda } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } }$ | $$\theta = \int\limits _ { 0 } ^ { \lambda } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } }, $$ | conf 0.997
a01150014.png (14) |
| 3.(133.) |  |
$\omega = 2 \int _ { 0 } ^ { 1 / c } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } }$ | $$\omega = 2 \int\limits _ { 0 } ^ { 1 / c } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } }, $$ | conf 0.973
a01150021.png (21) |
| 4.(67.) |  |
$\overline { w } = 2 \int _ { 0 } ^ { 1 / \varepsilon } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } }$ | $$\widetilde{ w } = 2 \int\limits _ { 0 } ^ { 1 / \varepsilon } \frac { d x } { \sqrt { ( 1 - c ^ { 2 } x ^ { 2 } ) ( 1 - e ^ { 2 } x ^ { 2 } ) } },$$ | conf 0.107
a01150022.png (22) |
| 5.(105.) |  |
$\theta ( v + \pi i r ) = \theta ( r ) , \quad \theta ( v + \alpha _ { j } ) = e ^ { L _ { j } ( v ) } \theta ( v )$ | $$\theta ( v + \pi i r ) = \theta ( r ) , \quad \theta ( v + \alpha _ { j } ) = e ^ { L _ { j } ( v ) } \theta ( v ), $$ | conf 0.775
a01150044.png (44) |
| 6.(17.) |  |
$\left( \begin{array} { l l } { \alpha } & { b } \\ { c } & { d } \end{array} \right) \equiv \left( \begin{array} { l l } { 1 } & { 0 } \\ { 0 } & { 1 } \end{array} \right) ( \operatorname { mod } 7 )$ | $$\left( \begin{array} { l l } { \alpha } & { b } \\ { c } & { d } \end{array} \right) \equiv \left( \begin{array} { l l } { 1 } & { 0 } \\ { 0 } & { 1 } \end{array} \right) ( \operatorname { mod } 7 ). $$ | conf 0.440
a01150078.png (78) |
Algebraic surface
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 7.(144.) |  |
$0 \rightarrow O _ { V } \rightarrow E _ { \alpha } \rightarrow T _ { V } \rightarrow 0$ | $$0 \rightarrow {\cal O} _ { V } \rightarrow E _ { \alpha } \rightarrow T _ { V } \rightarrow 0$$ | conf 0.981
a011640132.png (132) |
| 8.(73.) | 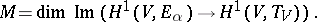 |
$M = \operatorname { dim } \operatorname { Im } ( H ^ { 1 } ( V , E _ { \alpha } ) \rightarrow H ^ { 1 } ( V , T _ { V } ) )$ | $$ M = \operatorname { dim } \operatorname { Im } ( H ^ { 1 } ( V , E _ { \alpha } ) \rightarrow H ^ { 1 } ( V , T _ { V } ) ). $$ | conf 0.997
a011640137.png (137) |
| 9.(88.) |  |
$\operatorname { dim } _ { k } H ^ { 2 } ( V , E _ { \alpha } ) + \operatorname { dim } _ { k } H ^ { 2 } ( V , T _ { V } )$ | $$ \operatorname { dim } _ { k } H ^ { 2 } ( V , E _ { \alpha } ) + \operatorname { dim } _ { k } H ^ { 2 } ( V , T _ { V } ). $$ | conf 0.996
a011640139.png (139) |
| 10.(117.) | 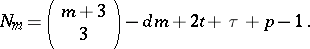 |
$N _ { m } = \left( \begin{array} { c } { m + 3 } \\ { 3 } \end{array} \right) - d m + 2 t + \tau + p - 1$ | $$ N _ { m } = \left( \begin{array} { c } { m + 3 } \\ { 3 } \end{array} \right) - d m + 2 t + \tau + p - 1. $$ | conf 0.369
a01164027.png (27) |
| 11.(72.) | 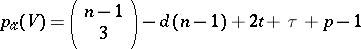 |
$p _ { \alpha } ( V ) = \left( \begin{array} { c } { n - 1 } \\ { 3 } \end{array} \right) - d ( n - 1 ) + 2 t + \tau + p - 1$ | $$ p _ { \alpha } ( V ) = \left( \begin{array} { c } { n - 1 } \\ { 3 } \end{array} \right) - d ( n - 1 ) + 2 t + \tau + p - 1 $$ | conf 0.396
a01164029.png (29) |
| 12.(68.)* | 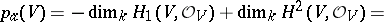 |
$p _ { x } ( V ) = - \operatorname { dim } _ { k } H _ { 1 } ( V , O _ { V } ) + \operatorname { dim } _ { k } H ^ { 2 } ( V , O _ { V } ) =$ | $$p _ { \alpha } ( V ) = - \operatorname { dim } _ { k } H _ { 1 } ( V , {\cal O} _ { V } ) + \operatorname { dim } _ { k } H ^ { 2 } ( V , {\cal O} _ { V } ) =$$ | conf 0.756 F
a01164047.png (47) |
| 13.(93.)* | 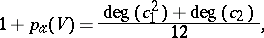 |
$1 + p _ { x } ( V ) = \frac { \operatorname { deg } ( c _ { 1 } ^ { 2 } ) + \operatorname { deg } ( c _ { 2 } ) } { 12 }$ | $$ 1 + p _ { \alpha } ( V ) = \frac { \operatorname { deg } ( c _ { 1 } ^ { 2 } ) + \operatorname { deg } ( c _ { 2 } ) } { 12 },$$ | conf 0.752 F
a01164053.png (53) |
Cartan subalgebra
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 14.(33.)* | 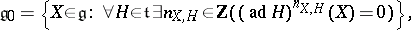 |
$\mathfrak { g } 0 = \{ X \in \mathfrak { g } : \forall H \in \mathfrak { t } \exists \mathfrak { n } X , H \in Z ( ( \text { ad } H ) ^ { n } X , H ( X ) = 0 ) \}$ | $$\mathfrak { g }_0 = \big\{ X \in \mathfrak { g } : \forall H \in \mathfrak { t } \exists { n }_{X,H} \in {\mathbb Z} ( ( \text { ad } H ) ^ { n_{X , H} } ( X ) = 0 ) \big\},$$ | conf 0.110 F
c0205509.png (9) |
Cartan theorem
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 15.(49.)* | 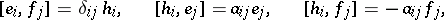 |
$f _ { j } ] = \delta _ { i j } h _ { i } , \quad [ h _ { i } , e _ { j } ] = \alpha _ { i j } e _ { j } , \quad [ h _ { i } , f _ { j } ] = - \alpha _ { j } f _ { j }$ | $$ [e_i, f _ { j } ] = \delta _ { i j } h _ { i } , \quad [ h _ { i } , e _ { j } ] = \alpha _ { i j } e _ { j } , \quad [ h _ { i } , f _ { j } ] = - \alpha _ { ij } f _ { j }, $$ | conf 0.149 F
c0205704.png (4) |
| 16.(55.)* | 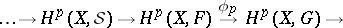 |
$\rightarrow H ^ { p } ( X , S ) \rightarrow H ^ { p } ( X , F ) \stackrel { \phi p } { \rightarrow } H ^ { p } ( X , G ) \rightarrow$ | $$ \dots \rightarrow H ^ { p } ( X , S ) \rightarrow H ^ { p } ( X , F ) \stackrel { \phi_p } { \rightarrow } H ^ { p } ( X , G ) \rightarrow $$ | conf 0.853 F
c02057064.png (64) |
Comitant
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 17.(7.) | 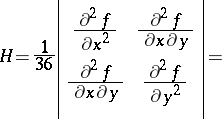 |
$H = \frac { 1 } { 36 } \left| \begin{array} { c c } { \frac { \partial ^ { 2 } f } { \partial x ^ { 2 } } } & { \frac { \partial ^ { 2 } f } { \partial x \partial y } } \\ { \frac { \partial ^ { 2 } f } { \partial x \partial y } } & { \frac { \partial ^ { 2 } f } { \partial y ^ { 2 } } } \end{array} \right| =$ | $$H = \frac { 1 } { 36 } \left| \begin{array} { c c } { \frac { \partial ^ { 2 } f } { \partial x ^ { 2 } } } & { \frac { \partial ^ { 2 } f } { \partial x \partial y } } \\ { \frac { \partial ^ { 2 } f } { \partial x \partial y } } & { \frac { \partial ^ { 2 } f } { \partial y ^ { 2 } } } \end{array} \right| =$$ | conf 0.956
c02333033.png (33) |
| 18.(76.) | 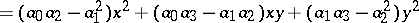 |
$= ( a _ { 0 } a _ { 2 } - a _ { 1 } ^ { 2 } ) x ^ { 2 } + ( a _ { 0 } a _ { 3 } - a _ { 1 } a _ { 2 } ) x y + ( a _ { 1 } a _ { 3 } - a _ { 2 } ^ { 2 } ) y ^ { 2 }$ | $$ = ( a _ { 0 } a _ { 2 } - a _ { 1 } ^ { 2 } ) x ^ { 2 } + ( a _ { 0 } a _ { 3 } - a _ { 1 } a _ { 2 } ) x y + ( a _ { 1 } a _ { 3 } - a _ { 2 } ^ { 2 } ) y ^ { 2 } $$ | conf 0.549
c02333034.png (34) |
| 19.(11.)* | 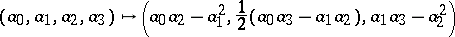 |
$( \alpha _ { 0 } , \alpha _ { 1 } , \alpha _ { 2 } , \alpha _ { 3 } ) \mapsto ( \alpha _ { 0 } \alpha _ { 2 } - \alpha _ { 1 } ^ { 2 } , \frac { 1 } { 2 } ( \alpha _ { 0 } \alpha _ { 3 } - \alpha _ { 1 } \alpha _ { 2 } ) , \alpha _ { 1 } \alpha _ { 3 } - \alpha _ { 2 } ^ { 2 } )$ | $$ ( \alpha _ { 0 } , \alpha _ { 1 } , \alpha _ { 2 } , \alpha _ { 3 } ) \mapsto ( \alpha _ { 0 } \alpha _ { 2 } - \alpha _ { 1 } ^ { 2 } , \frac { 1 } { 2 } ( \alpha _ { 0 } \alpha _ { 3 } - \alpha _ { 1 } \alpha _ { 2 } ) , \alpha _ { 1 } \alpha _ { 3 } - \alpha _ { 2 } ^ { 2 } ) $$ | conf 0.521 F
c02333035.png (35) |
Deformation
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 20.(26.) |  |
$\operatorname { Aut } _ { R ^ { \prime } } ( X ^ { \prime } | X _ { 0 } ) \rightarrow \operatorname { Aut } _ { R } ( X _ { R ^ { \prime } } ^ { \prime } \otimes R | X _ { 0 } )$ | $$ \operatorname { Aut } _ { R ^ { \prime } } ( X ^ { \prime } | X _ { 0 } ) \rightarrow \operatorname { Aut } _ { R } ( X _ { R ^ { \prime } } ^ { \prime } \otimes R | X _ { 0 } ) $$ | conf 0.683
\ d030700175.png (175) |
| 21.(27.) | 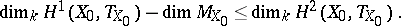 |
$\operatorname { dim } _ { k } H ^ { 1 } ( X _ { 0 } , T _ { X _ { 0 } } ) - \operatorname { dim } M _ { X _ { 0 } } \leq \operatorname { dim } _ { k } H ^ { 2 } ( X _ { 0 } , T _ { X _ { 0 } } )$ | $$ \operatorname { dim } _ { k } H ^ { 1 } ( X _ { 0 } , T _ { X _ { 0 } } ) - \operatorname { dim } M _ { X _ { 0 } } \leq \operatorname { dim } _ { k } H ^ { 2 } ( X _ { 0 } , T _ { X _ { 0 } } ). $$ | conf 0.944
d030700190.png (190) |
| 22.(78.)* | 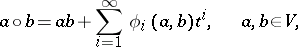 |
$\alpha \circ b = \alpha b + \sum _ { i = 1 } ^ { \infty } \phi _ { i } ( \alpha , b ) t ^ { i } , \quad \alpha , b \in V$ | $$ \alpha \circ b = \alpha b + \sum _ { i = 1 } ^ { \infty } \phi _ { i } ( \alpha , b ) t ^ { i } , \quad \alpha , b \in V, $$ | conf 0.097 F
d030700263.png (263) |
| 23.(96.)* | 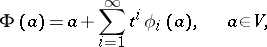 |
$\Phi ( \alpha ) = \alpha + \sum _ { i = 1 } ^ { \infty } t ^ { i } \phi _ { i } ( \alpha ) , \quad \alpha \in V$ | $$ \Phi ( \alpha ) = \alpha + \sum _ { i = 1 } ^ { \infty } t ^ { i } \phi _ { i } ( \alpha ) , \quad \alpha \in V, $$ | conf 0.873 F
d030700270.png (270) |
Differential algebra
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 24.(106.) | 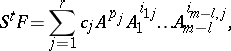 |
$S ^ { t } F = \sum _ { j = 1 } ^ { r } c _ { j } A ^ { p _ { j } } A _ { 1 } ^ { i _ { 1 j } } \dots A _ { m - l } ^ { i _ { m - l } , j }$ | $$ S ^ { t } F = \sum _ { j = 1 } ^ { r } c _ { j } A ^ { p _ { j } } A _ { 1 } ^ { i _ { 1 j } } \dots A _ { m - l } ^ { i _ { { m - l } , j } },$$ | conf 0.149
d031830107.png (107) |
| 25.(146.)* | 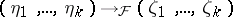 |
$( \eta _ { 1 } , \ldots , \eta _ { k } ) \rightarrow F ( \zeta _ { 1 } , \ldots , \zeta _ { k } )$ | $ ( \eta _ { 1 } , \ldots , \eta _ { k } ) \rightarrow {}_{\cal F} ( \zeta _ { 1 } , \ldots , \zeta _ { k } ) $ | conf 0.562 F
d031830141.png (141) |
| 26.(145.)$^F$* | 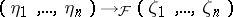 |
$( \eta _ { 1 } , \ldots , \eta _ { n } ) \rightarrow F ( \zeta _ { 1 } , \ldots , \zeta _ { n } )$ | $ ( \eta _ { 1 } , \ldots , \eta _ { n } ) \rightarrow {}_{\cal F} ( \zeta _ { 1 } , \ldots , \zeta _ { n } ) $ | conf 0.376 F
d031830150.png (150) |
| 27.(57.) | 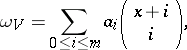 |
$\omega _ { V } = \sum _ { 0 \leq i \leq m } \alpha _ { i } \left( \begin{array} { c } { x + i } \\ { i } \end{array} \right)$ | $$ \omega _ { V } = \sum _ { 0 \leq i \leq m } \alpha _ { i } \left( \begin{array} { c } { x + i } \\ { i } \end{array} \right), $$ | conf 0.780
d03183016.png (16) |
| 28.(111.) | 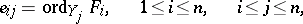 |
$e _ { i j } = \operatorname { ord } _ { Y } _ { j } F _ { i } , \quad 1 \leq i \leq n , \quad i \leq j \leq n$ | $$ e_ { i j } = \operatorname { ord } _ { { Y } _ { j } } F _ { i } , \quad 1 \leq i \leq n , \quad i \leq j \leq n, $$ | conf 0.187
d03183043.png (43) |
Dimension polynomial
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 29.(48.) | 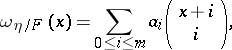 |
$\omega _ { \eta / F } ( x ) = \sum _ { 0 \leq i \leq m } \alpha _ { i } \left( \begin{array} { c } { x + i } \\ { i } \end{array} \right)$ | $$ \omega _ { \eta / F } ( x ) = \sum _ { 0 \leq i \leq m } \alpha _ { i } \left( \begin{array} { c } { x + i } \\ { i } \end{array} \right), $$ | conf 0.968
d03249029.png (29) |
Duality
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file | |
|---|---|---|---|---|---|
| 30.(118.)* |  |
$H ^ { p } ( X , F ) \times H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( F , \Omega ) ) \rightarrow C$ | $$ H ^ { p } ( X , {\cal F} ) \times H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( {\cal F} , \Omega ) ) \rightarrow {\mathbf C}, $$ | conf 0.824 F
d034120173.png (173) | |
| 31.(59.)* | 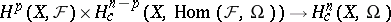 |
$H ^ { p } ( X , F ) \times H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( F , \Omega ) ) \rightarrow H _ { c } ^ { n } ( X , \Omega )$ | $$ H ^ { p } ( X , {\cal F} ) \times H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( {\cal F} , \Omega ) ) \rightarrow H _ { c } ^ { n } ( X , \Omega ) $$ | conf 0.921 F
d034120175.png (175) | |
| 32.(124.)* | 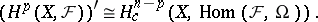 |
$( H ^ { p } ( X , F ) ) ^ { \prime } \cong H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( F , \Omega ) )$ | $$ ( H ^ { p } ( X , {\cal F} ) ) ^ { \prime } \cong H _ { c } ^ { n - p } ( X , \operatorname { Hom } ( {\cal F} , \Omega ) ). $$ | conf 0.829 F
d034120184.png (184) | |
| 33.(29.)* |  |
$\beta : \operatorname { Ext } _ { c } ^ { n - p - 1 } ( X F , \Omega ) \rightarrow \operatorname { Ext } _ { c } ^ { n - p - 1 } ( X \backslash Y || F , \Omega )$ | $$ \beta : \operatorname { Ext } _ { c } ^ { n - p - 1 } ( X ; {\cal F} , \Omega ) \rightarrow \operatorname { Ext } _ { c } ^ { n - p - 1 } ( X \backslash Y ; {\cal F} , \Omega ). $$ | conf 0.634 | F
d034120236.png (236) |
| 34.(77.)* | 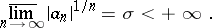 |
$\underset { n \rightarrow \infty } { \operatorname { lim } } | \alpha _ { n } | ^ { 1 / n } = \sigma < + \infty$ | $$ \underset { n \rightarrow \infty } { \overline { \lim } } | \alpha _ { n } | ^ { 1 / n } = \sigma < + \infty. $$ | conf 0.521 F
d034120247.png (247) | |
| 35.(58.)* | 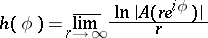 |
$h ( \phi ) = \operatorname { lim } _ { r \rightarrow \infty } \frac { \operatorname { ln } | A ( r e ^ { i \phi } ) | } { r }$ | $$ h ( \phi ) = \underset { n\rightarrow \infty }{\overline{ \lim } } \frac { \operatorname { ln } | A ( r e ^ { i \phi } ) | } { r } $$ | conf 0.861 F
d034120253.png (253) | |
| 36.(69.)* | 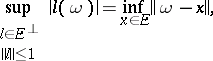 |
$\operatorname { sup } _ { l \in E ^ { \perp } } | l ( \omega ) | = \operatorname { inf } _ { x \in E } \| \omega - x \|$ | $$ \operatorname* { sup } _ { l \in E^\perp \atop \|l\|\le 1 } | l ( \omega ) | = \operatorname* { inf } _ { x \in E } \| \omega - x \|, $$ | conf 0.293 F
d034120360.png (360) | |
| 37.(15.) | 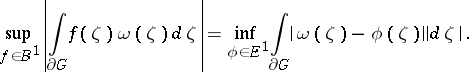 |
$\operatorname { sup } _ { f \in B ^ { 1 } } | \int _ { \partial G } f ( \zeta ) \omega ( \zeta ) d \zeta | = \operatorname { inf } _ { \phi \in E ^ { 1 } } \int _ { \partial G } | \omega ( \zeta ) - \phi ( \zeta ) \| d \zeta |$ | $$ \operatorname* { sup } _ { f \in B ^ { 1 } } \big| \int\limits _ { \partial G } f ( \zeta ) \omega ( \zeta ) d \zeta \big| = \operatorname* { inf } _ { \phi \in E ^ { 1 } } \int\limits _ { \partial G } | \omega ( \zeta ) - \phi ( \zeta ) | | d \zeta |. $$ | conf 0.508
d034120376.png (376) | |
| 38.(52.) | 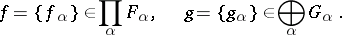 |
$f = \{ f _ { \alpha } \} \in \prod _ { \alpha } F _ { \alpha } , \quad g = \{ g _ { \alpha } \} \in \oplus _ { \alpha } G _ { \alpha }$ | $$ f = \{ f _ { \alpha } \} \in \prod _ { \alpha } F _ { \alpha } , \quad g = \{ g _ { \alpha } \} \in \operatorname*\oplus _ { \alpha } G _ { \alpha }. $$ | conf 0.491
d034120509.png (509) | |
| 39.(140.) | 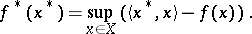 |
$f ^ { * } ( x ^ { * } ) = \operatorname { sup } _ { x \in X } ( \langle x ^ { * } , x \rangle - f ( x ) )$ | $$ f ^ { * } ( x ^ { * } ) = \operatorname*{ sup } _ { x \in X } ( \langle x ^ { * } , x \rangle - f ( x ) ) $$ | conf 0.900
d034120535.png (535) | |
| 40.(94.) | 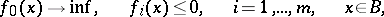 |
$f _ { 0 } ( x ) \rightarrow \text { inf, } \quad f _ { i } ( x ) \leq 0 , \quad i = 1 , \ldots , m , \quad x \in B$ | $$ f _ { 0 } ( x ) \rightarrow \text { inf, } \quad f _ { i } ( x ) \leq 0 , \quad i = 1 , \ldots , m , \quad x \in B, $$ | conf 0.810
d034120555.png (555) | |
| 41.(74.)* | 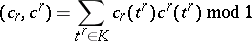 |
$( c _ { \gamma } , c ^ { r } ) = \sum _ { t ^ { r } \in K } c _ { r } ( t ^ { \prime } ) c ^ { r } ( t ^ { r } ) \operatorname { mod } 1$ | $$ ( c _ { \gamma } , c ^ { r } ) = \sum _ { t ^ { r } \in K } c _ { r } ( t ^ { \prime } ) c ^ { r } ( t ^ { r } ) \operatorname { mod } 1 $$ | conf 0.117 F
d03412079.png (79) |
Extension of a differential field
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 42.(63.) | 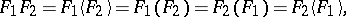 |
$F _ { 1 } F _ { 2 } = F _ { 1 } \langle F _ { 2 } \rangle = F _ { 1 } ( F _ { 2 } ) = F _ { 2 } ( F _ { 1 } ) = F _ { 2 } \langle F _ { 1 } \rangle$ | $$ F _ { 1 } F _ { 2 } = F _ { 1 } \langle F _ { 2 } \rangle = F _ { 1 } ( F _ { 2 } ) = F _ { 2 } ( F _ { 1 } ) = F _ { 2 } \langle F _ { 1 } \rangle, $$ | conf 0.628
e03696024.png (24) |
Formal group
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 43.(120.)* | 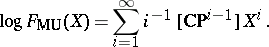 |
$\operatorname { og } F _ { MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ C ^ { - } P ^ { - 1 } ] X ^ { i }$ | $$ \operatorname { log } F _ {\rm MU } ( X ) = \sum _ { i = 1 } ^ { \infty } i ^ { - 1 } [ {\rm CP} ^ {i - 1 } ] X ^ { i }, $$ | conf 0.098 F
f040820118.png (118) |
| 44.(147.)* | 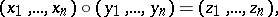 |
$( x _ { 1 } , \ldots , x _ { x } ) \circ ( y _ { 1 } , \ldots , y _ { n } ) = ( z _ { 1 } , \ldots , z _ { x } )$ | $$ ( x _ { 1 } , \ldots , x _ { n } ) \circ ( y _ { 1 } , \ldots , y _ { n } ) = ( z _ { 1 } , \ldots , z _ { n } ), $$ | conf 0.553 F
f04082059.png (59) |
Gel'fond-Schneider method
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 45.(148.) |  |
$\alpha ^ { \beta } = \operatorname { exp } \{ \beta \operatorname { log } \alpha \}$ | $$\empty$$ | conf 0.979
g1300205.png (5) |
Group
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 46.(22.)* | 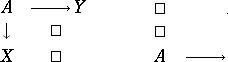 |
$\left. \begin{array} { l l l } { A } & { \rightarrow Y } & { \square } \\ { \downarrow } & { \square } & { } & { \square } \\ { X } & { \square } & { } & { A } \end{array} \right.$ | $$\empty$$ | conf 0.226 F
g04521075.png (75) |
Homogeneous space
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 47.(89.) | 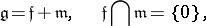 |
$\mathfrak { g } = \mathfrak { f } + \mathfrak { m } , \quad \mathfrak { f } \cap \mathfrak { m } = \{ 0 \}$ | $$\empty$$ | conf 0.793
h04769069.png (69) |
Hopf algebra
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 48.(103.) | 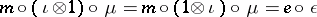 |
$m \circ ( \iota \otimes 1 ) \circ \mu = m \circ ( 1 \otimes \iota ) \circ \mu = e \circ \epsilon$ | $$\empty$$ | conf 0.618
h047970129.png (129) |
| 49.(107.)* |  |
$F _ { 1 } ( X || Y ) , \ldots , F _ { n } ( X || Y ) \in K [ X _ { 1 } , \ldots , X _ { n } || Y _ { 1 } , \ldots , Y _ { n } ] \}$ | $$\empty$$ | conf 0.353 F
h047970139.png (139) |
| 50.(97.) | 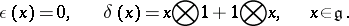 |
$\epsilon ( x ) = 0 , \quad \delta ( x ) = x \bigotimes 1 + 1 \bigotimes x , \quad x \in \mathfrak { g }$ | $$\empty$$ | conf 0.213
h04797042.png (42) |
Invariants, theory of
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 51.(149.)* |  |
$\alpha _ { 1 } , \ldots , i _ { R } \rightarrow \alpha _ { 2 } ^ { \prime } , \ldots , i _ { R }$ | $$\empty$$ | conf 0.142 F
i05235015.png (15) |
Jordan algebra
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 52.(150.) | 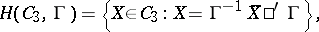 |
$H ( C _ { 3 } , \Gamma ) = \{ X \in C _ { 3 } : X = \Gamma ^ { - 1 } X \square ^ { \prime } \Gamma \}$ | $$\empty$$ | conf 0.651
j05427030.png (30) |
| 53.(42.) | 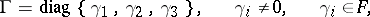 |
$\Gamma = \operatorname { diag } \{ \gamma _ { 1 } , \gamma _ { 2 } , \gamma _ { 3 } \} , \quad \gamma _ { i } \neq 0 , \quad \gamma _ { i } \in F$ | $$\empty$$ | conf 0.987
j05427031.png (31) |
| 54.(125.)* |  |
$\mathfrak { g } = \mathfrak { g } - 1 + \mathfrak { g } \mathfrak { d } + \mathfrak { g } _ { 1 }$ | $$\empty$$ | conf 0.598 F
j05427077.png (77) |
Jordan matrix
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 55.(6.)* |  |
$J = \left| \begin{array} { c c c c } { J _ { n _ { 1 } } ( \lambda _ { 1 } ) } & { \square } & { \square } & { \square } \\ { \square } & { \ldots } & { \square } & { 0 } \\ { 0 } & { \square } & { \ldots } & { \square } \\ { \square } & { \square } & { \square } & { J _ { n _ { S } } ( \lambda _ { s } ) } \end{array} \right|$ | $$J = \left\| \begin{array} { c c c c } J_{n_1}(\lambda_1) & 0 & 0 & 0 \\ 0 & \ddots & \ddots & 0 \\ 0 & \ddots & \ddots & 0 \\ 0 & 0 & 0 & J_{n_s}(\lambda_s) \end{array} \right\|,$$ | conf 0.072 F
j0543403.png (3) |
| 56.(64.) |  |
$C _ { m } ( \lambda ) = \operatorname { rk } ( A - \lambda E ) ^ { m - 1 } - 2 \operatorname { rk } ( A - \lambda E ) ^ { m } +$ | $$\empty$$ | conf 0.955
j05434030.png (30) |
| 57.(1.)* |  |
$J _ { m } ( \lambda ) = \| \begin{array} { c c c c c c } { \lambda } & { 1 } & { \square } & { \square } & { \square } & { \square } \\ { \square } & { \lambda } & { 1 } & { \square } & { 0 } & { \square } \\ { \square } & { \square } & { \cdots } & { \square } & { \square } & { \square } \\ { \square } & { \square } & { \square } & { \cdots } & { \square } & { \square } \\ { \square } & { 0 } & { \square } & { \square } & { \lambda } & { 1 } \\ { \square } & { \square } & { \square } & { \square } & { \square } & { \lambda } \end{array} ]$ | $$J_m(\lambda) = \left\| \begin{array} { c c c c c c } \lambda & 1 & \square & \square & \square & \square \\ \square & \lambda & 1 & \square & 0 & \square \\ \square & \square & \ddots & \ddots & \square & \square\\ \square & \square & \square & \ddots & \ddots & \square \\ \square & 0 & \square & \square & \lambda & 1 \\ \square & \square & \square & \square & \square & \lambda \end{array} \right\|,$$ | conf 0.098 F
j0543406.png (6) |
Lie algebra, semi-simple
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 58.(5.) | 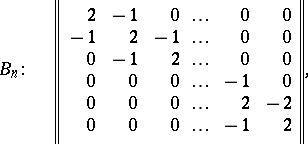 |
$\left\| \begin{array} { r r r r r r } { 2 } & { - 1 } & { 0 } & { \dots } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 1 } & { \dots } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { \dots } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 2 } & { - 2 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 2 } \end{array} \right\|$ | $$B_n: \quad \left\| \begin{array} { r r r r r r } { 2 } & { - 1 } & { 0 } & { \dots } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 1 } & { \dots } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { \dots } & { 0 } & { 0 } \\ \cdot & \cdot & \cdot & \dots & \cdot & \cdot \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 0 }\\ { 0 } & { 0 } & { 0 } & { \dots } & { 2 } & { - 2 }\\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 2 } \end{array} \right\|,$$ | conf 0.232
l058510127.png (127) |
| 59.(3.)* | 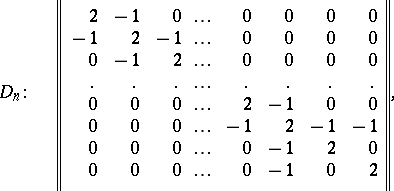 |
$\| \left. \begin{array} { r r r r r r r } { 2 } & { - 1 } & { 0 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 1 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 2 } & { - 1 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 2 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 0 } & { 2 } \end{array} \right. |$ | $$D_n: \quad \left\| \begin{array} { r r r r r r r } { 2 } & { - 1 } & { 0 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 1 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 }\\ { 0 } & { - 1 } & { 2 } & { \dots } & { 0 } & { 0 } & { 0 } & { 0 } \\ \cdot & \cdot & \cdot & \dots & \cdot & \cdot &\cdot & \cdot \\ { 0 } & { 0 } & { 0 } & { \dots } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { - 1 } & { 2 } & { - 1 } & { - 1 }\\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 2 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { \dots } & { 0 } & { - 1 } & { 0 } & { 2 } \end{array} \right\|,$$ | conf 0.055 F
l058510129.png (129) |
| 60.(8.)* | 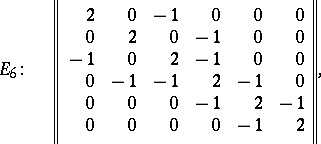 |
$\left\| \begin{array} { r r r r r r } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } \\ { - 1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\|$ | $$E_6: \quad \left\| \begin{array} { r r r r r r } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } \\ { - 1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\|,$$ | conf 0.628 F
l058510130.png (130) |
| 61.(4.) | 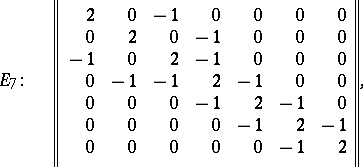 |
$\left\| \begin{array} { r r r r r r r } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\|$ | $$E_7: \quad \left\| \begin{array} { r r r r r r r } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ {-1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\|,$$ | conf 0.278
l058510131.png (131) |
| 62.(2.)* | 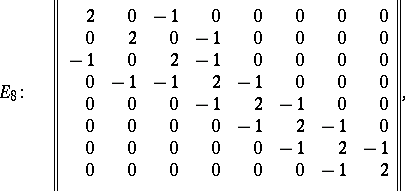 |
$\left. \begin{array} { r l l l l l l l } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right.$ | $$E_8: \quad \left\| \begin{array} { r r r r r r r r } { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 2 } & { 0 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ {-1 } & { 0 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { - 1 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } & { 0 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\|,$$ | conf 0.354 F
l058510132.png (132) |
| 63.(10.)* |  |
$\left\| \begin{array} { r r r r } { 2 } & { - 1 } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 2 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\| , \quad G _ { 2 } : \quad \left\| \begin{array} { r r } { 2 } & { - 1 } \\ { - 3 } & { 2 } \end{array} \right\|$ | $$F_4: \quad \left\| \begin{array} { r r r r } { 2 } & { - 1 } & { 0 } & { 0 } \\ { - 1 } & { 2 } & { - 2 } & { 0 } \\ { 0 } & { - 1 } & { 2 } & { - 1 } \\ { 0 } & { 0 } & { - 1 } & { 2 } \end{array} \right\| , \quad G _ { 2 } : \quad \left\| \begin{array} { r r } { 2 } & { - 1 } \\ { - 3 } & { 2 } \end{array} \right\|.$$ | conf 0.374 F
l058510133.png (133) |
| 64.(98.) | 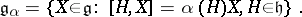 |
$\mathfrak { g } _ { \alpha } = \{ X \in \mathfrak { g } : [ H , X ] = \alpha ( H ) X , H \in \mathfrak { h } \}$ | $$\empty$$ | conf 0.976
l05851030.png (30) |
| 65.(126.) |  |
$\mathfrak { g } = \mathfrak { h } + \sum _ { \alpha \in \Sigma } \mathfrak { g } _ { \alpha }$ | $$\empty$$ | conf 0.945
l05851037.png (37) |
| 66.(61.)* | 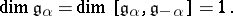 |
$\mathfrak { g } _ { \alpha } = \operatorname { dim } [ \mathfrak { g } _ { \alpha } , \mathfrak { g } _ { - \alpha } ] = 1$ | $$\empty$$ | conf 0.520 F
l05851044.png (44) |
| 67.(65.)* | 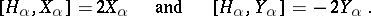 |
$[ H _ { \alpha } , X _ { \alpha } ] = 2 X _ { \alpha } \quad \text { and } \quad [ H _ { \alpha } , Y _ { \alpha } ] = - 2 Y _ { 0 }$ | $$\empty$$ | conf 0.539 F
l05851050.png (50) |
| 68.(70.) |  |
$\beta ( H _ { \alpha } ) = \frac { 2 ( \alpha , \beta ) } { ( \alpha , \alpha ) } , \quad \alpha , \beta \in \Sigma$ | $$\empty$$ | conf 0.997
l05851051.png (51) |
| 69.(112.) |  |
$[ \mathfrak { g } _ { \alpha } , \mathfrak { g } _ { \beta } ] = \mathfrak { g } _ { \alpha + \beta }$ | $$\empty$$ | conf 0.917
l05851057.png (57) |
| 70.(127.) | 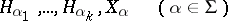 |
$H _ { \alpha _ { 1 } } , \ldots , H _ { \alpha _ { k } } , X _ { \alpha } \quad ( \alpha \in \Sigma )$ | $$\empty$$ | conf 0.432
l05851064.png (64) |
| 71.(113.)* | 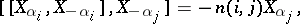 |
$[ [ X _ { \alpha _ { i } } , X _ { - } \alpha _ { i } ] , X _ { - \alpha _ { j } } ] = - n ( i , j ) X _ { \alpha _ { j } }$ | $$\empty$$ | conf 0.628 F
l05851069.png (69) |
| 72.(79.) | 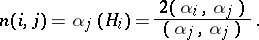 |
$n ( i , j ) = \alpha _ { j } ( H _ { i } ) = \frac { 2 ( \alpha _ { i } , \alpha _ { j } ) } { ( \alpha _ { j } , \alpha _ { j } ) }$ | $$\empty$$ | conf 0.992
l05851073.png (73) |
| 73.(13.) | 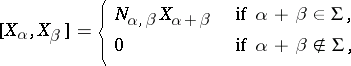 |
$[ X _ { \alpha } , X _ { \beta } ] = \left\{ \begin{array} { l l } { N _ { \alpha , \beta } X _ { \alpha + \beta } } & { \text { if } \alpha + \beta \in \Sigma } \\ { 0 } & { \text { if } \alpha + \beta \notin \Sigma } \end{array} \right.$ | $$\empty$$ | conf 0.988
l05851074.png (74) |
| 74.(80.) | 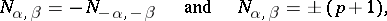 |
$N _ { \alpha , \beta } = - N _ { - \alpha , - \beta } \quad \text { and } \quad N _ { \alpha , \beta } = \pm ( p + 1 )$ | $$\empty$$ | conf 0.961
l05851078.png (78) |
| 75.(85.)* | 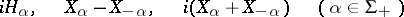 |
$X _ { \alpha } - X _ { - \alpha } , \quad i ( X _ { \alpha } + X _ { - \alpha } ) \quad ( \alpha \in \Sigma _ { + } )$ | $$\empty$$ | conf 0.691 F
l05851085.png (85) |
Lie algebra, solvable
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 76.(119.)* | 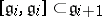 |
$[ \mathfrak { g } _ { i } , \mathfrak { g } _ { i } ] \subset \mathfrak { g } _ { \mathfrak { i } } + 1$ | $$\empty$$ | conf 0.276 F
l05852011.png (11) |
| 77.(141.) | 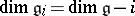 |
$\operatorname { dim } \mathfrak { g } _ { i } = \operatorname { dim } \mathfrak { g } - i$ | $$\empty$$ | conf 0.901
l05852046.png (46) |
Lie group
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 78.(62.)* | 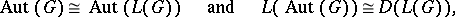 |
$( G ) \cong \operatorname { Aut } ( L ( G ) ) \quad \text { and } \quad L ( \operatorname { Aut } ( G ) ) \cong D ( L ( G ) )$ | $$\empty$$ | conf 0.693 F
l058590115.png (115) |
| 79.(50.) |  |
$( X , Y ) \rightarrow \operatorname { exp } ^ { - 1 } ( \operatorname { exp } X \operatorname { exp } Y ) , \quad X , Y \in L ( G )$ | $$\empty$$ | conf 0.856
l05859086.png (86) |
Lie group, compact
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 80.(121.)* |  |
$J = \left\| \begin{array} { c c } { 0 } & { E _ { x } } \\ { - E _ { x } } & { 0 } \end{array} \right\|$ | $$\empty$$ | conf 0.364 F
l05861012.png (12) |
Lie group, nilpotent
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 81.(83.) |  |
$N ( F ) = \{ g \in GL ( V ) : g v \equiv v \operatorname { mod } V _ { i } \text { for all } v \in V _ { i } , i \geq 1 \}$ | $$\empty$$ | conf 0.466
l0586604.png (4) |
Lie group, semi-simple
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 82.(35.)* |  |
$L ( \mathfrak { g } ) \cong \Gamma _ { 0 } ( \mathfrak { u } ) \cap \mathfrak { h } ^ { \prime } / \Gamma _ { 0 } ( [ \mathfrak { k } , \mathfrak { k } ] )$ | $$\empty$$ | conf 0.659 F
l058680102.png (102) |
| 83.(81.)* | 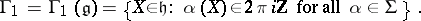 |
$\Gamma _ { 1 } = \Gamma _ { 1 } ( g ) = \{ X \in h : \alpha ( X ) \in 2 \pi i Z \text { for all } \alpha \in \Sigma \}$ | $$\empty$$ | conf 0.183 F
l05868032.png (32) |
Lie p-algebra
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 84.(36.) | 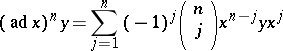 |
$( \operatorname { ad } x ) ^ { n } y = \sum _ { j = 1 } ^ { n } ( - 1 ) ^ { j } \left( \begin{array} { c } { n } \\ { j } \end{array} \right) x ^ { n - j } y x ^ { j }$ | $$\empty$$ | conf 0.356
l05872026.png (26) |
| 85.(99.) |  |
$\pi ( x + y ) = \pi ( x ) + \pi ( y ) , \quad \pi ( \lambda x ) = \lambda ^ { p } \pi ( x ) , \quad \lambda \in k$ | $$\empty$$ | conf 0.964
l05872078.png (78) |
Lie theorem
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 86.(134.) | 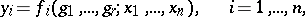 |
$y _ { i } = f _ { i } ( g _ { 1 } , \ldots , g _ { i } || x _ { 1 } , \ldots , x _ { n } ) , \quad i = 1 , \ldots , n$ | $$\empty$$ | conf 0.276
l05876010.png (10) |
| 87.(86.) | 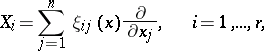 |
$X _ { i } = \sum _ { j = 1 } ^ { n } \xi _ { i j } ( x ) \frac { \partial } { \partial x _ { j } } , \quad i = 1 , \ldots , r$ | $$\empty$$ | conf 0.656
l05876016.png (16) |
| 88.(66.)* | 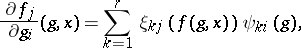 |
$\frac { \partial f _ { j } } { \partial g _ { i } } ( g , x ) = \sum _ { k = 1 } ^ { r } \xi _ { k j } ( f ( g _ { s } x ) ) \psi _ { k i } ( g )$ | $$\empty$$ | conf 0.336 F
l05876030.png (30) |
| 89.(19.)* | 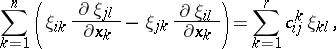 |
$\sum _ { k = 1 } ^ { N } ( \xi _ { i k } \frac { \partial \xi _ { j l } } { \partial x _ { k } } - \xi _ { j k } \frac { \partial \xi _ { i l } } { \partial x _ { k } } ) = \sum _ { k = 1 } ^ { r } c _ { i j } ^ { k } \xi _ { k l }$ | $$\empty$$ | conf 0.157 F
l05876037.png (37) |
| 90.(14.) | 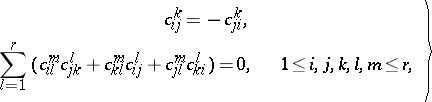 |
$\left. \begin{array} { c } { c _ { i j } ^ { k } = - c _ { j i } ^ { k } } \\ { \sum _ { l = 1 } ^ { r } ( c _ { i l } ^ { m } c _ { j k } ^ { l } + c _ { k l } ^ { m } c _ { i j } ^ { l } + c _ { j l } ^ { m } c _ { k i } ^ { l } ) = 0 , \quad 1 \leq i , j , k , l , m \leq r } \end{array} \right.$ | $$\empty$$ | conf 0.085
l05876052.png (52) |
Maximal torus
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 91.(95.) | 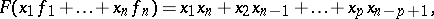 |
$F ( x _ { 1 } f _ { 1 } + \ldots + x _ { x } f _ { n } ) = x _ { 1 } x _ { n } + x _ { 2 } x _ { n } - 1 + \ldots + x _ { p } x _ { n } - p + 1$ | $$\empty$$ | conf 0.198
m06301072.png (72) |
Non-Abelian cohomology
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 92.(114.)* | 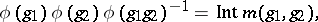 |
$\phi ( g _ { 1 } ) \phi ( g ) \phi ( g _ { 1 } g _ { 2 } ) ^ { - 1 } = \operatorname { Int } m ( g _ { 1 } , g _ { 2 } )$ | $$\empty$$ | conf 0.443 F
n066900110.png (110) |
| 93.(90.)* | 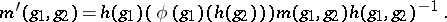 |
$( g _ { 1 } , g _ { 2 } ) = h ( g _ { 1 } ) ( \phi ( g _ { 1 } ) ( h ( g _ { 2 } ) ) ) m ( g _ { 1 } , g _ { 2 } ) h ( g _ { 1 } , g _ { 2 } ) ^ { - 1 }$ | $$\empty$$ | conf 0.764 F
n066900118.png (118) |
| 94.(44.) | 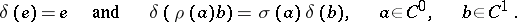 |
$\delta ( e ) = e \quad \text { and } \quad \delta ( \rho ( a ) b ) = \sigma ( a ) \delta ( b ) , \quad \alpha \in C ^ { 0 } , \quad b \in C ^ { 1 }$ | $$\empty$$ | conf 0.400
n06690016.png (16) |
| 95.(60.)* | 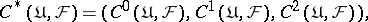 |
$C ^ { * } ( \mathfrak { U } , F ) = ( C ^ { 0 } ( \mathfrak { U } , F ) , C ^ { 1 } ( \mathfrak { U } , F ) , C ^ { 2 } ( \mathfrak { U } , F ) )$ | $$\empty$$ | conf 0.205 F
n06690028.png (28) |
Picard scheme
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 96.(39.)* | 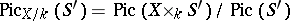 |
$\operatorname { Pic } _ { X / k } ( S ^ { \prime } ) = \operatorname { Fic } ( X \times k S ^ { \prime } ) / \operatorname { Fic } ( S ^ { \prime } )$ | $$\empty$$ | conf 0.345 F +
p07267025.png (25) |
Principal analytic fibration
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 97.(100.)* | 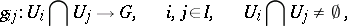 |
$g j : U _ { i } \cap U _ { j } \rightarrow G , \quad i , j \in I , \quad U _ { i } \cap U _ { j } \neq \emptyset$ | $$\empty$$ | conf 0.184 F
p07464025.png (25) |
Quantum groups
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 98.(101.) |  |
$\phi ^ { * } : \mathfrak { g } ^ { * } \otimes \mathfrak { g } ^ { * } \rightarrow \mathfrak { g } ^ { * }$ | $$\empty$$ | conf 0.837
q07631062.png (62) |
| 99.(108.) | 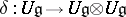 |
$\delta : U _ { \mathfrak { g } } \rightarrow U _ { \mathfrak { g } } \otimes U _ { \mathfrak { g } }$ | $$\empty$$ | conf 0.648
q07631071.png (71) |
| 100.(56.)* | 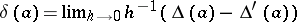 |
$\delta ( \alpha ) = \operatorname { lim } _ { h \rightarrow 0 } h ^ { - 1 } ( \Delta ( a ) - \Delta ^ { \prime } ( \alpha ) )$ | $$\empty$$ | conf 0.304 F
q07631072.png (72) |
| 101.(129.)* | 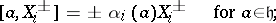 |
$[ \alpha , X _ { i } ^ { \pm } ] = \pm \alpha _ { i } ( \alpha ) X _ { i } ^ { \pm } \quad \text { for } a$ | $$\empty$$ | conf 0.544 F
q07631088.png (88) |
| 102.(128.) |  |
$[ X _ { i } ^ { + } , X _ { j } ^ { - } ] = 2 \delta _ { i j } h ^ { - 1 } \operatorname { sinh } ( h H _ { i } / 2 )$ | $$\empty$$ | conf 0.893
q07631089.png (89) |
| 103.(20.) | 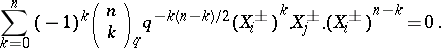 |
$\sum _ { k = 0 } ^ { n } ( - 1 ) ^ { k } \left( \begin{array} { l } { n } \\ { k } \end{array} \right) q ^ { - k ( n - k ) / 2 } ( X _ { i } ^ { \pm } ) ^ { k } X _ { j } ^ { \pm } \cdot ( X _ { i } ^ { \pm } ) ^ { n - k } = 0$ | $$\empty$$ | conf 0.055
q07631092.png (92) |
| 104.(30.) |  |
$\left( \begin{array} { l } { n } \\ { k } \end{array} \right) _ { q } = \frac { ( q ^ { n } - 1 ) \ldots ( q ^ { n - k + 1 } - 1 ) } { ( q ^ { k } - 1 ) \ldots ( q - 1 ) }$ | $$\empty$$ | conf 0.443
q07631095.png (95) |
| 105.(21.)* |  |
$\Delta ( X _ { i } ^ { \pm } ) = X _ { i } ^ { \pm } \bigotimes \operatorname { exp } ( \frac { h H _ { i } } { 4 } ) + \operatorname { exp } ( \frac { - h H _ { i } } { 4 } ) \otimes x _ { i } ^ { \pm }$ | $$\empty$$ | conf 0.212 F
q07631099.png (99) |
Rational representation
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 106.(91.) | 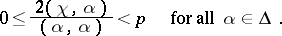 |
$0 \leq \frac { 2 ( \chi , \alpha ) } { ( \alpha , \alpha ) } < p \quad \text { for all } \alpha \in \Delta$ | $$\empty$$ | conf 0.879
r077630100.png (100) |
| 107.(135.) |  |
$\phi _ { 0 } \bigotimes \phi _ { 1 } ^ { Fr } \otimes \ldots \otimes \phi _ { d } ^ { FF ^ { d } }$ | $$\empty$$ | conf 0.136
r077630104.png (104) |
| 108.(45.)* | 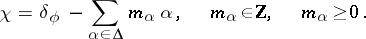 |
$\chi = \delta _ { \phi } - \sum _ { \alpha \in \Delta } m _ { \alpha } \alpha , \quad m _ { \alpha } \in Z , \quad m _ { \alpha } \geq 0$ | $$\empty$$ | conf 0.862 F
r07763055.png (55) |
Singular point
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 109.(31.) | 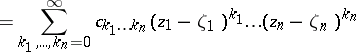 |
$\sum _ { k _ { 1 } , \ldots , k _ { n } = 0 } ^ { \infty } c _ { k _ { 1 } \cdots k _ { n } } ( z _ { 1 } - \zeta _ { 1 } ) ^ { k _ { 1 } } \ldots ( z _ { n } - \zeta _ { n } ) ^ { k _ { n } }$ | $$\empty$$ | conf 0.324
s085590225.png (225) |
| 110.(46.) | 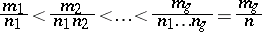 |
$\frac { m _ { 1 } } { n _ { 1 } } < \frac { m _ { 2 } } { n _ { 1 } n _ { 2 } } < \ldots < \frac { m _ { g } } { n _ { 1 } \ldots n _ { g } } = \frac { m _ { g } } { n }$ | $$\empty$$ | conf 0.459
s085590404.png (404) |
| 111.(115.)* | 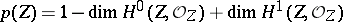 |
$p ( Z ) = 1 - \operatorname { dim } H ^ { 0 } ( Z , O _ { Z } ) + \operatorname { dim } H ^ { 1 } ( Z , O _ { Z } )$ | $$\empty$$ | conf 0.997 F
s085590429.png (429) |
| 112.(136.)* | 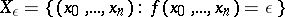 |
$X _ { \epsilon } = \{ ( x _ { 0 } , \ldots , x _ { x } ) : f ( x _ { 0 } , \ldots , x _ { x } ) = \epsilon \}$ | $$\empty$$ | conf 0.433 F
s085590440.png (440) |
| 113.(12.) |  |
$= \left\{ \begin{array} { l l } { ( x + \lambda ) ^ { 2 } \ldots ( x + k \lambda ) ^ { 2 } } & { \text { if } \mu = 2 k } \\ { ( x + \lambda ) ^ { 2 } \ldots ( x + k \lambda ) ^ { 2 } ( x + ( k + 1 ) \lambda ) } & { \text { if } \mu = 2 k + 1 } \end{array} \right.$ | $$\empty$$ | conf 0.870
s085590458.png (458) |
| 114.(75.) |  |
$( \frac { \partial F ( x , y , \lambda ) } { \partial x } , \frac { \partial F ( x , y , \lambda ) } { \partial y } )$ | $$\empty$$ | conf 0.986
s085590482.png (482) |
| 115.(137.) | 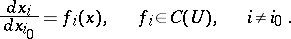 |
$\frac { d x _ { i } } { d x _ { i _ { 0 } } } = f _ { i } ( x ) , \quad f _ { i } \in C ( U ) , \quad i \neq i _ { 0 }$ | $$\empty$$ | conf 0.594
s085590515.png (515) |
| 116.(142.)* | 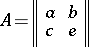 |
$A = \| \left. \begin{array} { l l } { \alpha } & { b } \\ { c } & { e } \end{array} \right. |$ | $$\empty$$ | conf 0.506 F
s085590527.png (527) |
| 117.(53.) | 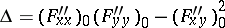 |
$\Delta = ( F _ { x x } ^ { \prime \prime } ) _ { 0 } ( F _ { y y } ^ { \prime \prime } ) _ { 0 } - ( F _ { x y } ^ { \prime \prime } ) _ { 0 } ^ { 2 }$ | $$\empty$$ | conf 0.920
s085590634.png (634) |
| 118.(16.)* | 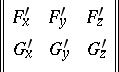 |
$\left| \begin{array} { l l l } { F _ { X } ^ { \prime } } & { F _ { y } ^ { \prime } } & { F _ { z } ^ { \prime } } \\ { G _ { \chi } ^ { \prime } } & { G _ { y } ^ { \prime } } & { G _ { Z } ^ { \prime } } \end{array} \right|$ | $$\empty$$ | conf 0.230 F
s085590645.png (645) |
| 119.(92.) | 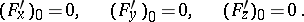 |
$( F _ { X } ^ { \prime } ) _ { 0 } = 0 , \quad ( F _ { y } ^ { \prime } ) _ { 0 } = 0 , \quad ( F _ { z } ^ { \prime } ) _ { 0 } = 0$ | $$\empty$$ | conf 0.300
s085590653.png (653) |
Solv manifold
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 120.(138.) | 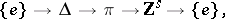 |
$\{ e \} \rightarrow \Delta \rightarrow \pi \rightarrow Z ^ { s } \rightarrow \{ e \}$ | $$\empty$$ | conf 0.972
s08610054.png (54) |
Stability theorems in algebraic K-theory
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 121.(71.) | 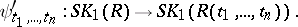 |
$\psi _ { t _ { 1 } , \ldots , t _ { R } } ^ { \prime } : S K _ { 1 } ( R ) \rightarrow S K _ { 1 } ( R ( t _ { 1 } , \ldots , t _ { n } ) )$ | $$\empty$$ | conf 0.379
s08706033.png (33) |
Steinberg module
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 122.(130.) | 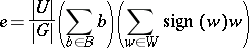 |
$e = \frac { | U | } { | G | } ( \sum _ { b \in B } b ) ( \sum _ { w \in W } \operatorname { sign } ( w ) w )$ | $$\empty$$ | conf 0.138
s13053016.png (16) |
Steinberg symbol
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 123.(24.)* |  |
$( x _ { i j } ( a ) , x _ { k l } ( b ) ) = \left\{ \begin{array} { l l } { 1 } & { \text { if } i \neq 1 , j \neq k } \\ { x _ { 1 } ( a b ) } & { \text { if } i \neq 1 , j = k } \end{array} \right.$ | $$\empty$$ | conf 0.381 F
s13054017.png (17) |
Tilting theory
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 124.(84.) |  |
$0 \rightarrow \Lambda \rightarrow T _ { 1 } \rightarrow \ldots \rightarrow T _ { n } \rightarrow 0$ | $$\empty$$ | conf 0.946
t130130105.png (105) |
Tits quadratic form
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 125.(18.) | 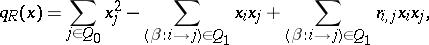 |
$q R ( x ) = \sum _ { j \in Q _ { 0 } } x _ { j } ^ { 2 } - \sum _ { \langle \beta : i \rightarrow j ) \in Q _ { 1 } } x _ { i } x _ { j } + \sum _ { \langle \beta : i \rightarrow j ) \in Q _ { 1 } } x _ { , j } x _ { i } x _ { j }$ | $$\empty$$ | conf 0.112
t130140104.png (104) |
| 126.(40.) | 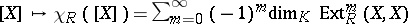 |
$[ X ] \mapsto \chi _ { R } ( [ X ] ) = \sum _ { m = 0 } ^ { \infty } ( - 1 ) ^ { m } \operatorname { dim } _ { K } \operatorname { Ext } _ { R } ^ { m } ( X , X )$ | $$\empty$$ | conf 0.116
t130140118.png (118) |
| 127.(132.)* | 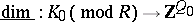 |
$\operatorname { dim } _ { 1 } : K _ { 0 } ( \operatorname { mod } R ) \rightarrow Z ^ { Q _ { 0 } }$ | $$\empty$$ | conf 0.287 F
t130140119.png (119) |
| 128.(37.)* | 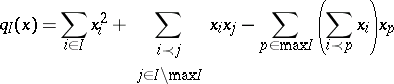 |
$q ( x ) = \sum _ { i \in I } x _ { i } ^ { 2 } + \sum _ { i \prec j } x _ { i } x _ { j } - \sum _ { p \in \operatorname { max } l } ( \sum _ { i \prec p } x _ { i } ) x _ { p }$ | $$\empty$$ | conf 0.197 F
t130140140.png (140) |
| 129.(131.)* | 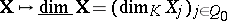 |
$X \mapsto \operatorname { dim } X = ( \operatorname { dim } _ { K } X _ { j } ) _ { j \in Q _ { 0 } }$ | $$\empty$$ | conf 0.819 F
t13014044.png (44) |
| 130.(25.) | 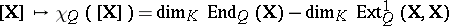 |
$[ X ] \mapsto \chi _ { Q } ( [ X ] ) = \operatorname { dim } _ { K } \operatorname { End } _ { Q } ( X ) - \operatorname { dim } _ { K } \operatorname { Ext } _ { Q } ^ { 1 } ( X , X )$ | $$\empty$$ | conf 0.661
t13014048.png (48) |
| 131.(38.)* | 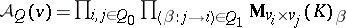 |
$A _ { Q } ( v ) = \prod _ { i , j \in Q _ { 0 } } \prod _ { \langle \beta : j \rightarrow i \rangle \in Q _ { 1 } } M _ { v _ { i } \times v _ { j } } ( K ) _ { \beta }$ | $$\empty$$ | conf 0.481 F
t13014056.png (56) |
| 132.(139.)* |  |
$\Phi ( x ) = \sum _ { j \in Q _ { 0 } } x _ { j } ^ { 2 } - \sum _ { i , j \in Q _ { 0 } } d _ { i j } x _ { i } x _ { j }$ | $$\empty$$ | conf 0.648 F
t1301406.png (6) |
Torus
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 133.(41.)* | 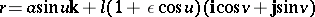 |
$r = \alpha \operatorname { sin } u k + l ( 1 + \epsilon \operatorname { cos } u ) ( i \operatorname { cos } v + j \operatorname { sin } v )$ | $$\empty$$ | conf 0.585 F
t0933502.png (2) |
| 134.(122.)* | 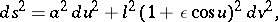 |
$d s ^ { 2 } = \alpha ^ { 2 } d u ^ { 2 } + l ^ { 2 } ( 1 + \epsilon \operatorname { cos } u ) ^ { 2 } d v ^ { 2 }$ | $$\empty$$ | conf 0.696 F
t0933507.png (7) |
Uniform distribution
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 135.(9.) |  |
$u _ { 3 } ( x ) = \left\{ \begin{array} { l l } { \frac { x ^ { 2 } } { 2 } , } & { 0 \leq x < 1 } \\ { \frac { [ x ^ { 2 } - 3 ( x - 1 ) ^ { 2 } ] } { 2 } , } & { 1 \leq x < 2 } \\ { \frac { [ x ^ { 2 } - 3 ( x - 1 ) ^ { 2 } + 3 ( x - 2 ) ^ { 2 } ] } { 2 } , } & { 2 \leq x < 3 } \\ { 0 , } & { x \notin [ 0,3 ] } \end{array} \right.$ | $$\empty$$ | conf 0.733
u09524027.png (27) |
| 136.(32.)* | 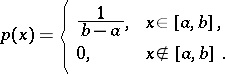 |
$p ( x ) = \left\{ \begin{array} { l l } { \frac { 1 } { b - \alpha } , } & { x \in [ \alpha , b ] } \\ { 0 , } & { x \notin [ \alpha , b ] } \end{array} \right.$ | $$\empty$$ | conf 0.681 F
u0952403.png (3) |
| 137.(34.) | 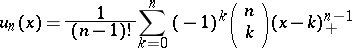 |
$u _ { n } ( x ) = \frac { 1 } { ( n - 1 ) ! } \sum _ { k = 0 } ^ { n } ( - 1 ) ^ { k } \left( \begin{array} { l } { n } \\ { k } \end{array} \right) ( x - k ) _ { + } ^ { n - 1 }$ | $$\empty$$ | conf 0.569
u09524030.png (30) |
| 138.(109.) | 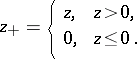 |
$z _ { + } = \left\{ \begin{array} { l l } { z , } & { z > 0 } \\ { 0 , } & { z \leq 0 } \end{array} \right.$ | $$\empty$$ | conf 0.676
u09524034.png (34) |
| 139.(43.) | 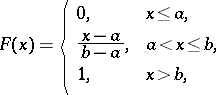 |
$F ( x ) = \left\{ \begin{array} { l l } { 0 , } & { x \leq a } \\ { \frac { x - a } { b - a } , } & { a < x \leq b } \\ { 1 , } & { x > b } \end{array} \right.$ | $$\empty$$ | conf 0.468
u0952407.png (7) |
| 140.(47.) |  |
$p ( x _ { 1 } , \ldots , x _ { n } ) = \left\{ \begin{array} { l l } { C \neq 0 , } & { x \in D } \\ { 0 , } & { x \notin D } \end{array} \right.$ | $$\empty$$ | conf 0.705
u09524072.png (72) |
Unipotent group
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 141.(143.) | 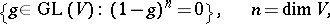 |
$\{ g \in \operatorname { GL } ( V ) : ( 1 - g ) ^ { n } = 0 \} , \quad n = \operatorname { dim } V$ | $$\empty$$ | conf 0.287
u0954106.png (6) |
Weyl module
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 142.(51.) | 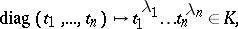 |
$\operatorname { diag } ( t _ { 1 } , \ldots , t _ { n } ) \mapsto t _ { 1 } ^ { \lambda _ { 1 } } \ldots t _ { n } ^ { \lambda _ { n } } \in K$ | $$\empty$$ | conf 0.507
w120090122.png (122) |
| 143.(54.)* |  |
$\chi _ { \lambda } = \sum _ { \mu \in \Lambda ( n ) } \operatorname { dim } _ { K } ( \Delta ( \lambda ) ^ { \mu } ) _ { e _ { \mu } }$ | $$\empty$$ | conf 0.461 F
w120090135.png (135) |
| 144.(110.) |  |
$\mathfrak { B } = \{ e _ { \pm } \alpha , h _ { \beta } : \alpha \in \Phi ^ { + } , \beta \in \Sigma \}$ | $$\empty$$ | conf 0.381
w120090259.png (259) |
| 145.(82.) |  |
$\left( \begin{array} { c } { h } \\ { i } \end{array} \right) = \frac { h ( h - 1 ) \ldots ( h - i + 1 ) } { i ! }$ | $$\empty$$ | conf 0.487
w120090342.png (342) |
| 146.(28.)* | 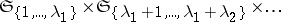 |
$\mathfrak { S } _ { \{ 1 , \ldots , \lambda _ { 1 } \} } \times \mathfrak { S } _ { \{ \lambda _ { 1 } + 1 , \ldots , \lambda _ { 1 } + \lambda _ { 2 } \} } \times$ | $$\empty$$ | conf 0.312 F
w12009095.png (95) |
| 147.(104.) |  |
$\ldots \times \mathfrak { S } _ { \{ \lambda _ { 1 } + \ldots + \lambda _ { n - 1 } + 1 , \ldots , r \} }$ | $$\empty$$ | conf 0.259
w12009096.png (96) |
Witt vector
| Nr. | Image of png File | $\TeX$, 1st version | $\TeX$, corrected version | Confidence, F?
png file |
|---|---|---|---|---|
| 148.(87.)* | 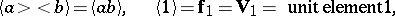 |
$\langle \alpha > < b \rangle = \langle \alpha b \rangle , \quad \langle 1 \rangle = f _ { 1 } = V _ { 1 } =$ | $$\empty$$ | conf 0.351 F
w098100172.png (172) |
| 149.(123.)* |  |
$\langle \alpha + b \rangle = \sum _ { n = 1 } ^ { \infty } V _ { n } \langle r _ { n } ( \alpha , b ) f$ | $$\empty$$ | conf 0.143 F
w098100177.png (177) |
| 150.(102.) | 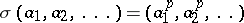 |
$\sigma ( \alpha _ { 1 } , \alpha _ { 2 } , \ldots ) = ( \alpha _ { 1 } ^ { p } , \alpha _ { 2 } ^ { p } , \ldots )$ | $$\empty$$ | conf 0.771
w098100190.png (190) |
Ulf Rehmann/Table of automatically generated TeX code. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Ulf_Rehmann/Table_of_automatically_generated_TeX_code&oldid=44184