Difference between revisions of "Cardinal characteristic"
m (typo) |
m (→Comments: links) |
||
| Line 58: | Line 58: | ||
The Suslin number of a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360173.png" /> is also called its cellularity, and its Lindelöf number also its Lindelöf degree (the latter is often denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360174.png" />). | The Suslin number of a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360173.png" /> is also called its cellularity, and its Lindelöf number also its Lindelöf degree (the latter is often denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360174.png" />). | ||
| − | The Urysohn–Tikhonov theorem, mentioned above, is also called Urysohn | + | The Urysohn–Tikhonov theorem, mentioned above, is also called the [[Urysohn metrization theorem]]. |
The fact that the class of dyadic compacta contains the class of compact Hausdorff topological groups is called Kuzminov's theorem (on compact groups). | The fact that the class of dyadic compacta contains the class of compact Hausdorff topological groups is called Kuzminov's theorem (on compact groups). | ||
| − | For the notion of a Fréchet–Urysohn space see [[ | + | For the notion of a Fréchet–Urysohn space see [[Sequential space]]. |
The problem of whether <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360175.png" /> for every space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360176.png" /> was solved by S. Todorčević [[#References|[a2]]], who found, without using extra set-theoretic hypotheses, spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360177.png" /> satisfying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360178.png" />. | The problem of whether <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360175.png" /> for every space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360176.png" /> was solved by S. Todorčević [[#References|[a2]]], who found, without using extra set-theoretic hypotheses, spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360177.png" /> satisfying <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360178.png" />. | ||
| − | The problems whether every hereditarily separable space is Lindelöf and whether every hereditarily Lindelöf space is separable generated a lot of research. Many examples were constructed using various extra set-theoretical assumptions, in particular the [[Continuum hypothesis| | + | The problems whether every hereditarily separable space is Lindelöf and whether every hereditarily Lindelöf space is separable generated a lot of research. Many examples were constructed using various extra set-theoretical assumptions, in particular the [[Continuum hypothesis|Continuum Hypothesis]]. Todorcevi<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c020/c020360/c020360179.png" /> [[#References|[a1]]] showed that the statement "every hereditarily separable space is Lindelöf" is consistent with the usual axioms of set theory. For much more information and other recent developments see various articles in [[#References|[a3]]], in particular Chapts. 1; 2, and [[#References|[a4]]]. |
====References==== | ====References==== | ||
Revision as of 17:59, 1 January 2018
2020 Mathematics Subject Classification: Primary: 54A25 [MSN][ZBL]
of a topological space
A function associating an infinite cardinal number to each space and taking the same value on homeomorphic spaces. Cardinal characteristics are also called cardinal invariants. The domain of definition of a cardinal invariant is the class of all topological spaces or some subclass of it. The following cardinal invariants arose at the first stage of development of general topology. Let $X$ be an arbitrary topological space. A trivial invariant is its cardinality $|X|$, i.e. the cardinality of the set of all its points. Its weight $w(X)$ is the smallest cardinality of a base of $X$. The density $d(X)$ is the smallest cardinality of a dense subset of $X$. The Suslin number $c(X)$ is the least infinite cardinal number $\mathfrak{t}$ such that the cardinality of every family of pairwise-disjoint non-empty open sets does not exceed $\mathfrak{t}$. The Lindelöf number $l(X)$ is the least infinite cardinal number $\mathfrak{t}$ such that every open covering of $X$ has a subcovering of cardinality $\le\mathfrak{t}$. These simple notions immediately showed their importance by entering in a decisive way in fundamental theorems and problems. Examples: a regular space of countable weight is metrizable (the Urysohn–Tikhonov theorem, 1925); a compact Hausdorff space is metrizable if and only if its weight is countable; the Suslin number of the space $X$ of a compact group is countable; for every space $X$ of countable weight its Lindelöf number $l(X)$ is countable. The Suslin problem — is it true that every ordered connected compact Hausdorff space $X$ for which $c(X)=\aleph_0$ is homeomorphic to the interval $[0,1]$ — leads to the question of the relationship between two cardinal invariants: the density and the Suslin number. For a positive solution of Suslin's problem it is sufficient to show, under the above assumptions, that $d(X) \le c(X)$. The question of comparison of cardinal invariants — the solution of which, as is clear from the above example, may be of key significance for a definitive conclusion on the structure of a space — is central in the theory of cardinal invariants. The reason for this lies in the very nature of the concept of a cardinal invariant: the values of a cardinal invariant are cardinal numbers, the class of which is well-ordered by magnitude. Consequently, one can try to compare the values of any two cardinal invariants $\phi_1$ and $\phi_2$. A series of mutually related questions arises. Is it true that for all $X$, $\phi_1(X) \le \phi_2(X)$; for which $X$ does $\phi_1(X) \le \phi_2(X)$ hold; when is $\phi_1(X) = \phi_2(X)$, etc.
It is possible to do arithmetic with cardinals: to multiply and to add them, and to raise them to a power. Correspondingly, it is possible to do arithmetic with cardinal invariants — to multiply and to add them as functions, etc. This opens up new possibilities for comparing cardinal invariants, using arithmetic. There always holds $$ c(X) \le d(X) \le w(X)\,;\ \ \ l(X) \le w(X) $$ that is, the Suslin number does not exceed the density, the density does not exceed the weight, and the Lindelöf number does not exceed the weight. But the density and the Lindelöf number are not comparable in this sense: There are spaces $X$,$Y$ and $Z$ for which $$ d(X) < l(X)\,,\ \ \ l(Y)<d(Y)=c(Y)\,,\ \ \ l(Z)=d(Z)=c(Z) \ . $$
The incomparability of cardinality and weight is unexpected at first sight: There are countable normal $T_1$-spaces of uncountable weight. But always $d(X) \le |X|$ and $l(X) \le |X|$. For every $T_0$-space $X$, $|X| \le \exp(w(X))$ (one writes $\exp(\mathfrak{t})$ instead of $2^{\mathfrak{t}}$). For every Hausdorff space $X$, $|X| \le \exp(\exp(d(X)))$. Always $w(X) \le \exp(|X|)$.
In a comparison problem it may happen that not just two, but more cardinal characteristics are involved. In that direction, particularly subtle, beautiful and often unexpected results have been obtained, striking in their generality: For each Hausdorff space $X$, $|X| \le \exp(c(X)\cdot\chi(X))$, where the character $\chi(X)$ is the least infinite cardinal number $\mathfrak{t}$ such that at each point of $X$ there is a local base of cardinality $\le \mathfrak{t}$ (see [1], [2]). Much research in the theory of cardinal invariants was stimulated by the problem of estimating the cardinality of a compact Hausdorff space satisfying the first axiom of countability, a problem which remained unsolved from 1923 to 1969. It then turned out that for each Hausdorff space $X$, $|X| \le \exp(l(X)\cdot\chi(X))$ (Arkhangel'skii's theorem, see [2], [4]).
The computation of cardinal invariants takes place in all parts of general topology because of the set-theoretic nature of the latter. Therefore, the theory of cardinal invariants finds application in practically all domains of general topology and in each approach to the investigation of spaces.
In particular, in the study of spaces by coverings, the Lindelöf number, the density and the Suslin number appeared from the very beginning. In the investigation and classification of spaces by continuous mappings (in particular, in the development of the theory of dyadic compacta and of the theory of absolutes) new cardinal invariants arose and played a key role: the spread and the $\pi$-weight. The spread $s(X)$ of a space $X$ is the least upper bound of the cardinalities of discrete subspaces of $X$, and the $\pi$-weight $\pi w(X)$ of a space $X$ is the minimum of the cardinalities of families $\mathcal{V}$ (called $\pi$-bases) of non-empty open sets in $X$ such that for each non-empty open set $U$ in $X$ there is a $V \in \mathcal{V}$ such that $V \subset U$. In the investigation of spaces by inverse spectra a major role is played by the Suslin number, the character and the weight.
Thus, there is an approach to general topology for which cardinal invariants appear both as the principal means of investigation of the structure of spaces, as a basic language in which the properties of spaces from various classes can be expressed and, finally, as a means of classification and selection of new classes of topological spaces. Basic here is, again, the problem of the comparison of cardinal characteristics. The fundamental question can be posed as follows. Given a class $\mathcal{P}$ of topological spaces to which the domains of definition of cardinal invariants are restricted. What are the basic relations between the cardinal invariants under these restrictions? By developing the theory of cardinal invariants for a class $\mathcal{P}$ one obtains the "cardinal portrait" of $\mathcal{P}$. A comparison of the cardinal portraits of two classes $\mathcal{P}_1$ and $\mathcal{P}_2$ allows one to judge the relationships between these classes and also to give effective means of proving that a concrete space belongs to one class or other.
This approach can be demonstrated by the class of metrizable spaces. The characteristic feature here is that for this class a number of fundamental cardinal invariants coincide: the Suslin number is equal to the density, to the weight and to the Lindelöf number. This fact is often applied; for example, to prove that some space is non-metrizable, it is enough to prove that at least two of the invariants mentioned above differ.
In the class of metrizable spaces the theory of cardinal invariants distinguishes itself from the general theory mainly by its simplifications, whereas in the class of compact Hausdorff spaces it changes its appearance completely and in a non-trivial way. Responsible for the particular appearance of this theory is the fact that for compact Hausdorff spaces the character and pseudo-character coincide, as well as the weight and the network weight. The pseudo-character $\psi(x,X)$ of $X$ at $x$ is the smallest number of open sets whose intersection is the point, and the character $\chi(x,X)$ of $X$ at $x$ is the least cardinality of a local base at $x$. The network weight $\mathrm{nw}(X)$ is the least cardinality of a family $\mathcal{S}$ of sets in $X$ satisfying the condition: If $x \in U \subset X$, where $U$ is open in $X$, then there is a $P \in \mathcal{S}$ for which $x \in P \subset U$ (such families are called networks in $X$). For every compact Hausdorff space $X$ the following hold: 1) $\psi(,X) = \chi(x,X)$ for all $x \in X)$; and 2) $\mathrm{nw}(X) = w(X)$. Therefore, the weight cannot increase under a continuous mapping onto a compact Hausdorff space, and if a compact Hausdorff space $X$ is the union of two subspaces $X_1$ and $X_2$, then the weight of $X$ does not exceed the maximum of the weights of $X_1$ and $X_2$ (the addition theorem for weights). For the same reason, the weight of a compact Hausdorff space never exceeds its cardinality; in particular, every countable compact Hausdorff space is metrizable. None of these theorems of the theory of cardinal invariants for the class of compact Hausdorff spaces can be extended to the class of completely-regular spaces. An important specific result is the following: If $X$ is a compact Hausdorff space, $\mathfrak{t}$ is a cardinal number, $\mathfrak{t} \le \aleph_0$ and if $\chi(x,X) \ge \mathfrak{t}$ for all $x \in X$, then $|X| \ge \exp\mathfrak{t}$ (the Čech–Pospišil theorem). Almost-all metrizability criteria for compact Hausdorff spaces are also theorems about cardinal invariants. Thus, metrizability of a compact Hausdorff space $X$ is equivalent to any of the following conditions: a) $w(X) = \aleph_0$; b) $\mathrm{nw}(X) = \aleph_0$; c) the diagonal in $X \times X$ is a $G_\delta$-set; or d) $X$ has a point-countable base.
In the investigation of the structure of compact Hausdorff spaces 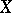 the tightness
the tightness 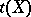 plays a major role. The tightness
plays a major role. The tightness 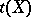 (see [2], [4]) of
(see [2], [4]) of 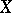 is the least cardinal number
is the least cardinal number 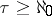 such that if
such that if 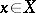 ,
, 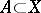 and
and 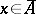 , then there is a
, then there is a 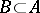 for which
for which 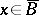 and
and 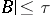 . The tightness does not increase when a compact Hausdorff space
. The tightness does not increase when a compact Hausdorff space  is raised to a finite power (in the class of completely-regular spaces this is not true).
is raised to a finite power (in the class of completely-regular spaces this is not true).
If the tightness of a compact Hausdorff space 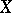 does not exceed
does not exceed  , then for each
, then for each 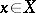 there is a family
there is a family 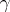 of non-empty open sets in
of non-empty open sets in  such that
such that  and each neighbourhood
and each neighbourhood  of
of  contains an element of
contains an element of 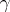 . Therefore the
. Therefore the 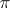 -weight of each separable compact Hausdorff space of countable tightness is equal to
-weight of each separable compact Hausdorff space of countable tightness is equal to  . The spread of a compact Hausdorff space majorizes its tightness.
. The spread of a compact Hausdorff space majorizes its tightness.
The fundamental properties of dyadic compacta are also, to a large extent, governed by theorems on cardinal characteristics. Thus, for each dyadic compactum the weight coincides with the spread and the tightness. The class of dyadic compacta contains the class of compact Hausdorff topological groups, thus, in particular, every compact Hausdorff group of countable tightness is metrizable.
In the theory of dyadic compacta (cf. Dyadic compactum) and in other parts of the theory of cardinal invariants, the question of the behaviour of these invariants under multiplication is of major importance. The following two theorems play an essential role here; the first of these implies the second. If 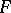 is a family of spaces such that
is a family of spaces such that 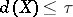 for each
for each 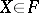 and if
and if 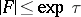 , then the density of the product of the spaces from
, then the density of the product of the spaces from 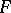 does not exceed
does not exceed  (see [1]–[4]). If
(see [1]–[4]). If 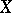 is the product of any set of spaces of densities not exceeding
is the product of any set of spaces of densities not exceeding  , then
, then 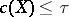 . In the latter result there is no limit on the number of factors. In particular, the Suslin number of any Tikhonov cube (the product of an arbitrary set of segments) is countable. Thus, the condition
. In the latter result there is no limit on the number of factors. In particular, the Suslin number of any Tikhonov cube (the product of an arbitrary set of segments) is countable. Thus, the condition  places no restriction on the cardinality of a space.
places no restriction on the cardinality of a space.
Many simply formulated questions on the behaviour of cardinal invariants under multiplication have turned out to be very delicate. For example, the question: Is it true that always 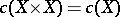 ? turns out to be related to the Suslin conjecture and the continuum hypothesis.
? turns out to be related to the Suslin conjecture and the continuum hypothesis.
On the other hand, the behaviour of cardinal invariants when passing from a space  to its image
to its image 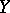 under a continuous mapping
under a continuous mapping 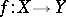 , is, on the whole, governed by simple general rules.
, is, on the whole, governed by simple general rules.
For example, 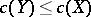 ,
, 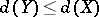 ,
, 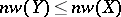 ,
, 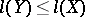 . If
. If 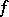 is a quotient mapping onto, then
is a quotient mapping onto, then 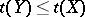 . The fact that the foundation of the theory of cardinal invariants consists of a system of simple universal rules of this kind also can be considered as one of the reasons ensuring the broad applicability of the theory.
. The fact that the foundation of the theory of cardinal invariants consists of a system of simple universal rules of this kind also can be considered as one of the reasons ensuring the broad applicability of the theory.
Significant information on the structure of spaces is obtained by consideration of the question: How do cardinal invariants behave on passing to a subspace? A cardinal invariant 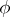 for which
for which  always implies
always implies 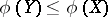 is called monotone. These include: weight, network weight, tightness, character, and spread. Non-monotone are the Suslin number, the density and the Lindelöf number. The following questions arise: Which are the spaces
is called monotone. These include: weight, network weight, tightness, character, and spread. Non-monotone are the Suslin number, the density and the Lindelöf number. The following questions arise: Which are the spaces 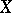 for which
for which 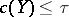 for all
for all 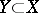 ; which are the spaces
; which are the spaces 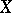 for which
for which 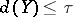 for all
for all 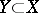 ; what effect on the topology of
; what effect on the topology of 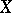 has the requirement:
has the requirement: 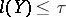 for all
for all 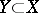 ? The answer to the first question is simple: the condition means that the spread of
? The answer to the first question is simple: the condition means that the spread of 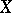 does not exceed
does not exceed  . But the two subsequent conditions single out new classes of spaces. The investigation of these classes turns out to depend on special hypotheses of set theory, in particular on Martin's axiom.
. But the two subsequent conditions single out new classes of spaces. The investigation of these classes turns out to depend on special hypotheses of set theory, in particular on Martin's axiom.
The theory of cardinal invariants has a peculiar character on the spaces of topological groups. E.g., the criterion for metrizability reduces here simply to the first axiom of countability. The major properties of linear topological spaces, in particular, of the spaces 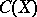 of continuous real-valued functions on a space
of continuous real-valued functions on a space 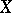 , can be formulated in the language of cardinal invariants. This refers to the theorems on Eberlein compacta (each Eberlein compactum is a Fréchet–Urysohn space, the weight of an Eberlein compactum is equal to its Suslin number); and the following theorem: If
, can be formulated in the language of cardinal invariants. This refers to the theorems on Eberlein compacta (each Eberlein compactum is a Fréchet–Urysohn space, the weight of an Eberlein compactum is equal to its Suslin number); and the following theorem: If 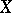 is a compact Hausdorff space, then the tightness of
is a compact Hausdorff space, then the tightness of 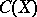 in the topology of pointwise convergence is countable.
in the topology of pointwise convergence is countable.
Between a number of cardinal invariants of the spaces 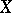 and
and 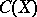 there is a duality-type correspondence.
there is a duality-type correspondence.
References
| [1] | I. Juhász, "Cardinal functions in topology" , North-Holland (1971) |
| [2] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
| [3] | R. Engelking, "Outline of general topology" , North-Holland (1968) (Translated from Polish) |
| [4] | A.V. Arkhangel'skii, "Structure and classification of topological spaces, and cardinal invariants" Russian Math. Surveys , 33 : 6 (1978) pp. 33–96 Uspekhi Mat. Nauk , 33 : 6 (1978) pp. 29–84 |
Comments
The usual terminology in the literature (cf. [1]–[4]) is cardinal function, or cardinal invariant.
The Suslin number of a space 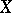 is also called its cellularity, and its Lindelöf number also its Lindelöf degree (the latter is often denoted by
is also called its cellularity, and its Lindelöf number also its Lindelöf degree (the latter is often denoted by 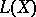 ).
).
The Urysohn–Tikhonov theorem, mentioned above, is also called the Urysohn metrization theorem.
The fact that the class of dyadic compacta contains the class of compact Hausdorff topological groups is called Kuzminov's theorem (on compact groups).
For the notion of a Fréchet–Urysohn space see Sequential space.
The problem of whether 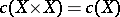 for every space
for every space 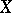 was solved by S. Todorčević [a2], who found, without using extra set-theoretic hypotheses, spaces
was solved by S. Todorčević [a2], who found, without using extra set-theoretic hypotheses, spaces 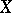 satisfying
satisfying 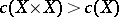 .
.
The problems whether every hereditarily separable space is Lindelöf and whether every hereditarily Lindelöf space is separable generated a lot of research. Many examples were constructed using various extra set-theoretical assumptions, in particular the Continuum Hypothesis. Todorcevi [a1] showed that the statement "every hereditarily separable space is Lindelöf" is consistent with the usual axioms of set theory. For much more information and other recent developments see various articles in [a3], in particular Chapts. 1; 2, and [a4].
[a1] showed that the statement "every hereditarily separable space is Lindelöf" is consistent with the usual axioms of set theory. For much more information and other recent developments see various articles in [a3], in particular Chapts. 1; 2, and [a4].
References
| [a1] | S. Todorcevic, "Forcing positive partition relations" Trans. Amer. Math. Soc. , 280 (1983) pp. 703–720 |
| [a2] | S. Todorcevic, "Remarks on cellularity in products" Compos. Math. , 57 (1986) pp. 357–372 |
| [a3] | K. Kunen (ed.) J.E. Vaughan (ed.) , Handbook of set-theoretic topology , North-Holland (1984) pp. Chapts. 1–2 |
| [a4] | I. Juhász, "Cardinal functions. Ten years later" , MC Tracts , 123 , North-Holland (1980) |
| [b1] | M.E. Rudin, "Lectures on set theoretic topology", Amer. Math. Soc. (1975) ISBN 0-8218-1673-X Zbl 0318.54001 |
Cardinal characteristic. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cardinal_characteristic&oldid=42677