Difference between revisions of "Si-ci-spiral"
From Encyclopedia of Mathematics
(Importing text file) |
(TeX) |
||
| Line 1: | Line 1: | ||
| − | A plane curve whose equation in rectangular Cartesian coordinates | + | {{TEX|done}} |
| + | A plane curve whose equation in rectangular Cartesian coordinates $(x,y)$ has the form | ||
| − | + | $$x=\operatorname{ci}(t),\quad y=\operatorname{si}(t),$$ | |
| − | where | + | where $\operatorname{ci}$ is the [[Integral cosine|integral cosine]], $\operatorname{si}$ is the [[Integral sine|integral sine]] and $t$ is a real parameter (see Fig.). |
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/s084980a.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/s084980a.gif" /> | ||
| Line 9: | Line 10: | ||
Figure: s084980a | Figure: s084980a | ||
| − | The arc length from | + | The arc length from $t=0$ to $t=t_0$ is equal to $\log t_0$, and the curvature is equal to $\kappa=t_0$. |
====References==== | ====References==== | ||
Revision as of 14:06, 3 August 2014
A plane curve whose equation in rectangular Cartesian coordinates $(x,y)$ has the form
$$x=\operatorname{ci}(t),\quad y=\operatorname{si}(t),$$
where $\operatorname{ci}$ is the integral cosine, $\operatorname{si}$ is the integral sine and $t$ is a real parameter (see Fig.).
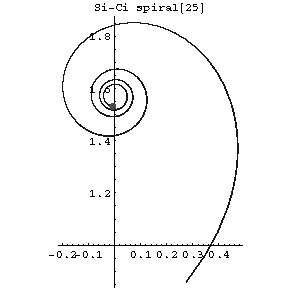
Figure: s084980a
The arc length from $t=0$ to $t=t_0$ is equal to $\log t_0$, and the curvature is equal to $\kappa=t_0$.
References
| [1] | E. Jahnke, F. Emde, F. Lösch, "Tafeln höheren Funktionen" , Teubner (1966) |
Comments
References
| [a1] | M. Abramowitz, I.A. Stegun, "Handbook of mathematical functions" , Dover, reprint (1972) |
How to Cite This Entry:
Si-ci-spiral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Si-ci-spiral&oldid=32696
Si-ci-spiral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Si-ci-spiral&oldid=32696
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article