|
|
| Line 1: |
Line 1: |
| − | One of the most important results in [[Algebraic topology|algebraic topology]] connecting homological (topological) characteristics of topological Hausdorff spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200201.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200202.png" /> (cf. also [[Hausdorff space|Hausdorff space]]) and a [[Continuous mapping|continuous mapping]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200203.png" />; it has applications, for example, in the fixed-point theory for mappings. There are variants of this theorem depending on the choice of the (co)homology functor <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200204.png" /> (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200205.png" />) when studying homomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200206.png" /> (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200207.png" />; see [[#References|[a1]]], [[#References|[a2]]] and [[Algebraic topology|algebraic topology]] for the necessary constructions and definitions).
| + | <!--This article has been texified automatically. Since there was no Nroff source code for this article, |
| | + | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist |
| | + | was used. |
| | + | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. |
| | | | |
| − | For the [[Functor|functor]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200208.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v1200209.png" /> is a group of coefficients, one defines <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002011.png" />-acyclicity of a set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002012.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002013.png" />, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002014.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002015.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002016.png" /> (and similarly for the functor <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002017.png" />). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002018.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002019.png" />-acyclic for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002020.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002021.png" /> is said to be acyclic.
| + | Out of 222 formulas, 219 were replaced by TEX code.--> |
| | | | |
| − | The simplest variant of the Vietoris–Begle theorem (close to [[#References|[a3]]]) is as follows. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002022.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002023.png" /> be compact Hausdorff spaces, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002024.png" /> be the Aleksandrov–Čech homology functor (over the field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002025.png" /> of rational numbers; cf. also [[Aleksandrov–Čech homology and cohomology|Aleksandrov–Čech homology and cohomology]]), let the mapping of compact pairs <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002026.png" /> have non-empty acyclic pre-images <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002027.png" /> for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002028.png" /> and let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002029.png" />; then the induced homomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002030.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002031.png" />, are isomorphisms (cf. also [[Homomorphism|Homomorphism]]; [[Isomorphism|Isomorphism]]). This result is also valid if one drops the condition of compactness of spaces and pairs and replaces it by the condition that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002032.png" /> be a proper mapping (cf. also [[Proper morphism|Proper morphism]]) [[#References|[a4]]].
| + | {{TEX|semi-auto}}{{TEX|partial}} |
| | + | One of the most important results in [[Algebraic topology|algebraic topology]] connecting homological (topological) characteristics of topological Hausdorff spaces $X$, $Y$ (cf. also [[Hausdorff space|Hausdorff space]]) and a [[Continuous mapping|continuous mapping]] $f : X \rightarrow Y$; it has applications, for example, in the fixed-point theory for mappings. There are variants of this theorem depending on the choice of the (co)homology functor $H_{*}$ (respectively, $H ^ { * }$) when studying homomorphisms $f _{*} : H * ( X ) \rightarrow H_{ *} ( Y )$ (respectively, $f ^ { * } : H ^ { * } ( Y ) \rightarrow H ^ { * } ( X )$; see [[#References|[a1]]], [[#References|[a2]]] and [[Algebraic topology|algebraic topology]] for the necessary constructions and definitions). |
| | | | |
| − | For the Aleksandrov–Kolmogorov functor <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002033.png" /> in the category of paracompact Hausdorff spaces and a bounded continuous surjective mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002034.png" /> one studies the cohomology homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002035.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002036.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002037.png" />-module. If the pre-image <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002038.png" />, for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002039.png" />, is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002040.png" />-acyclic for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002041.png" /> (for a fixed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002042.png" />), then the homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002043.png" /> is an isomorphism for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002044.png" /> and it is a [[Monomorphism|monomorphism]] for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002045.png" /> [[#References|[a2]]]. In the case of locally compact spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002046.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002047.png" />, the statement is valid for cohomologies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002048.png" /> with compact supports under the additional condition that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002049.png" /> be a proper mapping (cf. also [[Proper morphism|Proper morphism]]) [[#References|[a2]]]. | + | For the [[Functor|functor]] $H _ * (\, . \, ; G )$, where $G$ is a group of coefficients, one defines $q$-acyclicity of a set $M \subset X$ by $H _ { q } ( M , G ) = 0$, for $q > 0$, $H _ { 0 } ( M , G ) \cong G$ for $q = 0$ (and similarly for the functor $H ^ { * }$). If $M$ is $q$-acyclic for all $q \geq 0$, then $M$ is said to be acyclic. |
| | | | |
| − | In the case of metric spaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002050.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002051.png" />, the requirement that the pre-images <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002052.png" /> be <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002053.png" />-acyclic at all points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002054.png" /> can be weakened in that one allows sets <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002055.png" /> for which the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002056.png" />-acyclicity property is broken: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002057.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002058.png" />), <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002059.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002060.png" />), where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002061.png" /> is the group of coefficients. One defines the relative dimension of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002062.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002063.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002064.png" /> as the supremum of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002065.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002066.png" /> runs over the subsets bounded in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002067.png" />. One defines a "weight measure" of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002068.png" /> in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002069.png" /> by
| + | The simplest variant of the Vietoris–Begle theorem (close to [[#References|[a3]]]) is as follows. Let $X$, $Y$ be compact Hausdorff spaces, let $H_{*} ( X , \mathbf{Q} )$ be the Aleksandrov–Čech homology functor (over the field $\mathbf{Q}$ of rational numbers; cf. also [[Aleksandrov–Čech homology and cohomology|Aleksandrov–Čech homology and cohomology]]), let the mapping of compact pairs $f : ( X , X _ { 0 } ) \rightarrow ( Y , Y _ { 0 } )$ have non-empty acyclic pre-images $f ^ { - 1 } ( y )$ for any $y \in Y$ and let $f ^ { - 1 } ( Y _ { 0 } ) = X _ { 0 }$; then the induced homomorphisms $f_{*} : H _ { q } ( X , X _ { 0 } ) \rightarrow H _ { q } ( Y , Y _ { 0 } )$, $q \geq 0$, are isomorphisms (cf. also [[Homomorphism|Homomorphism]]; [[Isomorphism|Isomorphism]]). This result is also valid if one drops the condition of compactness of spaces and pairs and replaces it by the condition that $f$ be a proper mapping (cf. also [[Proper morphism|Proper morphism]]) [[#References|[a4]]]. |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002070.png" /></td> </tr></table>
| + | For the Aleksandrov–Kolmogorov functor $\overline { H } \square ^ { * }$ in the category of paracompact Hausdorff spaces and a bounded continuous surjective mapping $f : X \rightarrow Y$ one studies the cohomology homomorphism $f ^ { * } : \overline { H } \square ^ { * } ( Y , G ) \rightarrow \overline { H } \square ^ { * } ( X , G )$, where $G$ is an $\mathbf{R}$-module. If the pre-image $f ^ { - 1 } ( y )$, for any $y \in Y$, is $q$-acyclic for all $q < n$ (for a fixed $n > 0$), then the homomorphism $f ^ { * } : \overline { H } \square ^ { q } ( Y , G ) \rightarrow \overline { H } \square ^ { q } ( X , G )$ is an isomorphism for $q < n$ and it is a [[Monomorphism|monomorphism]] for $q = n$ [[#References|[a2]]]. In the case of locally compact spaces $X$, $Y$, the statement is valid for cohomologies $\overline { H } \square _ { c } ^ { * }$ with compact supports under the additional condition that $f$ be a proper mapping (cf. also [[Proper morphism|Proper morphism]]) [[#References|[a2]]]. |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002071.png" />, then the homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002072.png" /> is [[#References|[a5]]]:
| + | In the case of metric spaces $X$, $Y$, the requirement that the pre-images $f ^ { - 1 } ( y )$ be $q$-acyclic at all points $y \in Y$ can be weakened in that one allows sets $M _ { k } ( f ) \subset Y$ for which the $k$-acyclicity property is broken: $H ^ { k } ( f ^ { - 1 } ( y ) , G ) \neq 0$ ($k > 0$), $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) \notin G$ ($k = 0$), where $G$ is the group of coefficients. One defines the relative dimension of $M _ { k }$ in $Y$, $d _ { k } = \operatorname{rd} _ { Y } M _ { k }$ as the supremum of $\operatorname { dim } Q$, where $Q \subset M _ { k }$ runs over the subsets bounded in $Y$. One defines a "weight measure" of $M _ { k }$ in $Y$ by |
| | | | |
| − | for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002073.png" /> an [[Epimorphism|epimorphism]];
| + | \begin{equation*} \nu = \operatorname { max } _ { 0 \leq k \leq N - 1 } ( d _ { k } + k ). \end{equation*} |
| | | | |
| − | for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002074.png" /> an [[Isomorphism|isomorphism]]; and
| + | If $\nu < N - 1$, then the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is [[#References|[a5]]]: |
| | | | |
| − | for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002075.png" /> a [[Monomorphism|monomorphism]]. A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002076.png" /> is said to be an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002078.png" />-Vietoris mapping (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002079.png" />) if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002080.png" /> is a proper, surjective and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002081.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002082.png" /> [[#References|[a4]]]. From the previous statement it follows that for an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002083.png" />-Vietoris mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002084.png" /> the homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002085.png" /> is an isomorphism for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002086.png" />. For a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002087.png" />-Vietoris mapping, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002088.png" />, for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002089.png" />, i.e. all the pre-images <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002090.png" /> are acyclic; such mappings are called Vietoris mappings. | + | for $q = \nu + 1$ an [[Epimorphism|epimorphism]]; |
| | + | |
| | + | for $\nu + 1 < q < N$ an [[Isomorphism|isomorphism]]; and |
| | + | |
| | + | for $q = N$ a [[Monomorphism|monomorphism]]. A mapping $f : X \rightarrow Y$ is said to be an $n$-Vietoris mapping ($n \geq 1$) if $f$ is a proper, surjective and $\operatorname { rd } _{Y} ( M _ { k } (\, f ) ) \leq n - 2 - k $ for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002082.png"/> [[#References|[a4]]]. From the previous statement it follows that for an $n$-Vietoris mapping $f : X \rightarrow Y$ the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is an isomorphism for $q \geq n$. For a $1$-Vietoris mapping, $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) = G , H ^ { q } ( f ^ { - 1 } ( y ) , G ) = 0$, for all $q > 0$, i.e. all the pre-images $f ^ { - 1 } ( y )$ are acyclic; such mappings are called Vietoris mappings. |
| | | | |
| | ==Fixed-point theory.== | | ==Fixed-point theory.== |
| − | Vietoris–Begle-type theorems are connected with the problem of equality, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002091.png" />, for some <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002092.png" />, with the problem of coincidence of pairs <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002093.png" /> of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002094.png" />, and with the fixed-point problem for set-valued mappings (see, for example, [[#References|[a6]]], [[#References|[a7]]], [[#References|[a8]]], [[#References|[a4]]], [[#References|[a9]]], [[#References|[a10]]], [[#References|[a11]]], [[#References|[a13]]]). | + | Vietoris–Begle-type theorems are connected with the problem of equality, $f ( x ) = g ( x )$, for some $x$, with the problem of coincidence of pairs $( f , g )$ of mappings $f , g : X \rightarrow Y$, and with the fixed-point problem for set-valued mappings (see, for example, [[#References|[a6]]], [[#References|[a7]]], [[#References|[a8]]], [[#References|[a4]]], [[#References|[a9]]], [[#References|[a10]]], [[#References|[a11]]], [[#References|[a13]]]). |
| | | | |
| − | In fact, the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002095.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002096.png" /> is a surjection, gives a connection between the two problems: a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002097.png" /> at which <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002098.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v12002099.png" /> coincide, defines a fixed point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020100.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020101.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020102.png" />), and vice versa; in fact, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020103.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020104.png" /> is equal to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020105.png" /> at any point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020106.png" />. | + | In fact, the set-valued mapping $G = f \circ g ^ { - 1 } : Y \rightarrow Y$, where $g$ is a surjection, gives a connection between the two problems: a point $x _ { 0 }$ at which $f$ and $g$ coincide, defines a fixed point $y _ { 0 } = g ( x _ { 0 } )$ for $G$ ($y _ { 0 } \in G ( y _ { 0 } )$), and vice versa; in fact, if $y _ { 0 } \in \operatorname{Fix} G$, then $f$ is equal to $g$ at any point $x _ { 0 } \in g ^ { - 1 } ( y _ { 0 } )$. |
| | | | |
| − | For general set-valued mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020107.png" /> it is easy to construct a corresponding pair: consider the graph of the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020108.png" />, | + | For general set-valued mappings $F : X \rightarrow X$ it is easy to construct a corresponding pair: consider the graph of the set-valued mapping $F$, |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020109.png" /></td> </tr></table>
| + | \begin{equation*} \Gamma ( F ) = \{ ( x , y ) \in X \times X : y \in F ( x ) \} \end{equation*} |
| | | | |
| − | and its Cartesian projections <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020110.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020111.png" />. One obtains the pair <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020112.png" />, for which a point of coincidence <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020113.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020114.png" />, defines a fixed point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020115.png" /> of the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020116.png" />. | + | and its Cartesian projections $p ( x , y ) = x$, $q ( x , y ) = y$. One obtains the pair $( p , q ) : \Gamma ( F ) \rightarrow X$, for which a point of coincidence $( x _ { 0 } , y _ { 0 } ) \in \Gamma ( F )$, $p ( x _ { 0 } , y _ { 0 } ) = q ( x _ { 0 } , y _ { 0 } )$, defines a fixed point $x _ { 0 } \in F ( x _ { 0 } )$ of the set-valued mapping $F$. |
| | | | |
| | ==Topological characteristics.== | | ==Topological characteristics.== |
| − | Topological characteristics such as the Lefschetz number, the Kronecker characteristic, the rotation of the vector field (M.A. Krasnoselskii), the Brouwer–Hopf degree, are well known for single-valued mappings in finite-dimensional spaces (see, for example, [[#References|[a12]]]). Analogous characteristics for general set-valued mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020117.png" /> have been constructed on the basis of homomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020118.png" />, (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020119.png" />) of (co)homology groups of the pair <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020120.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020121.png" />. These set-valued mappings satisfy the general conditions of compactness of images and have the property of upper semi-continuity. However, there is also a homological condition for a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020122.png" /> to be <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020123.png" />-Vietoris, ensuring an isomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020124.png" /> (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020125.png" />) in homology (cohomology) of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020126.png" />, and permitting one to construct a homomorphism <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020127.png" /> (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020128.png" />), generated by the set-valued mapping in (co)homology by the formula <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020129.png" /> (respectively, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020130.png" />). | + | Topological characteristics such as the Lefschetz number, the Kronecker characteristic, the rotation of the vector field (M.A. Krasnoselskii), the Brouwer–Hopf degree, are well known for single-valued mappings in finite-dimensional spaces (see, for example, [[#References|[a12]]]). Analogous characteristics for general set-valued mappings $F$ have been constructed on the basis of homomorphisms $( p _ {*} , q _ { * } )$, (respectively, $( p ^ { * } , q ^ { * } )$) of (co)homology groups of the pair $( p , q )$ for $F$. These set-valued mappings satisfy the general conditions of compactness of images and have the property of upper semi-continuity. However, there is also a homological condition for a mapping $F$ to be $n$-Vietoris, ensuring an isomorphism $p_{ *}$ (respectively, $p ^ { * }$) in homology (cohomology) of dimension $q \geq n$, and permitting one to construct a homomorphism $F_{*}$ (respectively, $F ^ { * }$), generated by the set-valued mapping in (co)homology by the formula $F * = q * p * ^ { - 1 }$ (respectively, $F ^ { * } = p ^ { * - 1} q ^ { * }$). |
| | | | |
| − | S. Eilenberg and D. Montgomery [[#References|[a6]]] have generalized the classical construction of the Lefschetz number to set-valued upper semi-continuous mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020131.png" /> with acyclic images, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020132.png" /> is compact metric ANR-space: | + | S. Eilenberg and D. Montgomery [[#References|[a6]]] have generalized the classical construction of the Lefschetz number to set-valued upper semi-continuous mappings $F : X \rightarrow X$ with acyclic images, where $X$ is compact metric ANR-space: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020133.png" /></td> </tr></table>
| + | \begin{equation*} \Lambda ( F ) = \sum _ { n = 0 } ^ { \infty } ( - 1 ) ^ { n } \operatorname { tr } ( r _n* \circ t_n * ^ { - 1 } ); \end{equation*} |
| | | | |
| − | here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020134.png" /> is a canonical decomposition of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020135.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020136.png" /> are homomorphisms and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020137.png" /> is an isomorphism for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020138.png" /> (due to the Vietoris–Begle theorem). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020139.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020140.png" />. This result was generalized by many authors (see [[#References|[a9]]], [[#References|[a10]]], [[#References|[a4]]], [[#References|[a7]]]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants. | + | here, $F = r \circ t ^ { - 1 }$ is a canonical decomposition of $F$, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020136.png"/> are homomorphisms and $t _ { n_{*} }$ is an isomorphism for any $n \geq 0$ (due to the Vietoris–Begle theorem). If $\Lambda ( F ) \neq \theta$, then $\operatorname {Fix} F \neq \emptyset$. This result was generalized by many authors (see [[#References|[a9]]], [[#References|[a10]]], [[#References|[a4]]], [[#References|[a7]]]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants. |
| | | | |
| | ===Degree theory.=== | | ===Degree theory.=== |
| − | To describe the topological characteristics of set-valued mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020141.png" /> like the degree <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020142.png" /> or the Kronecker characteristics <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020143.png" /> some definitions are needed. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020144.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020145.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020146.png" /> be separable topological spaces (cf. also [[Separable space|Separable space]]), let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020147.png" /> be the space of compact subsets, and suppose the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020148.png" /> is upper semi-continuous. Such a mapping is called | + | To describe the topological characteristics of set-valued mappings $F$ like the degree $\operatorname { deg } F$ or the Kronecker characteristics $\gamma ( F )$ some definitions are needed. Let $X$, $Y$, $Z$ be separable topological spaces (cf. also [[Separable space|Separable space]]), let $K ( Y )$ be the space of compact subsets, and suppose the set-valued mapping $F : X \rightarrow K ( Y )$ is upper semi-continuous. Such a mapping is called |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020150.png" />-acyclic if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020151.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020152.png" /> (here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020153.png" /> is the set of points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020154.png" /> at which the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020155.png" />-acyclicity of the images <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020156.png" /> is broken);
| + | $n$-acyclic if $\operatorname { rd }_{X} ( N _ { K } ( F ) ) \leq n - k - 2$ for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020152.png"/> (here, $N _ { K } ( F ) \subset X$ is the set of points $x$ at which the $k$-acyclicity of the images $F ( x )$ is broken); |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020158.png" />-acyclic if it is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020159.png" />-acyclic; this is equivalent to acyclicity of every image <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020160.png" />. A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020161.png" /> is called generally <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020163.png" />-acyclic if there exist a space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020164.png" /> and single-valued continuous mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020165.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020166.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020167.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020168.png" />-Vietoris and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020169.png" /> for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020170.png" />. The collection <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020171.png" /> is then said to be a representation of the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020172.png" />, the pair <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020173.png" /> is called a selecting pair, and the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020174.png" /> is called a selector of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020175.png" />. For an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020176.png" />-acyclic mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020177.png" />, the projections of the graph <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020178.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020179.png" /> give a selecting pair:
| + | $F$-acyclic if it is $1$-acyclic; this is equivalent to acyclicity of every image $F ( x )$. A mapping $F : X \rightarrow K ( Y )$ is called generally $n$-acyclic if there exist a space $Z$ and single-valued continuous mappings $p : Z \rightarrow X$, $q : Z \rightarrow Y$, where $p$ is $n$-Vietoris and $q \circ p ^ { - 1 } ( x ) \subset F ( x )$ for all $x \in X$. The collection $\{ X , Y , Z , p , q \}$ is then said to be a representation of the set-valued mapping $F$, the pair $( p , q )$ is called a selecting pair, and the mapping $q \circ p ^ { - 1 }$ is called a selector of $F$. For an $n$-acyclic mapping $F$, the projections of the graph $t : X \times Y \supset \Gamma ( F ) \rightarrow X$, $r : X \times Y \supset \Gamma ( F ) \rightarrow Y$ give a selecting pair: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020180.png" /></td> </tr></table>
| + | \begin{equation*} F ( x ) = r \circ t ^ { - 1 } ( x ). \end{equation*} |
| | | | |
| − | As an example, consider the main construction of the degree of a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020181.png" /> from the unit disc <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020182.png" /> in the Euclidean space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020183.png" /> under the condition that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020184.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020185.png" />, is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020186.png" />-acyclic, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020187.png" />. A generalization of the Vietoris–Begle theorem given by E.G. Sklyarenko ensures the existence of cohomology isomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020188.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020189.png" /> over the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020190.png" />. Then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020191.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020192.png" /> is given by the equality <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020193.png" />. Here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020194.png" />, respectively <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020195.png" />, is a generator of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020196.png" />, respectively <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020197.png" />, which is isomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020198.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020199.png" /> (a construction given by D.G. Bourgin, L. Górniewicz, and others, see [[#References|[a9]]], [[#References|[a10]]], [[#References|[a4]]]). If the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020200.png" /> under consideration is generally acyclic, then for every selecting pair <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020201.png" /> the set-valued mapping (the selector of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020202.png" />) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020203.png" /> is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020204.png" />-acyclic, and for it <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020205.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020206.png" />; applying the previous construction for the selector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020207.png" /> of the set-valued mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020208.png" />, one obtains <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020209.png" /> for any selector <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020210.png" />. The generalized degree <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020211.png" /> is the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020212.png" /> generated by all selecting pairs <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020213.png" /> for the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020214.png" />-mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020215.png" />. A more general construction (without the condition that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020216.png" /> be <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020217.png" />-acyclic) was introduced by B.D. Gelman (see [[#References|[a10]]]); namely, the topological characteristic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020218.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020219.png" /> is defined by the equality | + | As an example, consider the main construction of the degree of a mapping $F : \overline { D } \square ^ { n + 1 } \rightarrow K ( E ^ { n + 1 } )$ from the unit disc $\overline { D } \square ^ { n + 1 } \subset E ^ { n + 1 }$ in the Euclidean space $E ^ { n + 1}$ under the condition that $F : S ^ { n } \rightarrow K ( E ^ { n + 1 } \backslash \theta )$, where $S ^ { n } = \partial \overline { D } \square ^ { n + 1 }$, is $m$-acyclic, $1 \leq m \leq n$. A generalization of the Vietoris–Begle theorem given by E.G. Sklyarenko ensures the existence of cohomology isomorphisms $t ^ { * } : H ^ { n } ( S ^ { n } ) \rightarrow H ^ { n } ( \Gamma _ { S ^ { n } } )$, $\hat { t } \square ^ { * } : H ^ { n + 1 } ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \rightarrow H ^ { n + 1 } ( \Gamma _ { \overline{D} \square ^ { n + 1 } } , \Gamma _ { S ^ { n } } )$ over the group $\bf Z$. Then $\operatorname { deg } ( F , \overline { D } \square ^ { n + 1 } , \theta ) = k$, where $k$ is given by the equality $( t ^ { * } ) ^ { - 1 } \circ ( t - r ) ^ { * } \beta _ { 1 } = k \beta _ { 2 }$. Here, $\beta _ { 1 }$, respectively $\beta_2$, is a generator of the group $H ^ { n } ( E ^ { n + 1 } \backslash \theta )$, respectively $H ^ { n } ( S ^ { n } )$, which is isomorphic to $\bf Z$, and $( t - r ) : ( \Gamma _ { S ^ { n } } ) \rightarrow ( E ^ { n + 1 } \backslash 0 )$ (a construction given by D.G. Bourgin, L. Górniewicz, and others, see [[#References|[a9]]], [[#References|[a10]]], [[#References|[a4]]]). If the mapping $F : X \rightarrow K ( Y )$ under consideration is generally acyclic, then for every selecting pair $( p , q ) \subset F$ the set-valued mapping (the selector of $F$) $G = p \circ q ^ { - 1 } : X \rightarrow K ( Y )$ is $m$-acyclic, and for it $r = p$, $t = q$; applying the previous construction for the selector $G$ of the set-valued mapping $F : ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \rightarrow ( K ( E ^ { n + 1 } ) , K ( E ^ { n + 1 } \backslash \theta ) )$, one obtains $\operatorname { deg } ( G , \overline { D } \square ^ { n + 1 } , \theta )$ for any selector $G$. The generalized degree $\operatorname { Deg } ( F , \overline { D } \square ^ { n + 1 } , \theta )$ is the set $\{ \operatorname { deg } ( G , \overline { D } \square ^ { n + 1 } , \theta ) \}$ generated by all selecting pairs $( p , q )$ for the $n$-mapping $F$. A more general construction (without the condition that $F$ be $m$-acyclic) was introduced by B.D. Gelman (see [[#References|[a10]]]); namely, the topological characteristic $\kappa ( F , \overline { D } \square ^ { n + 1 } ) = k$, where $k$ is defined by the equality |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020220.png" /></td> </tr></table>
| + | \begin{equation*} \delta ^ { * } \circ ( t - r ) ^ { * } \beta _ { 1 } = k ( \widehat{t ^ { * }} \square ^ { - 1 } \beta _ { 3 } ), \end{equation*} |
| | | | |
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020221.png" /> is a generator in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020222.png" />, all the generators <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020223.png" /> are in accordance with the orientation of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020224.png" />, and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020225.png" /> is a connecting homomorphism.
| + | $\beta_3$ is a generator in $H ^ { n + 1 } ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \cong \mathbf{Z}$, all the generators $\beta _ { i }$ are in accordance with the orientation of $E ^ { n + 1}$, and $\delta ^ { * } : H ^ { n } ( \Gamma _ { S ^ { n } } ) \rightarrow H ^ { n + 1 } ( \Gamma _ { \bar{D} \square ^ { n + 1 } } , \Gamma _ { S ^ { n } } )$ is a connecting homomorphism. |
| | | | |
| − | Note that an earlier definition of rotation of a set-valued field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020226.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/v/v120/v120020/v120020227.png" />, with non-acyclic images was given in [[#References|[a8]]], [[#References|[a13]]], [[#References|[a14]]]. | + | Note that an earlier definition of rotation of a set-valued field $\Phi x = x - F x$, $\Phi : \partial U \rightarrow E ^ { n + 1 } {\color{blue} \backslash} 0$, with non-acyclic images was given in [[#References|[a8]]], [[#References|[a13]]], [[#References|[a14]]]. |
| | | | |
| | ====References==== | | ====References==== |
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> S. Eilenberg, N. Steenrod, "Foundations of algebraic topology" , Princeton Univ. Press (1952)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E.G. Begle, "The Vietoris mappings theorem for bicompact spaces" ''Ann. of Math.'' , '''51''' : 2 (1950) pp. 534–550</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> L. Górniewicz, "Homological methods in fixed-point theory of multi-valued maps" ''Dissert. Math.'' , '''CXXIX''' (1976) pp. 1–71</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> E.G. Sklyarenko, "Of some applications of theory of bundles in general topology" ''Uspekhi Mat. Nauk'' , '''19''' : 6 (1964) pp. 47–70 (In Russian)</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S. Eilenberg, D. Montgomery, "Fixed point theorems for multi-valued transformations" ''Amer. J. Math.'' , '''68''' (1946) pp. 214–222</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> A. Granas, J.W. Jaworowski, "Some theorems on multi-valued maps of subsets of the Euclidean space" ''Bull. Acad. Polon. Sci.'' , '''7''' : 5 (1959) pp. 277–283</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> Yu.G. Borisovich, B.D. Gelman, V.V. Obukhovskii, "Of some topological invariants of set-valued maps with nonconvex images" ''Proc. Sem. Functional Analysis, Voronezh State Univ.'' , '''12''' (1969) pp. 85–95</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Topological methods in the fixed-point theory of multi-valued maps" ''Russian Math. Surveys'' , '''35''' : 1 (1980) pp. 65–143 (In Russian)</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Multivalued mappings" ''J. Soviet Math.'' , '''24''' (1984) pp. 719–791 (In Russian)</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> Yu.G. Borisovich, "A modern appoach to the theory of topological characteristics of nonlinear operators II" , ''Global analysis: Studies and Applications IV'' , ''Lecture Notes Math.'' , '''1453''' , Springer (1990) pp. 21–49</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> Yu.G. Borisovich, N.M. Bliznyakov, T.N. Fomenko, Y.A. Izrailevich, "Introduction to differential and algebraic topology" , Kluwer Acad. Publ. (1995)</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> L. Górniewicz, "On non-acyclic multi-valued mappings of subsets of Euclidean spaces" ''Bull. Acad. Polon. Sci.'' , '''20''' : 5 (1972) pp. 379–385</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> D.G. Bouvgin, "Cones and Vietoris–Begle type theorems" ''Trans. Amer. Math. Soc.'' , '''174''' (1972) pp. 155–183</TD></TR></table> | + | <table><tr><td valign="top">[a1]</td> <td valign="top"> S. Eilenberg, N. Steenrod, "Foundations of algebraic topology" , Princeton Univ. Press (1952)</td></tr><tr><td valign="top">[a2]</td> <td valign="top"> E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966)</td></tr><tr><td valign="top">[a3]</td> <td valign="top"> E.G. Begle, "The Vietoris mappings theorem for bicompact spaces" ''Ann. of Math.'' , '''51''' : 2 (1950) pp. 534–550</td></tr><tr><td valign="top">[a4]</td> <td valign="top"> L. Górniewicz, "Homological methods in fixed-point theory of multi-valued maps" ''Dissert. Math.'' , '''CXXIX''' (1976) pp. 1–71</td></tr><tr><td valign="top">[a5]</td> <td valign="top"> E.G. Sklyarenko, "Of some applications of theory of bundles in general topology" ''Uspekhi Mat. Nauk'' , '''19''' : 6 (1964) pp. 47–70 (In Russian)</td></tr><tr><td valign="top">[a6]</td> <td valign="top"> S. Eilenberg, D. Montgomery, "Fixed point theorems for multi-valued transformations" ''Amer. J. Math.'' , '''68''' (1946) pp. 214–222</td></tr><tr><td valign="top">[a7]</td> <td valign="top"> A. Granas, J.W. Jaworowski, "Some theorems on multi-valued maps of subsets of the Euclidean space" ''Bull. Acad. Polon. Sci.'' , '''7''' : 5 (1959) pp. 277–283</td></tr><tr><td valign="top">[a8]</td> <td valign="top"> Yu.G. Borisovich, B.D. Gelman, V.V. Obukhovskii, "Of some topological invariants of set-valued maps with nonconvex images" ''Proc. Sem. Functional Analysis, Voronezh State Univ.'' , '''12''' (1969) pp. 85–95</td></tr><tr><td valign="top">[a9]</td> <td valign="top"> Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Topological methods in the fixed-point theory of multi-valued maps" ''Russian Math. Surveys'' , '''35''' : 1 (1980) pp. 65–143 (In Russian)</td></tr><tr><td valign="top">[a10]</td> <td valign="top"> Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Multivalued mappings" ''J. Soviet Math.'' , '''24''' (1984) pp. 719–791 (In Russian)</td></tr><tr><td valign="top">[a11]</td> <td valign="top"> Yu.G. Borisovich, "A modern appoach to the theory of topological characteristics of nonlinear operators II" , ''Global analysis: Studies and Applications IV'' , ''Lecture Notes Math.'' , '''1453''' , Springer (1990) pp. 21–49</td></tr><tr><td valign="top">[a12]</td> <td valign="top"> Yu.G. Borisovich, N.M. Bliznyakov, T.N. Fomenko, Y.A. Izrailevich, "Introduction to differential and algebraic topology" , Kluwer Acad. Publ. (1995)</td></tr><tr><td valign="top">[a13]</td> <td valign="top"> L. Górniewicz, "On non-acyclic multi-valued mappings of subsets of Euclidean spaces" ''Bull. Acad. Polon. Sci.'' , '''20''' : 5 (1972) pp. 379–385</td></tr><tr><td valign="top">[a14]</td> <td valign="top"> D.G. Bouvgin, "Cones and Vietoris–Begle type theorems" ''Trans. Amer. Math. Soc.'' , '''174''' (1972) pp. 155–183</td></tr></table> |
One of the most important results in algebraic topology connecting homological (topological) characteristics of topological Hausdorff spaces $X$, $Y$ (cf. also Hausdorff space) and a continuous mapping $f : X \rightarrow Y$; it has applications, for example, in the fixed-point theory for mappings. There are variants of this theorem depending on the choice of the (co)homology functor $H_{*}$ (respectively, $H ^ { * }$) when studying homomorphisms $f _{*} : H * ( X ) \rightarrow H_{ *} ( Y )$ (respectively, $f ^ { * } : H ^ { * } ( Y ) \rightarrow H ^ { * } ( X )$; see [a1], [a2] and algebraic topology for the necessary constructions and definitions).
For the functor $H _ * (\, . \, ; G )$, where $G$ is a group of coefficients, one defines $q$-acyclicity of a set $M \subset X$ by $H _ { q } ( M , G ) = 0$, for $q > 0$, $H _ { 0 } ( M , G ) \cong G$ for $q = 0$ (and similarly for the functor $H ^ { * }$). If $M$ is $q$-acyclic for all $q \geq 0$, then $M$ is said to be acyclic.
The simplest variant of the Vietoris–Begle theorem (close to [a3]) is as follows. Let $X$, $Y$ be compact Hausdorff spaces, let $H_{*} ( X , \mathbf{Q} )$ be the Aleksandrov–Čech homology functor (over the field $\mathbf{Q}$ of rational numbers; cf. also Aleksandrov–Čech homology and cohomology), let the mapping of compact pairs $f : ( X , X _ { 0 } ) \rightarrow ( Y , Y _ { 0 } )$ have non-empty acyclic pre-images $f ^ { - 1 } ( y )$ for any $y \in Y$ and let $f ^ { - 1 } ( Y _ { 0 } ) = X _ { 0 }$; then the induced homomorphisms $f_{*} : H _ { q } ( X , X _ { 0 } ) \rightarrow H _ { q } ( Y , Y _ { 0 } )$, $q \geq 0$, are isomorphisms (cf. also Homomorphism; Isomorphism). This result is also valid if one drops the condition of compactness of spaces and pairs and replaces it by the condition that $f$ be a proper mapping (cf. also Proper morphism) [a4].
For the Aleksandrov–Kolmogorov functor $\overline { H } \square ^ { * }$ in the category of paracompact Hausdorff spaces and a bounded continuous surjective mapping $f : X \rightarrow Y$ one studies the cohomology homomorphism $f ^ { * } : \overline { H } \square ^ { * } ( Y , G ) \rightarrow \overline { H } \square ^ { * } ( X , G )$, where $G$ is an $\mathbf{R}$-module. If the pre-image $f ^ { - 1 } ( y )$, for any $y \in Y$, is $q$-acyclic for all $q < n$ (for a fixed $n > 0$), then the homomorphism $f ^ { * } : \overline { H } \square ^ { q } ( Y , G ) \rightarrow \overline { H } \square ^ { q } ( X , G )$ is an isomorphism for $q < n$ and it is a monomorphism for $q = n$ [a2]. In the case of locally compact spaces $X$, $Y$, the statement is valid for cohomologies $\overline { H } \square _ { c } ^ { * }$ with compact supports under the additional condition that $f$ be a proper mapping (cf. also Proper morphism) [a2].
In the case of metric spaces $X$, $Y$, the requirement that the pre-images $f ^ { - 1 } ( y )$ be $q$-acyclic at all points $y \in Y$ can be weakened in that one allows sets $M _ { k } ( f ) \subset Y$ for which the $k$-acyclicity property is broken: $H ^ { k } ( f ^ { - 1 } ( y ) , G ) \neq 0$ ($k > 0$), $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) \notin G$ ($k = 0$), where $G$ is the group of coefficients. One defines the relative dimension of $M _ { k }$ in $Y$, $d _ { k } = \operatorname{rd} _ { Y } M _ { k }$ as the supremum of $\operatorname { dim } Q$, where $Q \subset M _ { k }$ runs over the subsets bounded in $Y$. One defines a "weight measure" of $M _ { k }$ in $Y$ by
\begin{equation*} \nu = \operatorname { max } _ { 0 \leq k \leq N - 1 } ( d _ { k } + k ). \end{equation*}
If $\nu < N - 1$, then the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is [a5]:
for $q = \nu + 1$ an epimorphism;
for $\nu + 1 < q < N$ an isomorphism; and
for $q = N$ a monomorphism. A mapping $f : X \rightarrow Y$ is said to be an $n$-Vietoris mapping ($n \geq 1$) if $f$ is a proper, surjective and $\operatorname { rd } _{Y} ( M _ { k } (\, f ) ) \leq n - 2 - k $ for all 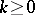 [a4]. From the previous statement it follows that for an $n$-Vietoris mapping $f : X \rightarrow Y$ the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is an isomorphism for $q \geq n$. For a $1$-Vietoris mapping, $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) = G , H ^ { q } ( f ^ { - 1 } ( y ) , G ) = 0$, for all $q > 0$, i.e. all the pre-images $f ^ { - 1 } ( y )$ are acyclic; such mappings are called Vietoris mappings.
[a4]. From the previous statement it follows that for an $n$-Vietoris mapping $f : X \rightarrow Y$ the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is an isomorphism for $q \geq n$. For a $1$-Vietoris mapping, $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) = G , H ^ { q } ( f ^ { - 1 } ( y ) , G ) = 0$, for all $q > 0$, i.e. all the pre-images $f ^ { - 1 } ( y )$ are acyclic; such mappings are called Vietoris mappings.
Fixed-point theory.
Vietoris–Begle-type theorems are connected with the problem of equality, $f ( x ) = g ( x )$, for some $x$, with the problem of coincidence of pairs $( f , g )$ of mappings $f , g : X \rightarrow Y$, and with the fixed-point problem for set-valued mappings (see, for example, [a6], [a7], [a8], [a4], [a9], [a10], [a11], [a13]).
In fact, the set-valued mapping $G = f \circ g ^ { - 1 } : Y \rightarrow Y$, where $g$ is a surjection, gives a connection between the two problems: a point $x _ { 0 }$ at which $f$ and $g$ coincide, defines a fixed point $y _ { 0 } = g ( x _ { 0 } )$ for $G$ ($y _ { 0 } \in G ( y _ { 0 } )$), and vice versa; in fact, if $y _ { 0 } \in \operatorname{Fix} G$, then $f$ is equal to $g$ at any point $x _ { 0 } \in g ^ { - 1 } ( y _ { 0 } )$.
For general set-valued mappings $F : X \rightarrow X$ it is easy to construct a corresponding pair: consider the graph of the set-valued mapping $F$,
\begin{equation*} \Gamma ( F ) = \{ ( x , y ) \in X \times X : y \in F ( x ) \} \end{equation*}
and its Cartesian projections $p ( x , y ) = x$, $q ( x , y ) = y$. One obtains the pair $( p , q ) : \Gamma ( F ) \rightarrow X$, for which a point of coincidence $( x _ { 0 } , y _ { 0 } ) \in \Gamma ( F )$, $p ( x _ { 0 } , y _ { 0 } ) = q ( x _ { 0 } , y _ { 0 } )$, defines a fixed point $x _ { 0 } \in F ( x _ { 0 } )$ of the set-valued mapping $F$.
Topological characteristics.
Topological characteristics such as the Lefschetz number, the Kronecker characteristic, the rotation of the vector field (M.A. Krasnoselskii), the Brouwer–Hopf degree, are well known for single-valued mappings in finite-dimensional spaces (see, for example, [a12]). Analogous characteristics for general set-valued mappings $F$ have been constructed on the basis of homomorphisms $( p _ {*} , q _ { * } )$, (respectively, $( p ^ { * } , q ^ { * } )$) of (co)homology groups of the pair $( p , q )$ for $F$. These set-valued mappings satisfy the general conditions of compactness of images and have the property of upper semi-continuity. However, there is also a homological condition for a mapping $F$ to be $n$-Vietoris, ensuring an isomorphism $p_{ *}$ (respectively, $p ^ { * }$) in homology (cohomology) of dimension $q \geq n$, and permitting one to construct a homomorphism $F_{*}$ (respectively, $F ^ { * }$), generated by the set-valued mapping in (co)homology by the formula $F * = q * p * ^ { - 1 }$ (respectively, $F ^ { * } = p ^ { * - 1} q ^ { * }$).
S. Eilenberg and D. Montgomery [a6] have generalized the classical construction of the Lefschetz number to set-valued upper semi-continuous mappings $F : X \rightarrow X$ with acyclic images, where $X$ is compact metric ANR-space:
\begin{equation*} \Lambda ( F ) = \sum _ { n = 0 } ^ { \infty } ( - 1 ) ^ { n } \operatorname { tr } ( r _n* \circ t_n * ^ { - 1 } ); \end{equation*}
here, $F = r \circ t ^ { - 1 }$ is a canonical decomposition of $F$, where 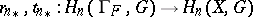 are homomorphisms and $t _ { n_{*} }$ is an isomorphism for any $n \geq 0$ (due to the Vietoris–Begle theorem). If $\Lambda ( F ) \neq \theta$, then $\operatorname {Fix} F \neq \emptyset$. This result was generalized by many authors (see [a9], [a10], [a4], [a7]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants.
are homomorphisms and $t _ { n_{*} }$ is an isomorphism for any $n \geq 0$ (due to the Vietoris–Begle theorem). If $\Lambda ( F ) \neq \theta$, then $\operatorname {Fix} F \neq \emptyset$. This result was generalized by many authors (see [a9], [a10], [a4], [a7]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants.
Degree theory.
To describe the topological characteristics of set-valued mappings $F$ like the degree $\operatorname { deg } F$ or the Kronecker characteristics $\gamma ( F )$ some definitions are needed. Let $X$, $Y$, $Z$ be separable topological spaces (cf. also Separable space), let $K ( Y )$ be the space of compact subsets, and suppose the set-valued mapping $F : X \rightarrow K ( Y )$ is upper semi-continuous. Such a mapping is called
$n$-acyclic if $\operatorname { rd }_{X} ( N _ { K } ( F ) ) \leq n - k - 2$ for all 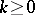 (here, $N _ { K } ( F ) \subset X$ is the set of points $x$ at which the $k$-acyclicity of the images $F ( x )$ is broken);
(here, $N _ { K } ( F ) \subset X$ is the set of points $x$ at which the $k$-acyclicity of the images $F ( x )$ is broken);
$F$-acyclic if it is $1$-acyclic; this is equivalent to acyclicity of every image $F ( x )$. A mapping $F : X \rightarrow K ( Y )$ is called generally $n$-acyclic if there exist a space $Z$ and single-valued continuous mappings $p : Z \rightarrow X$, $q : Z \rightarrow Y$, where $p$ is $n$-Vietoris and $q \circ p ^ { - 1 } ( x ) \subset F ( x )$ for all $x \in X$. The collection $\{ X , Y , Z , p , q \}$ is then said to be a representation of the set-valued mapping $F$, the pair $( p , q )$ is called a selecting pair, and the mapping $q \circ p ^ { - 1 }$ is called a selector of $F$. For an $n$-acyclic mapping $F$, the projections of the graph $t : X \times Y \supset \Gamma ( F ) \rightarrow X$, $r : X \times Y \supset \Gamma ( F ) \rightarrow Y$ give a selecting pair:
\begin{equation*} F ( x ) = r \circ t ^ { - 1 } ( x ). \end{equation*}
As an example, consider the main construction of the degree of a mapping $F : \overline { D } \square ^ { n + 1 } \rightarrow K ( E ^ { n + 1 } )$ from the unit disc $\overline { D } \square ^ { n + 1 } \subset E ^ { n + 1 }$ in the Euclidean space $E ^ { n + 1}$ under the condition that $F : S ^ { n } \rightarrow K ( E ^ { n + 1 } \backslash \theta )$, where $S ^ { n } = \partial \overline { D } \square ^ { n + 1 }$, is $m$-acyclic, $1 \leq m \leq n$. A generalization of the Vietoris–Begle theorem given by E.G. Sklyarenko ensures the existence of cohomology isomorphisms $t ^ { * } : H ^ { n } ( S ^ { n } ) \rightarrow H ^ { n } ( \Gamma _ { S ^ { n } } )$, $\hat { t } \square ^ { * } : H ^ { n + 1 } ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \rightarrow H ^ { n + 1 } ( \Gamma _ { \overline{D} \square ^ { n + 1 } } , \Gamma _ { S ^ { n } } )$ over the group $\bf Z$. Then $\operatorname { deg } ( F , \overline { D } \square ^ { n + 1 } , \theta ) = k$, where $k$ is given by the equality $( t ^ { * } ) ^ { - 1 } \circ ( t - r ) ^ { * } \beta _ { 1 } = k \beta _ { 2 }$. Here, $\beta _ { 1 }$, respectively $\beta_2$, is a generator of the group $H ^ { n } ( E ^ { n + 1 } \backslash \theta )$, respectively $H ^ { n } ( S ^ { n } )$, which is isomorphic to $\bf Z$, and $( t - r ) : ( \Gamma _ { S ^ { n } } ) \rightarrow ( E ^ { n + 1 } \backslash 0 )$ (a construction given by D.G. Bourgin, L. Górniewicz, and others, see [a9], [a10], [a4]). If the mapping $F : X \rightarrow K ( Y )$ under consideration is generally acyclic, then for every selecting pair $( p , q ) \subset F$ the set-valued mapping (the selector of $F$) $G = p \circ q ^ { - 1 } : X \rightarrow K ( Y )$ is $m$-acyclic, and for it $r = p$, $t = q$; applying the previous construction for the selector $G$ of the set-valued mapping $F : ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \rightarrow ( K ( E ^ { n + 1 } ) , K ( E ^ { n + 1 } \backslash \theta ) )$, one obtains $\operatorname { deg } ( G , \overline { D } \square ^ { n + 1 } , \theta )$ for any selector $G$. The generalized degree $\operatorname { Deg } ( F , \overline { D } \square ^ { n + 1 } , \theta )$ is the set $\{ \operatorname { deg } ( G , \overline { D } \square ^ { n + 1 } , \theta ) \}$ generated by all selecting pairs $( p , q )$ for the $n$-mapping $F$. A more general construction (without the condition that $F$ be $m$-acyclic) was introduced by B.D. Gelman (see [a10]); namely, the topological characteristic $\kappa ( F , \overline { D } \square ^ { n + 1 } ) = k$, where $k$ is defined by the equality
\begin{equation*} \delta ^ { * } \circ ( t - r ) ^ { * } \beta _ { 1 } = k ( \widehat{t ^ { * }} \square ^ { - 1 } \beta _ { 3 } ), \end{equation*}
$\beta_3$ is a generator in $H ^ { n + 1 } ( \overline { D } \square ^ { n + 1 } , S ^ { n } ) \cong \mathbf{Z}$, all the generators $\beta _ { i }$ are in accordance with the orientation of $E ^ { n + 1}$, and $\delta ^ { * } : H ^ { n } ( \Gamma _ { S ^ { n } } ) \rightarrow H ^ { n + 1 } ( \Gamma _ { \bar{D} \square ^ { n + 1 } } , \Gamma _ { S ^ { n } } )$ is a connecting homomorphism.
Note that an earlier definition of rotation of a set-valued field $\Phi x = x - F x$, $\Phi : \partial U \rightarrow E ^ { n + 1 } {\color{blue} \backslash} 0$, with non-acyclic images was given in [a8], [a13], [a14].
References
| [a1] | S. Eilenberg, N. Steenrod, "Foundations of algebraic topology" , Princeton Univ. Press (1952) |
| [a2] | E.H. Spanier, "Algebraic topology" , McGraw-Hill (1966) |
| [a3] | E.G. Begle, "The Vietoris mappings theorem for bicompact spaces" Ann. of Math. , 51 : 2 (1950) pp. 534–550 |
| [a4] | L. Górniewicz, "Homological methods in fixed-point theory of multi-valued maps" Dissert. Math. , CXXIX (1976) pp. 1–71 |
| [a5] | E.G. Sklyarenko, "Of some applications of theory of bundles in general topology" Uspekhi Mat. Nauk , 19 : 6 (1964) pp. 47–70 (In Russian) |
| [a6] | S. Eilenberg, D. Montgomery, "Fixed point theorems for multi-valued transformations" Amer. J. Math. , 68 (1946) pp. 214–222 |
| [a7] | A. Granas, J.W. Jaworowski, "Some theorems on multi-valued maps of subsets of the Euclidean space" Bull. Acad. Polon. Sci. , 7 : 5 (1959) pp. 277–283 |
| [a8] | Yu.G. Borisovich, B.D. Gelman, V.V. Obukhovskii, "Of some topological invariants of set-valued maps with nonconvex images" Proc. Sem. Functional Analysis, Voronezh State Univ. , 12 (1969) pp. 85–95 |
| [a9] | Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Topological methods in the fixed-point theory of multi-valued maps" Russian Math. Surveys , 35 : 1 (1980) pp. 65–143 (In Russian) |
| [a10] | Yu.G. Borisovich, B.D. Gelman, A.D. Myshkis, V.V. Obukhovskii, "Multivalued mappings" J. Soviet Math. , 24 (1984) pp. 719–791 (In Russian) |
| [a11] | Yu.G. Borisovich, "A modern appoach to the theory of topological characteristics of nonlinear operators II" , Global analysis: Studies and Applications IV , Lecture Notes Math. , 1453 , Springer (1990) pp. 21–49 |
| [a12] | Yu.G. Borisovich, N.M. Bliznyakov, T.N. Fomenko, Y.A. Izrailevich, "Introduction to differential and algebraic topology" , Kluwer Acad. Publ. (1995) |
| [a13] | L. Górniewicz, "On non-acyclic multi-valued mappings of subsets of Euclidean spaces" Bull. Acad. Polon. Sci. , 20 : 5 (1972) pp. 379–385 |
| [a14] | D.G. Bouvgin, "Cones and Vietoris–Begle type theorems" Trans. Amer. Math. Soc. , 174 (1972) pp. 155–183 |
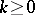 [a4]. From the previous statement it follows that for an $n$-Vietoris mapping $f : X \rightarrow Y$ the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is an isomorphism for $q \geq n$. For a $1$-Vietoris mapping, $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) = G , H ^ { q } ( f ^ { - 1 } ( y ) , G ) = 0$, for all $q > 0$, i.e. all the pre-images $f ^ { - 1 } ( y )$ are acyclic; such mappings are called Vietoris mappings.
[a4]. From the previous statement it follows that for an $n$-Vietoris mapping $f : X \rightarrow Y$ the homomorphism $f ^ { * } : H ^ { q } ( Y , G ) \rightarrow H ^ { q } ( X , G )$ is an isomorphism for $q \geq n$. For a $1$-Vietoris mapping, $H ^ { 0 } ( f ^ { - 1 } ( y ) , G ) = G , H ^ { q } ( f ^ { - 1 } ( y ) , G ) = 0$, for all $q > 0$, i.e. all the pre-images $f ^ { - 1 } ( y )$ are acyclic; such mappings are called Vietoris mappings.
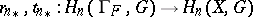 are homomorphisms and $t _ { n_{*} }$ is an isomorphism for any $n \geq 0$ (due to the Vietoris–Begle theorem). If $\Lambda ( F ) \neq \theta$, then $\operatorname {Fix} F \neq \emptyset$. This result was generalized by many authors (see [a9], [a10], [a4], [a7]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants.
are homomorphisms and $t _ { n_{*} }$ is an isomorphism for any $n \geq 0$ (due to the Vietoris–Begle theorem). If $\Lambda ( F ) \neq \theta$, then $\operatorname {Fix} F \neq \emptyset$. This result was generalized by many authors (see [a9], [a10], [a4], [a7]). These generalizations involve weaker conditions of acyclicity, as well as certain different variants.
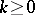 (here, $N _ { K } ( F ) \subset X$ is the set of points $x$ at which the $k$-acyclicity of the images $F ( x )$ is broken);
(here, $N _ { K } ( F ) \subset X$ is the set of points $x$ at which the $k$-acyclicity of the images $F ( x )$ is broken);