Difference between revisions of "Bramble-Hilbert lemma"
Ulf Rehmann (talk | contribs) m (moved Bramble–Hilbert lemma to Bramble-Hilbert lemma: ascii title) |
m (AUTOMATIC EDIT (latexlist): Replaced 121 formulas out of 125 by TEX code with an average confidence of 2.0 and a minimal confidence of 2.0.) |
||
| Line 1: | Line 1: | ||
| + | <!--This article has been texified automatically. Since there was no Nroff source code for this article, | ||
| + | the semi-automatic procedure described at https://encyclopediaofmath.org/wiki/User:Maximilian_Janisch/latexlist | ||
| + | was used. | ||
| + | If the TeX and formula formatting is correct, please remove this message and the {{TEX|semi-auto}} category. | ||
| + | |||
| + | Out of 125 formulas, 121 were replaced by TEX code.--> | ||
| + | |||
| + | {{TEX|semi-auto}}{{TEX|partial}} | ||
''Hilbert–Bramble lemma'' | ''Hilbert–Bramble lemma'' | ||
| − | An abstract theoretical tool for studying the approximation error of functions in Sobolev spaces (cf. also [[Approximation of functions|Approximation of functions]]; [[Sobolev space|Sobolev space]]) by algebraic polynomials. The general formulation of the lemma was given and proven first by J.H. Bramble and S.R. Hilbert [[#References|[a1]]] in terms of a class of linear functionals on Sobolev spaces that annihilate the set of polynomials | + | An abstract theoretical tool for studying the approximation error of functions in Sobolev spaces (cf. also [[Approximation of functions|Approximation of functions]]; [[Sobolev space|Sobolev space]]) by algebraic polynomials. The general formulation of the lemma was given and proven first by J.H. Bramble and S.R. Hilbert [[#References|[a1]]] in terms of a class of linear functionals on Sobolev spaces that annihilate the set of polynomials $P _ { K }$ (see the definition below), an intermediate to $P _ { k - 1}$ (polynomials of degree $k - 1$) and $P _ { k }$, i.e. $P _ { k - 1 } \subset P _ { K } \subset P _ { k }$. This formulation was motivated by the work of G. Birkhoff, M. Schultz and R.S. Varga [[#References|[a3]]] and applied to estimate the error of the [[Hermite interpolation formula|Hermite interpolation formula]] [[#References|[a1]]], [[Spline interpolation|spline interpolation]] and Fourier transformation (cf. [[Fourier transform|Fourier transform]]) [[#References|[a2]]]. |
| − | The Bramble–Hilbert lemma has a wide range of applications. It is used in the analysis of projection operators in the function spaces | + | The Bramble–Hilbert lemma has a wide range of applications. It is used in the analysis of projection operators in the function spaces $L_{2}$ and $W _ { 2 } ^ { 1 }$ for designing optimal domain decomposition and multi-level methods. Its application to the $L_{2}$-error of the finite element approximations of elliptic problems of second order leads to sharp estimates with respect to both the regularity of the solution and rate of convergence. It is an indispensible tool in the error analysis of finite element [[#References|[a4]]], [[#References|[a5]]], finite volume [[#References|[a8]]], finite difference [[#References|[a9]]], collocation, and boundary element methods for solving partial differential equations. The complete error analysis of the finite difference and finite volume schemes in [[#References|[a9]]] is based on the Bramble–Hilbert lemma. |
| − | To formulate the lemma, some notation regarding Sobolev spaces of real-valued functions on a bounded domain | + | To formulate the lemma, some notation regarding Sobolev spaces of real-valued functions on a bounded domain $T$ in $N$-dimensional Euclidean space $\mathbf{R} ^ { N }$ is needed. It is assumed that $T$ satisfies the strong cone condition (cf. [[Cone condition|Cone condition]]) and has diameter $\rho = \rho ( T ) = \operatorname { diam } ( T )$. The boundary of $T$ is denoted by $\partial T$. |
| − | The notations | + | The notations $\alpha$, $\beta$ and $\gamma$ will be used for multi-indices, with $| \alpha | = \sum _ { j = 1 } ^ { N } \alpha _ { j }$ and $D ^ { \alpha } = D _ { 1 } ^ { \alpha _ { 1 } } \ldots D _ { N } ^ { \alpha _ { N } }$, where $D _ { j } = \partial / \partial x_ { j } $. Let $L _ { p } ( T )$ be the set of all functions $u$ such that $\int _ { T } | u ( x ) | ^ { p } d x$ exists and is finite. The [[Norm|norm]] in $L _ { p }$ is given by $\| u \| _ { p , T } = ( \int _ { T } | u ( x ) | ^ { p } d x ) ^ { 1 / p }$. Let $W _ { p } ^ { m } ( T )$ be the set of all functions in $L _ { p } ( T )$ whose distributional derivatives of order less than equal to $m$ (a non-negative integer) are in $L _ { p } ( T )$. It will be assumed that $1 \leq p < \infty$. The norm and the [[Semi-norm|semi-norm]] on $W _ { p } ^ { m } ( T )$ are, respectively, |
| − | + | \begin{equation*} \| u \| _ { p , m , T } = \sum _ { | \alpha | \leq m } \| D ^ { \alpha } u \| _ { p , T }, \end{equation*} | |
| − | + | \begin{equation*} | u | _ { p , m , T } = \sum _ { | \alpha | = m } \| D ^ { \alpha } u \| _ { p , T }. \end{equation*} | |
| − | Let | + | Let $K$ be any subset of the set of multi-indices $\gamma$ of length $m$ (i.e. $| \gamma | = m$) which contains the indices of the form $\gamma _ { l } = m$, $\gamma_j = 0$ for $j \neq l$, $l = 1 , \ldots , N$. The set of polynomials $q$ such that $D ^ { \gamma } q = 0$ for all $\gamma \in K$ will be denoted by $P _ { K }$. Obviously, $P _ { k - 1 } \subset P _ { K } \subset P _ { k }$. |
| − | The Bramble–Hilbert lemma ([[#References|[a1]]]) is as follows: Let | + | The Bramble–Hilbert lemma ([[#References|[a1]]]) is as follows: Let $F ( u )$ be a bounded [[Linear functional|linear functional]] on $W _ { p } ^ { m } ( T )$ that vanishes for polynomials in $P _ { K }$, i.e. |
| − | 1) | + | 1) $| F ( u ) | \leq C \sum _ { j = 0 } ^ { m } \rho ^ { j - N / p } | u | _ { p , j , T }$, where the constant $C$ is independent of $\rho$ and the function $u$; |
| − | 2) | + | 2) $F ( q ) = 0$ for $q \in P _ { K }$. Then there is a constant $C _ { 1 }$, independent of $\rho$ and $u$, such that |
| − | + | \begin{equation*} | F ( u ) | \leq C _ { 1 } \sum _ { \alpha \in K } \rho ^ { m - N / p } \| D ^ { \alpha } u \| _ { p , T }. \end{equation*} | |
Note that the lemma deals with function spaces of many variables. For functions in a single variable, the inequality derived by the lemma follows immediately from the Peano kernel theorem for the remainder of the Taylor expansion (see, e.g., [[#References|[a10]]]). | Note that the lemma deals with function spaces of many variables. For functions in a single variable, the inequality derived by the lemma follows immediately from the Peano kernel theorem for the remainder of the Taylor expansion (see, e.g., [[#References|[a10]]]). | ||
| − | In many applications, a given domain | + | In many applications, a given domain $\Omega$ is split into a number of non-overlapping subdomains $T$, usually triangles, rectangles, tetrahedra, hexahedra, etc., so that $\rho = \rho ( T )$ is small. Consider the error of the [[Lagrange interpolation formula|Lagrange interpolation formula]] for a function $u \in W _ { p } ^ { m } ( \Omega )$ with polynomials of degree $m - 1$ over each $T$ and take $\overline { \Omega } = \cup \overline{T}$. Here, the domains $T$ are non-degenerate simplices in $\mathbf{R} ^ { N }$, $\rho = \operatorname { max } _ { T } \rho ( T )$, $K = \{ \gamma : | \gamma | = m \}$, and $P _ { K } = P _ { m - 1 }$. Assume that $p > N$ and $m = 2$ and let $q_{l}$ be a piecewise polynomial of degree $1$ which interpolates the values of the function $u$ at the vertices of each simplex $T$. Consider the linear functional $F ( u ) = u ( x ) - q _{I} ( x )$ at a fixed point $x \in T$. By the Sobolev imbedding theorem (cf. also [[Imbedding theorems|Imbedding theorems]]), |
| − | + | \begin{equation*} | u ( x ) | \leq C \sum _ { j = 0 } ^ { 2 } \rho ^ { j - N / p } | u | _ { p , j , T }. \end{equation*} | |
| − | Therefore | + | Therefore $F ( u )$ is bounded in $W _ { p } ^ { m } ( T )$. Obviously, $F ( u ) = 0$ if $u$ is a polynomial of degree $1$, since the linear interpolant recovers $u$ exactly. Therefore, by the Bramble–Hilbert lemma: $| F ( u ) | \leq C _ { 1 } \rho ^ { 2 - N / p } | u | _ { p , 2 , T }$. An estimate of the $L _ { p }$-error for the interpolant $q_{l}$ over $T$ follows immediately after taking this inequality to power $p$ and integrating it over $T$. The estimate for the error in the whole domain $\Omega$ follows by summing all local estimates, taking the maximal $\rho$, and using the additivity of the integrals: |
| − | + | \begin{equation*} \| u - q _ { l } \| _ { p , \Omega } \leq C \rho ^ { 2 } | u | _ { p , 2 , \Omega }. \end{equation*} | |
| − | If | + | If $\rho$ tends to $0$, this estimate gives the rate at which the piecewise-linear interpolant converges to $u$ in the $L _ { p }$-norm. Similarly, one can obtain bounds for the $L _ { p }$-norm of the first derivatives. This is a typical application of the Bramble–Hilbert lemma in constructive function theory and in numerical methods for differential equations. |
| − | The original proof of the lemma was abstract and the size of the constant | + | The original proof of the lemma was abstract and the size of the constant $C _ { 1 }$ could not be estimated. This was the reason that T. Dupont and L.R. Scott [[#References|[a7]]] gave a new constructive proof of the main result. |
| − | Consider the polynomial | + | Consider the polynomial $Q_m u$ which is an averaged Taylor polynomial of degree $m - 1$ over $T$ (for the exact construction, see [[#References|[a4]]], Chap. 4). This construction of $Q_m u$ is related to the polynomials used by S.L. Sobolev [[#References|[a11]]] to study the spaces that bear his name. Here, $T$ is considered to be a star-shaped domain with respect to a ball $B$, that is, for all $x \in B$, the closed convex hull of $\{ x \} \cup B$ is a subset of $T$, and one defines |
| − | + | \begin{equation*} \rho _ { \operatorname { max } } = \operatorname { sup } \{ \rho = \rho ( B ) : T\, \text { star shaped w.r.t. } B \}. \end{equation*} | |
| − | The revised Bramble–Hilbert lemma now reads ([[#References|[a4]]], p. 100): Let | + | The revised Bramble–Hilbert lemma now reads ([[#References|[a4]]], p. 100): Let $T$ be a star-shaped domain with respect to the ball $B$. Then |
| − | <table class="eq" style="width:100%;"> <tr><td | + | <table class="eq" style="width:100%;"> <tr><td style="width:94%;text-align:center;" valign="top"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b130/b130220/b130220115.png"/></td> </tr></table> |
| − | + | \begin{equation*} k = 0 , \dots , m. \end{equation*} | |
| − | Here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b130/b130220/b130220117.png" /> is the quotient of | + | Here, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b130/b130220/b130220117.png"/> is the quotient of $\rho$ and the diameter <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/b/b130/b130220/b130220119.png"/>, and the constant $C _ { m , N, \epsilon}$ does not depend on $\rho$ while its dependence on $m$ and $k$ is estimated explicitly from above. |
| − | This variant of the lemma was proved by Dupont and Scott [[#References|[a7]]] for | + | This variant of the lemma was proved by Dupont and Scott [[#References|[a7]]] for $m$ non-integer and for domains that are finite unions of domains that are star-shaped with respect to a ball, while more general results were established in [[#References|[a6]]]. |
====References==== | ====References==== | ||
| − | <table>< | + | <table><tr><td valign="top">[a1]</td> <td valign="top"> J.H. Bramble, S.R. Hilbert, "Bounds for a class of linear functionals with applications to Hermite interpolation" ''Numer. Math.'' , '''16''' (1971) pp. 362–369</td></tr><tr><td valign="top">[a2]</td> <td valign="top"> J.H. Bramble, S.R. Hilbert, "Estimation of linear functionals on Sobolev spaces with application to Fourier transforms and spline interpolation" ''SIAM J. Numer. Anal.'' , '''7''' (1970) pp. 112–124</td></tr><tr><td valign="top">[a3]</td> <td valign="top"> G. Birkhoff, M. Schultz, R. Varga, "Hermite interpolation in one and two variables with application to partial differential equations" ''Numer. Math.'' , '''11''' (1968) pp. 232–256</td></tr><tr><td valign="top">[a4]</td> <td valign="top"> S.C. Brenner, L.R. Scott, "The mathematical theory of finite element methods" , Springer (1994)</td></tr><tr><td valign="top">[a5]</td> <td valign="top"> P.G. Ciarlet, "The finite element method for elliptic problems" , North-Holland (1978)</td></tr><tr><td valign="top">[a6]</td> <td valign="top"> L.T. Dechevski, E. Quak, "On the Bramble–Hilbert lemma" ''Numer. Funct. Anal. Optim.'' , '''11''' (1990) pp. 485–495</td></tr><tr><td valign="top">[a7]</td> <td valign="top"> T. Dupont, L.R. Scott, "Polynomial approximation of functionals in Sobolev spaces" ''Math. Comput.'' , '''34''' (1979) pp. 441–463</td></tr><tr><td valign="top">[a8]</td> <td valign="top"> R. Li, Z. Chen, W. Wu, "Generalized difference methods for differential equations. Numerical analysis of finite volume methods" , M. Dekker (2000)</td></tr><tr><td valign="top">[a9]</td> <td valign="top"> A.A. Samarskii, R.D. Lazarov, V.L. Makarov, "Finite difference schemes for differential equations having generalized solutions" , Visshaya Shkola Publ., Moscow (1987) (In Russian)</td></tr><tr><td valign="top">[a10]</td> <td valign="top"> A. Sard, "Linear approximation" , ''Math. Surveys'' , '''9''' , Amer. Math. Soc. (1963)</td></tr><tr><td valign="top">[a11]</td> <td valign="top"> S.L. Sobolev, "Applications of the functional analysis in mathematical physics" , Amer. Math. Soc. (1963) (In Russian)</td></tr></table> |
Revision as of 16:57, 1 July 2020
Hilbert–Bramble lemma
An abstract theoretical tool for studying the approximation error of functions in Sobolev spaces (cf. also Approximation of functions; Sobolev space) by algebraic polynomials. The general formulation of the lemma was given and proven first by J.H. Bramble and S.R. Hilbert [a1] in terms of a class of linear functionals on Sobolev spaces that annihilate the set of polynomials $P _ { K }$ (see the definition below), an intermediate to $P _ { k - 1}$ (polynomials of degree $k - 1$) and $P _ { k }$, i.e. $P _ { k - 1 } \subset P _ { K } \subset P _ { k }$. This formulation was motivated by the work of G. Birkhoff, M. Schultz and R.S. Varga [a3] and applied to estimate the error of the Hermite interpolation formula [a1], spline interpolation and Fourier transformation (cf. Fourier transform) [a2].
The Bramble–Hilbert lemma has a wide range of applications. It is used in the analysis of projection operators in the function spaces $L_{2}$ and $W _ { 2 } ^ { 1 }$ for designing optimal domain decomposition and multi-level methods. Its application to the $L_{2}$-error of the finite element approximations of elliptic problems of second order leads to sharp estimates with respect to both the regularity of the solution and rate of convergence. It is an indispensible tool in the error analysis of finite element [a4], [a5], finite volume [a8], finite difference [a9], collocation, and boundary element methods for solving partial differential equations. The complete error analysis of the finite difference and finite volume schemes in [a9] is based on the Bramble–Hilbert lemma.
To formulate the lemma, some notation regarding Sobolev spaces of real-valued functions on a bounded domain $T$ in $N$-dimensional Euclidean space $\mathbf{R} ^ { N }$ is needed. It is assumed that $T$ satisfies the strong cone condition (cf. Cone condition) and has diameter $\rho = \rho ( T ) = \operatorname { diam } ( T )$. The boundary of $T$ is denoted by $\partial T$.
The notations $\alpha$, $\beta$ and $\gamma$ will be used for multi-indices, with $| \alpha | = \sum _ { j = 1 } ^ { N } \alpha _ { j }$ and $D ^ { \alpha } = D _ { 1 } ^ { \alpha _ { 1 } } \ldots D _ { N } ^ { \alpha _ { N } }$, where $D _ { j } = \partial / \partial x_ { j } $. Let $L _ { p } ( T )$ be the set of all functions $u$ such that $\int _ { T } | u ( x ) | ^ { p } d x$ exists and is finite. The norm in $L _ { p }$ is given by $\| u \| _ { p , T } = ( \int _ { T } | u ( x ) | ^ { p } d x ) ^ { 1 / p }$. Let $W _ { p } ^ { m } ( T )$ be the set of all functions in $L _ { p } ( T )$ whose distributional derivatives of order less than equal to $m$ (a non-negative integer) are in $L _ { p } ( T )$. It will be assumed that $1 \leq p < \infty$. The norm and the semi-norm on $W _ { p } ^ { m } ( T )$ are, respectively,
\begin{equation*} \| u \| _ { p , m , T } = \sum _ { | \alpha | \leq m } \| D ^ { \alpha } u \| _ { p , T }, \end{equation*}
\begin{equation*} | u | _ { p , m , T } = \sum _ { | \alpha | = m } \| D ^ { \alpha } u \| _ { p , T }. \end{equation*}
Let $K$ be any subset of the set of multi-indices $\gamma$ of length $m$ (i.e. $| \gamma | = m$) which contains the indices of the form $\gamma _ { l } = m$, $\gamma_j = 0$ for $j \neq l$, $l = 1 , \ldots , N$. The set of polynomials $q$ such that $D ^ { \gamma } q = 0$ for all $\gamma \in K$ will be denoted by $P _ { K }$. Obviously, $P _ { k - 1 } \subset P _ { K } \subset P _ { k }$.
The Bramble–Hilbert lemma ([a1]) is as follows: Let $F ( u )$ be a bounded linear functional on $W _ { p } ^ { m } ( T )$ that vanishes for polynomials in $P _ { K }$, i.e.
1) $| F ( u ) | \leq C \sum _ { j = 0 } ^ { m } \rho ^ { j - N / p } | u | _ { p , j , T }$, where the constant $C$ is independent of $\rho$ and the function $u$;
2) $F ( q ) = 0$ for $q \in P _ { K }$. Then there is a constant $C _ { 1 }$, independent of $\rho$ and $u$, such that
\begin{equation*} | F ( u ) | \leq C _ { 1 } \sum _ { \alpha \in K } \rho ^ { m - N / p } \| D ^ { \alpha } u \| _ { p , T }. \end{equation*}
Note that the lemma deals with function spaces of many variables. For functions in a single variable, the inequality derived by the lemma follows immediately from the Peano kernel theorem for the remainder of the Taylor expansion (see, e.g., [a10]).
In many applications, a given domain $\Omega$ is split into a number of non-overlapping subdomains $T$, usually triangles, rectangles, tetrahedra, hexahedra, etc., so that $\rho = \rho ( T )$ is small. Consider the error of the Lagrange interpolation formula for a function $u \in W _ { p } ^ { m } ( \Omega )$ with polynomials of degree $m - 1$ over each $T$ and take $\overline { \Omega } = \cup \overline{T}$. Here, the domains $T$ are non-degenerate simplices in $\mathbf{R} ^ { N }$, $\rho = \operatorname { max } _ { T } \rho ( T )$, $K = \{ \gamma : | \gamma | = m \}$, and $P _ { K } = P _ { m - 1 }$. Assume that $p > N$ and $m = 2$ and let $q_{l}$ be a piecewise polynomial of degree $1$ which interpolates the values of the function $u$ at the vertices of each simplex $T$. Consider the linear functional $F ( u ) = u ( x ) - q _{I} ( x )$ at a fixed point $x \in T$. By the Sobolev imbedding theorem (cf. also Imbedding theorems),
\begin{equation*} | u ( x ) | \leq C \sum _ { j = 0 } ^ { 2 } \rho ^ { j - N / p } | u | _ { p , j , T }. \end{equation*}
Therefore $F ( u )$ is bounded in $W _ { p } ^ { m } ( T )$. Obviously, $F ( u ) = 0$ if $u$ is a polynomial of degree $1$, since the linear interpolant recovers $u$ exactly. Therefore, by the Bramble–Hilbert lemma: $| F ( u ) | \leq C _ { 1 } \rho ^ { 2 - N / p } | u | _ { p , 2 , T }$. An estimate of the $L _ { p }$-error for the interpolant $q_{l}$ over $T$ follows immediately after taking this inequality to power $p$ and integrating it over $T$. The estimate for the error in the whole domain $\Omega$ follows by summing all local estimates, taking the maximal $\rho$, and using the additivity of the integrals:
\begin{equation*} \| u - q _ { l } \| _ { p , \Omega } \leq C \rho ^ { 2 } | u | _ { p , 2 , \Omega }. \end{equation*}
If $\rho$ tends to $0$, this estimate gives the rate at which the piecewise-linear interpolant converges to $u$ in the $L _ { p }$-norm. Similarly, one can obtain bounds for the $L _ { p }$-norm of the first derivatives. This is a typical application of the Bramble–Hilbert lemma in constructive function theory and in numerical methods for differential equations.
The original proof of the lemma was abstract and the size of the constant $C _ { 1 }$ could not be estimated. This was the reason that T. Dupont and L.R. Scott [a7] gave a new constructive proof of the main result.
Consider the polynomial $Q_m u$ which is an averaged Taylor polynomial of degree $m - 1$ over $T$ (for the exact construction, see [a4], Chap. 4). This construction of $Q_m u$ is related to the polynomials used by S.L. Sobolev [a11] to study the spaces that bear his name. Here, $T$ is considered to be a star-shaped domain with respect to a ball $B$, that is, for all $x \in B$, the closed convex hull of $\{ x \} \cup B$ is a subset of $T$, and one defines
\begin{equation*} \rho _ { \operatorname { max } } = \operatorname { sup } \{ \rho = \rho ( B ) : T\, \text { star shaped w.r.t. } B \}. \end{equation*}
The revised Bramble–Hilbert lemma now reads ([a4], p. 100): Let $T$ be a star-shaped domain with respect to the ball $B$. Then
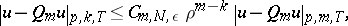 |
\begin{equation*} k = 0 , \dots , m. \end{equation*}
Here,  is the quotient of $\rho$ and the diameter
is the quotient of $\rho$ and the diameter  , and the constant $C _ { m , N, \epsilon}$ does not depend on $\rho$ while its dependence on $m$ and $k$ is estimated explicitly from above.
, and the constant $C _ { m , N, \epsilon}$ does not depend on $\rho$ while its dependence on $m$ and $k$ is estimated explicitly from above.
This variant of the lemma was proved by Dupont and Scott [a7] for $m$ non-integer and for domains that are finite unions of domains that are star-shaped with respect to a ball, while more general results were established in [a6].
References
| [a1] | J.H. Bramble, S.R. Hilbert, "Bounds for a class of linear functionals with applications to Hermite interpolation" Numer. Math. , 16 (1971) pp. 362–369 |
| [a2] | J.H. Bramble, S.R. Hilbert, "Estimation of linear functionals on Sobolev spaces with application to Fourier transforms and spline interpolation" SIAM J. Numer. Anal. , 7 (1970) pp. 112–124 |
| [a3] | G. Birkhoff, M. Schultz, R. Varga, "Hermite interpolation in one and two variables with application to partial differential equations" Numer. Math. , 11 (1968) pp. 232–256 |
| [a4] | S.C. Brenner, L.R. Scott, "The mathematical theory of finite element methods" , Springer (1994) |
| [a5] | P.G. Ciarlet, "The finite element method for elliptic problems" , North-Holland (1978) |
| [a6] | L.T. Dechevski, E. Quak, "On the Bramble–Hilbert lemma" Numer. Funct. Anal. Optim. , 11 (1990) pp. 485–495 |
| [a7] | T. Dupont, L.R. Scott, "Polynomial approximation of functionals in Sobolev spaces" Math. Comput. , 34 (1979) pp. 441–463 |
| [a8] | R. Li, Z. Chen, W. Wu, "Generalized difference methods for differential equations. Numerical analysis of finite volume methods" , M. Dekker (2000) |
| [a9] | A.A. Samarskii, R.D. Lazarov, V.L. Makarov, "Finite difference schemes for differential equations having generalized solutions" , Visshaya Shkola Publ., Moscow (1987) (In Russian) |
| [a10] | A. Sard, "Linear approximation" , Math. Surveys , 9 , Amer. Math. Soc. (1963) |
| [a11] | S.L. Sobolev, "Applications of the functional analysis in mathematical physics" , Amer. Math. Soc. (1963) (In Russian) |
Bramble-Hilbert lemma. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bramble-Hilbert_lemma&oldid=22183