Difference between revisions of "Conformal invariants"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 7: | Line 7: | ||
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018026.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018026.png" /></td> </tr></table> | ||
| − | on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018027.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018028.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018029.png" /> is the signature of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018030.png" />. A manifold is (locally) conformally flat if it is locally conformally equivalent to a flat manifold; the modifier | + | on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018027.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018028.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018029.png" /> is the signature of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018030.png" />. A manifold is (locally) conformally flat if it is locally conformally equivalent to a flat manifold; the modifier "locally" is a tacit part of the definition, normally omitted. Clearly, conformally flat manifolds have no non-trivial conformal invariants. |
For any smooth manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018031.png" />, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018032.png" /> be the ring of smooth real-valued functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018033.png" /> (regarded as an algebra over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018034.png" />), let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018035.png" /> be the usual <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018036.png" />-module of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018037.png" />-forms over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018038.png" />, and for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018039.png" />, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018040.png" /> denote the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018041.png" />-fold tensor product <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018042.png" /> over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018043.png" />. In particular, the non-degenerate symmetric form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018044.png" /> of a Riemannian manifold will be regarded as a symmetric element of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018045.png" />, as above. The conformal invariance condition <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018046.png" /> is entirely local, so that one may as well assume that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018047.png" /> is itself an open set in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018048.png" />. One finds that the signature is of little interest in the construction of conformal invariants, since strategically placed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018049.png" /> signs turn constructions for the strictly Riemannian case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018050.png" /> into corresponding constructions for the general case. Hence the existence of conformal invariants depends only on the dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018051.png" />. | For any smooth manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018031.png" />, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018032.png" /> be the ring of smooth real-valued functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018033.png" /> (regarded as an algebra over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018034.png" />), let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018035.png" /> be the usual <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018036.png" />-module of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018037.png" />-forms over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018038.png" />, and for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018039.png" />, let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018040.png" /> denote the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018041.png" />-fold tensor product <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018042.png" /> over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018043.png" />. In particular, the non-degenerate symmetric form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018044.png" /> of a Riemannian manifold will be regarded as a symmetric element of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018045.png" />, as above. The conformal invariance condition <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018046.png" /> is entirely local, so that one may as well assume that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018047.png" /> is itself an open set in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018048.png" />. One finds that the signature is of little interest in the construction of conformal invariants, since strategically placed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018049.png" /> signs turn constructions for the strictly Riemannian case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018050.png" /> into corresponding constructions for the general case. Hence the existence of conformal invariants depends only on the dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c12018051.png" />. | ||
| Line 168: | Line 168: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Bach, "Zur Weylschen Relativitätstheorie und der Weylschen Erweiterung des Krümmungstensorbegriffs" ''Math. Z.'' , '''9''' (1921) pp. 110–135 (Also: Jahrbuch 48, 1035) {{MR|1544454}} {{ZBL|48.1035.01}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> T.N. Bailey, M.G. Eastwood, C.R. Graham, "Invariant theory for conformal and CR geometry" ''Ann. of Math.'' , '''139''' : 2 (1994) pp. 491–552 {{MR|1283869}} {{ZBL|0814.53017}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> T.N. Bailey, A.R. Gover, "Exceptional invariants in the parabolic invariant theory of conformal geometry" ''Proc. Amer. Math. Soc.'' , '''123''' (1995) pp. 2535–2543 {{MR|1243161}} {{ZBL|0844.53008}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> S.S. Chern, "An elementary proof of the existence of isothermal parameters on a surface" ''Proc. Amer. Math. Soc.'' , '''6''' (1955) pp. 771–782 {{MR|0074856}} {{ZBL|0066.15402}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> S.S. Chern, "On a conformal invariant of three-dimensional manifolds" , ''Aspects of Math. and its Appl.'' , North-Holland (1986) pp. 245–252 {{MR|0849555}} {{ZBL|0589.53011}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S.S. Chern, J. Simons, "Characteristic forms and geometric invariants" ''Ann. of Math.'' , '''99''' (1974) pp. 48–69 {{MR|0353327}} {{ZBL|0283.53036}} {{ZBL|0591.53050}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> E. Cotton, "Sur les variétes à trois dimensions" ''Ann. Fac. Sci. Toulouse'' , '''1''' (1899) pp. 385–438 (Also: Jahrbuch 30, 538-539) {{MR|1508211}} {{ZBL|30.0538.01}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> C. Fefferman, C.R. Graham, "Conformal invariants" , ''The Mathematical Heritage of Élie Cartan (Lyon, 1984)'' , ''Astérisque'' (1985) pp. 95–116 {{MR|0837196}} {{ZBL|0602.53007}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> A. Korn, "Zwei Anwendungen der Methode der sukzessiven Anwendungen" ''Schwarz Festschrift'' (1914) pp. 215–229 (Also: Jahrbuch 45, 568) {{MR|}} {{ZBL|45.0568.01}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> L. Lichtenstein, "Zur Theorie der konformen Abbildungen nichtanalytischer, singularitätenfreier Flächenstücke auf ebene Gebiete" ''Bull. Internat. Acad. Sci. Gracovie, Cl. Sci. Math. Nat. Ser. A.'' (1916) pp. 192–217 (Also: Jahrbuch 46, 547)</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> H. Osborn, "Affine connection complexes" ''Acta Applic. Math.'' (to appear) {{MR|1741659}} {{ZBL|0956.53014}} </TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> J.A. Schouten, "Über die konforme Abbildung <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c120/c120180/c120180509.png" />-dimensionaler Mannigfaltigkeiten mit quadratischer Maß bestimmung auf eine Mannigfaltigkeit mit euklidischer Maß bestimmung" ''Math. Z.'' , '''11''' (1921) pp. 58–88 (Also: Jahrbuch 48, 857-858)</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> J.A. Schouten, J. Haantjes, "Beitgräge zur allgemeinen (gekrümmten) konformen Differentialgeometrie I–II" ''Math. Ann.'' , '''112/113''' (1936) pp. 594–629; 568–583</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> H. Weyl, "Reine Infinitesimalgeometrie" ''Math. Z.'' , '''2''' (1918) pp. 384–411 (Also: Jahrbuch 46, 1301) {{MR|1544327}} {{ZBL|46.1301.01}} </TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> H. Weyl, "The classical groups" , Princeton Univ. Press (1939) (Reprint: 1946) {{MR|0000255}} {{ZBL|0020.20601}} {{ZBL|65.0058.02}} </TD></TR></table> |
Revision as of 10:54, 1 April 2012
Let 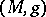 be any Riemannian manifold, consisting of a smooth manifold
be any Riemannian manifold, consisting of a smooth manifold  and a non-degenerate symmetric form
and a non-degenerate symmetric form 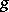 on the tangent bundle of
on the tangent bundle of 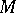 , not necessarily positive-definite. By definition, for any strictly positive smooth function
, not necessarily positive-definite. By definition, for any strictly positive smooth function 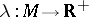 the Riemannian manifold
the Riemannian manifold 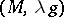 is conformally equivalent to
is conformally equivalent to  (cf. also Conformal mapping), and a tensor
(cf. also Conformal mapping), and a tensor 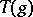 (cf. also Tensor analysis) constructed from
(cf. also Tensor analysis) constructed from  and its covariant derivatives is a conformal invariant if and only if for some fixed weight
and its covariant derivatives is a conformal invariant if and only if for some fixed weight  the tensor
the tensor  is independent of
is independent of 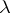 . The tensor
. The tensor 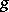 is itself a trivial conformal invariant of weight
is itself a trivial conformal invariant of weight 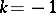 , and the dimension of
, and the dimension of 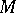 and signature of
and signature of 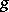 can be regarded as trivial conformal invariants, of weight
can be regarded as trivial conformal invariants, of weight 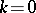 . However, there are many non-trivial conformal invariants of Riemannian manifolds of dimension
. However, there are many non-trivial conformal invariants of Riemannian manifolds of dimension 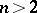 , and non-trivial scalar conformal invariants have been the subject of much recent work, sketched below. One can also extend the definition to include conformal invariants that are not tensors; these will not be considered below.
, and non-trivial scalar conformal invariants have been the subject of much recent work, sketched below. One can also extend the definition to include conformal invariants that are not tensors; these will not be considered below.
An  -dimensional Riemannian manifold
-dimensional Riemannian manifold  is flat in a neighbourhood
is flat in a neighbourhood 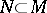 of a point
of a point 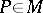 if there are coordinate functions
if there are coordinate functions 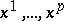 ,
, 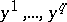 such that
such that
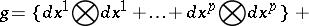 |
 |
on  , where
, where 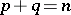 and
and  is the signature of
is the signature of 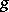 . A manifold is (locally) conformally flat if it is locally conformally equivalent to a flat manifold; the modifier "locally" is a tacit part of the definition, normally omitted. Clearly, conformally flat manifolds have no non-trivial conformal invariants.
. A manifold is (locally) conformally flat if it is locally conformally equivalent to a flat manifold; the modifier "locally" is a tacit part of the definition, normally omitted. Clearly, conformally flat manifolds have no non-trivial conformal invariants.
For any smooth manifold 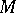 , let
, let 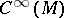 be the ring of smooth real-valued functions
be the ring of smooth real-valued functions 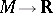 (regarded as an algebra over
(regarded as an algebra over 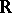 ), let
), let 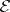 be the usual
be the usual 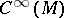 -module of
-module of 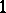 -forms over
-forms over 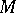 , and for any
, and for any 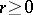 , let
, let 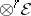 denote the
denote the  -fold tensor product
-fold tensor product 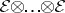 over
over 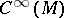 . In particular, the non-degenerate symmetric form
. In particular, the non-degenerate symmetric form 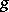 of a Riemannian manifold will be regarded as a symmetric element of
of a Riemannian manifold will be regarded as a symmetric element of 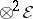 , as above. The conformal invariance condition
, as above. The conformal invariance condition 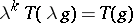 is entirely local, so that one may as well assume that
is entirely local, so that one may as well assume that  is itself an open set in
is itself an open set in 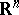 . One finds that the signature is of little interest in the construction of conformal invariants, since strategically placed
. One finds that the signature is of little interest in the construction of conformal invariants, since strategically placed 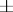 signs turn constructions for the strictly Riemannian case
signs turn constructions for the strictly Riemannian case 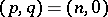 into corresponding constructions for the general case. Hence the existence of conformal invariants depends only on the dimension
into corresponding constructions for the general case. Hence the existence of conformal invariants depends only on the dimension  .
.
In the next few paragraphs the discussion of conformal invariants is organized by dimension  ; at the end the discussion centres exclusively on recent work concerning scalar conformal invariants for the cases
; at the end the discussion centres exclusively on recent work concerning scalar conformal invariants for the cases  .
.
Dimension one.
Any 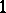 -dimensional Riemannian manifold
-dimensional Riemannian manifold 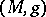 is trivially conformally flat, so that there are no non-trivial conformal invariants in dimension
is trivially conformally flat, so that there are no non-trivial conformal invariants in dimension  .
.
Dimension two.
If 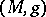 is a Riemannian manifold of dimension
is a Riemannian manifold of dimension 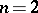 , let
, let
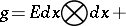 |
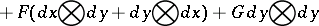 |
in some neighbourhood of any point 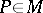 . The question of conformal flatness of
. The question of conformal flatness of 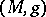 breaks into two cases, as follows.
breaks into two cases, as follows.
i) If 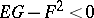 the usual method of factoring
the usual method of factoring 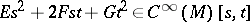 into a product of two linear homogeneous factors leads to a product
into a product of two linear homogeneous factors leads to a product 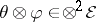 of linearly independent
of linearly independent 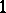 -forms, whose symmetric part is
-forms, whose symmetric part is 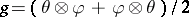 . Since
. Since 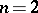 , there are smooth functions
, there are smooth functions 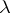 ,
, 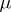 ,
, 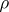 ,
, 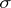 in a neighbourhood of
in a neighbourhood of 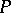 such that
such that 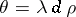 and
and 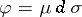 , so that
, so that 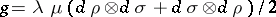 . By setting
. By setting 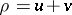 and
and 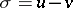 , one then has
, one then has  in a neighbourhood of
in a neighbourhood of 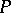 ; hence
; hence 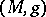 is conformally flat.
is conformally flat.
ii) The case 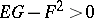 is the classical problem of finding isothermal coordinates for a Riemann surface, first solved by C.F. Gauss in a more restricted setting. More recent treatments of the same problem are given in [a9], [a10], [a4]; these results are easily adapted to the smooth case to show that any (smooth) Riemannian surface
is the classical problem of finding isothermal coordinates for a Riemann surface, first solved by C.F. Gauss in a more restricted setting. More recent treatments of the same problem are given in [a9], [a10], [a4]; these results are easily adapted to the smooth case to show that any (smooth) Riemannian surface 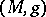 with a positive-definite (or negative-definite) metric
with a positive-definite (or negative-definite) metric 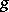 is conformally flat. It follows from i) and ii) that there are no non-trivial conformal invariants in dimension
is conformally flat. It follows from i) and ii) that there are no non-trivial conformal invariants in dimension 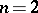 .
.
Dimension at least three.
Some classical conformal invariants in dimensions 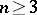 are as follows (their constructions will be sketched later):
are as follows (their constructions will be sketched later):
In 1899, E. Cotton [a7] assigned a tensor 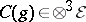 to any Riemannian manifold
to any Riemannian manifold  of any dimension
of any dimension  ; it is conformally invariant of weight
; it is conformally invariant of weight  only in the special case
only in the special case 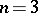 , and J.A. Schouten [a12] showed that in this case
, and J.A. Schouten [a12] showed that in this case 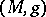 is conformally flat if and only if
is conformally flat if and only if 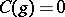 .
.
In 1918, H. Weyl [a14] constructed a tensor  for any Riemannian manifold
for any Riemannian manifold 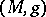 of dimension
of dimension 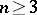 , conformally invariant of weight
, conformally invariant of weight 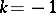 for all dimensions
for all dimensions 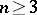 although it vanishes identically for
although it vanishes identically for 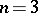 . Schouten [a12] showed that a Riemannian manifold
. Schouten [a12] showed that a Riemannian manifold 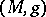 of dimension
of dimension  is conformally flat if and only if
is conformally flat if and only if 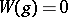 , and
, and 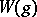 is now known as the Weyl curvature tensor (cf. also Weyl tensor).
is now known as the Weyl curvature tensor (cf. also Weyl tensor).
The remaining classical tensor 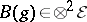 was constructed by R. Bach [a1] in 1921; although
was constructed by R. Bach [a1] in 1921; although 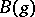 exists in any dimension
exists in any dimension 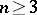 , it is conformally invariant, of weight
, it is conformally invariant, of weight 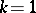 , only for Riemannian manifolds
, only for Riemannian manifolds 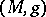 of dimension
of dimension 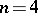 , and in this dimension
, and in this dimension 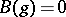 if and only if
if and only if 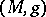 is conformally equivalent to an Einstein manifold (see below).
is conformally equivalent to an Einstein manifold (see below).
Algebraic background.
The primarily algebraic background needed to describe these three classical conformal invariants is also needed to sketch the more recent construction of the scalar conformal invariants, mentioned earlier. Let 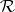 be any commutative ring with unit that is also an algebra over the real numbers; the ring
be any commutative ring with unit that is also an algebra over the real numbers; the ring  will later be
will later be 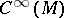 for a smooth manifold
for a smooth manifold 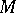 . Let
. Let 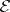 be an
be an 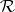 -module, let
-module, let 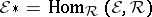 , let
, let 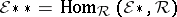 , and assume that the natural homomorphism from
, and assume that the natural homomorphism from 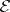 to its double dual
to its double dual 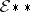 is an isomorphism
is an isomorphism  ; the
; the 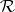 -module
-module 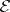 will later be the
will later be the 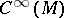 -module of
-module of 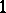 -forms on
-forms on 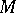 , and
, and 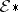 will be the
will be the 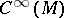 -module of smooth vector fields on
-module of smooth vector fields on  . As before, for any
. As before, for any 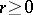 let
let 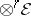 denote the
denote the  -fold tensor product over
-fold tensor product over 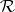 , later the
, later the 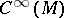 -module of contravariant tensors of degree
-module of contravariant tensors of degree  over
over  .
.
If 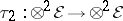 is the
is the 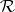 -module isomorphism that interchanges the two factors
-module isomorphism that interchanges the two factors 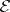 , an element
, an element  is symmetric if
is symmetric if  . Let
. Let 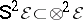 be the submodule of symmetric elements; it consists of
be the submodule of symmetric elements; it consists of 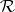 -linear combinations of products of the form
-linear combinations of products of the form 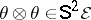 . One can regard any
. One can regard any 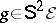 as a homomorphism
as a homomorphism 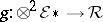 , so that there is an induced homomorphism
, so that there is an induced homomorphism 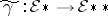 such that
such that  for any
for any  . The isomorphism
. The isomorphism 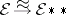 permits one to regard
permits one to regard  as a homomorphism
as a homomorphism  , and
, and  is non-degenerate if
is non-degenerate if 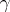 is an isomorphism. In this case the inverse
is an isomorphism. In this case the inverse 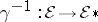 provides a unique element
provides a unique element 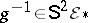 that can be regarded as a homomorphism
that can be regarded as a homomorphism 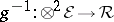 with values
with values 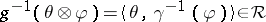 for any
for any 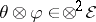 . One easily verifies that
. One easily verifies that 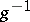 is itself non-degenerate.
is itself non-degenerate.
For any 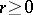 , let
, let  be an unordered pair of distinct elements in
be an unordered pair of distinct elements in 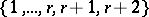 and let
and let 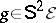 be non-degenerate. Then one can evaluate
be non-degenerate. Then one can evaluate 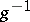 on the tensor product
on the tensor product 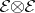 of the
of the 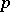 th and
th and  th factors of
th factors of 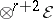 to obtain a well-defined
to obtain a well-defined 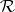 -linear contraction
-linear contraction 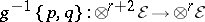 . The symmetry of
. The symmetry of 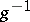 guarantees that
guarantees that  does not require an ordering of
does not require an ordering of 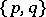 . Similarly, if
. Similarly, if 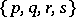 is any unordered subset of
is any unordered subset of 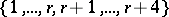 , there is a well-defined
, there is a well-defined 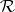 -linear contraction
-linear contraction 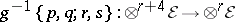 , where
, where 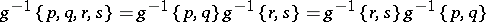 .
.
An element  is alternating if
is alternating if  , and there is a submodule
, and there is a submodule  that consists of all such alternating elements. If
that consists of all such alternating elements. If 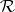 is the ring
is the ring  for a Riemannian manifold
for a Riemannian manifold 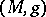 , and if
, and if 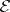 is the
is the 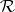 -module of
-module of 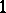 -forms on
-forms on 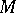 , then the classical Riemannian curvature tensor of
, then the classical Riemannian curvature tensor of 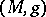 (cf. also Curvature tensor; Riemann tensor) is a symmetric element
(cf. also Curvature tensor; Riemann tensor) is a symmetric element 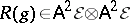 , for the submodule
, for the submodule 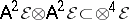 ; a construction is sketched below. The corresponding Ricci curvature is the contraction
; a construction is sketched below. The corresponding Ricci curvature is the contraction 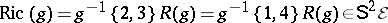 , and the corresponding scalar curvature is the contraction
, and the corresponding scalar curvature is the contraction 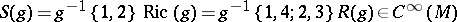 . In case
. In case 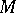 is of dimension
is of dimension 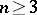 , there is a nameless tensor
, there is a nameless tensor
 |
that is used to construct all three classical conformal invariants.
The construction of the Weyl curvature tensor 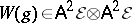 uses a
uses a 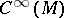 -module homomorphism from the submodule
-module homomorphism from the submodule 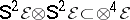 to the submodule of symmetric elements in
to the submodule of symmetric elements in 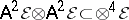 . If
. If 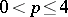 , let
, let 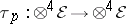 be the isomorphism that permutes the
be the isomorphism that permutes the 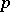 th factor
th factor 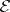 in
in 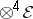 to the left of the first
to the left of the first  factors
factors 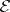 in
in 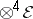 , so that
, so that 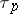 is cyclic in the usual sense that
is cyclic in the usual sense that 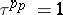 , and
, and 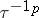 simply places the first factor into the
simply places the first factor into the 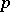 th slot; in particular,
th slot; in particular, 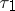 is the identity, and
is the identity, and 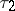 interchanges the first two factors as before. For any
interchanges the first two factors as before. For any 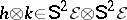 , set
, set
 |
By looking at the special cases 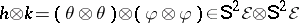 , for any
, for any  and
and 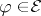 , one obtains
, one obtains
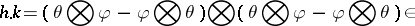 |
 |
these cases induce the announced homomorphism 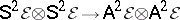 .
.
For any Riemannian manifold 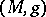 of dimension
of dimension 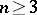 , the Weyl curvature tensor is the difference
, the Weyl curvature tensor is the difference 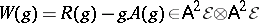 , which is a non-trivial conformal invariant of weight
, which is a non-trivial conformal invariant of weight 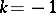 whenever
whenever 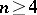 . Although the principal feature of
. Although the principal feature of 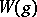 is that
is that 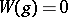 if and only if the Riemannian manifold
if and only if the Riemannian manifold 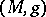 of dimension
of dimension 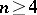 is conformally flat, it also provides a basic tool for constructing other conformal invariants for manifolds of dimensions
is conformally flat, it also provides a basic tool for constructing other conformal invariants for manifolds of dimensions 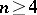 . For example, for any
. For example, for any  , let
, let 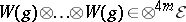 be the tensor product of
be the tensor product of 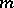 copies of
copies of 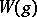 , and let
, and let  as unordered sets. Then the contraction
as unordered sets. Then the contraction
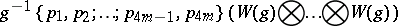 |
is a non-trivial scalar conformal invariant 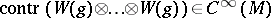 of weight
of weight 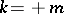 for any Riemannian manifold
for any Riemannian manifold 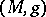 of dimension
of dimension  .
.
The curvatures 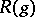 ,
, 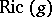 ,
, 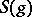 , and the tensor
, and the tensor 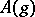 assigned to any Riemannian manifold
assigned to any Riemannian manifold 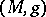 are all constructed via the Levi-Civita connection associated to
are all constructed via the Levi-Civita connection associated to  , defined below, so that
, defined below, so that 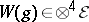 depends implicitly upon the Levi-Civita connection. The remaining classical conformal invariants
depends implicitly upon the Levi-Civita connection. The remaining classical conformal invariants 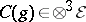 and
and 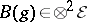 , for Riemannian manifolds of dimensions
, for Riemannian manifolds of dimensions 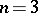 and
and 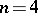 , respectively, as well as most of the scalar conformal invariants that will be introduced below, will be constructed explicitly via a version of the Levi-Civita connection that is sketched in the next two paragraphs; more details of this version appear in [a11].
, respectively, as well as most of the scalar conformal invariants that will be introduced below, will be constructed explicitly via a version of the Levi-Civita connection that is sketched in the next two paragraphs; more details of this version appear in [a11].
Levi-Civita connection.
For any smooth manifold 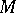 with
with 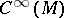 -module
-module 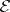 of
of 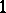 -forms as before, a connection (cf. also Connections on a manifold) is a sequence of real linear homomorphisms
-forms as before, a connection (cf. also Connections on a manifold) is a sequence of real linear homomorphisms  such that the complex
such that the complex 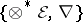 covers the classical de Rham complex
covers the classical de Rham complex 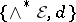 (cf. also Differential form); that is, the diagram
(cf. also Differential form); that is, the diagram
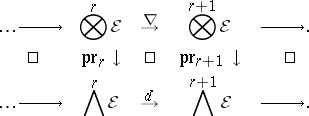 |
commutes for the usual projections  from tensor products to exterior products over
from tensor products to exterior products over 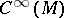 , where
, where 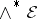 is the quotient of
is the quotient of 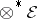 by the two-sided ideal generated by
by the two-sided ideal generated by 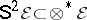 . Furthermore, if
. Furthermore, if 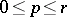 and if
and if 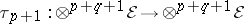 is the permutation with parity
is the permutation with parity  that moves the
that moves the  st factor
st factor  to the left of the first
to the left of the first 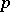 factors
factors 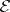 , then
, then
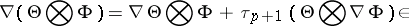 |
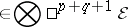 |
for any 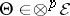 and
and 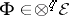 ; the product rule is
; the product rule is
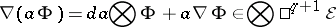 |
for 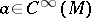 . It follows that the covering
. It follows that the covering  of
of 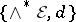 also preserves products. If
also preserves products. If 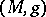 is a Riemannian manifold, with metric
is a Riemannian manifold, with metric 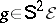 as usual, there is a unique connection
as usual, there is a unique connection 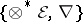 such that
such that 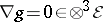 ; this is the Levi-Civita connection associated to
; this is the Levi-Civita connection associated to 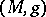 (cf. also Levi-Civita connection).
(cf. also Levi-Civita connection).
One useful property of any connection  for any smooth manifold
for any smooth manifold 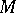 is that for any
is that for any  the composition
the composition
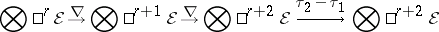 |
is 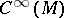 -linear, where
-linear, where 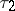 interchanges the first two factors
interchanges the first two factors 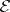 of
of 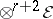 and
and 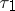 is the identity isomorphism; for any
is the identity isomorphism; for any  the homomorphism
the homomorphism  is the curvature operator
is the curvature operator  . In particular, for any Riemannian manifold
. In particular, for any Riemannian manifold  and corresponding Levi-Civita connection, the tensor product of
and corresponding Levi-Civita connection, the tensor product of 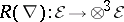 and the identity isomorphism
and the identity isomorphism  restricts to a
restricts to a  -linear mapping
-linear mapping 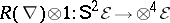 , and the image
, and the image 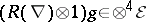 of the metric
of the metric 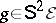 itself is the Riemannian curvature tensor
itself is the Riemannian curvature tensor 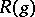 , lying in the submodule
, lying in the submodule 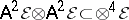 .
.
Even though the Levi-Civita connection 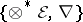 of a Riemannian manifold
of a Riemannian manifold 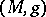 is defined in part by the requirement that
is defined in part by the requirement that 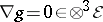 for the Riemannian metric
for the Riemannian metric  , observe that the definition
, observe that the definition 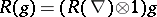 of the Riemannian curvature is obtained by applying the curvature operator
of the Riemannian curvature is obtained by applying the curvature operator 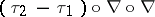 only to the first factor of
only to the first factor of 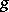 . Consequently,
. Consequently, 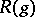 ,
, 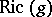 ,
, 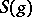 , and
, and 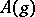 all require the first two derivatives of
all require the first two derivatives of 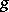 , in the obvious sense. The same remark applies to the Weyl curvature tensor
, in the obvious sense. The same remark applies to the Weyl curvature tensor 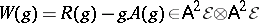 .
.
Cotton tensor.
Let 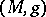 be any Riemannian manifold of dimension
be any Riemannian manifold of dimension 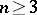 , with
, with 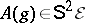 as before, let
as before, let
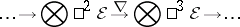 |
be the Levi-Civita connection, which restricts to 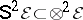 , and let
, and let 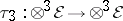 be the cyclic permutation of the factors
be the cyclic permutation of the factors 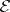 that moves the third factor
that moves the third factor 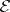 to the left of the first two factors
to the left of the first two factors 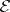 . The Cotton tensor is
. The Cotton tensor is
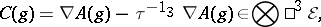 |
which visibly depends on third derivatives of 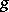 ; this is equivalent to the original definition of E. Cotton [a7], and it has the evident cyclic symmetry
; this is equivalent to the original definition of E. Cotton [a7], and it has the evident cyclic symmetry 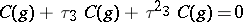 . Furthermore,
. Furthermore, 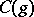 is a conformal invariant if
is a conformal invariant if 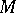 is of dimension
is of dimension 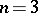 , and Schouten [a12] showed in this case that
, and Schouten [a12] showed in this case that 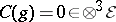 if and only if
if and only if 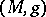 is conformally flat, as noted earlier.
is conformally flat, as noted earlier.
Closed oriented  -dimensional Riemannian manifolds.
-dimensional Riemannian manifolds.
If one considers closed oriented  -dimensional Riemannian manifolds
-dimensional Riemannian manifolds 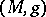 , the Chern–Simons invariant
, the Chern–Simons invariant  is shown in [a6] to depend only on the conformal equivalence class
is shown in [a6] to depend only on the conformal equivalence class 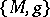 of
of 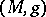 , and
, and  is a critical value if and only if
is a critical value if and only if 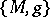 is conformally flat. S.S. Chern [a5] gave a simplified proof of this result by using the criterion
is conformally flat. S.S. Chern [a5] gave a simplified proof of this result by using the criterion 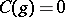 of the preceding paragraph.
of the preceding paragraph.
Bach tensor.
For any Riemannian manifold 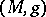 of dimension
of dimension 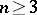 , the Bach tensor is
, the Bach tensor is
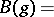 |
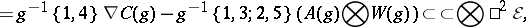 |
for the Levi-Civita connection
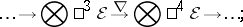 |
one easily verifies that the Bach tensor is an element of 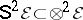 . It is conformally invariant only in the special case
. It is conformally invariant only in the special case 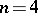 , and in that case one has
, and in that case one has 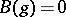 if and only if
if and only if 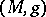 is conformally equivalent to a Riemannian manifold
is conformally equivalent to a Riemannian manifold 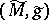 such that the Ricci curvature
such that the Ricci curvature  is a constant multiple of the metric
is a constant multiple of the metric 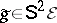 itself. Riemannian manifolds with the latter property are known as Einstein manifolds.
itself. Riemannian manifolds with the latter property are known as Einstein manifolds.
Recall that for any 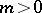 the contractions
the contractions
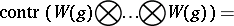 |
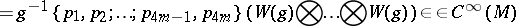 |
of the 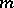 -fold tensor product of the Weyl curvature tensor
-fold tensor product of the Weyl curvature tensor 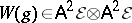 are scalar conformal invariants of weight
are scalar conformal invariants of weight 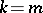 , and observe that any
, and observe that any  -linear combination of such contractions is also a scalar conformal invariant of weight
-linear combination of such contractions is also a scalar conformal invariant of weight 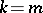 . Such scalar conformal invariants involve the Riemannian metric
. Such scalar conformal invariants involve the Riemannian metric 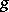 and its first and second order derivatives. However, the derivative
and its first and second order derivatives. However, the derivative 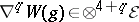 is not itself conformally invariant if
is not itself conformally invariant if 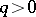 , so that in general one cannot expect contractions of products
, so that in general one cannot expect contractions of products 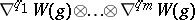 to produce conformal invariants if
to produce conformal invariants if 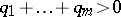 . The following observations suggest a reasonable modification of the construction.
. The following observations suggest a reasonable modification of the construction.
First, observe that if 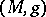 and
and 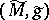 are Riemannian manifolds for which there is an embedding
are Riemannian manifolds for which there is an embedding 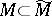 with
with  , then any scalar conformal invariant of
, then any scalar conformal invariant of 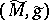 restricts to the corresponding scalar conformal invariant of
restricts to the corresponding scalar conformal invariant of  . Since the construction of conformal invariants is an entirely local question, it suffices to consider embeddings of open sets
. Since the construction of conformal invariants is an entirely local question, it suffices to consider embeddings of open sets 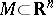 into open sets
into open sets 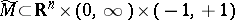 , for example. The hypotheses can be weakened if the conformal equivalence class of
, for example. The hypotheses can be weakened if the conformal equivalence class of 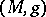 has a real-analytic representative with coordinates
has a real-analytic representative with coordinates 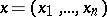 . One can then assign a coordinate
. One can then assign a coordinate  and use power series about
and use power series about 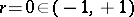 to describe the Riemannian metric
to describe the Riemannian metric 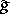 of an embedding, knowing that only the restrictions of the derivatives
of an embedding, knowing that only the restrictions of the derivatives 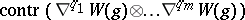 to the submanifold
to the submanifold 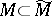 are of any interest, the inclusion being
are of any interest, the inclusion being
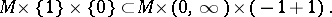 |
The second observation is a classical result, not directly related to conformal invariants. Given any Riemannian manifold 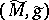 , with Levi-Civita connection
, with Levi-Civita connection  and Riemannian curvature
and Riemannian curvature 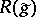 , if
, if 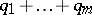 is an even number, then the contractions
is an even number, then the contractions 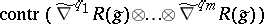 involve derivatives of
involve derivatives of  of order up to
of order up to 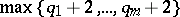 ; furthermore, such contractions are visibly coordinate-free. Results in [a15] imply that if
; furthermore, such contractions are visibly coordinate-free. Results in [a15] imply that if  is locally real-analytic, then any coordinate-free polynomial combination of
is locally real-analytic, then any coordinate-free polynomial combination of 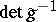 and the components of the derivatives
and the components of the derivatives 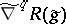 is a
is a 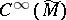 -linear combination of such contractions, which are known as Weyl invariants.
-linear combination of such contractions, which are known as Weyl invariants.
The third observation is that if 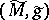 is a Ricci-flat Riemannian manifold, in the sense that
is a Ricci-flat Riemannian manifold, in the sense that 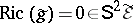 , then
, then 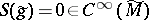 so that
so that 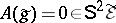 ; in this case the Riemannian curvature tensor itself is a classical conformal invariant:
; in this case the Riemannian curvature tensor itself is a classical conformal invariant: 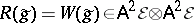 . Even though one cannot expect the derivatives
. Even though one cannot expect the derivatives 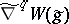 nor contractions of products of such derivatives to be conformal invariants, the identifications
nor contractions of products of such derivatives to be conformal invariants, the identifications 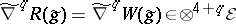 suggest that the contractions
suggest that the contractions 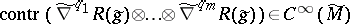 may be of value in the Ricci-flat case, whenever
may be of value in the Ricci-flat case, whenever 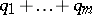 is an even number.
is an even number.
General construction of scalar conformal invariants.
The preceding observations lead to a general construction of scalar conformal invariants of 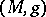 , with a dimensional restriction that will be specified later. One first covers
, with a dimensional restriction that will be specified later. One first covers  by sufficiently small coordinate neighbourhoods
by sufficiently small coordinate neighbourhoods 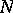 and writes
and writes 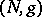 for each resulting Riemannian manifold
for each resulting Riemannian manifold 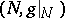 . For each
. For each  C. Fefferman and C.R. Graham [a8] use a technique that appeared independently in [a13] to introduce a codimension-
C. Fefferman and C.R. Graham [a8] use a technique that appeared independently in [a13] to introduce a codimension- embedding
embedding 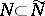 , described later, and to devise a Cauchy problem whose solution provides a Ricci-flat manifold
, described later, and to devise a Cauchy problem whose solution provides a Ricci-flat manifold 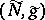 with
with 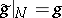 . A further feature of the construction guarantees that any Weyl invariant in
. A further feature of the construction guarantees that any Weyl invariant in 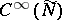 restricts to a conformal invariant of
restricts to a conformal invariant of 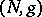 , of weight
, of weight 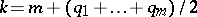 . Since
. Since 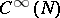 -linear combinations of scalar conformal invariants of weight
-linear combinations of scalar conformal invariants of weight  are also scalar conformal invariants of weight
are also scalar conformal invariants of weight 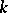 , for any fixed
, for any fixed 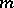 -tuple
-tuple 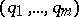 of non-negative integers with an even sum one can use a smooth partition of unity subordinate to the covering of
of non-negative integers with an even sum one can use a smooth partition of unity subordinate to the covering of  by the coordinate neighbourhoods
by the coordinate neighbourhoods 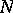 to obtain a scalar conformal invariant of
to obtain a scalar conformal invariant of 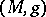 itself, known as a Weyl conformal invariant.
itself, known as a Weyl conformal invariant.
T.N. Bailey, M.G. Eastwood and Graham [a2] completed the proof of the following Fefferman–Graham conjecture [a8], which depends upon the parity of  : If
: If  is a Riemannian manifold of odd dimension
is a Riemannian manifold of odd dimension  , then every scalar conformal invariant of
, then every scalar conformal invariant of 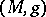 is a Weyl conformal invariant. If
is a Weyl conformal invariant. If 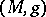 is a Riemannian manifold of even dimension
is a Riemannian manifold of even dimension  , then the preceding statement is true only for scalar conformal invariants of weight
, then the preceding statement is true only for scalar conformal invariants of weight 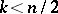 , and there is a conformally invariant element in
, and there is a conformally invariant element in  of weight
of weight 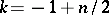 that serves as an obstruction to finding a formal power series solution of the Cauchy problems used to construct the ambient manifolds
that serves as an obstruction to finding a formal power series solution of the Cauchy problems used to construct the ambient manifolds 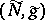 ; the obstruction vanishes if
; the obstruction vanishes if 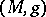 is conformally equivalent to an Einstein manifold; if
is conformally equivalent to an Einstein manifold; if 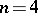 the obstruction is the Bach conformal invariant
the obstruction is the Bach conformal invariant 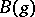 .
.
There are some exceptional scalar conformal invariants for even dimensions  and weight
and weight  , first observed in [a2]; the catalogue of all such exceptional invariants was completed in [a3].
, first observed in [a2]; the catalogue of all such exceptional invariants was completed in [a3].
Fefferman–Graham method.
This method, introduced in [a8], allows one to construct the codimension- embeddings
embeddings 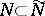 of the Riemannian manifolds
of the Riemannian manifolds 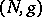 , and to formulate the Cauchy problems whose solutions turn each ambient space
, and to formulate the Cauchy problems whose solutions turn each ambient space 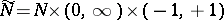 into a Ricci-flat manifold
into a Ricci-flat manifold  with the desired properties.
with the desired properties.
One starts with the fibration over 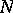 in which the fibre over each
in which the fibre over each 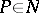 consists of positive multiples
consists of positive multiples 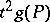 of the metric
of the metric 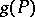 at
at 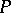 ; one may as well suppose that
; one may as well suppose that  . The multiplicative group
. The multiplicative group 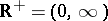 of real numbers
of real numbers 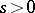 acts on the fibres by mapping
acts on the fibres by mapping 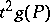 into
into 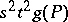 , and this permits one to regard the fibration as a fibre bundle with structure group
, and this permits one to regard the fibration as a fibre bundle with structure group 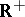 (cf. also Principal fibre bundle). Clearly, any section of the fibre bundle can be regarded as a Riemannian manifold that is conformally equivalent to
(cf. also Principal fibre bundle). Clearly, any section of the fibre bundle can be regarded as a Riemannian manifold that is conformally equivalent to  .
.
Let 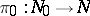 be the corresponding principal fibre bundle, and observe that since
be the corresponding principal fibre bundle, and observe that since 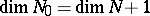 , the pullback
, the pullback 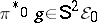 of the metric
of the metric 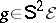 over
over  needs at least one additional term to serve as a Riemannian metric over
needs at least one additional term to serve as a Riemannian metric over 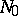 . It is useful to replace
. It is useful to replace 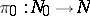 by another
by another 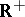 -bundle
-bundle 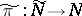 with
with 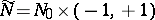 , and to try to construct a (non-degenerate) metric
, and to try to construct a (non-degenerate) metric 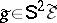 on
on 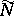 such that
such that
1) the restriction 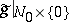 is
is 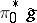 ;
;
2) the group elements 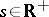 map
map 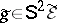 into
into 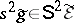 over all of
over all of  ;
;
3) 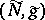 is Ricci-flat, with the consequence
is Ricci-flat, with the consequence 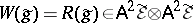 noted earlier. There is an implicit additional assumption, that the conformal equivalence class containing
noted earlier. There is an implicit additional assumption, that the conformal equivalence class containing 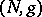 is real-analytic in the sense that there is a representative
is real-analytic in the sense that there is a representative 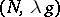 of the conformal class of
of the conformal class of 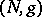 for which one can choose coordinates
for which one can choose coordinates 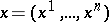 in
in 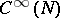 such that
such that 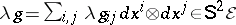 , for coefficients
, for coefficients 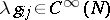 that are real-analytic functions of
that are real-analytic functions of  ; one may as well assume that
; one may as well assume that 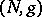 itself has this property.
itself has this property.
The Fefferman–Graham method [a8] leads to a metric of the form
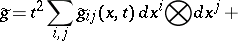 |
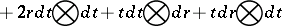 |
that satisfies 1)–3) for all 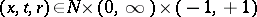 (
(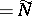 ), for real-analytic functions
), for real-analytic functions 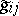 of
of 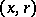 that satisfy the initial condition 1),
that satisfy the initial condition 1), 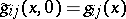 as formal power series about
as formal power series about 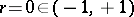 ; convergence is obtained in some neighbourhood of
; convergence is obtained in some neighbourhood of 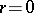 . Observe that the metric
. Observe that the metric 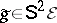 trivially satisfies the homogeneity condition 2) over all of
trivially satisfies the homogeneity condition 2) over all of 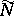 . The Riemannian curvature
. The Riemannian curvature 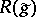 is itself conformally invariant by the consequence
is itself conformally invariant by the consequence 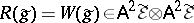 of condition 3), and the homogeneity condition implies that any Weyl invariant
of condition 3), and the homogeneity condition implies that any Weyl invariant 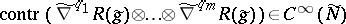 restricts over the section
restricts over the section 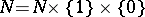 of
of  to a Weyl conformal invariant in
to a Weyl conformal invariant in 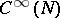 , as required.
, as required.
References
| [a1] | R. Bach, "Zur Weylschen Relativitätstheorie und der Weylschen Erweiterung des Krümmungstensorbegriffs" Math. Z. , 9 (1921) pp. 110–135 (Also: Jahrbuch 48, 1035) MR1544454 Zbl 48.1035.01 |
| [a2] | T.N. Bailey, M.G. Eastwood, C.R. Graham, "Invariant theory for conformal and CR geometry" Ann. of Math. , 139 : 2 (1994) pp. 491–552 MR1283869 Zbl 0814.53017 |
| [a3] | T.N. Bailey, A.R. Gover, "Exceptional invariants in the parabolic invariant theory of conformal geometry" Proc. Amer. Math. Soc. , 123 (1995) pp. 2535–2543 MR1243161 Zbl 0844.53008 |
| [a4] | S.S. Chern, "An elementary proof of the existence of isothermal parameters on a surface" Proc. Amer. Math. Soc. , 6 (1955) pp. 771–782 MR0074856 Zbl 0066.15402 |
| [a5] | S.S. Chern, "On a conformal invariant of three-dimensional manifolds" , Aspects of Math. and its Appl. , North-Holland (1986) pp. 245–252 MR0849555 Zbl 0589.53011 |
| [a6] | S.S. Chern, J. Simons, "Characteristic forms and geometric invariants" Ann. of Math. , 99 (1974) pp. 48–69 MR0353327 Zbl 0283.53036 Zbl 0591.53050 |
| [a7] | E. Cotton, "Sur les variétes à trois dimensions" Ann. Fac. Sci. Toulouse , 1 (1899) pp. 385–438 (Also: Jahrbuch 30, 538-539) MR1508211 Zbl 30.0538.01 |
| [a8] | C. Fefferman, C.R. Graham, "Conformal invariants" , The Mathematical Heritage of Élie Cartan (Lyon, 1984) , Astérisque (1985) pp. 95–116 MR0837196 Zbl 0602.53007 |
| [a9] | A. Korn, "Zwei Anwendungen der Methode der sukzessiven Anwendungen" Schwarz Festschrift (1914) pp. 215–229 (Also: Jahrbuch 45, 568) Zbl 45.0568.01 |
| [a10] | L. Lichtenstein, "Zur Theorie der konformen Abbildungen nichtanalytischer, singularitätenfreier Flächenstücke auf ebene Gebiete" Bull. Internat. Acad. Sci. Gracovie, Cl. Sci. Math. Nat. Ser. A. (1916) pp. 192–217 (Also: Jahrbuch 46, 547) |
| [a11] | H. Osborn, "Affine connection complexes" Acta Applic. Math. (to appear) MR1741659 Zbl 0956.53014 |
| [a12] | J.A. Schouten, "Über die konforme Abbildung  -dimensionaler Mannigfaltigkeiten mit quadratischer Maß bestimmung auf eine Mannigfaltigkeit mit euklidischer Maß bestimmung" Math. Z. , 11 (1921) pp. 58–88 (Also: Jahrbuch 48, 857-858) -dimensionaler Mannigfaltigkeiten mit quadratischer Maß bestimmung auf eine Mannigfaltigkeit mit euklidischer Maß bestimmung" Math. Z. , 11 (1921) pp. 58–88 (Also: Jahrbuch 48, 857-858) |
| [a13] | J.A. Schouten, J. Haantjes, "Beitgräge zur allgemeinen (gekrümmten) konformen Differentialgeometrie I–II" Math. Ann. , 112/113 (1936) pp. 594–629; 568–583 |
| [a14] | H. Weyl, "Reine Infinitesimalgeometrie" Math. Z. , 2 (1918) pp. 384–411 (Also: Jahrbuch 46, 1301) MR1544327 Zbl 46.1301.01 |
| [a15] | H. Weyl, "The classical groups" , Princeton Univ. Press (1939) (Reprint: 1946) MR0000255 Zbl 0020.20601 Zbl 65.0058.02 |
Conformal invariants. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Conformal_invariants&oldid=13909