Difference between revisions of "Tangle move"
(Importing text file) |
(→References: zbl link) |
||
| (One intermediate revision by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | For given | + | For given $n$-tangles $T_1$ and $T_2$ (cf. also [[Tangle]]), the tangle move, or more specifically the $(T_1,T_2)$-move, is substitution of the tangle $T_2$ in the place of the tangle $T_1$ in a link (or tangle). The simplest tangle $2$-move is a crossing change. This can be generalized to $n$-moves (cf. [[Montesinos–Nakanishi conjecture]] or [[#References|[a5]]]), $(m,q)$-moves (cf. Fig.a1), and $(p/q)$-rational moves, where a rational $(p/q)$-tangle is substituted in place of the identity tangle [[#References|[a6]]] (Fig.a2 illustrates a $(13/5)$-rational move). |
| − | A | + | A $(p/q)$-rational move preserves the space of Fox $p$-colourings of a link or tangle (cf. [[Fox-n-colouring|Fox $n$-colouring]]). For a fixed prime number $p$, there is a conjecture that any link can be reduced to a trivial link by $(p/q)$-rational moves ($|q| \le p/2$). |
| − | Kirby moves (cf. [[ | + | Kirby moves (cf. [[Kirby calculus]]) can be interpreted as tangle moves on framed links. |
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t130020a.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t130020a.gif" /> | ||
| Line 13: | Line 13: | ||
Figure: t130020b | Figure: t130020b | ||
| − | Habiro | + | Habiro $C_n$-moves [[#References|[a2]]] are prominent in the theory of Vassiliev–Gusarov invariants of links and $3$-manifolds. The simplest and most extensively studied Habiro move (beyond the crossing change) is the $\Delta$-move on a $3$-tangle (cf. Fig.a3). One can reduce every knot into the trivial knot by $\Delta$-moves [[#References|[a4]]]. |
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t130020c.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/t130020c.gif" /> | ||
| Line 20: | Line 20: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> T. Harikae, Y. Uchida, "Irregular dihedral branched coverings of knots" M. Bozhüyük (ed.) , ''Topics in Knot Theory'' , ''NATO ASI Ser. C'' , '''399''' , Kluwer Acad. Publ. (1993) pp. 269–276</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> K. Habiro, "Claspers and finite type invariants of links" ''Geometry and Topology'' , '''4''' (2000) pp. 1–83</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Kirby, "Problems in low-dimensional topology" W. Kazez (ed.) , ''Geometric Topology (Proc. Georgia Internat. Topology Conf., 1993)'' , ''Studies in Adv. Math.'' , '''2''' , Amer. Math. Soc. /IP (1997) pp. 35–473</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H. Murakami, Y. Nakanishi, "On a certain move generating link homology" ''Math. Ann.'' , '''284''' (1989) pp. 75–89</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> J.H. Przytycki, " | + | <table> |
| + | <TR><TD valign="top">[a1]</TD> <TD valign="top"> T. Harikae, Y. Uchida, "Irregular dihedral branched coverings of knots" M. Bozhüyük (ed.) , ''Topics in Knot Theory'' , ''NATO ASI Ser. C'' , '''399''' , Kluwer Acad. Publ. (1993) pp. 269–276</TD></TR> | ||
| + | <TR><TD valign="top">[a2]</TD> <TD valign="top"> K. Habiro, "Claspers and finite type invariants of links" ''Geometry and Topology'' , '''4''' (2000) pp. 1–83 {{ZBL|0941.57015}}</TD></TR> | ||
| + | <TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Kirby, "Problems in low-dimensional topology" W. Kazez (ed.) , ''Geometric Topology (Proc. Georgia Internat. Topology Conf., 1993)'' , ''Studies in Adv. Math.'' , '''2''' , Amer. Math. Soc. /IP (1997) pp. 35–473</TD></TR> | ||
| + | <TR><TD valign="top">[a4]</TD> <TD valign="top"> H. Murakami, Y. Nakanishi, "On a certain move generating link homology" ''Math. Ann.'' , '''284''' (1989) pp. 75–89</TD></TR> | ||
| + | <TR><TD valign="top">[a5]</TD> <TD valign="top"> J.H. Przytycki, "$3$-coloring and other elementary invariants of knots" , ''Knot Theory'' , '''42''' , Banach Center Publ. (1998) pp. 275–295</TD></TR> | ||
| + | <TR><TD valign="top">[a6]</TD> <TD valign="top"> Y. Uchida, S. Suzuki (ed.) , ''Knots '96, Proc. Fifth Internat. Research Inst. of MSJ'' , World Sci. (1997) pp. 109–113</TD></TR> | ||
| + | </table> | ||
| + | |||
| + | {{TEX|done}} | ||
Latest revision as of 18:11, 1 June 2023
For given $n$-tangles $T_1$ and $T_2$ (cf. also Tangle), the tangle move, or more specifically the $(T_1,T_2)$-move, is substitution of the tangle $T_2$ in the place of the tangle $T_1$ in a link (or tangle). The simplest tangle $2$-move is a crossing change. This can be generalized to $n$-moves (cf. Montesinos–Nakanishi conjecture or [a5]), $(m,q)$-moves (cf. Fig.a1), and $(p/q)$-rational moves, where a rational $(p/q)$-tangle is substituted in place of the identity tangle [a6] (Fig.a2 illustrates a $(13/5)$-rational move).
A $(p/q)$-rational move preserves the space of Fox $p$-colourings of a link or tangle (cf. Fox $n$-colouring). For a fixed prime number $p$, there is a conjecture that any link can be reduced to a trivial link by $(p/q)$-rational moves ($|q| \le p/2$).
Kirby moves (cf. Kirby calculus) can be interpreted as tangle moves on framed links.
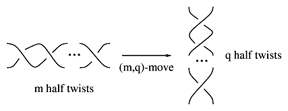
Figure: t130020a
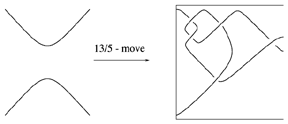
Figure: t130020b
Habiro $C_n$-moves [a2] are prominent in the theory of Vassiliev–Gusarov invariants of links and $3$-manifolds. The simplest and most extensively studied Habiro move (beyond the crossing change) is the $\Delta$-move on a $3$-tangle (cf. Fig.a3). One can reduce every knot into the trivial knot by $\Delta$-moves [a4].
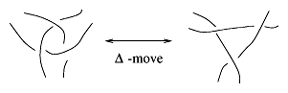
Figure: t130020c
References
| [a1] | T. Harikae, Y. Uchida, "Irregular dihedral branched coverings of knots" M. Bozhüyük (ed.) , Topics in Knot Theory , NATO ASI Ser. C , 399 , Kluwer Acad. Publ. (1993) pp. 269–276 |
| [a2] | K. Habiro, "Claspers and finite type invariants of links" Geometry and Topology , 4 (2000) pp. 1–83 Zbl 0941.57015 |
| [a3] | R. Kirby, "Problems in low-dimensional topology" W. Kazez (ed.) , Geometric Topology (Proc. Georgia Internat. Topology Conf., 1993) , Studies in Adv. Math. , 2 , Amer. Math. Soc. /IP (1997) pp. 35–473 |
| [a4] | H. Murakami, Y. Nakanishi, "On a certain move generating link homology" Math. Ann. , 284 (1989) pp. 75–89 |
| [a5] | J.H. Przytycki, "$3$-coloring and other elementary invariants of knots" , Knot Theory , 42 , Banach Center Publ. (1998) pp. 275–295 |
| [a6] | Y. Uchida, S. Suzuki (ed.) , Knots '96, Proc. Fifth Internat. Research Inst. of MSJ , World Sci. (1997) pp. 109–113 |
Tangle move. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tangle_move&oldid=18153