Difference between revisions of "Non-oscillation interval"
From Encyclopedia of Mathematics
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | n0672201.png | ||
| + | $#A+1 = 8 n = 1 | ||
| + | $#C+1 = 8 : ~/encyclopedia/old_files/data/N067/N.0607220 Non\AAhoscillation interval, | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
''interval of disconjugacy'' | ''interval of disconjugacy'' | ||
| − | A connected interval | + | A connected interval $ J $ |
| + | on the real axis $ \mathbf R $ | ||
| + | such that any non-trivial solution $ x = x ( t) $ | ||
| + | of a given ordinary linear differential equation of order $ n $ | ||
| + | with real coefficients, | ||
| − | + | $$ \tag{* } | |
| + | x ^ {(} n) + a _ {1} ( t) x ^ {(} n- 1) + \dots + a _ {n} ( t) x | ||
| + | = 0 , | ||
| + | $$ | ||
| − | has on it more than | + | has on it more than $ n- 1 $ |
| + | zeros, an $ m $- | ||
| + | fold zero counted $ m $ | ||
| + | times. Properties of solutions of (*) on a non-oscillation interval have been well studied (see, for example, [[#References|[1]]]–[[#References|[3]]]). There are several generalizations of the concept of a non-oscillation interval, to linear systems of differential equations, to non-linear differential equations, and also to other types of equations (difference, with deviating argument). | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> P. Hartman, "Ordinary differential equations" , Birkhäuser (1982)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A.Yu. Levin, "Non-oscillation of solutions of the equation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/n/n067/n067220/n0672209.png" />" ''Russian Math. Surveys'' , '''24''' : 2 (1969) pp. 43–99 ''Uspekhi Mat. Nauk'' , '''24''' : 2 (1969) pp. 43–96</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> W.A. Coppel, "Disconjugacy" , Springer (1971)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> P. Hartman, "Ordinary differential equations" , Birkhäuser (1982)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A.Yu. Levin, "Non-oscillation of solutions of the equation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/n/n067/n067220/n0672209.png" />" ''Russian Math. Surveys'' , '''24''' : 2 (1969) pp. 43–99 ''Uspekhi Mat. Nauk'' , '''24''' : 2 (1969) pp. 43–96</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> W.A. Coppel, "Disconjugacy" , Springer (1971)</TD></TR></table> | ||
Latest revision as of 08:03, 6 June 2020
interval of disconjugacy
A connected interval $ J $ on the real axis $ \mathbf R $ such that any non-trivial solution $ x = x ( t) $ of a given ordinary linear differential equation of order $ n $ with real coefficients,
$$ \tag{* } x ^ {(} n) + a _ {1} ( t) x ^ {(} n- 1) + \dots + a _ {n} ( t) x = 0 , $$
has on it more than $ n- 1 $ zeros, an $ m $- fold zero counted $ m $ times. Properties of solutions of (*) on a non-oscillation interval have been well studied (see, for example, [1]–[3]). There are several generalizations of the concept of a non-oscillation interval, to linear systems of differential equations, to non-linear differential equations, and also to other types of equations (difference, with deviating argument).
References
| [1] | P. Hartman, "Ordinary differential equations" , Birkhäuser (1982) |
| [2] | A.Yu. Levin, "Non-oscillation of solutions of the equation 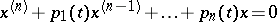 " Russian Math. Surveys , 24 : 2 (1969) pp. 43–99 Uspekhi Mat. Nauk , 24 : 2 (1969) pp. 43–96 |
| [3] | W.A. Coppel, "Disconjugacy" , Springer (1971) |
How to Cite This Entry:
Non-oscillation interval. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Non-oscillation_interval&oldid=17615
Non-oscillation interval. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Non-oscillation_interval&oldid=17615
This article was adapted from an original article by Yu.V. Komlenko (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article