Difference between revisions of "Integral representation of an analytic function"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| (One intermediate revision by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | i0516201.png | ||
| + | $#A+1 = 145 n = 0 | ||
| + | $#C+1 = 145 : ~/encyclopedia/old_files/data/I051/I.0501620 Integral representation of an analytic function | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
The representation of an analytic function by an integral depending on a parameter. Integral representations of analytic functions arose in the early stages of development of function theory and mathematical analysis in general as a suitable apparatus for the explicit representation of analytic solutions of differential equations, for the investigation of the asymptotics of these solutions and for their analytic continuation. Somewhat later integral representations of analytic functions found application in the solution of [[Boundary value problems of analytic function theory|boundary value problems of analytic function theory]] and singular integral equations (cf. [[Singular integral equation|Singular integral equation]]), in the study of interior and [[Boundary properties of analytic functions|boundary properties of analytic functions]] of various classes, as well as in the solution of other problems of mathematical analysis. In the process of development of function theory, the study of properties of the most important individual integral representations of analytic functions established an independent chapter in function theory (see, for example, [[Cauchy integral|Cauchy integral]]; [[Poisson integral|Poisson integral]]; [[Schwarz integral|Schwarz integral]]). | The representation of an analytic function by an integral depending on a parameter. Integral representations of analytic functions arose in the early stages of development of function theory and mathematical analysis in general as a suitable apparatus for the explicit representation of analytic solutions of differential equations, for the investigation of the asymptotics of these solutions and for their analytic continuation. Somewhat later integral representations of analytic functions found application in the solution of [[Boundary value problems of analytic function theory|boundary value problems of analytic function theory]] and singular integral equations (cf. [[Singular integral equation|Singular integral equation]]), in the study of interior and [[Boundary properties of analytic functions|boundary properties of analytic functions]] of various classes, as well as in the solution of other problems of mathematical analysis. In the process of development of function theory, the study of properties of the most important individual integral representations of analytic functions established an independent chapter in function theory (see, for example, [[Cauchy integral|Cauchy integral]]; [[Poisson integral|Poisson integral]]; [[Schwarz integral|Schwarz integral]]). | ||
A wide class of integral representations of analytic functions, used for obtaining and studying analytic solutions of differential equations, can be described by the general formula | A wide class of integral representations of analytic functions, used for obtaining and studying analytic solutions of differential equations, can be described by the general formula | ||
| − | + | $$ \tag{1 } | |
| + | f ( z) = \int\limits _ { L } | ||
| + | K ( z , \zeta ) v ( \zeta ) \ | ||
| + | d \zeta , | ||
| + | $$ | ||
| − | where | + | where $ K ( z , \zeta ) $ |
| + | is the kernel of the integral representation, $ v ( \zeta ) $ | ||
| + | is its density and $ L $ | ||
| + | is a contour (or system of contours) in the complex plane in which both variables $ z $ | ||
| + | and $ \zeta $ | ||
| + | vary. An appropriate and, as far as possible, simplest solution of the three interrelated questions on the choice of the kernel $ K $, | ||
| + | the density $ v $ | ||
| + | and the contour $ L $ | ||
| + | for the representation of a given function $ f ( z) $( | ||
| + | or given class of functions) is the determining factor from the point of view of the successful application of the method of integral representations of analytic functions. In turn, the properties of the representation (1) depend essentially on whether the kernel $ K ( z , \zeta ) $ | ||
| + | is an entire function of the complex variables $ z , \zeta $, | ||
| + | or whether it is singular, that is, has some singularities. In general, the kernel of an integral representation of an analytic function does not have to be an analytic function of the variables $ z , \zeta $; | ||
| + | the analyticity of $ f ( z) $ | ||
| + | may be ensured by specific properties of the density. Nor is it necessary, in general, that formula (1) be a formula with a single integration; there are types of integral representations of analytic functions in which iterated integrals are used. | ||
| − | The general scheme for obtaining integral representations of special functions | + | The general scheme for obtaining integral representations of special functions $ f ( z) $ |
| + | that are solutions of certain ordinary differential equations $ \mathfrak L _ {z} [ f ] ( z) = 0 $ | ||
| + | reduces in the main to the following. By an appropriate choice of the kernel $ K $, | ||
| + | most often non-singular, the following formula for the action of the operator $ \mathfrak L _ {z} $ | ||
| + | should hold: | ||
| − | + | $$ \tag{2 } | |
| + | \mathfrak L _ {z} [ f ] ( z) = \ | ||
| + | \int\limits _ { L } | ||
| + | \mathfrak L _ {z} [ K ] ( z , \zeta ) v ( \zeta ) d \zeta = | ||
| + | $$ | ||
| − | + | $$ | |
| + | = \ | ||
| + | \int\limits _ { L } \mathfrak M _ \zeta [ K ] ( z , \zeta ) v ( \zeta ) d \zeta = | ||
| + | $$ | ||
| − | + | $$ | |
| + | = \ | ||
| + | \int\limits _ { L } K ( z , \zeta ) \widetilde{\mathfrak L} _ \zeta [ v | ||
| + | ] ( \zeta ) d \zeta + P [ v , K ] , | ||
| + | $$ | ||
| − | that is, the kernel must, in turn, as far as possible satisfy a simple partial differential equation | + | that is, the kernel must, in turn, as far as possible satisfy a simple partial differential equation $ \mathfrak L _ {z} [ K ] = \mathfrak M _ \zeta [ K ] $, |
| + | thus allowing successive integration by parts with the aim of recovering the original form of the kernel and a transfer to the action of the adjoint operator $ \widetilde{\mathfrak L} _ \zeta $ | ||
| + | thus obtained on the density $ v $. | ||
| + | Having obtained a formula of the type (2), one selects a fairly simple density $ v $ | ||
| + | satisfying the adjoint equation $ \widetilde{\mathfrak L} _ \zeta [ v ] ( \zeta ) = 0 $, | ||
| + | and a contour $ L $ | ||
| + | guaranteeing that the term $ P [ v , K ] $ | ||
| + | vanishes. Here one must bear in mind that the choice of the contour $ L $ | ||
| + | determines a particular solution of the original equation $ \mathfrak L _ {z} [ f ] ( z) = 0 $. | ||
| + | The following kernels are used most often: | ||
| − | + | $$ | |
| + | K ( z , \zeta ) = e ^ {z \zeta } \ \ | ||
| + | \textrm{ or } \ \ | ||
| + | K ( z , \zeta ) = e ^ {i z \zeta } , | ||
| + | $$ | ||
| − | + | $$ | |
| + | K ( z , \zeta ) = z ^ \zeta \ \textrm{ and } \ \ | ||
| + | K ( z , \zeta ) = ( z - \zeta ) ^ \alpha , | ||
| + | $$ | ||
sometimes called the Laplace–Fourier kernel, the Mellin kernel and the Euler kernel, respectively. Various changes of variables lead to modified forms of the kernels. In the form written above, integral representations of analytic functions are closely related with the method of integral transforms (cf. [[Integral transform|Integral transform]]). | sometimes called the Laplace–Fourier kernel, the Mellin kernel and the Euler kernel, respectively. Various changes of variables lead to modified forms of the kernels. In the form written above, integral representations of analytic functions are closely related with the method of integral transforms (cf. [[Integral transform|Integral transform]]). | ||
| Line 25: | Line 85: | ||
In this way, for example, a well-known integral representation of the Bessel functions is obtained: | In this way, for example, a well-known integral representation of the Bessel functions is obtained: | ||
| − | + | $$ \tag{3 } | |
| + | J _ {p} ( z) = \ | ||
| + | |||
| + | \frac{\Gamma ( 1 / 2 - p ) }{2 i \pi ^ {3/2} } | ||
| + | |||
| + | \left ( | ||
| + | \frac{z}{2} | ||
| + | \right ) ^ {p} | ||
| + | \int\limits _ { L } | ||
| + | e ^ {i z \zeta } | ||
| + | ( \zeta ^ {2} - 1 ) ^ {p-} 1/2 d \zeta , | ||
| + | $$ | ||
| + | |||
| + | where the contour $ L $ | ||
| + | takes the form of a figure eight containing the points $ - 1 $ | ||
| + | and $ + 1 $. | ||
| + | The representation (3) is distinctive in that, on the one hand, its density $ v ( \zeta ) = ( \zeta ^ {2} - 1 ) ^ {p-} 1/2 $ | ||
| + | is considerably simpler than the transcendental functions $ J _ {p} ( z) $ | ||
| + | being represented, and on the other hand it enables one to survey fairly simply the properties of the functions $ J _ {p} ( z) $, | ||
| + | in particular, to study their asymptotics. | ||
| + | |||
| + | An appropriate modification of the contour $ L $ | ||
| + | enables one to obtain analytic continuations, in other words, to obtain integral representations that can be used throughout the entire domain of existence. For example, the Euler integral of the second kind, | ||
| − | + | $$ | |
| + | \Gamma ( z) = \ | ||
| + | \int\limits _ { 0 } ^ \infty t ^ {z-} 1 e ^ {-} t d t ,\ \mathop{\rm Re} z > 0 , | ||
| + | $$ | ||
| − | + | represents the [[Gamma-function|gamma-function]] $ \Gamma ( z) $ | |
| + | for $ \mathop{\rm Re} z > 0 $, | ||
| + | and if the contour of integration $ L _ {1} $ | ||
| + | is taken to be a loop (Fig. a), then one obtains the integral representation | ||
| − | + | $$ | |
| + | \Gamma ( z) = \ | ||
| − | + | \frac{1}{e ^ {2 i \pi z } - 1 } | |
| − | + | \int\limits _ {L _ {1} } | |
| + | \zeta ^ {z-} 1 e ^ {- \zeta } d \zeta , | ||
| + | $$ | ||
| − | which holds for all | + | which holds for all $ z $ |
| + | except for the points $ z = - 1 , - 2 \dots $ | ||
| + | at which $ \Gamma ( z) $ | ||
| + | has simple poles. | ||
<img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/i051620a.gif" /> | <img style="border:1px solid;" src="https://www.encyclopediaofmath.org/legacyimages/common_img/i051620a.gif" /> | ||
| Line 49: | Line 143: | ||
Similarly, there is an analytic continuation of the Euler integral of the first kind, | Similarly, there is an analytic continuation of the Euler integral of the first kind, | ||
| − | + | $$ | |
| + | B ( z , w ) = \ | ||
| + | \int\limits _ { 0 } ^ { 1 } | ||
| + | t ^ {z-} 1 ( 1 - t ) ^ {w-} 1 d t ,\ \ | ||
| + | \mathop{\rm Re} z , \mathop{\rm Re} w > 0 , | ||
| + | $$ | ||
| − | expressing the [[Beta-function|beta-function]] | + | expressing the [[Beta-function|beta-function]] $ B ( z , w ) $ |
| + | for $ \mathop{\rm Re} z , \mathop{\rm Re} w > 0 $. | ||
| + | It is obtained by going over a contour in the form of a double loop $ L _ {2} $( | ||
| + | Fig. b). | ||
One has studied integral representations of special functions (see [[#References|[1]]], [[#References|[2]]]) as well as integral representations of very wide classes of functions, in connection with integral transforms [[#References|[7]]]. | One has studied integral representations of special functions (see [[#References|[1]]], [[#References|[2]]]) as well as integral representations of very wide classes of functions, in connection with integral transforms [[#References|[7]]]. | ||
| Line 57: | Line 159: | ||
Of universal character in the theory of analytic functions is the singular Cauchy kernel | Of universal character in the theory of analytic functions is the singular Cauchy kernel | ||
| − | + | $$ | |
| + | K ( z , \zeta ) = \ | ||
| + | |||
| + | \frac{1}{2 \pi i } | ||
| + | |||
| + | \frac{1}{( \zeta - z ) } | ||
| + | |||
| + | $$ | ||
and the corresponding integral representation, the [[Cauchy integral|Cauchy integral]] | and the corresponding integral representation, the [[Cauchy integral|Cauchy integral]] | ||
| − | + | $$ \tag{4 } | |
| + | f ( z) = \ | ||
| + | |||
| + | \frac{1}{2 \pi i } | ||
| + | |||
| + | \int\limits _ { L } | ||
| + | |||
| + | \frac{f ( \zeta ) d \zeta }{\zeta - z } | ||
| + | . | ||
| + | $$ | ||
| + | |||
| + | This integral representation expresses the values of a single-valued analytic function $ f ( z) $ | ||
| + | in a domain $ D $ | ||
| + | bounded by a simple closed contour $ L $( | ||
| + | or a system of such contours), for example, in the case when the function $ f ( z) $ | ||
| + | is continuous in the closed domain $ \overline{D}\; = D \cup L $; | ||
| + | in the complementary domain $ C \overline{D}\; $, | ||
| + | $ \infty \in C \overline{D}\; $, | ||
| + | the integral on the right-hand side of (4) vanishes identically. The fundamental role of the representation (4) in the theory of analytic functions is due to the fact that the Cauchy integral is the convolution of $ f ( z) $ | ||
| + | with the fundamental solution $ 1 / ( 2 \pi i z ) $ | ||
| + | of the Cauchy–Riemann operator | ||
| + | |||
| + | $$ | ||
| + | |||
| + | \frac \partial {\partial \overline{z}\; } | ||
| + | = \ | ||
| + | |||
| + | \frac{1}{2} | ||
| + | |||
| + | \left ( | ||
| + | |||
| + | \frac \partial {\partial x } | ||
| + | + i | ||
| − | + | \frac \partial {\partial y } | |
| − | + | \right ) . | |
| + | $$ | ||
| − | Therefore all the fundamental properties of the analytic function can be obtained from the representation (4). From the point of view of general properties of integral representations of analytic functions, the Cauchy integral is distinguished by the especially simple structure of the kernel and by the fact that the density | + | Therefore all the fundamental properties of the analytic function can be obtained from the representation (4). From the point of view of general properties of integral representations of analytic functions, the Cauchy integral is distinguished by the especially simple structure of the kernel and by the fact that the density $ v ( \zeta ) = f ( \zeta ) $ |
| + | coincides with the values of the represented function on the contour $ L $. | ||
| + | This last property remains true if under the integral sign in (4), the Cauchy kernel $ 1 / 2 \pi i ( \zeta - z ) $ | ||
| + | is replaced by any function $ K ( z , \zeta ) $ | ||
| + | that is a single-valued analytic function in $ z $ | ||
| + | in a closed domain $ D $ | ||
| + | and has a simple pole at the point $ z = \zeta $ | ||
| + | with residue 1. The Cauchy kernel is the simplest of such functions $ K ( z , \zeta ) $, | ||
| + | but the above method of choosing the kernel in the Cauchy integral is often used in the solution of boundary value problems. | ||
| − | The fact that the density | + | The fact that the density $ v ( \zeta ) $ |
| + | coincides with the boundary value $ f ( \zeta ) $ | ||
| + | of the analytic function $ f ( z) $ | ||
| + | is in essence merely a form of expressing the property of analyticity. In using the integral representation (4) with an a priori arbitrarily given density $ v ( \zeta ) $, | ||
| + | an integral of Cauchy type is obtained in which the relationship between the density and the boundary values is expressed in a considerably more complex way in terms of a singular integral over the contour $ L $. | ||
In boundary value problems of analytic function theory the role of Cauchy-type integrals and their modifications is exceptionally important for the solution of singular integral equations (see [[#References|[5]]], [[#References|[6]]]). | In boundary value problems of analytic function theory the role of Cauchy-type integrals and their modifications is exceptionally important for the solution of singular integral equations (see [[#References|[5]]], [[#References|[6]]]). | ||
| Line 75: | Line 229: | ||
In studying the interior and boundary properties of analytic functions of various classes, more general integral representations of analytic functions than (1) are used, in the form of integrals depending on a parameter, | In studying the interior and boundary properties of analytic functions of various classes, more general integral representations of analytic functions than (1) are used, in the form of integrals depending on a parameter, | ||
| − | + | $$ \tag{5 } | |
| + | f ( z) = \int\limits _ { L } K ( z , \zeta ) d \mu ( \zeta ) , | ||
| + | $$ | ||
| + | |||
| + | with respect to a Borel boundary measure $ \mu $ | ||
| + | which is in general complex, concentrated on the contour $ L $ | ||
| + | bounding $ D $ | ||
| + | and can be expressed by some procedure or other in terms of the function $ f ( z) $ | ||
| + | being represented. | ||
| + | |||
| + | For example, all functions $ f ( z) $ | ||
| + | that are regular in the unit disc $ D = \{ {z } : {| z | < 1 } \} $ | ||
| + | and have positive real part, $ \mathop{\rm Re} f ( z) > 0 $, | ||
| + | can be characterized by their representation in the Herglotz formula | ||
| + | |||
| + | $$ | ||
| + | f ( z) = \int\limits | ||
| + | |||
| + | \frac{\zeta + z }{\zeta - z } | ||
| + | d \mu ( \zeta ) + i c ,\ \ | ||
| + | \mathop{\rm Im} c = 0 , | ||
| + | $$ | ||
| − | + | the idea of which essentially goes back to the [[Schwarz integral|Schwarz integral]]. Here $ \mu $ | |
| + | is an arbitrary positive measure concentrated on the circle $ L = \{ \zeta : {| \zeta | = 1 } \} $. | ||
| + | In the theory of univalent functions, a wide variety of other integral representations of analytic functions of type (5) find important applications; they are also known under the name of parametric representations or structural formulas (cf. [[Parametric representation of univalent functions|Parametric representation of univalent functions]]). Thus, for the class of typically-real functions $ f ( z) = z + c _ {2} z ^ {2} + \dots $ | ||
| + | in the disc $ D = \{ {z } : {| z | < 1 } \} $( | ||
| + | that is, functions $ f ( z) $ | ||
| + | for which $ \mathop{\rm Im} f ( x) = 0 $ | ||
| + | for $ - 1 < x < 1 $ | ||
| + | and $ \mathop{\rm Im} f ( z) \cdot \mathop{\rm Im} z > 0 $ | ||
| + | for $ \mathop{\rm Im} z \neq 0 $) | ||
| + | a typical representation is | ||
| − | + | $$ | |
| + | f ( z) = \int\limits | ||
| − | + | \frac{z d \mu ( \zeta ) }{( z - \zeta ) ( z - \overline \zeta \; ) } | |
| + | , | ||
| + | $$ | ||
| − | + | where $ \mu $ | |
| + | is an arbitrary measure concentrated on the circle $ L $ | ||
| + | and normalized by the condition $ \| \mu \| = \int d \mu ( \zeta ) = 1 $. | ||
| + | One also often uses a modification of the representation (5) in the form | ||
| − | + | $$ | |
| + | f ( z) = \phi | ||
| + | \left \{ | ||
| + | \int\limits K ( z , \zeta ) d \mu ( \zeta ) | ||
| + | \right \} , | ||
| + | $$ | ||
| − | where | + | where $ \phi : \mathbf C \rightarrow \mathbf C $ |
| + | is a specially chosen function, as simple as possible, for example $ \phi ( z) = \mathop{\rm exp} z $. | ||
| − | + | By regarding the measure $ \mu $ | |
| + | as a functional, the representation (5) can be interpreted as the value $ \langle \mu _ \zeta , K ( z , \zeta ) \rangle $ | ||
| + | of the functional $ \mu $ | ||
| + | on the kernel $ K ( z , \zeta ) $. | ||
| + | Consequently, a development of the method of integral representations of analytic functions is the analytic representation of generalized functions $ V $ | ||
| + | as the value of $ V $ | ||
| + | on the kernel $ K ( z , \zeta ) $: | ||
| − | + | $$ \tag{6 } | |
| + | \widehat{V} ( z) = \langle V _ \zeta , K ( z , \zeta ) \rangle . | ||
| + | $$ | ||
| − | + | Here, in the complement of the support of $ V $ | |
| + | the function $ \widehat{V} ( z) $ | ||
| + | is analytic (the kernel $ K ( z , \zeta ) $ | ||
| + | is assumed to be analytic in $ z $ | ||
| + | for $ z \neq \zeta $). | ||
| + | Representations of the form (6) find important applications in mathematical physics (see [[#References|[10]]], [[#References|[11]]]). | ||
| − | + | In the theory of analytic functions $ f ( z) $ | |
| + | of several complex variables $ z = ( z _ {1} \dots z _ {n} ) $, | ||
| + | $ n \geq 1 $, | ||
| + | integral representations in their simplest form can be expressed by a general formula: | ||
| − | + | $$ \tag{7 } | |
| + | f ( z) = \int\limits v ( \zeta ) \omega ( z , \zeta ) , | ||
| + | $$ | ||
| − | + | where $ v ( \zeta ) $ | |
| + | is a density, somehow related to $ f ( z) $, | ||
| + | $ \omega ( z , \zeta ) $ | ||
| + | is a differential form in the variables $ \zeta = ( \zeta _ {1} \dots \zeta _ {n} ) $, | ||
| + | $ \overline \zeta \; = ( \overline \zeta \; _ {1} \dots \overline \zeta \; _ {n} ) $, | ||
| + | whose coefficients depend on the parameters $ z = ( z _ {1} \dots z _ {n} ) $, | ||
| + | $ \overline{z}\; = ( \overline{z}\; _ {1} \dots \overline{z}\; _ {n} ) $, | ||
| + | and the integration is carried out over the entire boundary $ \partial D $ | ||
| + | of the domain of definition $ D $ | ||
| + | of $ f ( z) $ | ||
| + | or over some part of it. Representations in the form of a linear combination of integrals of the type (7) have also been used. For example, a function $ f ( z) $ | ||
| + | holomorphic in a polydisc-type domain $ D = D _ {1} \times \dots \times D _ {n} $ | ||
| + | and continuous in the closure $ \overline{D}\; $ | ||
| + | is representable throughout $ D $ | ||
| + | by a Cauchy integral: | ||
| − | + | $$ | |
| + | f ( z ) = \int\limits f ( \zeta ) \omega ( z , \zeta ) , | ||
| + | $$ | ||
| − | where | + | where the differential form $ \omega $ |
| + | has a very simple form: | ||
| − | + | $$ | |
| + | \omega ( z , \zeta ) = \ | ||
| − | + | \frac{1}{( 2 \pi i ) ^ {n} } | |
| − | + | \frac{d \zeta _ {1} \wedge \dots \wedge d \zeta _ {n} }{( \zeta _ {1} - z _ {1} ) \dots ( \zeta _ {n} - z _ {n} ) } | |
| + | = \ | ||
| + | K ( z , \zeta ) d \zeta , | ||
| + | $$ | ||
| − | and the integration is carried out over the skeleton | + | and the integration is carried out over the skeleton $ \Gamma = \partial D _ {1} \times {} \dots \times \partial D _ {n} $ |
| + | of $ D $( | ||
| + | see [[Bergman–Weil representation|Bergman–Weil representation]]; [[Leray formula|Leray formula]]; [[Bochner–Martinelli representation formula|Bochner–Martinelli representation formula]], and also [[#References|[8]]], [[#References|[9]]]). | ||
As in the case of a single complex variable, the further development of integral representations (7) are representations of the form: | As in the case of a single complex variable, the further development of integral representations (7) are representations of the form: | ||
| − | + | $$ | |
| + | f ( z) = \langle V _ \zeta , K ( z , \zeta ) \rangle | ||
| + | $$ | ||
or | or | ||
| − | + | $$ | |
| + | f ( z) = \langle V _ \zeta , \omega ( z , \zeta ) \rangle , | ||
| + | $$ | ||
| − | expressing the analytic function | + | expressing the analytic function $ f ( z) $ |
| + | in some domain in the form of the value of a functional $ V $ | ||
| + | on the kernel $ K ( z , \zeta ) $ | ||
| + | or on the kernel-form $ \omega ( z , \zeta ) $. | ||
| + | Here $ V $ | ||
| + | is interpreted, respectively, as a generalized function on a definite function space or as a current on a definite space of differential forms. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> V.I. Smirnov, "A course of higher mathematics" , '''2''' , Addison-Wesley (1964) (Translated from Russian) {{MR|0182690}} {{MR|0182688}} {{MR|0182687}} {{MR|0177069}} {{MR|0168707}} {{ZBL|0122.29703}} {{ZBL|0121.25904}} {{ZBL|0118.28402}} {{ZBL|0117.03404}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Kratzer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960) {{MR|0124531}} {{ZBL|0093.07101}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> I.I. [I.I. Privalov] Priwalow, "Randeigenschaften analytischer Funktionen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian) {{MR|0083565}} {{ZBL|}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> G.M. Goluzin, "Geometric theory of functions of a complex variable" , ''Transl. Math. Monogr.'' , '''26''' , Amer. Math. Soc. (1969) (Translated from Russian) {{MR|0247039}} {{ZBL|0183.07502}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> N.I. Muskhelishvili, "Singular integral equations" , Wolters-Noordhoff (1972) (Translated from Russian) {{MR|0355494}} {{ZBL|0488.45002}} {{ZBL|0174.16202}} {{ZBL|0174.16201}} {{ZBL|0103.07502}} {{ZBL|0108.29203}} {{ZBL|0051.33203}} {{ZBL|0041.22601}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> F.D. Gakhov, "Boundary value problems" , Pergamon (1966) (Translated from Russian) {{MR|0198152}} {{ZBL|0141.08001}} </TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> M.M. Dzhrbashyan, "Integral transforms and representation of functions in the complex domain" , Moscow (1966) (In Russian)</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian)</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> B.V. Shabat, "Introduction of complex analysis" , '''1–2''' , Moscow (1976) (In Russian) {{MR|}} {{ZBL|0799.32001}} {{ZBL|0732.32001}} {{ZBL|0732.30001}} {{ZBL|0578.32001}} {{ZBL|0574.30001}} </TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> H. Bremermann, "Distributions, complex variables and Fourier transforms" , Addison-Wesley (1965) {{MR|0208364}} {{ZBL|0151.18102}} </TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> V.S. Vladimirov, "Generalized functions in mathematical physics" , MIR (1977) (Translated from Russian) {{MR|0703520}} {{MR|0564116}} {{MR|0549767}} {{MR|0450966}} {{ZBL|0485.00014}} {{ZBL|0515.46034}} {{ZBL|0515.46033}} </TD></TR></table> |
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| Line 138: | Line 382: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> G.M. [G.M. Khenkin] Henkin, J. Leiterer, "Theory of functions on complex manifolds" , Birkhäuser (1984) {{MR|0795028}} {{MR|0774049}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> R.M. Range, "Holomorphic functions and integral representation in several complex variables" , Springer (1986) {{MR|0847923}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> S.G. Krantz, "Function theory of several complex variables" , Wiley (1982) {{MR|0635928}} {{ZBL|0471.32008}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> S. Bergman, "The kernel function and conformal mapping" , Amer. Math. Soc. (1950) {{MR|0038439}} {{ZBL|0040.19001}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> C. Carathéodory, "Theory of functions of a complex variable" , Chelsea, reprint (1954) (Translated from German) {{MR|1570711}} {{MR|0064861}} {{MR|0060009}} {{ZBL|0056.06703}} {{ZBL|0055.30301}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> L.V. Ahlfors, "Complex analysis" , McGraw-Hill (1978) {{MR|0510197}} {{ZBL|0395.30001}} {{ZBL|0154.31904}} {{ZBL|0052.07002}} </TD></TR></table> |
Latest revision as of 22:12, 5 June 2020
The representation of an analytic function by an integral depending on a parameter. Integral representations of analytic functions arose in the early stages of development of function theory and mathematical analysis in general as a suitable apparatus for the explicit representation of analytic solutions of differential equations, for the investigation of the asymptotics of these solutions and for their analytic continuation. Somewhat later integral representations of analytic functions found application in the solution of boundary value problems of analytic function theory and singular integral equations (cf. Singular integral equation), in the study of interior and boundary properties of analytic functions of various classes, as well as in the solution of other problems of mathematical analysis. In the process of development of function theory, the study of properties of the most important individual integral representations of analytic functions established an independent chapter in function theory (see, for example, Cauchy integral; Poisson integral; Schwarz integral).
A wide class of integral representations of analytic functions, used for obtaining and studying analytic solutions of differential equations, can be described by the general formula
$$ \tag{1 } f ( z) = \int\limits _ { L } K ( z , \zeta ) v ( \zeta ) \ d \zeta , $$
where $ K ( z , \zeta ) $ is the kernel of the integral representation, $ v ( \zeta ) $ is its density and $ L $ is a contour (or system of contours) in the complex plane in which both variables $ z $ and $ \zeta $ vary. An appropriate and, as far as possible, simplest solution of the three interrelated questions on the choice of the kernel $ K $, the density $ v $ and the contour $ L $ for the representation of a given function $ f ( z) $( or given class of functions) is the determining factor from the point of view of the successful application of the method of integral representations of analytic functions. In turn, the properties of the representation (1) depend essentially on whether the kernel $ K ( z , \zeta ) $ is an entire function of the complex variables $ z , \zeta $, or whether it is singular, that is, has some singularities. In general, the kernel of an integral representation of an analytic function does not have to be an analytic function of the variables $ z , \zeta $; the analyticity of $ f ( z) $ may be ensured by specific properties of the density. Nor is it necessary, in general, that formula (1) be a formula with a single integration; there are types of integral representations of analytic functions in which iterated integrals are used.
The general scheme for obtaining integral representations of special functions $ f ( z) $ that are solutions of certain ordinary differential equations $ \mathfrak L _ {z} [ f ] ( z) = 0 $ reduces in the main to the following. By an appropriate choice of the kernel $ K $, most often non-singular, the following formula for the action of the operator $ \mathfrak L _ {z} $ should hold:
$$ \tag{2 } \mathfrak L _ {z} [ f ] ( z) = \ \int\limits _ { L } \mathfrak L _ {z} [ K ] ( z , \zeta ) v ( \zeta ) d \zeta = $$
$$ = \ \int\limits _ { L } \mathfrak M _ \zeta [ K ] ( z , \zeta ) v ( \zeta ) d \zeta = $$
$$ = \ \int\limits _ { L } K ( z , \zeta ) \widetilde{\mathfrak L} _ \zeta [ v ] ( \zeta ) d \zeta + P [ v , K ] , $$
that is, the kernel must, in turn, as far as possible satisfy a simple partial differential equation $ \mathfrak L _ {z} [ K ] = \mathfrak M _ \zeta [ K ] $, thus allowing successive integration by parts with the aim of recovering the original form of the kernel and a transfer to the action of the adjoint operator $ \widetilde{\mathfrak L} _ \zeta $ thus obtained on the density $ v $. Having obtained a formula of the type (2), one selects a fairly simple density $ v $ satisfying the adjoint equation $ \widetilde{\mathfrak L} _ \zeta [ v ] ( \zeta ) = 0 $, and a contour $ L $ guaranteeing that the term $ P [ v , K ] $ vanishes. Here one must bear in mind that the choice of the contour $ L $ determines a particular solution of the original equation $ \mathfrak L _ {z} [ f ] ( z) = 0 $. The following kernels are used most often:
$$ K ( z , \zeta ) = e ^ {z \zeta } \ \ \textrm{ or } \ \ K ( z , \zeta ) = e ^ {i z \zeta } , $$
$$ K ( z , \zeta ) = z ^ \zeta \ \textrm{ and } \ \ K ( z , \zeta ) = ( z - \zeta ) ^ \alpha , $$
sometimes called the Laplace–Fourier kernel, the Mellin kernel and the Euler kernel, respectively. Various changes of variables lead to modified forms of the kernels. In the form written above, integral representations of analytic functions are closely related with the method of integral transforms (cf. Integral transform).
In this way, for example, a well-known integral representation of the Bessel functions is obtained:
$$ \tag{3 } J _ {p} ( z) = \ \frac{\Gamma ( 1 / 2 - p ) }{2 i \pi ^ {3/2} } \left ( \frac{z}{2} \right ) ^ {p} \int\limits _ { L } e ^ {i z \zeta } ( \zeta ^ {2} - 1 ) ^ {p-} 1/2 d \zeta , $$
where the contour $ L $ takes the form of a figure eight containing the points $ - 1 $ and $ + 1 $. The representation (3) is distinctive in that, on the one hand, its density $ v ( \zeta ) = ( \zeta ^ {2} - 1 ) ^ {p-} 1/2 $ is considerably simpler than the transcendental functions $ J _ {p} ( z) $ being represented, and on the other hand it enables one to survey fairly simply the properties of the functions $ J _ {p} ( z) $, in particular, to study their asymptotics.
An appropriate modification of the contour $ L $ enables one to obtain analytic continuations, in other words, to obtain integral representations that can be used throughout the entire domain of existence. For example, the Euler integral of the second kind,
$$ \Gamma ( z) = \ \int\limits _ { 0 } ^ \infty t ^ {z-} 1 e ^ {-} t d t ,\ \mathop{\rm Re} z > 0 , $$
represents the gamma-function $ \Gamma ( z) $ for $ \mathop{\rm Re} z > 0 $, and if the contour of integration $ L _ {1} $ is taken to be a loop (Fig. a), then one obtains the integral representation
$$ \Gamma ( z) = \ \frac{1}{e ^ {2 i \pi z } - 1 } \int\limits _ {L _ {1} } \zeta ^ {z-} 1 e ^ {- \zeta } d \zeta , $$
which holds for all $ z $ except for the points $ z = - 1 , - 2 \dots $ at which $ \Gamma ( z) $ has simple poles.
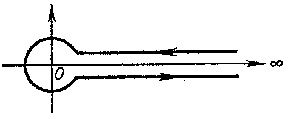
Figure: i051620a
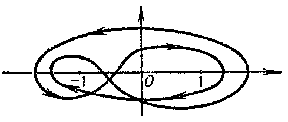
Figure: i051620b
Similarly, there is an analytic continuation of the Euler integral of the first kind,
$$ B ( z , w ) = \ \int\limits _ { 0 } ^ { 1 } t ^ {z-} 1 ( 1 - t ) ^ {w-} 1 d t ,\ \ \mathop{\rm Re} z , \mathop{\rm Re} w > 0 , $$
expressing the beta-function $ B ( z , w ) $ for $ \mathop{\rm Re} z , \mathop{\rm Re} w > 0 $. It is obtained by going over a contour in the form of a double loop $ L _ {2} $( Fig. b).
One has studied integral representations of special functions (see [1], [2]) as well as integral representations of very wide classes of functions, in connection with integral transforms [7].
Of universal character in the theory of analytic functions is the singular Cauchy kernel
$$ K ( z , \zeta ) = \ \frac{1}{2 \pi i } \frac{1}{( \zeta - z ) } $$
and the corresponding integral representation, the Cauchy integral
$$ \tag{4 } f ( z) = \ \frac{1}{2 \pi i } \int\limits _ { L } \frac{f ( \zeta ) d \zeta }{\zeta - z } . $$
This integral representation expresses the values of a single-valued analytic function $ f ( z) $ in a domain $ D $ bounded by a simple closed contour $ L $( or a system of such contours), for example, in the case when the function $ f ( z) $ is continuous in the closed domain $ \overline{D}\; = D \cup L $; in the complementary domain $ C \overline{D}\; $, $ \infty \in C \overline{D}\; $, the integral on the right-hand side of (4) vanishes identically. The fundamental role of the representation (4) in the theory of analytic functions is due to the fact that the Cauchy integral is the convolution of $ f ( z) $ with the fundamental solution $ 1 / ( 2 \pi i z ) $ of the Cauchy–Riemann operator
$$ \frac \partial {\partial \overline{z}\; } = \ \frac{1}{2} \left ( \frac \partial {\partial x } + i \frac \partial {\partial y } \right ) . $$
Therefore all the fundamental properties of the analytic function can be obtained from the representation (4). From the point of view of general properties of integral representations of analytic functions, the Cauchy integral is distinguished by the especially simple structure of the kernel and by the fact that the density $ v ( \zeta ) = f ( \zeta ) $ coincides with the values of the represented function on the contour $ L $. This last property remains true if under the integral sign in (4), the Cauchy kernel $ 1 / 2 \pi i ( \zeta - z ) $ is replaced by any function $ K ( z , \zeta ) $ that is a single-valued analytic function in $ z $ in a closed domain $ D $ and has a simple pole at the point $ z = \zeta $ with residue 1. The Cauchy kernel is the simplest of such functions $ K ( z , \zeta ) $, but the above method of choosing the kernel in the Cauchy integral is often used in the solution of boundary value problems.
The fact that the density $ v ( \zeta ) $ coincides with the boundary value $ f ( \zeta ) $ of the analytic function $ f ( z) $ is in essence merely a form of expressing the property of analyticity. In using the integral representation (4) with an a priori arbitrarily given density $ v ( \zeta ) $, an integral of Cauchy type is obtained in which the relationship between the density and the boundary values is expressed in a considerably more complex way in terms of a singular integral over the contour $ L $.
In boundary value problems of analytic function theory the role of Cauchy-type integrals and their modifications is exceptionally important for the solution of singular integral equations (see [5], [6]).
In studying the interior and boundary properties of analytic functions of various classes, more general integral representations of analytic functions than (1) are used, in the form of integrals depending on a parameter,
$$ \tag{5 } f ( z) = \int\limits _ { L } K ( z , \zeta ) d \mu ( \zeta ) , $$
with respect to a Borel boundary measure $ \mu $ which is in general complex, concentrated on the contour $ L $ bounding $ D $ and can be expressed by some procedure or other in terms of the function $ f ( z) $ being represented.
For example, all functions $ f ( z) $ that are regular in the unit disc $ D = \{ {z } : {| z | < 1 } \} $ and have positive real part, $ \mathop{\rm Re} f ( z) > 0 $, can be characterized by their representation in the Herglotz formula
$$ f ( z) = \int\limits \frac{\zeta + z }{\zeta - z } d \mu ( \zeta ) + i c ,\ \ \mathop{\rm Im} c = 0 , $$
the idea of which essentially goes back to the Schwarz integral. Here $ \mu $ is an arbitrary positive measure concentrated on the circle $ L = \{ \zeta : {| \zeta | = 1 } \} $. In the theory of univalent functions, a wide variety of other integral representations of analytic functions of type (5) find important applications; they are also known under the name of parametric representations or structural formulas (cf. Parametric representation of univalent functions). Thus, for the class of typically-real functions $ f ( z) = z + c _ {2} z ^ {2} + \dots $ in the disc $ D = \{ {z } : {| z | < 1 } \} $( that is, functions $ f ( z) $ for which $ \mathop{\rm Im} f ( x) = 0 $ for $ - 1 < x < 1 $ and $ \mathop{\rm Im} f ( z) \cdot \mathop{\rm Im} z > 0 $ for $ \mathop{\rm Im} z \neq 0 $) a typical representation is
$$ f ( z) = \int\limits \frac{z d \mu ( \zeta ) }{( z - \zeta ) ( z - \overline \zeta \; ) } , $$
where $ \mu $ is an arbitrary measure concentrated on the circle $ L $ and normalized by the condition $ \| \mu \| = \int d \mu ( \zeta ) = 1 $. One also often uses a modification of the representation (5) in the form
$$ f ( z) = \phi \left \{ \int\limits K ( z , \zeta ) d \mu ( \zeta ) \right \} , $$
where $ \phi : \mathbf C \rightarrow \mathbf C $ is a specially chosen function, as simple as possible, for example $ \phi ( z) = \mathop{\rm exp} z $.
By regarding the measure $ \mu $ as a functional, the representation (5) can be interpreted as the value $ \langle \mu _ \zeta , K ( z , \zeta ) \rangle $ of the functional $ \mu $ on the kernel $ K ( z , \zeta ) $. Consequently, a development of the method of integral representations of analytic functions is the analytic representation of generalized functions $ V $ as the value of $ V $ on the kernel $ K ( z , \zeta ) $:
$$ \tag{6 } \widehat{V} ( z) = \langle V _ \zeta , K ( z , \zeta ) \rangle . $$
Here, in the complement of the support of $ V $ the function $ \widehat{V} ( z) $ is analytic (the kernel $ K ( z , \zeta ) $ is assumed to be analytic in $ z $ for $ z \neq \zeta $). Representations of the form (6) find important applications in mathematical physics (see [10], [11]).
In the theory of analytic functions $ f ( z) $ of several complex variables $ z = ( z _ {1} \dots z _ {n} ) $, $ n \geq 1 $, integral representations in their simplest form can be expressed by a general formula:
$$ \tag{7 } f ( z) = \int\limits v ( \zeta ) \omega ( z , \zeta ) , $$
where $ v ( \zeta ) $ is a density, somehow related to $ f ( z) $, $ \omega ( z , \zeta ) $ is a differential form in the variables $ \zeta = ( \zeta _ {1} \dots \zeta _ {n} ) $, $ \overline \zeta \; = ( \overline \zeta \; _ {1} \dots \overline \zeta \; _ {n} ) $, whose coefficients depend on the parameters $ z = ( z _ {1} \dots z _ {n} ) $, $ \overline{z}\; = ( \overline{z}\; _ {1} \dots \overline{z}\; _ {n} ) $, and the integration is carried out over the entire boundary $ \partial D $ of the domain of definition $ D $ of $ f ( z) $ or over some part of it. Representations in the form of a linear combination of integrals of the type (7) have also been used. For example, a function $ f ( z) $ holomorphic in a polydisc-type domain $ D = D _ {1} \times \dots \times D _ {n} $ and continuous in the closure $ \overline{D}\; $ is representable throughout $ D $ by a Cauchy integral:
$$ f ( z ) = \int\limits f ( \zeta ) \omega ( z , \zeta ) , $$
where the differential form $ \omega $ has a very simple form:
$$ \omega ( z , \zeta ) = \ \frac{1}{( 2 \pi i ) ^ {n} } \frac{d \zeta _ {1} \wedge \dots \wedge d \zeta _ {n} }{( \zeta _ {1} - z _ {1} ) \dots ( \zeta _ {n} - z _ {n} ) } = \ K ( z , \zeta ) d \zeta , $$
and the integration is carried out over the skeleton $ \Gamma = \partial D _ {1} \times {} \dots \times \partial D _ {n} $ of $ D $( see Bergman–Weil representation; Leray formula; Bochner–Martinelli representation formula, and also [8], [9]).
As in the case of a single complex variable, the further development of integral representations (7) are representations of the form:
$$ f ( z) = \langle V _ \zeta , K ( z , \zeta ) \rangle $$
or
$$ f ( z) = \langle V _ \zeta , \omega ( z , \zeta ) \rangle , $$
expressing the analytic function $ f ( z) $ in some domain in the form of the value of a functional $ V $ on the kernel $ K ( z , \zeta ) $ or on the kernel-form $ \omega ( z , \zeta ) $. Here $ V $ is interpreted, respectively, as a generalized function on a definite function space or as a current on a definite space of differential forms.
References
| [1] | V.I. Smirnov, "A course of higher mathematics" , 2 , Addison-Wesley (1964) (Translated from Russian) MR0182690 MR0182688 MR0182687 MR0177069 MR0168707 Zbl 0122.29703 Zbl 0121.25904 Zbl 0118.28402 Zbl 0117.03404 |
| [2] | A. Kratzer, W. Franz, "Transzendente Funktionen" , Akademie Verlag (1960) MR0124531 Zbl 0093.07101 |
| [3] | I.I. [I.I. Privalov] Priwalow, "Randeigenschaften analytischer Funktionen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian) MR0083565 |
| [4] | G.M. Goluzin, "Geometric theory of functions of a complex variable" , Transl. Math. Monogr. , 26 , Amer. Math. Soc. (1969) (Translated from Russian) MR0247039 Zbl 0183.07502 |
| [5] | N.I. Muskhelishvili, "Singular integral equations" , Wolters-Noordhoff (1972) (Translated from Russian) MR0355494 Zbl 0488.45002 Zbl 0174.16202 Zbl 0174.16201 Zbl 0103.07502 Zbl 0108.29203 Zbl 0051.33203 Zbl 0041.22601 |
| [6] | F.D. Gakhov, "Boundary value problems" , Pergamon (1966) (Translated from Russian) MR0198152 Zbl 0141.08001 |
| [7] | M.M. Dzhrbashyan, "Integral transforms and representation of functions in the complex domain" , Moscow (1966) (In Russian) |
| [8] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian) |
| [9] | B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1976) (In Russian) Zbl 0799.32001 Zbl 0732.32001 Zbl 0732.30001 Zbl 0578.32001 Zbl 0574.30001 |
| [10] | H. Bremermann, "Distributions, complex variables and Fourier transforms" , Addison-Wesley (1965) MR0208364 Zbl 0151.18102 |
| [11] | V.S. Vladimirov, "Generalized functions in mathematical physics" , MIR (1977) (Translated from Russian) MR0703520 MR0564116 MR0549767 MR0450966 Zbl 0485.00014 Zbl 0515.46034 Zbl 0515.46033 |
Comments
The theory of integral representations of functions of several complex variables is in rapid development, cf. [a1]–[a3].
See also Kernel function; Bergman kernel function (and [a4]).
For one-variable theory see, e.g., [a5], [a6].
The consideration of generalized functions as (generalized) boundary values of analytic functions has led to the notion of a hyperfunction.
References
| [a1] | G.M. [G.M. Khenkin] Henkin, J. Leiterer, "Theory of functions on complex manifolds" , Birkhäuser (1984) MR0795028 MR0774049 |
| [a2] | R.M. Range, "Holomorphic functions and integral representation in several complex variables" , Springer (1986) MR0847923 |
| [a3] | S.G. Krantz, "Function theory of several complex variables" , Wiley (1982) MR0635928 Zbl 0471.32008 |
| [a4] | S. Bergman, "The kernel function and conformal mapping" , Amer. Math. Soc. (1950) MR0038439 Zbl 0040.19001 |
| [a5] | C. Carathéodory, "Theory of functions of a complex variable" , Chelsea, reprint (1954) (Translated from German) MR1570711 MR0064861 MR0060009 Zbl 0056.06703 Zbl 0055.30301 |
| [a6] | L.V. Ahlfors, "Complex analysis" , McGraw-Hill (1978) MR0510197 Zbl 0395.30001 Zbl 0154.31904 Zbl 0052.07002 |
Integral representation of an analytic function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Integral_representation_of_an_analytic_function&oldid=15180