|
|
| (4 intermediate revisions by one other user not shown) |
| Line 1: |
Line 1: |
| − | The set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800201.png" /> of real points of an [[Algebraic variety|algebraic variety]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800202.png" /> defined over the field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800203.png" /> of real numbers. A real algebraic variety is said to be non-singular if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800204.png" /> is non-singular. In such a case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800205.png" /> is a smooth variety, and its dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800206.png" /> is equal to the dimension of the complex variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800207.png" />; the latter is known as the complexification of the variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800208.png" />.
| + | <!-- |
| | + | r0800201.png |
| | + | $#A+1 = 170 n = 1 |
| | + | $#C+1 = 170 : ~/encyclopedia/old_files/data/R080/R.0800020 Real algebraic variety |
| | + | Automatically converted into TeX, above some diagnostics. |
| | + | Please remove this comment and the {{TEX|auto}} line below, |
| | + | if TeX found to be correct. |
| | + | --> |
| | | | |
| − | Non-singular regular complete intersections have been most thoroughly studied. These are varieties <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r0800209.png" /> in the projective space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002010.png" /> which are non-singular regular intersections of hypersurfaces <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002011.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002012.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002013.png" /> is a homogeneous real polynomial in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002014.png" /> variables of degree <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002015.png" />. In such a case the matrix
| + | {{TEX|auto}} |
| | + | {{TEX|done}} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002016.png" /></td> </tr></table>
| + | The set $ A = X ( \mathbf R ) $ |
| | + | of real points of an [[Algebraic variety|algebraic variety]] $ X $ |
| | + | defined over the field $ \mathbf R $ |
| | + | of real numbers. A real algebraic variety is said to be non-singular if $ X $ |
| | + | is non-singular. In such a case $ A $ |
| | + | is a smooth variety, and its dimension $ \mathop{\rm dim} A $ |
| | + | is equal to the dimension of the complex variety $ \mathbf C A = X ( \mathbf C ) $; |
| | + | the latter is known as the complexification of the variety $ A $. |
| | | | |
| − | has rank <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002017.png" /> at all points <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002018.png" />; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002019.png" />.
| + | Non-singular regular complete intersections have been most thoroughly studied. These are varieties $ X $ |
| | + | in the projective space $ \mathbf R P ^ {q} $ |
| | + | which are non-singular regular intersections of hypersurfaces $ p _ {i} ( z) = 0 $, |
| | + | $ 1 \leq i \leq s $, |
| | + | where $ p _ {i} ( z) $ |
| | + | is a homogeneous real polynomial in $ q $ |
| | + | variables of degree $ m _ {i} $. |
| | + | In such a case the matrix |
| | | | |
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002020.png" /> denote the real algebraic variety defined as the intersection system
| + | $$ |
| | + | \left \| |
| | + | \frac{\partial p _ {i} }{\partial z _ {j} } |
| | + | \right \| |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002021.png" /></td> </tr></table>
| + | has rank $ s $ |
| | + | at all points $ z \in \mathbf C A $; |
| | + | $ \mathop{\rm dim} A = n = q- s $. |
| | + | |
| | + | Let $ B $ |
| | + | denote the real algebraic variety defined as the intersection system |
| | + | |
| | + | $$ |
| | + | p _ {i} ( z) = 0 ,\ 1\leq i \leq s- 1,\ p( z) = p _ {s} ( z) \ \textrm{ and } \ \ |
| | + | m = m _ {s} . |
| | + | $$ |
| | | | |
| | Examples of regular complete intersections are: | | Examples of regular complete intersections are: |
| | | | |
| − | 1) A plane real algebraic curve; here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002022.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002023.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002024.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002025.png" />. | + | 1) A plane real algebraic curve; here $ q= 2 $, |
| | + | $ s= 1 $, |
| | + | $ \mathbf C B = \mathbf C P ^ {2} $, |
| | + | $ B = \mathbf R P ^ {2} $. |
| | + | |
| | + | 2) A real algebraic hypersurface; here $ s= 1 $, |
| | + | $ \mathbf C B = \mathbf C P ^ {q} $, |
| | + | $ B = \mathbf R P ^ {q} $. |
| | + | In particular, if $ q= 3 $, |
| | + | a real algebraic surface is obtained. |
| | | | |
| − | 2) A real algebraic hypersurface; here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002026.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002027.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002028.png" />. In particular, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002029.png" />, a real algebraic surface is obtained.
| + | 3) A real algebraic space curve; here $ q= 3 $, |
| | + | $ s= 2 $. |
| | + | The surface $ B $ |
| | + | is defined by an equation $ p _ {1} ( z) = 0 $, |
| | + | while the curve $ A $ |
| | + | is cut out on $ B $ |
| | + | by a surface $ p _ {2} ( z) = 0 $. |
| | | | |
| − | 3) A real algebraic space curve; here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002030.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002031.png" />. The surface <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002032.png" /> is defined by an equation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002033.png" />, while the curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002034.png" /> is cut out on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002035.png" /> by a surface <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002036.png" />.
| + | A real algebraic curve $ A $ |
| | + | of order $ m _ {1} $ |
| | + | in the plane $ \mathbf R P ^ {2} $ |
| | + | consists of finitely many components diffeomorphic to a circle. If $ m _ {1} $ |
| | + | is even, these components are all two-sidedly imbedded in $ \mathbf R P ^ {2} $; |
| | + | if $ m _ {1} $ |
| | + | is odd, one component is imbedded one-sidedly, while the remaining ones are imbedded two-sidedly. A two-sidedly imbedded component of $ A $ |
| | + | is called an oval of $ A $. |
| | + | An oval lying inside an odd number of other ovals of $ A $ |
| | + | is called odd, while the remaining ovals are even. |
| | | | |
| − | A real algebraic curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002037.png" /> of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002038.png" /> in the plane <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002039.png" /> consists of finitely many components diffeomorphic to a circle. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002040.png" /> is even, these components are all two-sidedly imbedded in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002041.png" />; if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002042.png" /> is odd, one component is imbedded one-sidedly, while the remaining ones are imbedded two-sidedly. A two-sidedly imbedded component of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002043.png" /> is called an oval of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002044.png" />. An oval lying inside an odd number of other ovals of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002045.png" /> is called odd, while the remaining ovals are even.
| + | The number of components of a plane real algebraic curve of order $ m _ {1} $ |
| | + | is not larger than $ ( m _ {1} - 1 ) ( m _ {1} - 2 ) / 2 + 1 $( |
| | + | Harnack's theorem) [[#References|[1]]]. For each $ m _ {1} $ |
| | + | there exists a plane real algebraic curve with this largest number of components — the $ M $- |
| | + | curve. (For methods of constructing $ M $- |
| | + | curves see [[#References|[1]]], [[#References|[2]]], [[#References|[3]]]; for a generalization of these results to include space curves, see [[#References|[2]]].) |
| | | | |
| − | The number of components of a plane real algebraic curve of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002046.png" /> is not larger than <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002047.png" /> (Harnack's theorem) [[#References|[1]]]. For each <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002048.png" /> there exists a plane real algebraic curve with this largest number of components — the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002050.png" />-curve. (For methods of constructing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002051.png" />-curves see [[#References|[1]]], [[#References|[2]]], [[#References|[3]]]; for a generalization of these results to include space curves, see [[#References|[2]]].)
| + | D. Hilbert posed in 1900 the problem of studying the topology of real algebraic varieties, and also of the imbedding of real algebraic varieties into $ \mathbf R P ^ {q} $ |
| | + | and of one real algebraic variety into another (Hilbert's 16th problem). He also pointed out difficult partial problems: the study of the mutual locations of the ovals of a sixth-order curve, and the topology and the imbedding of a real algebraic fourth-order surface into $ \mathbf R P ^ {3} $. |
| | + | These partial problems have been solved [[#References|[12]]], [[#References|[13]]]. |
| | | | |
| − | D. Hilbert posed in 1900 the problem of studying the topology of real algebraic varieties, and also of the imbedding of real algebraic varieties into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002052.png" /> and of one real algebraic variety into another (Hilbert's 16th problem). He also pointed out difficult partial problems: the study of the mutual locations of the ovals of a sixth-order curve, and the topology and the imbedding of a real algebraic fourth-order surface into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002053.png" />. These partial problems have been solved [[#References|[12]]], [[#References|[13]]].
| + | For a plane real algebraic curve $ A $ |
| | + | of even order $ m _ {1} $ |
| | + | the following exact inequality is valid: |
| | | | |
| − | For a plane real algebraic curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002054.png" /> of even order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002055.png" /> the following exact inequality is valid:
| + | $$ |
| | + | - |
| | + | \frac{1}{8} |
| | + | ( 3 m _ {1} ^ {2} - 6 m _ {1} ) \leq P - N \leq |
| | + | \frac{1}{8} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002056.png" /></td> </tr></table>
| + | ( 3 m _ {1} ^ {2} - 6 m _ {1} ) + 1 , |
| | + | $$ |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002057.png" /> is the number of even ovals and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002058.png" /> is the number of odd ovals of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002059.png" /> (Petrovskii's theorem). If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002060.png" /> is odd, a similar inequality is valid for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002061.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002062.png" /> is a straight line in general position [[#References|[4]]]. When these results are generalized to include the case of a real algebraic hypersurface of even order, the role of the difference <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002063.png" /> is played by the [[Euler characteristic|Euler characteristic]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002064.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002065.png" />, while if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002066.png" /> is odd, the role of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002067.png" /> is played by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002068.png" />. Thus, for a real algebraic hypersurface <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002069.png" /> of even order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002070.png" />, | + | where $ P $ |
| | + | is the number of even ovals and $ N $ |
| | + | is the number of odd ovals of $ A $( |
| | + | Petrovskii's theorem). If $ m _ {1} $ |
| | + | is odd, a similar inequality is valid for $ A \cup L $, |
| | + | where $ L $ |
| | + | is a straight line in general position [[#References|[4]]]. When these results are generalized to include the case of a real algebraic hypersurface of even order, the role of the difference $ P- N $ |
| | + | is played by the [[Euler characteristic|Euler characteristic]] $ \chi ( B _ {+} ) $, |
| | + | where $ B _ {+} = \{ {z \in B } : {p( z) \geq 0 } \} $, |
| | + | while if $ q $ |
| | + | is odd, the role of $ P- N $ |
| | + | is played by $ \chi ( A) $. |
| | + | Thus, for a real algebraic hypersurface $ A $ |
| | + | of even order $ m _ {1} $, |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002071.png" /></td> </tr></table>
| + | $$ |
| | + | | \chi ( B _ {+} ) | \leq |
| | + | \frac{( m _ {1} - 1) ^ {q} }{2} |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002072.png" /> is the number of terms of the polynomial
| + | - s( q; m _ {1} ) + |
| | + | \frac{1}{2} |
| | + | , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002073.png" /></td> </tr></table>
| + | where $ s ( q ; m _ {1)} $ |
| | + | is the number of terms of the polynomial |
| | | | |
| − | of degree not higher than <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002074.png" />; if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002075.png" /> is odd, then for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002076.png" />,
| + | $$ |
| | + | \prod _ {i = 1 } ^ { q } ( 1 + x _ {i} + \dots + x _ {i} ^ {m-2} ) , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002077.png" /></td> </tr></table>
| + | of degree not higher than $ ( qm _ {1} - 2q - m _ {1} ) / 2 $; |
| | + | if $ q $ |
| | + | is odd, then for any $ m _ {1} $, |
| | | | |
| − | [[#References|[5]]]. The following inequality is satisfied for a real algebraic space curve (in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002078.png" />) for even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002079.png" />:
| + | $$ |
| | + | | \chi ( A) | \leq ( m _ {1} - 1 ) ^ {q} - 2s ( q ; m _ {1} ) + 1 , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002080.png" /></td> </tr></table>
| + | [[#References|[5]]]. The following inequality is satisfied for a real algebraic space curve (in $ \mathbf R P ^ {3} $) |
| | + | for even $ m _ {1} $: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002081.png" /></td> </tr></table>
| + | $$ |
| | + | | \chi ( B _ {+} ) | \leq |
| | + | \frac{1}{3} |
| | + | m _ {1} ^ {3} + |
| | | | |
| − | (if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002082.png" />, this estimate is exact [[#References|[6]]]). Petrovskii's theorem has been generalized to arbitrary real algebraic varieties .
| + | \frac{3}{8} |
| | + | m _ {1} m _ {2} ^ {2} + |
| | + | \frac{1}{4} |
| | + | m _ {1} ^ {2} m _ {2} + |
| | + | $$ |
| | | | |
| − | For a plane real algebraic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002083.png" />-curve of even order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002084.png" /> the following congruence is valid:
| + | $$ |
| | + | - m _ {1} ^ {2} - m _ {1} m _ {2} + |
| | + | \frac{7}{6} |
| | + | m _ {1} + |
| | + | \frac{| \chi ( B) | }{2} |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002085.png" /></td> </tr></table>
| + | $$ |
| | | | |
| − | [[#References|[8]]], , [[#References|[13]]]. In proving this congruence ([[#References|[8]]], ), real algebraic varieties were studied by methods of differential topology in a form which opened the way for further investigations. Let the plane real algebraic curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002086.png" /> have even order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002087.png" /> and let the sign of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002088.png" /> be chosen so that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002089.png" /> is orientable, while <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002090.png" /> denote, respectively, the number of ovals of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002091.png" /> which externally bound the components of the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002092.png" /> with positive, zero and negative Euler characteristics. In a similar manner, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002093.png" /> are the numbers of such odd ovals for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002094.png" />. Then [[#References|[8]]], [[#References|[3]]],
| + | (if $ m _ {1} = 2 $, |
| | + | this estimate is exact [[#References|[6]]]). Petrovskii's theorem has been generalized to arbitrary real algebraic varieties . |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002095.png" /></td> </tr></table>
| + | For a plane real algebraic $ M $- |
| | + | curve of even order $ m _ {1} $ |
| | + | the following congruence is valid: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002096.png" /></td> </tr></table>
| + | $$ |
| | + | P - N \equiv \left ( |
| | + | \frac{m _ {1} }{2} |
| | + | \right ) ^ {2} \mathop{\rm mod} 8 , |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002097.png" /></td> </tr></table>
| + | [[#References|[8]]], , [[#References|[13]]]. In proving this congruence ([[#References|[8]]], ), real algebraic varieties were studied by methods of differential topology in a form which opened the way for further investigations. Let the plane real algebraic curve $ A $ |
| | + | have even order $ m = 2k $ |
| | + | and let the sign of $ p( z) $ |
| | + | be chosen so that $ B _ {+} $ |
| | + | is orientable, while $ P _ {+} , P _ {0} , P _ {-} $ |
| | + | denote, respectively, the number of ovals of $ A $ |
| | + | which externally bound the components of the set $ B _ {+} $ |
| | + | with positive, zero and negative Euler characteristics. In a similar manner, $ N _ {+} , N _ {0} , N _ {-} $ |
| | + | are the numbers of such odd ovals for $ B _ {-} = \{ {z \in B } : {p( z) \leq 0 } \} $. |
| | + | Then [[#References|[8]]], [[#References|[3]]], |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002098.png" /></td> </tr></table>
| + | $$ |
| | + | P _ {-} + P _ {0} \leq |
| | + | \frac{1}{2} |
| | + | ( k - 1 ) ( k - 2 ) + E ( k) , |
| | + | $$ |
| | + | |
| | + | $$ |
| | + | N _ {-} + N _ {0} \leq |
| | + | \frac{1}{2} |
| | + | ( k - 1 ) ( k - 2 ) , |
| | + | $$ |
| | + | |
| | + | $$ |
| | + | P _ {-} \geq N - |
| | + | \frac{3}{2} |
| | + | k ( k - 1 ) , |
| | + | $$ |
| | + | |
| | + | $$ |
| | + | N _ {-} \geq P - |
| | + | \frac{3}{2} |
| | + | k ( k - 1 ) , |
| | + | $$ |
| | | | |
| | where | | where |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r08002099.png" /></td> </tr></table>
| + | $$ |
| | + | E ( k) = |
| | + | \frac{1}{2} |
| | + | ( 1 + ( - 1 ) ^ {k} ) . |
| | + | $$ |
| | | | |
| − | For an arbitrary real algebraic variety in a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020100.png" />-dimensional projective space the following inequality is valid: | + | For an arbitrary real algebraic variety in a $ q $- |
| | + | dimensional projective space the following inequality is valid: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020101.png" /></td> </tr></table>
| + | $$ |
| | + | \mathop{\rm dim} H _ {*} ( A ; \mathbf Z _ {2} ) \leq \mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) , |
| | + | $$ |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020102.png" /> is the homology space of the variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020103.png" /> with coefficients in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020104.png" /> . This inequality is a generalization of Harnack's theorem. If | + | where $ H _ {*} ( A; \mathbf Z _ {2} ) = \sum H _ {i} ( A; \mathbf Z _ {2} ) $ |
| | + | is the homology space of the variety $ A $ |
| | + | with coefficients in $ \mathbf Z _ {2} $. |
| | + | This inequality is a generalization of Harnack's theorem. If |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020105.png" /></td> </tr></table>
| + | $$ |
| | + | \mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) - \mathop{\rm dim} H _ {*} ( A ; \mathbf Z _ {2} ) = 2t, |
| | + | $$ |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020106.png" /> is always an integer, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020107.png" /> is said to be an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020108.png" />-variety. If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020109.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020110.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020111.png" />-variety. | + | where $ t $ |
| | + | is always an integer, $ A $ |
| | + | is said to be an $ ( M- t) $- |
| | + | variety. If $ t= 0 $, |
| | + | $ A $ |
| | + | is an $ M $- |
| | + | variety. |
| | | | |
| | The validity of the following congruences has been demonstrated: | | The validity of the following congruences has been demonstrated: |
| | | | |
| − | A) For an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020112.png" />-variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020113.png" /> and for even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020114.png" />: | + | A) For an $ M $- |
| | + | variety $ A $ |
| | + | and for even $ n $: |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020115.png" /></td> </tr></table>
| + | $$ |
| | + | \chi ( A ) \equiv \sigma ( \mathbf C A ) \mathop{\rm mod} 16 , |
| | + | $$ |
| | | | |
| − | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020116.png" /> is the [[Signature|signature]] of the variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020117.png" /> . | + | where $ \sigma ( \mathbf C A ) $ |
| | + | is the [[signature]] of the variety $ \mathbf C A $. |
| | | | |
| − | B) For an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020118.png" />-variety <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020119.png" /> and even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020120.png" /> ([[#References|[13]]]): | + | B) For an $ ( M- 1) $- |
| | + | variety $ A $ |
| | + | and even $ n $([[#References|[13]]]): |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020121.png" /></td> </tr></table>
| + | $$ |
| | + | \chi ( A ) \equiv \sigma ( \mathbf C A ) \pm 2 \mathop{\rm mod} 16 , |
| | + | $$ |
| | | | |
| | cf. the overview [[#References|[3]]]. | | cf. the overview [[#References|[3]]]. |
| | | | |
| − | C) For a regular complete intersection, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020122.png" /> is even, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020123.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020124.png" />-variety and the inclusion homomorphism | + | C) For a regular complete intersection, if $ n $ |
| | + | is even, $ A $ |
| | + | is an $ ( M- 1) $- |
| | + | variety and the inclusion homomorphism |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020125.png" /></td> </tr></table>
| + | $$ |
| | + | i _ {*} : H _ {n / 2 } ( A ; \mathbf Z _ {2} ) \rightarrow H _ {n / 2 } |
| | + | ( \mathbf R P ^ {q} ; \mathbf Z _ {2} ) |
| | + | $$ |
| | | | |
| | is zero, then | | is zero, then |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020126.png" /></td> </tr></table>
| + | $$ |
| | + | d = m _ {1} m _ {2} \dots \equiv 2 \mathop{\rm mod} 4 |
| | + | $$ |
| | | | |
| | and | | and |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020127.png" /></td> </tr></table>
| + | $$ |
| | + | \chi ( A ) \equiv - \sigma ( \mathbf C A ) + |
| | + | \left \{ |
| | + | \begin{array}{rl} |
| | + | 2 \mathop{\rm mod} 16 & \textrm{ if } d \equiv 2 \mathop{\rm mod} 8 , \\ |
| | + | - 2 \mathop{\rm mod} 16 & \textrm{ if } d \equiv - 2 \mathop{\rm mod} 8 . \\ |
| | + | \end{array} |
| | | | |
| − | In this case, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020128.png" /> is even, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020129.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020130.png" />-variety and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020131.png" /> is zero ([[#References|[11]]]):
| + | \right .$$ |
| | | | |
| − | if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020132.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020133.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020134.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020135.png" />, | + | In this case, if $ n $ |
| | + | is even, $ A $ |
| | + | is an $ ( M- 2) $- |
| | + | variety and $ i _ {*} $ |
| | + | is zero ([[#References|[11]]]): |
| | | | |
| − | if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020136.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020137.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020138.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020139.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020140.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020141.png" />, | + | if $ d \equiv 0 $ |
| | + | $ \mathop{\rm mod} 8 $, |
| | + | $ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $ |
| | + | $ \mathop{\rm mod} 16 $, |
| | | | |
| − | if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020142.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020143.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020144.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020145.png" /> or <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020146.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020147.png" />. | + | if $ d \equiv 2 $ |
| | + | $ \mathop{\rm mod} 8 $, |
| | + | $ \chi ( A) \equiv - \sigma ( \mathbf C A ) + 4 $ |
| | + | $ \mathop{\rm mod} 16 $ |
| | + | or $ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $ |
| | + | $ \mathop{\rm mod} 16 $, |
| | | | |
| − | In particular, for a real algebraic surface <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020148.png" /> of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020149.png" />,
| + | if $ d \begin{array}{c} |
| | + | > \\ |
| | + | = |
| | + | \end{array} |
| | + | 2 $ |
| | + | $ \mathop{\rm mod} 8 $, |
| | + | $ \chi ( A) \equiv - \sigma ( \mathbf C A ) - 4 $ |
| | + | $ \mathop{\rm mod} 16 $ |
| | + | or $ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $ |
| | + | $ \mathop{\rm mod} 16 $. |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020150.png" /></td> </tr></table>
| + | In particular, for a real algebraic surface $ A $ |
| | + | of order $ m _ {1} $, |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020151.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020152.png" />-surface, then
| + | $$ |
| | + | \mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) = m _ {1} ^ {3} |
| | + | - 4 m _ {1} ^ {2} + 6 m _ {1} . |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020153.png" /></td> </tr></table>
| + | If $ A $ |
| | + | is an $ M $- |
| | + | surface, then |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020154.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020155.png" />-surface, then
| + | $$ |
| | + | \chi ( A) \equiv |
| | + | \frac{1}{3} |
| | + | ( 4 m _ {1} - m _ {1} ^ {3} ) \mathop{\rm mod} 16 . |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020156.png" /></td> </tr></table>
| + | If $ A $ |
| | + | is an $ ( M- 1) $- |
| | + | surface, then |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020157.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020158.png" />-surface and contracts to a point in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020159.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020160.png" /> <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020161.png" /> and
| + | $$ |
| | + | \chi ( A) \equiv |
| | + | \frac{1}{3} |
| | + | ( 4 m _ {1} - m _ {1} ^ {3} ) \pm 2 |
| | + | \mathop{\rm mod} 16 . |
| | + | $$ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020162.png" /></td> </tr></table>
| + | If $ A $ |
| | + | is an $ ( M- 1) $- |
| | + | surface and contracts to a point in $ \mathbf R P ^ {3} $, |
| | + | then $ m _ {1} \equiv 2 $ |
| | + | $ \mathop{\rm mod} 4 $ |
| | + | and |
| | | | |
| − | If <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020163.png" /> is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020164.png" />-surface and contracts to a point in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020165.png" />, then
| + | $$ |
| | + | \chi ( A) \equiv \left \{ |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020166.png" /></td> </tr></table>
| + | \begin{array}{rl} |
| | + | 2 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 2 \mathop{\rm mod} 8 , \\ |
| | + | - 2 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv - 2 \mathop{\rm mod} 8 . \\ |
| | + | \end{array} |
| | + | \right . |
| | + | $$ |
| | | | |
| − | Certain congruences have also been proved , [[#References|[13]]] for odd <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020167.png" />. In particular, for a plane real algebraic curve <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020168.png" /> which is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020169.png" />-curve of even order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020170.png" />:
| + | If $ A $ |
| | + | is an $ ( M- 2) $- |
| | + | surface and contracts to a point in $ \mathbf R P ^ {3} $, |
| | + | then |
| | | | |
| − | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020171.png" /></td> </tr></table>
| + | $$ |
| | + | \chi ( A) \equiv \left \{ |
| | + | \begin{array}{rl} |
| | + | 0 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 0 \mathop{\rm mod} 8, \\ |
| | + | 0 , 4 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 2 \mathop{\rm mod} 8 , \\ |
| | + | 0 , - 4 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv - 2 \mathop{\rm mod} 8 . \\ |
| | + | \end{array} |
| | + | |
| | + | \right .$$ |
| | + | |
| | + | Certain congruences have also been proved , [[#References|[13]]] for odd $ n $. |
| | + | In particular, for a plane real algebraic curve $ A $ |
| | + | which is an $ ( M- 1) $- |
| | + | curve of even order $ m _ {1} $: |
| | + | |
| | + | $$ |
| | + | P - N \equiv \left ( |
| | + | \frac{m _ 1}{2} |
| | + | \right ) ^ {2} |
| | + | \pm 1 \mathop{\rm mod} 8 . |
| | + | $$ |
| | | | |
| | Certain results have also been obtained [[#References|[13]]] for real algebraic varieties with singularities. For an interesting approach to the study of real algebraic varieties see [[#References|[14]]]. | | Certain results have also been obtained [[#References|[13]]] for real algebraic varieties with singularities. For an interesting approach to the study of real algebraic varieties see [[#References|[14]]]. |
| | | | |
| | ====References==== | | ====References==== |
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A. Harnack, "Ueber die Vieltheitigkeit der ebenen algebraischen Kurven" ''Math. Ann.'' , '''10''' (1876) pp. 189–198</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> D. Hilbert, "Ueber die reellen Züge algebraischer Kurven" ''Math. Ann.'' , '''38''' (1891) pp. 115–138</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> D. Hilbert, "Mathematische Probleme" ''Arch. Math. Phys.'' , '''1''' (1901) pp. 213–237</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> I.G. Petrovskii, "On the topology of real plane algebraic curves" ''Ann. of Math.'' , '''39''' : 1 (1938) pp. 189–209</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> O.A. Oleinik, I.G. Petrovskii, "On the topology of real algebraic surfaces" ''Transl. Amer. Math. Soc.'' , '''7''' (1952) pp. 399–417 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''13''' (1949) pp. 389–402</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> O.A. Oleinik, "On the topology of real algebraic curves on an algebraic surface" ''Mat. Sb.'' , '''29''' (1951) pp. 133–156 (In Russian)</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> , ''Hilbert problems'' , Moscow (1969) (In Russian)</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> V.I. Arnol'd, "Distribution of the ovals of the real plane of algebraic curves, of involutions of four-dimensional smooth manifolds, and the arithmetic of integer-valued quadratic forms" ''Funct. Anal. Appl.'' , '''5''' : 3 (1971) pp. 169–176 ''Funkts. Anal.'' , '''5''' : 3 (1971) pp. 1–9</TD></TR><TR><TD valign="top">[9a]</TD> <TD valign="top"> V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" ''Funct. Anal. Appl.'' , '''6''' : 4 (1972) pp. 301–306 ''Funkts. Anal.'' , '''6''' : 4 (1972) pp. 58–64</TD></TR><TR><TD valign="top">[9b]</TD> <TD valign="top"> V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" ''Funct. Anal. Appl.'' , '''7''' : 2 (1973) pp. 163–165 ''Funkts. Anal.'' , '''7''' : 2 (1973) pp. 91–92</TD></TR><TR><TD valign="top">[10a]</TD> <TD valign="top"> V.M. Kharlamov, "A generalized Petrovskii inequality" ''Funct. Anal. Appl.'' , '''8''' : 2 (1974) pp. 132–137 ''Funkts. Anal.'' , '''8''' : 2 (1974) pp. 50–56</TD></TR><TR><TD valign="top">[10b]</TD> <TD valign="top"> V.M. Kharlamov, "A generalized Petrovskii inequality II" ''Funct. Anal. Appl.'' , '''9''' : 3 (1975) pp. 266–268 ''Funkts. Anal.'' , '''9''' : 3 (1975) pp. 93–94</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> V.M. Kharlamov, "Additive congruences for the Euler characteristic of real algebraic manifolds of even dimensions" ''Funct. Anal. Appl.'' , '''9''' : 2 (1975) pp. 134–141 ''Funkts. Anal.'' , '''9''' : 2 (1975) pp. 51–60</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> V.M. Kharlamov, "The topological type of nonsingular surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020172.png" /> of degree four" ''Funct. Anal. Appl.'' , '''10''' : 4 (1976) pp. 295–304 ''Funkts. Anal.'' , '''10''' : 4 (1976) pp. 55–68</TD></TR><TR><TD valign="top">[13]</TD> <TD valign="top"> D.A. Gudkov, "The topology of real projective algebraic varieties" ''Russian Math. Surveys'' , '''29''' : 4 (1974) pp. 1–80 ''Uspekhi Mat. Nauk'' , '''29''' : 4 (1974) pp. 3–79</TD></TR><TR><TD valign="top">[14]</TD> <TD valign="top"> D. Sullivan, "Geometric topology" , '''I. Localization, periodicity, and Galois symmetry''' , M.I.T. (1971)</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A. Harnack, "Ueber die Vieltheitigkeit der ebenen algebraischen Kurven" ''Math. Ann.'' , '''10''' (1876) pp. 189–198</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> D. Hilbert, "Ueber die reellen Züge algebraischer Kurven" ''Math. Ann.'' , '''38''' (1891) pp. 115–138</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> D. Hilbert, "Mathematische Probleme" ''Arch. Math. Phys.'' , '''1''' (1901) pp. 213–237 {{MR|}} {{ZBL|32.0084.05}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> I.G. Petrovskii, "On the topology of real plane algebraic curves" ''Ann. of Math.'' , '''39''' : 1 (1938) pp. 189–209 {{MR|1503398}} {{ZBL|}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> O.A. Oleinik, I.G. Petrovskii, "On the topology of real algebraic surfaces" ''Transl. Amer. Math. Soc.'' , '''7''' (1952) pp. 399–417 ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''13''' (1949) pp. 389–402 {{MR|0048095}} {{ZBL|}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> O.A. Oleinik, "On the topology of real algebraic curves on an algebraic surface" ''Mat. Sb.'' , '''29''' (1951) pp. 133–156 (In Russian) {{MR|44863}} {{ZBL|}} </TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> , ''Hilbert problems'' , Moscow (1969) (In Russian) {{MR|}} {{ZBL|0187.35502}} {{ZBL|0186.18601}} {{ZBL|0181.15503}} </TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> V.I. Arnol'd, "Distribution of the ovals of the real plane of algebraic curves, of involutions of four-dimensional smooth manifolds, and the arithmetic of integer-valued quadratic forms" ''Funct. Anal. Appl.'' , '''5''' : 3 (1971) pp. 169–176 ''Funkts. Anal.'' , '''5''' : 3 (1971) pp. 1–9 {{MR|}} {{ZBL|0268.53001}} </TD></TR><TR><TD valign="top">[9a]</TD> <TD valign="top"> V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" ''Funct. Anal. Appl.'' , '''6''' : 4 (1972) pp. 301–306 ''Funkts. Anal.'' , '''6''' : 4 (1972) pp. 58–64</TD></TR><TR><TD valign="top">[9b]</TD> <TD valign="top"> V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" ''Funct. Anal. Appl.'' , '''7''' : 2 (1973) pp. 163–165 ''Funkts. Anal.'' , '''7''' : 2 (1973) pp. 91–92</TD></TR><TR><TD valign="top">[10a]</TD> <TD valign="top"> V.M. Kharlamov, "A generalized Petrovskii inequality" ''Funct. Anal. Appl.'' , '''8''' : 2 (1974) pp. 132–137 ''Funkts. Anal.'' , '''8''' : 2 (1974) pp. 50–56 {{MR|}} {{ZBL|0301.14021}} </TD></TR><TR><TD valign="top">[10b]</TD> <TD valign="top"> V.M. Kharlamov, "A generalized Petrovskii inequality II" ''Funct. Anal. Appl.'' , '''9''' : 3 (1975) pp. 266–268 ''Funkts. Anal.'' , '''9''' : 3 (1975) pp. 93–94</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> V.M. Kharlamov, "Additive congruences for the Euler characteristic of real algebraic manifolds of even dimensions" ''Funct. Anal. Appl.'' , '''9''' : 2 (1975) pp. 134–141 ''Funkts. Anal.'' , '''9''' : 2 (1975) pp. 51–60</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> V.M. Kharlamov, "The topological type of nonsingular surfaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/r/r080/r080020/r080020172.png" /> of degree four" ''Funct. Anal. Appl.'' , '''10''' : 4 (1976) pp. 295–304 ''Funkts. Anal.'' , '''10''' : 4 (1976) pp. 55–68 {{MR|}} {{ZBL|0362.14013}} </TD></TR><TR><TD valign="top">[13]</TD> <TD valign="top"> D.A. Gudkov, "The topology of real projective algebraic varieties" ''Russian Math. Surveys'' , '''29''' : 4 (1974) pp. 1–80 ''Uspekhi Mat. Nauk'' , '''29''' : 4 (1974) pp. 3–79 {{MR|0399085}} {{ZBL|0316.14018}} </TD></TR><TR><TD valign="top">[14]</TD> <TD valign="top"> D. Sullivan, "Geometric topology" , '''I. Localization, periodicity, and Galois symmetry''' , M.I.T. (1971) {{MR|0494074}} {{MR|0494075}} {{ZBL|1078.55001}} {{ZBL|0871.57021}} {{ZBL|0366.57003}} </TD></TR></table> |
| − | | |
| − | | |
| | | | |
| | ====Comments==== | | ====Comments==== |
| − |
| |
| | | | |
| | ====References==== | | ====References==== |
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> O. Viro, "Successes of the last five years in the topology of real algebraic varieties" , ''Proc. Internat. Congress Mathematicians (Warszawa, 1983)'' , PWN & North-Holland (1984) pp. 603–619</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> G. Wilson, "Hilbert's sixteenth problem" ''Topology'' , '''17''' (1978) pp. 53–74</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> O. Viro, "Successes of the last five years in the topology of real algebraic varieties" , ''Proc. Internat. Congress Mathematicians (Warszawa, 1983)'' , PWN & North-Holland (1984) pp. 603–619</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> G. Wilson, "Hilbert's sixteenth problem" ''Topology'' , '''17''' (1978) pp. 53–74</TD></TR></table> |
The set $ A = X ( \mathbf R ) $
of real points of an algebraic variety $ X $
defined over the field $ \mathbf R $
of real numbers. A real algebraic variety is said to be non-singular if $ X $
is non-singular. In such a case $ A $
is a smooth variety, and its dimension $ \mathop{\rm dim} A $
is equal to the dimension of the complex variety $ \mathbf C A = X ( \mathbf C ) $;
the latter is known as the complexification of the variety $ A $.
Non-singular regular complete intersections have been most thoroughly studied. These are varieties $ X $
in the projective space $ \mathbf R P ^ {q} $
which are non-singular regular intersections of hypersurfaces $ p _ {i} ( z) = 0 $,
$ 1 \leq i \leq s $,
where $ p _ {i} ( z) $
is a homogeneous real polynomial in $ q $
variables of degree $ m _ {i} $.
In such a case the matrix
$$
\left \|
\frac{\partial p _ {i} }{\partial z _ {j} }
\right \|
$$
has rank $ s $
at all points $ z \in \mathbf C A $;
$ \mathop{\rm dim} A = n = q- s $.
Let $ B $
denote the real algebraic variety defined as the intersection system
$$
p _ {i} ( z) = 0 ,\ 1\leq i \leq s- 1,\ p( z) = p _ {s} ( z) \ \textrm{ and } \ \
m = m _ {s} .
$$
Examples of regular complete intersections are:
1) A plane real algebraic curve; here $ q= 2 $,
$ s= 1 $,
$ \mathbf C B = \mathbf C P ^ {2} $,
$ B = \mathbf R P ^ {2} $.
2) A real algebraic hypersurface; here $ s= 1 $,
$ \mathbf C B = \mathbf C P ^ {q} $,
$ B = \mathbf R P ^ {q} $.
In particular, if $ q= 3 $,
a real algebraic surface is obtained.
3) A real algebraic space curve; here $ q= 3 $,
$ s= 2 $.
The surface $ B $
is defined by an equation $ p _ {1} ( z) = 0 $,
while the curve $ A $
is cut out on $ B $
by a surface $ p _ {2} ( z) = 0 $.
A real algebraic curve $ A $
of order $ m _ {1} $
in the plane $ \mathbf R P ^ {2} $
consists of finitely many components diffeomorphic to a circle. If $ m _ {1} $
is even, these components are all two-sidedly imbedded in $ \mathbf R P ^ {2} $;
if $ m _ {1} $
is odd, one component is imbedded one-sidedly, while the remaining ones are imbedded two-sidedly. A two-sidedly imbedded component of $ A $
is called an oval of $ A $.
An oval lying inside an odd number of other ovals of $ A $
is called odd, while the remaining ovals are even.
The number of components of a plane real algebraic curve of order $ m _ {1} $
is not larger than $ ( m _ {1} - 1 ) ( m _ {1} - 2 ) / 2 + 1 $(
Harnack's theorem) [1]. For each $ m _ {1} $
there exists a plane real algebraic curve with this largest number of components — the $ M $-
curve. (For methods of constructing $ M $-
curves see [1], [2], [3]; for a generalization of these results to include space curves, see [2].)
D. Hilbert posed in 1900 the problem of studying the topology of real algebraic varieties, and also of the imbedding of real algebraic varieties into $ \mathbf R P ^ {q} $
and of one real algebraic variety into another (Hilbert's 16th problem). He also pointed out difficult partial problems: the study of the mutual locations of the ovals of a sixth-order curve, and the topology and the imbedding of a real algebraic fourth-order surface into $ \mathbf R P ^ {3} $.
These partial problems have been solved [12], [13].
For a plane real algebraic curve $ A $
of even order $ m _ {1} $
the following exact inequality is valid:
$$
-
\frac{1}{8}
( 3 m _ {1} ^ {2} - 6 m _ {1} ) \leq P - N \leq
\frac{1}{8}
( 3 m _ {1} ^ {2} - 6 m _ {1} ) + 1 ,
$$
where $ P $
is the number of even ovals and $ N $
is the number of odd ovals of $ A $(
Petrovskii's theorem). If $ m _ {1} $
is odd, a similar inequality is valid for $ A \cup L $,
where $ L $
is a straight line in general position [4]. When these results are generalized to include the case of a real algebraic hypersurface of even order, the role of the difference $ P- N $
is played by the Euler characteristic $ \chi ( B _ {+} ) $,
where $ B _ {+} = \{ {z \in B } : {p( z) \geq 0 } \} $,
while if $ q $
is odd, the role of $ P- N $
is played by $ \chi ( A) $.
Thus, for a real algebraic hypersurface $ A $
of even order $ m _ {1} $,
$$
| \chi ( B _ {+} ) | \leq
\frac{( m _ {1} - 1) ^ {q} }{2}
- s( q; m _ {1} ) +
\frac{1}{2}
,
$$
where $ s ( q ; m _ {1)} $
is the number of terms of the polynomial
$$
\prod _ {i = 1 } ^ { q } ( 1 + x _ {i} + \dots + x _ {i} ^ {m-2} ) ,
$$
of degree not higher than $ ( qm _ {1} - 2q - m _ {1} ) / 2 $;
if $ q $
is odd, then for any $ m _ {1} $,
$$
| \chi ( A) | \leq ( m _ {1} - 1 ) ^ {q} - 2s ( q ; m _ {1} ) + 1 ,
$$
[5]. The following inequality is satisfied for a real algebraic space curve (in $ \mathbf R P ^ {3} $)
for even $ m _ {1} $:
$$
| \chi ( B _ {+} ) | \leq
\frac{1}{3}
m _ {1} ^ {3} +
\frac{3}{8}
m _ {1} m _ {2} ^ {2} +
\frac{1}{4}
m _ {1} ^ {2} m _ {2} +
$$
$$
- m _ {1} ^ {2} - m _ {1} m _ {2} +
\frac{7}{6}
m _ {1} +
\frac{| \chi ( B) | }{2}
$$
(if $ m _ {1} = 2 $,
this estimate is exact [6]). Petrovskii's theorem has been generalized to arbitrary real algebraic varieties .
For a plane real algebraic $ M $-
curve of even order $ m _ {1} $
the following congruence is valid:
$$
P - N \equiv \left (
\frac{m _ {1} }{2}
\right ) ^ {2} \mathop{\rm mod} 8 ,
$$
[8], , [13]. In proving this congruence ([8], ), real algebraic varieties were studied by methods of differential topology in a form which opened the way for further investigations. Let the plane real algebraic curve $ A $
have even order $ m = 2k $
and let the sign of $ p( z) $
be chosen so that $ B _ {+} $
is orientable, while $ P _ {+} , P _ {0} , P _ {-} $
denote, respectively, the number of ovals of $ A $
which externally bound the components of the set $ B _ {+} $
with positive, zero and negative Euler characteristics. In a similar manner, $ N _ {+} , N _ {0} , N _ {-} $
are the numbers of such odd ovals for $ B _ {-} = \{ {z \in B } : {p( z) \leq 0 } \} $.
Then [8], [3],
$$
P _ {-} + P _ {0} \leq
\frac{1}{2}
( k - 1 ) ( k - 2 ) + E ( k) ,
$$
$$
N _ {-} + N _ {0} \leq
\frac{1}{2}
( k - 1 ) ( k - 2 ) ,
$$
$$
P _ {-} \geq N -
\frac{3}{2}
k ( k - 1 ) ,
$$
$$
N _ {-} \geq P -
\frac{3}{2}
k ( k - 1 ) ,
$$
where
$$
E ( k) =
\frac{1}{2}
( 1 + ( - 1 ) ^ {k} ) .
$$
For an arbitrary real algebraic variety in a $ q $-
dimensional projective space the following inequality is valid:
$$
\mathop{\rm dim} H _ {*} ( A ; \mathbf Z _ {2} ) \leq \mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) ,
$$
where $ H _ {*} ( A; \mathbf Z _ {2} ) = \sum H _ {i} ( A; \mathbf Z _ {2} ) $
is the homology space of the variety $ A $
with coefficients in $ \mathbf Z _ {2} $.
This inequality is a generalization of Harnack's theorem. If
$$
\mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) - \mathop{\rm dim} H _ {*} ( A ; \mathbf Z _ {2} ) = 2t,
$$
where $ t $
is always an integer, $ A $
is said to be an $ ( M- t) $-
variety. If $ t= 0 $,
$ A $
is an $ M $-
variety.
The validity of the following congruences has been demonstrated:
A) For an $ M $-
variety $ A $
and for even $ n $:
$$
\chi ( A ) \equiv \sigma ( \mathbf C A ) \mathop{\rm mod} 16 ,
$$
where $ \sigma ( \mathbf C A ) $
is the signature of the variety $ \mathbf C A $.
B) For an $ ( M- 1) $-
variety $ A $
and even $ n $([13]):
$$
\chi ( A ) \equiv \sigma ( \mathbf C A ) \pm 2 \mathop{\rm mod} 16 ,
$$
cf. the overview [3].
C) For a regular complete intersection, if $ n $
is even, $ A $
is an $ ( M- 1) $-
variety and the inclusion homomorphism
$$
i _ {*} : H _ {n / 2 } ( A ; \mathbf Z _ {2} ) \rightarrow H _ {n / 2 }
( \mathbf R P ^ {q} ; \mathbf Z _ {2} )
$$
is zero, then
$$
d = m _ {1} m _ {2} \dots \equiv 2 \mathop{\rm mod} 4
$$
and
$$
\chi ( A ) \equiv - \sigma ( \mathbf C A ) +
\left \{
\begin{array}{rl}
2 \mathop{\rm mod} 16 & \textrm{ if } d \equiv 2 \mathop{\rm mod} 8 , \\
- 2 \mathop{\rm mod} 16 & \textrm{ if } d \equiv - 2 \mathop{\rm mod} 8 . \\
\end{array}
\right .$$
In this case, if $ n $
is even, $ A $
is an $ ( M- 2) $-
variety and $ i _ {*} $
is zero ([11]):
if $ d \equiv 0 $
$ \mathop{\rm mod} 8 $,
$ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $
$ \mathop{\rm mod} 16 $,
if $ d \equiv 2 $
$ \mathop{\rm mod} 8 $,
$ \chi ( A) \equiv - \sigma ( \mathbf C A ) + 4 $
$ \mathop{\rm mod} 16 $
or $ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $
$ \mathop{\rm mod} 16 $,
if $ d \begin{array}{c}
> \\
=
\end{array}
2 $
$ \mathop{\rm mod} 8 $,
$ \chi ( A) \equiv - \sigma ( \mathbf C A ) - 4 $
$ \mathop{\rm mod} 16 $
or $ \chi ( A) \equiv \pm \sigma ( \mathbf C A ) $
$ \mathop{\rm mod} 16 $.
In particular, for a real algebraic surface $ A $
of order $ m _ {1} $,
$$
\mathop{\rm dim} H _ {*} ( \mathbf C A ; \mathbf Z _ {2} ) = m _ {1} ^ {3}
- 4 m _ {1} ^ {2} + 6 m _ {1} .
$$
If $ A $
is an $ M $-
surface, then
$$
\chi ( A) \equiv
\frac{1}{3}
( 4 m _ {1} - m _ {1} ^ {3} ) \mathop{\rm mod} 16 .
$$
If $ A $
is an $ ( M- 1) $-
surface, then
$$
\chi ( A) \equiv
\frac{1}{3}
( 4 m _ {1} - m _ {1} ^ {3} ) \pm 2
\mathop{\rm mod} 16 .
$$
If $ A $
is an $ ( M- 1) $-
surface and contracts to a point in $ \mathbf R P ^ {3} $,
then $ m _ {1} \equiv 2 $
$ \mathop{\rm mod} 4 $
and
$$
\chi ( A) \equiv \left \{
\begin{array}{rl}
2 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 2 \mathop{\rm mod} 8 , \\
- 2 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv - 2 \mathop{\rm mod} 8 . \\
\end{array}
\right .
$$
If $ A $
is an $ ( M- 2) $-
surface and contracts to a point in $ \mathbf R P ^ {3} $,
then
$$
\chi ( A) \equiv \left \{
\begin{array}{rl}
0 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 0 \mathop{\rm mod} 8, \\
0 , 4 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv 2 \mathop{\rm mod} 8 , \\
0 , - 4 \mathop{\rm mod} 16 & \textrm{ if } m _ {1} \equiv - 2 \mathop{\rm mod} 8 . \\
\end{array}
\right .$$
Certain congruences have also been proved , [13] for odd $ n $.
In particular, for a plane real algebraic curve $ A $
which is an $ ( M- 1) $-
curve of even order $ m _ {1} $:
$$
P - N \equiv \left (
\frac{m _ 1}{2}
\right ) ^ {2}
\pm 1 \mathop{\rm mod} 8 .
$$
Certain results have also been obtained [13] for real algebraic varieties with singularities. For an interesting approach to the study of real algebraic varieties see [14].
References
| [1] | A. Harnack, "Ueber die Vieltheitigkeit der ebenen algebraischen Kurven" Math. Ann. , 10 (1876) pp. 189–198 |
| [2] | D. Hilbert, "Ueber die reellen Züge algebraischer Kurven" Math. Ann. , 38 (1891) pp. 115–138 |
| [3] | D. Hilbert, "Mathematische Probleme" Arch. Math. Phys. , 1 (1901) pp. 213–237 Zbl 32.0084.05 |
| [4] | I.G. Petrovskii, "On the topology of real plane algebraic curves" Ann. of Math. , 39 : 1 (1938) pp. 189–209 MR1503398 |
| [5] | O.A. Oleinik, I.G. Petrovskii, "On the topology of real algebraic surfaces" Transl. Amer. Math. Soc. , 7 (1952) pp. 399–417 Izv. Akad. Nauk SSSR Ser. Mat. , 13 (1949) pp. 389–402 MR0048095 |
| [6] | O.A. Oleinik, "On the topology of real algebraic curves on an algebraic surface" Mat. Sb. , 29 (1951) pp. 133–156 (In Russian) MR44863 |
| [7] | , Hilbert problems , Moscow (1969) (In Russian) Zbl 0187.35502 Zbl 0186.18601 Zbl 0181.15503 |
| [8] | V.I. Arnol'd, "Distribution of the ovals of the real plane of algebraic curves, of involutions of four-dimensional smooth manifolds, and the arithmetic of integer-valued quadratic forms" Funct. Anal. Appl. , 5 : 3 (1971) pp. 169–176 Funkts. Anal. , 5 : 3 (1971) pp. 1–9 Zbl 0268.53001 |
| [9a] | V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" Funct. Anal. Appl. , 6 : 4 (1972) pp. 301–306 Funkts. Anal. , 6 : 4 (1972) pp. 58–64 |
| [9b] | V.A. Rokhlin, "Congruences modulo 16 in Hilbert's sixteenth problem" Funct. Anal. Appl. , 7 : 2 (1973) pp. 163–165 Funkts. Anal. , 7 : 2 (1973) pp. 91–92 |
| [10a] | V.M. Kharlamov, "A generalized Petrovskii inequality" Funct. Anal. Appl. , 8 : 2 (1974) pp. 132–137 Funkts. Anal. , 8 : 2 (1974) pp. 50–56 Zbl 0301.14021 |
| [10b] | V.M. Kharlamov, "A generalized Petrovskii inequality II" Funct. Anal. Appl. , 9 : 3 (1975) pp. 266–268 Funkts. Anal. , 9 : 3 (1975) pp. 93–94 |
| [11] | V.M. Kharlamov, "Additive congruences for the Euler characteristic of real algebraic manifolds of even dimensions" Funct. Anal. Appl. , 9 : 2 (1975) pp. 134–141 Funkts. Anal. , 9 : 2 (1975) pp. 51–60 |
| [12] | V.M. Kharlamov, "The topological type of nonsingular surfaces in 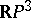 of degree four" Funct. Anal. Appl. , 10 : 4 (1976) pp. 295–304 Funkts. Anal. , 10 : 4 (1976) pp. 55–68 Zbl 0362.14013 of degree four" Funct. Anal. Appl. , 10 : 4 (1976) pp. 295–304 Funkts. Anal. , 10 : 4 (1976) pp. 55–68 Zbl 0362.14013 |
| [13] | D.A. Gudkov, "The topology of real projective algebraic varieties" Russian Math. Surveys , 29 : 4 (1974) pp. 1–80 Uspekhi Mat. Nauk , 29 : 4 (1974) pp. 3–79 MR0399085 Zbl 0316.14018 |
| [14] | D. Sullivan, "Geometric topology" , I. Localization, periodicity, and Galois symmetry , M.I.T. (1971) MR0494074 MR0494075 Zbl 1078.55001 Zbl 0871.57021 Zbl 0366.57003 |
References
| [a1] | O. Viro, "Successes of the last five years in the topology of real algebraic varieties" , Proc. Internat. Congress Mathematicians (Warszawa, 1983) , PWN & North-Holland (1984) pp. 603–619 |
| [a2] | G. Wilson, "Hilbert's sixteenth problem" Topology , 17 (1978) pp. 53–74 |
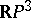 of degree four" Funct. Anal. Appl. , 10 : 4 (1976) pp. 295–304 Funkts. Anal. , 10 : 4 (1976) pp. 55–68 Zbl 0362.14013
of degree four" Funct. Anal. Appl. , 10 : 4 (1976) pp. 295–304 Funkts. Anal. , 10 : 4 (1976) pp. 55–68 Zbl 0362.14013