Inverse hyperbolic functions
Functions inverse to the hyperbolic functions. The inverse hyperbolic functions are the inverse hyperbolic sine, cosine and tangent: ,
\cosh ^ {-1} x ,
\mathop{\rm tanh} ^ {-1} x ;
other notations are: { \mathop{\rm arg} \sinh } x ,
{ \mathop{\rm arg} \cosh } x ,
{ \mathop{\rm arg} \mathop{\rm tanh} } x .
The inverse hyperbolic functions of a real variable x are defined by the formulas
\sinh ^ {-1} x = \ \mathop{\rm ln} ( x + \sqrt {x ^ {2} + 1 } ) ,\ \ - \infty < x < + \infty ,
\cosh ^ {-1} x = \ \pm \mathop{\rm ln} ( x + \sqrt {x ^ {2} - 1 } ) ,\ \ x \geq 1 ,
\mathop{\rm tanh} ^ {-1} x = \frac{1}{2} \mathop{\rm ln} \frac{1 + x }{1 - x } ,\ | x | < 1 .
The inverse hyperbolic functions are single-valued and continuous at each point of their domain of definition, except for \cosh ^ {-1} x , which is two-valued. In studying the properties of the inverse hyperbolic functions, one of the continuous branches of \cosh ^ {-1} x is chosen, that is, in the formula above only one sign is taken (usually plus). For the graphs of these functions see the figure.
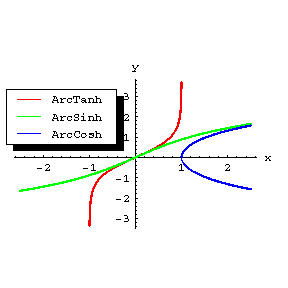
Figure: i052370a
There a number of relations between the inverse hyperbolic functions. For example,
\sinh ^ {-1} x = \ \mathop{\rm tanh} ^ {-1} \ \frac{x}{\sqrt {x ^ {2} + 1 } } ,\ \ \mathop{\rm tanh} ^ {-1} x = \ \sinh ^ {-1} \ \frac{x}{\sqrt {1 - x ^ {2} } } .
The derivatives of the inverse hyperbolic functions are given by the formulas
( \sinh ^ {-1} x ) ^ \prime = \ \frac{1}{\sqrt {x ^ {2} + 1 } } ,\ \ ( \cosh ^ {-1} x ) ^ \prime = \pm \frac{1}{\sqrt {x ^ {2} - 1 } } ,
( \mathop{\rm tanh} ^ {-1} x ) ^ \prime = \frac{1}{ {1 - x ^ {2} } } .
The inverse hyperbolic functions of a complex variable z are defined by the same formulas as those for a real variable x , where \mathop{\rm ln} z is understood to be the many-valued logarithmic function. The inverse hyperbolic functions of a complex variable are the analytic continuations to the complex plane of the corresponding functions of a real variable.
The inverse hyperbolic functions can be expressed in terms of the inverse trigonometric functions by the formulas
\sinh ^ {-1} z = - i { \mathop{\rm arc} \sin } i z ,
\cosh ^ {-1} z = i { \mathop{\rm arc} \cos } z ,
\mathop{\rm tanh} ^ {-1} z = - i { \mathop{\rm arc} \mathop{\rm tan} } i z .
Comments
The notations { \mathop{\rm arc} \sinh } x , { \mathop{\rm arc} \cosh } x and { \mathop{\rm arc} \mathop{\rm tanh} } x are also quite common.
References
| [a1] | M.R. Spiegel, "Complex variables" , Schaum's Outline Series , McGraw-Hill (1974) |
| [a2] | M. Abramowitz, I.A. Stegun, "Handbook of mathematical functions" , Dover, reprint (1972) |
Inverse hyperbolic functions. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Inverse_hyperbolic_functions&oldid=55169