Tetrahedron
From Encyclopedia of Mathematics
The convex hull of a set of four points, not all in one plane. In particular, a regular tetrahedron is one of the five types of Platonic polyhedra (cf. Platonic solids). A tetrahedron has 4 (triangular) faces, 6 edges and 4 vertices (at each of which 3 edges meet). If $a$ is the length of an edge of the regular tetrahedron, then its volume is
$$\frac{a^3\sqrt2}{12}\approx0.1179a^3.$$
A tetrahedron is a triangular pyramid.
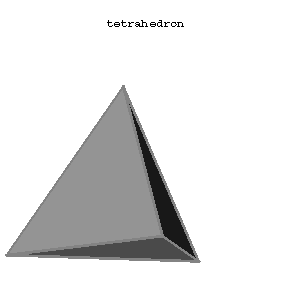
Figure: t092510a
Comments
A solid tetrahedron is also called a $3$-simplex. The Schläfli symbol of a tetrahedron is $\{3,3\}$.
References
| [a1] | H.S.M. Coxeter, "Introduction to geometry" , Wiley (1969) pp. 149; 185 |
| [a2] | H. Senechal (ed.) G. Fleck (ed.) , Shaping space , Birkhäuser (1988) pp. 5; 7; 100; 133; 175 |
How to Cite This Entry:
Tetrahedron. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tetrahedron&oldid=31505
Tetrahedron. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tetrahedron&oldid=31505
This article was adapted from an original article by BSE-3 (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article