Riemann tensor
Riemann curvature tensor
A four-valent tensor that is studied in the theory of curvature of spaces. Let 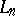 be a space with an affine connection and let
be a space with an affine connection and let  be the Christoffel symbols (cf. Christoffel symbol) of the connection of
be the Christoffel symbols (cf. Christoffel symbol) of the connection of  . The components (coordinates) of the Riemann tensor, which is once contravariant and three times covariant, take the form
. The components (coordinates) of the Riemann tensor, which is once contravariant and three times covariant, take the form
 |
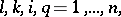 |
where 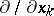 is the symbol of differentiation with respect to the space coordinate
is the symbol of differentiation with respect to the space coordinate  ,
,  . In a Riemannian space
. In a Riemannian space  with a metric tensor
with a metric tensor 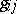 , in addition to the tensor
, in addition to the tensor 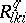 the four times covariant Riemann tensor obtained by lowering the upper index
the four times covariant Riemann tensor obtained by lowering the upper index  using the metric tensor
using the metric tensor 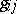 is also studied
is also studied
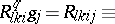 |
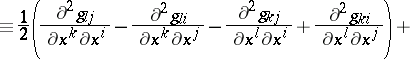 |
 |
Here 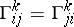 since the Riemannian connection (without torsion) is considered on
since the Riemannian connection (without torsion) is considered on  . In an arbitrary space with an affine connection without torsion the coordinates of the Riemann tensor satisfy the first Bianchi identity
. In an arbitrary space with an affine connection without torsion the coordinates of the Riemann tensor satisfy the first Bianchi identity
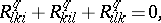 |
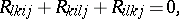 |
i.e. the cyclic sum with respect to the first three subscripts is zero.
The Riemann tensor possesses the following properties:
1)  ;
;
2)  ;
;
3)  ,
,  ;
;
4) 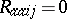 ,
,  , if both subscripts of one pair are identical, then the corresponding coordinate equals zero:
, if both subscripts of one pair are identical, then the corresponding coordinate equals zero: 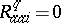 ;
;
5) the second Bianchi identity is applicable to the absolute derivatives of the Riemann tensor:
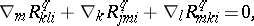 |
where  is the symbol for covariant differentiation in the direction of the coordinate
is the symbol for covariant differentiation in the direction of the coordinate 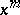 . The same identity is applicable to the tensor
. The same identity is applicable to the tensor 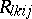 .
.
A Riemann tensor has, in all, 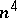 coordinates,
coordinates,  being the dimension of the space, among which
being the dimension of the space, among which 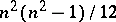 are essential. Between the latter no additional dependencies result from the properties listed above.
are essential. Between the latter no additional dependencies result from the properties listed above.
When 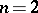 the Riemann tensor has one essential coordinate,
the Riemann tensor has one essential coordinate, 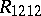 ; it forms part of the definition of the intrinsic, or Riemannian, curvature of the surface:
; it forms part of the definition of the intrinsic, or Riemannian, curvature of the surface: 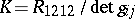 (see Gaussian curvature).
(see Gaussian curvature).
The Riemann tensor was defined by B. Riemann in 1861 (published in 1876).
References
| [1] | P.K. [P.K. Rashevskii] Rashewski, "Riemannsche Geometrie und Tensoranalyse" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian) |
| [2] | L.P. Eisenhart, "Riemannian geometry" , Princeton Univ. Press (1949) |
| [3] | D. Gromoll, W. Klingenberg, W. Meyer, "Riemannsche Geometrie im Grossen" , Springer (1968) |
Comments
References
| [a1] | S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1969) |
| [a2] | N.J. Hicks, "Notes on differential geometry" , v. Nostrand (1965) |
| [a3] | J.A. Schouten, D.J. Struik, "Einführung in die neueren Methoden der Differentialgeometrie" , 2 , Noordhoff (1924) |
| [a4] | M. Spivak, "A comprehensive introduction to differential geometry" , 1979 , Publish or Perish pp. 1–5 |
| [a5] | W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German) |
| [a6] | L.P. Eisenhart, "An introduction to differential geometry with the use of the tensor calculus" , Princeton Univ. Press (1947) |
| [a7] | J.A. Schouten, "Ricci-calculus. An introduction to tensor analysis and its geometrical applications" , Springer (1954) (Translated from German) |
Riemann tensor. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemann_tensor&oldid=15143