Quantum field theory
The theory of relativistic quantum systems. The origins of quantum field theory are connected with problems of the interaction of matter with radiation and with the attempts to construct a relativistic quantum mechanics (P.A.M. Dirac (1927), W. Heisenberg, W. Pauli, and others). At relativistic (that is, high) energies there cannot be a consistent quantum mechanics of particles, since a relativistic quantum particle is capable of producing new (similar or different) particles and of vanishing itself. As a result one cannot distinguish a definite number of mechanical degrees of freedom connected with this particle, but one has to talk about a system with a variable, generally infinite, number of degrees of freedom. Quantum field theory unifies the description of fields and particles, which in classical physics appear as two distinct entities.
The notion of a quantum field plays a central role in the theory. It is convenient to explain how it is introduced by the example of an electromagnetic field, since this is the only field having a clear content both in the classical and the quantum case.
A classical electromagnetic field satisfies the Maxwell equations. These equations can be rewritten in the form of the Hamilton canonical equations, so that the field potential plays the role of coordinate while its derivative with respect to time is the momentum in the corresponding phase space. The field is represented as a canonical system with an infinite number of degrees of freedom since the potential at each point is an independent coordinate. This system can be quantized in the same way as an ordinary mechanical system. In the quantum situation, the basic concepts are the states, described by vectors in Hilbert space, and the observables, described by self-adjoint operators (cf. Self-adjoint operator) acting on this space. Quantization consists in replacing the canonical coordinates and momenta by operators so that the Poisson brackets are replaced by commutators of the corresponding operators. A quantum field becomes an operator acting on state vectors, and it brings about a transition between states with different numbers of quantum particles (photons), that is, the operator describes the creation and destruction (radiation and absorption) of the quanta of the field.
In a similar way one can associate a quantum field with fundamental particles of any other sort. The equations for the operator of a free field are obtained from the fundamental requirement of relativity theory, namely the conditions of invariance with respect to the Poincaré group. The type of particles is characterized by the rest mass 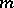 , the spin, that is, the intrinsic angular momentum
, the spin, that is, the intrinsic angular momentum  , which can take integer or half-integer values including zero, and the various charges (electric charge, baryon number, lepton number, etc.). The first two numbers
, which can take integer or half-integer values including zero, and the various charges (electric charge, baryon number, lepton number, etc.). The first two numbers  define an irreducible representation of the Poincaré group by which the field is transformed and therefore the equations of the field are transformed as well.
define an irreducible representation of the Poincaré group by which the field is transformed and therefore the equations of the field are transformed as well.
A free classical scalar field 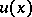 is subject to the equation
is subject to the equation 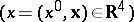
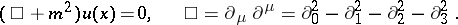 | (1) |
This is the variational Euler equation for the action functional
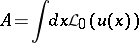 |
with Lagrangian density
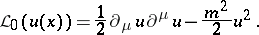 |
If one regards 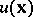 ,
, 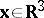 , as a canonical coordinate, then the conjugate momentum is
, as a canonical coordinate, then the conjugate momentum is 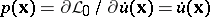 (the dot denotes differentiation with respect to time). The quantization is carried out by associating the functions
(the dot denotes differentiation with respect to time). The quantization is carried out by associating the functions  with the operator-valued functions
with the operator-valued functions 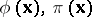 , which satisfy the commutation relations (the quantum Poisson brackets)
, which satisfy the commutation relations (the quantum Poisson brackets)
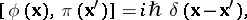 |
where 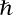 is the Planck constant (below
is the Planck constant (below 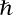 is set equal to 1). The Hamilton operator has the form
is set equal to 1). The Hamilton operator has the form
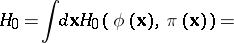 |
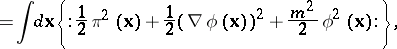 |
that is,  is the same function of the quantum operators
is the same function of the quantum operators 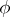 and
and 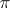 as the classical Hamiltonian, apart from the order of (non-commuting) operators. The symbol
as the classical Hamiltonian, apart from the order of (non-commuting) operators. The symbol  of the normal product makes this order precise. The Hamiltonian equations of motion
of the normal product makes this order precise. The Hamiltonian equations of motion
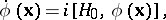 |
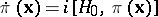 |
are equivalent to the equation
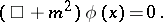 | (2) |
The quantum field in four-dimensional space-time is necessarily a generalized, rather than an ordinary, operator-valued function; therefore 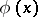 must be understood as a symbolic description only.
must be understood as a symbolic description only.
A mathematical meaning is attached to these symbols in the formulation of Fock spaces (cf. Fock space), which represent a realization of the state space in quantum field theory. The state of a particle at a given moment of time is described by a complex square-integrable function 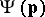 with respect to the relativistically-invariant measure
with respect to the relativistically-invariant measure
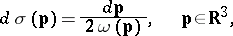 |
where 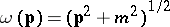 is the relativistic energy of a particle with mass
is the relativistic energy of a particle with mass  . These functions form a Hilbert space
. These functions form a Hilbert space 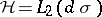 . A system of
. A system of  identical particles is described by a square-integrable function
identical particles is described by a square-integrable function 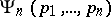 that is symmetric (for bosons, i.e. particles with integral spin) or anti-symmetric (for fermions, i.e. particles with half-integral spin) with respect to permutation of any two coordinates. These functions (for bosons) belong to the Hilbert space
that is symmetric (for bosons, i.e. particles with integral spin) or anti-symmetric (for fermions, i.e. particles with half-integral spin) with respect to permutation of any two coordinates. These functions (for bosons) belong to the Hilbert space 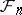 , which is the symmetric tensor product of
, which is the symmetric tensor product of  copies of
copies of  . One introduces, for the description of systems with a variable number of particles, the direct sum of the spaces
. One introduces, for the description of systems with a variable number of particles, the direct sum of the spaces  , namely the Fock space
, namely the Fock space 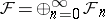 ,
,  . The vector
. The vector 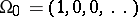 is called the vacuum and is interpreted as the state of a system without particles. Vectors of the form
is called the vacuum and is interpreted as the state of a system without particles. Vectors of the form  are called partial vectors and are identified with
are called partial vectors and are identified with 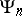 . It is convenient to regard the functions
. It is convenient to regard the functions 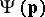 of
of 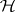 as functions of the
as functions of the 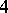 -vector
-vector 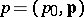 , where
, where  . The representation of the Poincaré group
. The representation of the Poincaré group 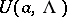 is then given by the formula
is then given by the formula
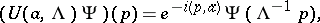 |
where  is a Lorentz-invariant (cf. Lorentz transformation) bilinear form. The representation defined in
is a Lorentz-invariant (cf. Lorentz transformation) bilinear form. The representation defined in  naturally induces a representation in the whole of the Fock space
naturally induces a representation in the whole of the Fock space  . The generator of the shifts along the
. The generator of the shifts along the  -axis is the same as the Hamiltonian
-axis is the same as the Hamiltonian 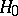 . Here the simplest representation of the Poincaré group, corresponding to spin
. Here the simplest representation of the Poincaré group, corresponding to spin 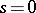 , has been described.
, has been described.
The various operators on the Fock space are expressed in terms of creation operators and annihilation operators. Let  be a single-particle wave function (that is,
be a single-particle wave function (that is, 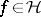 ). Then the annihilation operator
). Then the annihilation operator  is defined by the formula
is defined by the formula
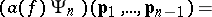 |
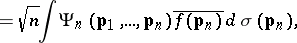 |
and the creation operator 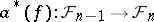 is its adjoint. In particular,
is its adjoint. In particular, 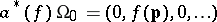 , that is, the operator
, that is, the operator  produces from the vacuum a particle with wave function
produces from the vacuum a particle with wave function  , while
, while 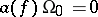 . The creation and annihilation operators are usually written in the symbolic form
. The creation and annihilation operators are usually written in the symbolic form
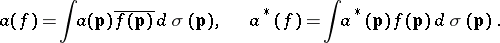 |
The Fourier transform of the sum of the creation and annihilation operators 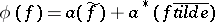 is a symmetric operator for real
is a symmetric operator for real 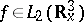 and is called the free (scalar) quantum field at time zero. The quantum field at time
and is called the free (scalar) quantum field at time zero. The quantum field at time 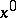 has the form
has the form
 |
and as an operator-valued generalized function 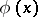 satisfies on its domain of definition equation (2) and the canonical commutation relations
satisfies on its domain of definition equation (2) and the canonical commutation relations
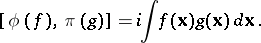 | (3) |
Thus, in the Fock space the canonical quantization described above is realized.
The theory of a free quantum field can be set forth with mathematical rigour and consistency. For interacting fields the situation is different. Although in quantum field theory there have indeed been a number of important results on problems admitting a correct mathematical formulation, the main problem of the foundation of the theory of interacting fields so far remains unsolved: No non-trivial example has been constructed in four space-time dimensions that satisfies all physical requirements. Concrete physical computations rely on heuristic schemes of quantum field theory at the basis of which there lies, in the majority of cases, some perturbation theory [5], [21]. The equation for an interacting field has a non-linear term 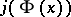 :
:
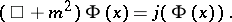 | (4) |
This equation, as well as (1), can be obtained as a variational equation for 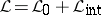 , where
, where
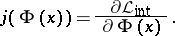 |
The Lagrangian of the interaction 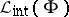 is chosen in the form of a non-linear invariant combination of the fields participating in the interaction and their derivatives. In the simplest case of a scalar field interacting with itself,
is chosen in the form of a non-linear invariant combination of the fields participating in the interaction and their derivatives. In the simplest case of a scalar field interacting with itself,  . When
. When 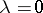 one obtains a free field. The interaction of a quantum field
one obtains a free field. The interaction of a quantum field  can be explicitly expressed in terms of the initial data
can be explicitly expressed in terms of the initial data 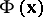 and
and  by the formula
by the formula
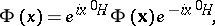 |
where, however, the exponent now contains the complete Hamiltonian
 |
By choosing as the initial data the values of the free field  and
and 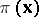 , one can express the solution of the non-linear equation (4) in terms of the creation operators
, one can express the solution of the non-linear equation (4) in terms of the creation operators 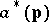 and annihilation operators
and annihilation operators 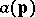 of the free particles.
of the free particles.
In this scheme it is necessary to introduce into the discussion non-interacting particles and to consider interaction as an extra which in the subsequent discussion one has to "include" and "exclude" by means of a special adiabatic procedure (see [5]). At the same time, for these interactions one can alter the spectrum and other characteristics of the fields under consideration quite considerably. In the standard method, the field 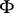 is taken to correspond to those particles that appear in the linearized equation. Only for a fairly weak interaction of type
is taken to correspond to those particles that appear in the linearized equation. Only for a fairly weak interaction of type 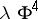 can it be proved that this is the case. However, in perturbation theory the spectrum is generally altered. The eigen vector
can it be proved that this is the case. However, in perturbation theory the spectrum is generally altered. The eigen vector  corresponding to the minimum eigen value of
corresponding to the minimum eigen value of  (more precisely, one ought to talk about the mass operator) is called the renormalized vacuum and is interpreted as the state without particles. The eigen values corresponding to the remaining points of the discrete spectrum are called renormalized single-particle states. It is different from the state
(more precisely, one ought to talk about the mass operator) is called the renormalized vacuum and is interpreted as the state without particles. The eigen values corresponding to the remaining points of the discrete spectrum are called renormalized single-particle states. It is different from the state  and has mass
and has mass 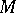 that differs from the mass
that differs from the mass 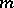 .
.
A direct application of perturbation theory gives meaningless divergent expressions (the so-called "ultraviolet divergences" ). If regularization of these divergences is carried out in accordance with physical principles (the main condition proving to be relativistic and gauge invariance of the whole procedure), then all the arbitrariness (in electrodynamics) leads to a formally-infinite renormalization of the mass and charge of the electron, as was proved in 1948 by J. Schwinger, R.P. Feynman and F.G. Dyson.
A final and rigorously mathematical analysis of quantum field theory within the framework of perturbation theory was given by N.N. Bogolyubov (1951–1955). He pointed out that ultraviolet divergences in perturbation theory emerge as the result of multiplying generalized functions at points where their supports intersect, and formulated a method of multiplying them in which the physical requirements of relativistic invariance, causality, etc., are fulfilled. In practical calculation, perturbation theory is not applied directly to equation (4) but rather to various other objects which can be obtained from it, for example the 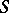 -matrix (see Scattering matrix) or the Green functions (cf. Green function). The calculation of the
-matrix (see Scattering matrix) or the Green functions (cf. Green function). The calculation of the 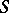 -matrix is one of the main problems in elementary particle physics. Its matrix entries have a simple expression in terms of the Green function of the field
-matrix is one of the main problems in elementary particle physics. Its matrix entries have a simple expression in terms of the Green function of the field  :
:
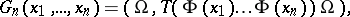 |
where  is the symbol of chronological ordering:
is the symbol of chronological ordering:
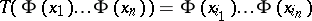 |
if
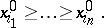 |
By expanding  in a power series in
in a power series in 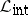 and using, for example, the representation for
and using, for example, the representation for 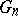 in the form of a functional integral,
in the form of a functional integral,
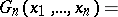 | (5) |
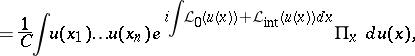 |
one can express it to each order of perturbation in terms of integrals of products of the simplest Green functions of a free field,
 |
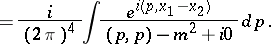 |
For calculations in terms of perturbation theory the technique of Feynman diagrams has been developed. Here arise products of Green functions 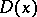 at coincident points. For example, the function
at coincident points. For example, the function  is for the model
is for the model  proportional to the expression
proportional to the expression
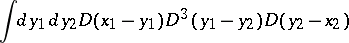 | (6) |
up to second order in  . This integral diverges, but it can be regularized, that is, a sensible meaning can be given to it. First of all one has to attach a meaning to the product of the generalized functions
. This integral diverges, but it can be regularized, that is, a sensible meaning can be given to it. First of all one has to attach a meaning to the product of the generalized functions 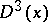 . This can be achieved as follows. Consider the smoothing function
. This can be achieved as follows. Consider the smoothing function
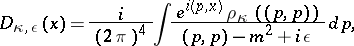 |
where  and
and  is a function such that
is a function such that 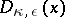 is sufficiently smooth, for example,
is sufficiently smooth, for example, 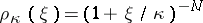 ,
, 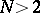 , and
, and 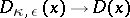 as
as 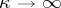 ,
, 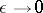 (
(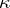 is called the ultraviolet cut-off). It can be assumed that the Lagrangian
is called the ultraviolet cut-off). It can be assumed that the Lagrangian 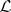 in (5) may be replaced by
in (5) may be replaced by
 |
There exist constants  and
and 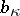 (growing unboundedly as
(growing unboundedly as 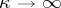 ) such that the limit
) such that the limit
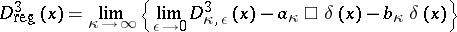 |
exists in the sense of generalized functions. The function  is a regularization of
is a regularization of 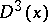 . It is defined up to terms
. It is defined up to terms 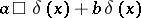 , where
, where  and
and 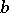 are arbitrary constants. The whole expression (6) is regularized in the same way and no new arbitrariness appears. Similarly all the Green functions in each order in
are arbitrary constants. The whole expression (6) is regularized in the same way and no new arbitrariness appears. Similarly all the Green functions in each order in 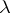 of perturbation are regularized. A similar procedure can be carried out for
of perturbation are regularized. A similar procedure can be carried out for  , like for any polynomial. For
, like for any polynomial. For 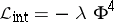 a regularization procedure can be carried out in a self-consistent way in all orders in
a regularization procedure can be carried out in a self-consistent way in all orders in 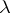 of perturbation in the following sense. There exist constants
of perturbation in the following sense. There exist constants  (represented as power series in
(represented as power series in 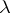 of degree 2 or higher; simple rules for calculating them have been developed) such that on replacing the Lagrangian
of degree 2 or higher; simple rules for calculating them have been developed) such that on replacing the Lagrangian 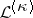 in (5) by the renormalized Lagrangian
in (5) by the renormalized Lagrangian
 |
one obtains finite expressions at each order of the power series expansion in 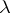 as
as  .
. 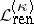 is called the renormalized Lagrangian, and one says that divergent counter-terms are added to the original
is called the renormalized Lagrangian, and one says that divergent counter-terms are added to the original 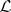 . These counter-terms have the same structure as the original Lagrangian (that is, they are linear combinations of the expressions
. These counter-terms have the same structure as the original Lagrangian (that is, they are linear combinations of the expressions 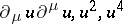 ). As a result, the quantities
). As a result, the quantities 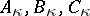 can be fixed if one regards the mass and charge of the system as given. (The mass is defined as the value of
can be fixed if one regards the mass and charge of the system as given. (The mass is defined as the value of 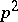 for which
for which 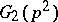 has a pole, and the charge as the value of
has a pole, and the charge as the value of 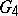 at some specific point.) The analysis of the non-uniqueness leads to the important concept of the renormalization group [5].
at some specific point.) The analysis of the non-uniqueness leads to the important concept of the renormalization group [5].
If in the given model it is possible to remove the ultraviolet divergences by adding counter-terms of the same structure as the original Lagrangian, then such a model is called renormalizable, otherwise non-renormalizable. All models of a scalar field in which the interaction is a polynomial of degree exceeding 4 are non-renormalizable. This relates only to perturbation theory. The consistent formulation of the above method of isolating "infinities" that arise from the multiplication of singular generalized functions to all orders in perturbation theory is the content of the so-called theorem on the "R-operation" , obtained by Bogolyubov and O.S. Parasyuk (1956) [5], [19]. In this way a theorem on the existence of quantum fields to all orders in perturbation theory has been proved. Subsequent investigations have shown that a number of perturbation theories are at best asymptotic, and estimates based on it are applicable only when particles of not too high energy participate in the process. The removal of ultraviolet divergences has been successfully carried out only within the framework of perturbation theory.
All the above relates to real four-dimensional space-time. In three-dimensional space-time the interaction becomes less singular and it is possible to restrict oneself to adding a counter-term 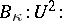 , where
, where  is of second order in
is of second order in 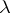 . In two-dimensional space-time counter-terms are not necessary.
. In two-dimensional space-time counter-terms are not necessary.
Here only the simplest model of a scalar field with interaction  has been described. In quantum field theory one has to deal with more complicated multi-component systems of interacting Fermi and Bose fields. For example, the chiral field takes, even at the classical level, values in certain homogeneous spaces that are not necessarily linear (for example, the sphere); the gauge fields are connections in certain vector bundles; these are related to the electromagnetic field, the gravitational field and the Yang–Mills field (see [11], [15]).
has been described. In quantum field theory one has to deal with more complicated multi-component systems of interacting Fermi and Bose fields. For example, the chiral field takes, even at the classical level, values in certain homogeneous spaces that are not necessarily linear (for example, the sphere); the gauge fields are connections in certain vector bundles; these are related to the electromagnetic field, the gravitational field and the Yang–Mills field (see [11], [15]).
The perturbation method is not applicable in those cases when the interaction constant, the basic parameter of the expansion, is known to be greater than 1, as for the strong nuclear interactions of mesons and nucleons. For strong interactions other methods are used in which a significant role is played by considering the  -matrix in the large and studying the general properties of its matrix entries that directly describe the quantities of experimental interest, which are the amplitudes of creation and scattering processes. In this connection, quantum fields (or currents) that can be expressed in terms of the
-matrix in the large and studying the general properties of its matrix entries that directly describe the quantities of experimental interest, which are the amplitudes of creation and scattering processes. In this connection, quantum fields (or currents) that can be expressed in terms of the 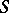 -matrix play an important role, since the central condition of causality is imposed on the
-matrix play an important role, since the central condition of causality is imposed on the  -matrix (Bogolyubov, 1955), as a restriction on the support of specific matrix entries of the
-matrix (Bogolyubov, 1955), as a restriction on the support of specific matrix entries of the 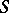 -matrix. Together with other physical requirements, such as relativistic invariance and the character of the energy spectrum, the causality condition enables one to establish holomorphy properties of generalized functions, in particular the scattering amplitude, as functions of several complex variables. These analytic properties prove to be sufficient for the deduction of special types of integral representations for the scattering amplitude, namely the "dispersion relations81U30dispersion relations" . A rigorous proof of the dispersion relations [4] required the development of special mathematical methods lying at the junction of the theory of generalized functions and the theory of functions of several complex variables and, in particular, the proof of the edge-of-the-wedge theorem of Bogolyubov and the
-matrix. Together with other physical requirements, such as relativistic invariance and the character of the energy spectrum, the causality condition enables one to establish holomorphy properties of generalized functions, in particular the scattering amplitude, as functions of several complex variables. These analytic properties prove to be sufficient for the deduction of special types of integral representations for the scattering amplitude, namely the "dispersion relations81U30dispersion relations" . A rigorous proof of the dispersion relations [4] required the development of special mathematical methods lying at the junction of the theory of generalized functions and the theory of functions of several complex variables and, in particular, the proof of the edge-of-the-wedge theorem of Bogolyubov and the  -convex hull theorem of V.S. Vladimirov [8]. Dispersion relations have become the basis of many of the concrete methods for calculation in the theory of strong interactions (A.A. Logunov, S. Mandelstam and others). They touch upon so-called axiomatic field theory, in which the compatibility of the axioms and their corollaries touching on questions of existence and properties of fields are explained.
-convex hull theorem of V.S. Vladimirov [8]. Dispersion relations have become the basis of many of the concrete methods for calculation in the theory of strong interactions (A.A. Logunov, S. Mandelstam and others). They touch upon so-called axiomatic field theory, in which the compatibility of the axioms and their corollaries touching on questions of existence and properties of fields are explained.
The method of perturbation theory presupposes that for the equation (4), of which the quantum field  is a solution, one selects the simplest initial data
is a solution, one selects the simplest initial data 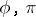 , defined in the Fock space of free particles. There exist many unitarily-inequivalent realizations (representations) of the commutation relations (3); here there is a difference between quantum field theory as a system with an infinite number of degrees of freedom and quantum mechanics, in which there is the von Neumann theorem on the uniqueness of these representations. For physics, any realization of the initial data for which equation (4) has a solution
, defined in the Fock space of free particles. There exist many unitarily-inequivalent realizations (representations) of the commutation relations (3); here there is a difference between quantum field theory as a system with an infinite number of degrees of freedom and quantum mechanics, in which there is the von Neumann theorem on the uniqueness of these representations. For physics, any realization of the initial data for which equation (4) has a solution  and for which the Hamiltonian
and for which the Hamiltonian  is bounded below is of interest. The Hilbert space in which such a representation is realized is called a sector. Some models can appear in several phases (a phase is a sector with a unique vacuum), for example, the
is bounded below is of interest. The Hilbert space in which such a representation is realized is called a sector. Some models can appear in several phases (a phase is a sector with a unique vacuum), for example, the  -model for sufficiently large
-model for sufficiently large 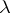 has two phases, arising from phase transition. In the construction of phases the method of quasi-averages (cf. Quasi-averages, method of) is applied. The representation of phase transition and, related to it, the phenomenon of spontaneous symmetry breakdown has played an important role, in particular in the unified theory of weak and electromagnetic interactions. It was proved in 1974 that in certain two-dimensional models there are so-called soliton sectors in which a vacuum is absent but which have a rich spectrum (L.D. Faddeev and others).
has two phases, arising from phase transition. In the construction of phases the method of quasi-averages (cf. Quasi-averages, method of) is applied. The representation of phase transition and, related to it, the phenomenon of spontaneous symmetry breakdown has played an important role, in particular in the unified theory of weak and electromagnetic interactions. It was proved in 1974 that in certain two-dimensional models there are so-called soliton sectors in which a vacuum is absent but which have a rich spectrum (L.D. Faddeev and others).
In quantum field theory there are many problems requiring for their solution methods in various areas of mathematics that are being intensively studied. Conventionally, they are divided into the following groups.
1) The analysis of the axioms and their corollaries for quantum fields and the 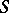 -matrix. Besides the tools of functional analysis, such as the theory of self-adjoint operators, generalized functions and group representations, one here uses methods of the theory of functions of several complex variables,
-matrix. Besides the tools of functional analysis, such as the theory of self-adjoint operators, generalized functions and group representations, one here uses methods of the theory of functions of several complex variables, 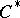 -algebras and von Neumann algebras, and, more recently, methods of probability theory. The fundamental quantities, the Green functions or the Wightman functions
-algebras and von Neumann algebras, and, more recently, methods of probability theory. The fundamental quantities, the Green functions or the Wightman functions 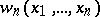 , are boundary values of functions that are holomorphic in certain domains
, are boundary values of functions that are holomorphic in certain domains 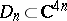 . The unsolved problem here is the construction of envelopes of holomorphy
. The unsolved problem here is the construction of envelopes of holomorphy  . The domain
. The domain 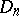 contains Euclidean points of the form
contains Euclidean points of the form  . For a number of models in two- and three-dimensional space-time it has been proved that the values of the Wightman functions at these points are moments of a probability measure. The further analysis of this Euclidean approach to quantum field theory from the point of view of probability theory is worthy of attention.
. For a number of models in two- and three-dimensional space-time it has been proved that the values of the Wightman functions at these points are moments of a probability measure. The further analysis of this Euclidean approach to quantum field theory from the point of view of probability theory is worthy of attention.
There is a general algebraic approach to field theory, at the basis of which are the algebras of observables, that is,  -algebras or von Neumann algebras endowed with certain natural structural properties from a physical point of view. Here one of the fundamental problems is the analysis of the connection between algebraic fields and algebraic observables, and also the problem of the description of the dynamics within the framework of this approach.
-algebras or von Neumann algebras endowed with certain natural structural properties from a physical point of view. Here one of the fundamental problems is the analysis of the connection between algebraic fields and algebraic observables, and also the problem of the description of the dynamics within the framework of this approach.
2) Constructive quantum field theory has the very important problem of proving the existence of a model of quantum field theory in 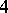 -dimensional space-time. In the two- and three-dimensional case the existence of a number of models has been proved using the Euclidean (probabilistic) approach by applying methods that are developed in analogy to statistical mechanics; here a further mathematical analysis is required of such questions as the spectrum of the Hamiltonian, phase transitions, the properties of the
-dimensional space-time. In the two- and three-dimensional case the existence of a number of models has been proved using the Euclidean (probabilistic) approach by applying methods that are developed in analogy to statistical mechanics; here a further mathematical analysis is required of such questions as the spectrum of the Hamiltonian, phase transitions, the properties of the 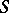 -matrix, etc. [13].
-matrix, etc. [13].
3) In formal quantum field theory, the existence of which is known in each order of perturbation theory, there are also a number of problems admitting a mathematical treatment. In this connection there are, for example, the analysis of the perturbation series (in particular, the analytic properties of Feynman diagrams), the study of classical equations of field theory and the quasi-classical corrections to them; here there arise non-linear equations of elliptic and hyperbolic types, for the analysis of which one applies in particular the inverse-scattering method, methods of differential and algebraic geometry and topology, etc.
References
| [1] | A.I. Akhiezer, V.B. Berestetskii, "Quantenelektrodynamik" , H. Deutsch , Frankfurt a.M. (1962) (Translated from Russian) |
| [2] | F.A. Berezin, "The method of second quantization" , Acad. Press (1966) (Translated from Russian) (Revised (augmented) second edition: Kluwer, 1989) |
| [3] | N.N. Bogolyubov, A.A. Logunov, I.T. Todorov, "Introduction to axiomatic quantum field theory" , Benjamin (1975) (Translated from Russian) |
| [4] | N.N. Bogolyubov, B.V. Medvedev, M.K. Polivanov, "Questions in the theory of dispersion relations" , Moscow (1958) (In Russian) |
| [5] | N.N. Bogolyubov, D.V. Shirkov, "Introduction to the theory of quantized fields" , Interscience (1959) (Translated from Russian) |
| [6] | A. Wightman, "Problems in relativistic dynamics of quantized fields" , Moscow (1968) (In Russian; translated from English) |
| [7] | A.N. Vasil'ev, "Functional methods in quantum field theory and statistics" , Leningrad (1976) (In Russian) |
| [8] | V.S. Vladimirov, "Methods of the theory of functions of several complex variables" , M.I.T. (1966) (Translated from Russian) |
| [9] | R. Jost, "The general theory of quantized fields" , Amer. Math. Soc. (1965) |
| [10] | , Constructive quantum field theory , Lect. notes in physics , 25 , Springer (1973) |
| [11] | V.N. Popov, "Functional integrals in quantum field theory and statistical physics" , Reidel (1983) (Translated from Russian) |
| [12] | M. Reed, B. Simon, "Methods of modern mathematical physics" , 1–4 , Acad. Press (1972–1978) |
| [13] | B. Simon, "The  Euclidean (quantum) field theory" , Princeton Univ. Press (1974) Euclidean (quantum) field theory" , Princeton Univ. Press (1974) |
| [14] | I. Segal, "Mathematical problems of relativistic physics" , Amer. Math. Soc. (1963) |
| [15] | A.A. Slavnov, L.D. Faddeev, "Introduction to the theory of gauge fields" , Moscow (1978) (In Russian) |
| [16] | R.F. Streater, A.S. Wightman, "PCT, Spin, statistics and all that" , Benjamin (1964) |
| [17] | F. Pham, "Introduction à l'Aeetude topologique des singularités de Landau" , Gauthier-Villars (1967) |
| [18] | K. Friedrichs, "Perturbation of spectra of operators in Hilbert space" , Amer. Math. Soc. (1965) |
| [19] | C. Hepp, "Théorie de la renormalisation" , Lect. notes in physics , 2 , Springer (1969) |
| [20] | A.S. Švarts, "Mathematical foundations of quantum field theory" , Moscow (1975) (In Russian) |
| [21] | S. Schweber, "Introduction to relativistic quantum field theory" , Harper & Row (1961) |
| [22] | , Quantum theory of gauge fields , Moscow (1977) (In Russian; translated from English) |
Comments
There is now a vast literature on the existence of specific quantum fields in two and three space-time dimensions. See [13], [a1], [a2], and [a4] for recent expositions of part of these developments and several viewpoints.
For the notation 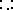 see Wick product.
see Wick product.
References
| [a1] | J. Glimm, A. Jaffe, "Quantum physics, a functional integral point of view" , Springer (1987) |
| [a2] | E. Seiler, "Gauge theories as a problem of constructive quantum field theory and statistical mechanics" , Lect. notes in physics , 159 , Springer (1982) |
| [a3] | C. Itzykson, J. Zuber, "Quantum field theory" , McGraw-Hill (1988) |
| [a4] | J.C. Baez, I.E. Segal, Z. Zhou, "Introduction to algebraic and constructive quantum field theory" , Princeton Univ. Press (1990) |
| [a5] | J.D. Ajörken, S. Drell, "Relativistic quantum mechanics" , McGraw-Hill (1964) |
| [a6] | J.D. Ajörken, S. Drell, "Relativistic quantum fields" , McGraw-Hill (1965) |
| [a7] | O.I. [O.I. Zav'yalov] Zavialov, "Renormalized quantum field theory" , Kluwer (1990) (Translated from Russian) |
| [a8] | S.S. [S.S. Khorozhii] Horuzhy, "Introduction to algebraic quantum field theory" , Kluwer (1990) (Translated from Russian) |
| [a9] | N.N. Bogolyubov, A.A. Logunov, A.I. Oksak, I.T. Todorov, "General principles of quantum field theory" , Kluwer (1990) (Translated from Russian) |
Quantum field theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quantum_field_theory&oldid=11452