Operator ergodic theorem
A general name for theorems on the limit of means along an unboundedly lengthening "time interval"  , or
, or  , for the powers
, for the powers  of a linear operator
of a linear operator  acting on a Banach space (or even on a topological vector space, see [5])
acting on a Banach space (or even on a topological vector space, see [5])  , or for a one-parameter semi-group of linear operators
, or for a one-parameter semi-group of linear operators  acting on
acting on  (cf. also Ergodic theorem). In the latter case one can also examine the limit of means along an unboundedly diminishing time interval (local ergodic theorems, see [5], [6]; one also speaks of "ergodicity at zero" , see [1]). Means can be understood in various senses in the same way as in the theory of summation of series. The most frequently used means are the Cesàro means
(cf. also Ergodic theorem). In the latter case one can also examine the limit of means along an unboundedly diminishing time interval (local ergodic theorems, see [5], [6]; one also speaks of "ergodicity at zero" , see [1]). Means can be understood in various senses in the same way as in the theory of summation of series. The most frequently used means are the Cesàro means
 |
or
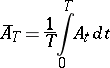 |
and the Abel means, [1],
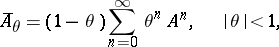 |
or
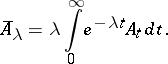 |
The conditions of ergodic theorems automatically ensure the convergence of these infinite series or integrals; under these conditions, although the Abel means are formed by using all 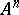 or
or 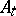 , the values of
, the values of 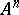 or
or 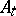 in a finite period of time, unboundedly increasing when
in a finite period of time, unboundedly increasing when 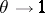 (or
(or  ), play a major part. The limit of the means (
), play a major part. The limit of the means ( , etc.) can be understood in various senses: In the strong or weak operator topology (statistical ergodic theorems, i.e. the von Neumann ergodic theorem — historically the first operator ergodic theorem — and its generalizations), in the uniform operator topology (uniform ergodic theorems, see [1], [2], [3]), while if
, etc.) can be understood in various senses: In the strong or weak operator topology (statistical ergodic theorems, i.e. the von Neumann ergodic theorem — historically the first operator ergodic theorem — and its generalizations), in the uniform operator topology (uniform ergodic theorems, see [1], [2], [3]), while if 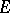 is a function space on a measure space, then also in the sense of almost-everywhere convergence of the means
is a function space on a measure space, then also in the sense of almost-everywhere convergence of the means  , etc., where
, etc., where 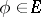 (individual ergodic theorems, i.e. the Birkhoff ergodic theorem and its generalizations; see, for example, the Ornstein–Chacon ergodic theorem; these are not always called operator ergodic theorems, however). Some operator ergodic theorems compare the force of various of the above-mentioned variants with each other, establishing that, from the existence of limits of means in one sense, it follows that limits exist in another sense [1]. Some theorems speak not of the limit of means, but of the limit of the ratios of two means (e.g. the Ornstein–Chacon theorem).
(individual ergodic theorems, i.e. the Birkhoff ergodic theorem and its generalizations; see, for example, the Ornstein–Chacon ergodic theorem; these are not always called operator ergodic theorems, however). Some operator ergodic theorems compare the force of various of the above-mentioned variants with each other, establishing that, from the existence of limits of means in one sense, it follows that limits exist in another sense [1]. Some theorems speak not of the limit of means, but of the limit of the ratios of two means (e.g. the Ornstein–Chacon theorem).
There are also operator ergodic theorems for  -parameter and even more general semi-groups.
-parameter and even more general semi-groups.
References
| [1] | E. Hille, R.S. Phillips, "Functional analysis and semi-groups" , Amer. Math. Soc. (1957) |
| [2] | N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Wiley (1988) |
| [3] | J. Neveu, "Mathematical foundations of the calculus of probabilities" , Holden-Day (1965) (Translated from French) |
| [4] | A.M. Vershik, S.A. Yuzvinskii, "Dynamical systems with invariant measure" Progress in Math. , 8 (1970) pp. 151–215 Itogi Nauk. Mat. Anal. , 967 (1969) pp. 133–187 |
| [5] | A.B. Katok, Ya.G. Sinai, A.M. Stepin, "Theory of dynamical systems and general transformation groups with invariant measure" J. Soviet Math. , 7 : 2 (1977) pp. 974–1041 Itogi Nauk. i Tekhn. Mat. Anal. , 13 (1975) pp. 129–262 |
| [6] | U. Krengel, "Recent progress in ergodic theorems" Astérisque , 50 (1977) pp. 151–192 |
| [7] | U. Krengel, "Ergodic theorems" , de Gruyter (1985) |
Operator ergodic theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Operator_ergodic_theorem&oldid=11719