Morse theory
The common name for three different theories based on ideas of M. Morse [1] and describing the relation between algebraic-topological properties of topological spaces and extremal properties of functions (functionals) on them. Morse theory is a branch of variational calculus in the large (calculus of variations in the large); however, the latter is broader: for example, it includes the theory of categories (cf. Category (in the sense of Lyusternik–Shnirel'man)).
1) Morse theory of critical points (cf. Critical point) of smooth functions 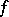 on a smooth manifold
on a smooth manifold  (briefly, Morse theory 1) is divided into two parts: local and global. The local part is related to the idea of a critical point of a smooth function, the Hessian of a function at its critical point, the Morse index of a critical point, etc. The basic result is the Morse lemma, which describes the structure of a smooth function in a neighbourhood of a non-degenerate critical point.
(briefly, Morse theory 1) is divided into two parts: local and global. The local part is related to the idea of a critical point of a smooth function, the Hessian of a function at its critical point, the Morse index of a critical point, etc. The basic result is the Morse lemma, which describes the structure of a smooth function in a neighbourhood of a non-degenerate critical point.
The study of smooth functions in neighbourhoods of degenerate points does not properly belong to Morse theory, it does rather belong to the separate theory of singularities of differentiable mappings.
The basic results in global Morse theory are as follows. Let  be a function on a smooth manifold
be a function on a smooth manifold  . If the set
. If the set 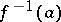 does not contain a critical point of
does not contain a critical point of  and does not intersect the boundary of
and does not intersect the boundary of  , then
, then 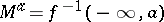 is a smooth manifold with boundary
is a smooth manifold with boundary  . If the set
. If the set  is compact, does not intersect the boundary of
is compact, does not intersect the boundary of 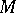 and does not contain a critical point of
and does not contain a critical point of 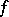 , then there is a smooth isotopy
, then there is a smooth isotopy 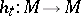 ,
,  (realized by shifting along the trajectories of the gradient of
(realized by shifting along the trajectories of the gradient of 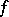 ), such that
), such that 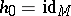 and
and 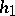 diffeomorphically maps
diffeomorphically maps  onto
onto 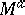 . In particular,
. In particular, 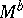 is diffeomorphic to
is diffeomorphic to 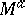 and the inclusion
and the inclusion  is a homotopy equivalence.
is a homotopy equivalence.
If  is compact, does not intersect the boundary of
is compact, does not intersect the boundary of 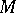 and contains precisely one critical point
and contains precisely one critical point 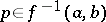 with Morse index
with Morse index 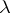 , then
, then 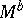 is diffeomorphic to a manifold obtained from
is diffeomorphic to a manifold obtained from 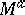 by glueing a handle of index
by glueing a handle of index  (see Morse surgery). In particular, if
(see Morse surgery). In particular, if 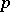 is the unique global minimum point of
is the unique global minimum point of 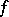 , then for small
, then for small 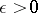 the set
the set 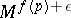 is diffeomorphic to the disc
is diffeomorphic to the disc  , where
, where  . Hence it follows that if
. Hence it follows that if 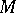 is a closed smooth manifold having a function with precisely two critical points (both non-degenerate), then
is a closed smooth manifold having a function with precisely two critical points (both non-degenerate), then 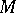 is obtained by glueing two smooth discs along their common boundary and, therefore, it is homeomorphic (but in general not diffeomorphic) to the sphere
is obtained by glueing two smooth discs along their common boundary and, therefore, it is homeomorphic (but in general not diffeomorphic) to the sphere 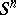 .
.
Since glueing a handle of index  is homotopically equivalent to glueing a cell of dimension
is homotopically equivalent to glueing a cell of dimension 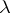 , the following fundamental theorem of Morse theory 1 follows immediately: Corresponding to each Morse function
, the following fundamental theorem of Morse theory 1 follows immediately: Corresponding to each Morse function 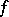 on a smooth manifold
on a smooth manifold 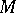 (without boundary) is a CW-complex homotopically equivalent to
(without boundary) is a CW-complex homotopically equivalent to 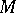 ; its cells are in bijective correspondence with the critical points of
; its cells are in bijective correspondence with the critical points of 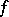 and the dimension of a cell is equal to the index of the corresponding critical point. The Morse inequalities are an immediate consequence of this theorem. An analogous theorem is valid for a Morse function on a triple
and the dimension of a cell is equal to the index of the corresponding critical point. The Morse inequalities are an immediate consequence of this theorem. An analogous theorem is valid for a Morse function on a triple 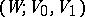 .
.
2) Morse theory of geodesics on a Riemannian manifold (briefly, Morse theory 2) describes the homotopy type of the loop space 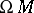 of a smooth manifold
of a smooth manifold  with a Riemannian metric
with a Riemannian metric 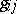 . Its aim is to transfer the results of Morse theory 1 to this space (more correctly, to a suitable model of it). The role of
. Its aim is to transfer the results of Morse theory 1 to this space (more correctly, to a suitable model of it). The role of 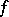 is played here by an action functional
is played here by an action functional 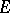 (sometimes called energy functional, [5]), defined on the space
(sometimes called energy functional, [5]), defined on the space 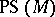 of piecewise-smooth paths
of piecewise-smooth paths  ,
, 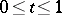 , whose value on a path
, whose value on a path 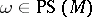 is defined, in local coordinates
is defined, in local coordinates  , by the formula
, by the formula
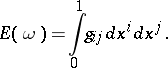 |
In the initial construction of Morse theory the length functional
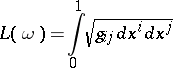 |
was considered, but for many technical reasons 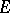 turned out to be preferable. At the same time the extremals of
turned out to be preferable. At the same time the extremals of 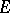 (that is, paths
(that is, paths  for which the linear functional
for which the linear functional 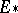 defined by the variation
defined by the variation  of
of  is zero on
is zero on  ) coincide with the geodesics of the metric
) coincide with the geodesics of the metric 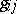 (the extremals of the functional
(the extremals of the functional 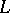 ) in their natural parametrization.
) in their natural parametrization.
Let  and
and 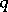 be two (not necessarily distinct) points of
be two (not necessarily distinct) points of 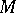 , and let
, and let 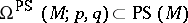 be the space of piecewise-smooth paths joining
be the space of piecewise-smooth paths joining 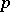 to
to 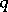 . For each
. For each  , put
, put
 |
If  is complete, then
is complete, then 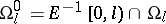 (the interior of
(the interior of  ) is a deformation retract of a smooth manifold
) is a deformation retract of a smooth manifold 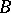 whose points are "polygonal geodesics" with a fixed number of links, joining
whose points are "polygonal geodesics" with a fixed number of links, joining 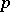 to
to 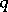 (so that, in particular,
(so that, in particular, 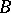 contains all geodesics from
contains all geodesics from 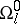 ). Here
). Here 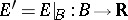 is a smooth function; for any
is a smooth function; for any 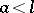 the set
the set 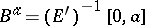 is compact and is a deformation retract of
is compact and is a deformation retract of 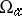 ; the critical points of
; the critical points of 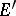 coincide with the extremals of the functional
coincide with the extremals of the functional 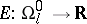 and are geodesics of length
and are geodesics of length  joining
joining 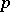 and
and 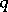 ; the Morse indices of the critical points of
; the Morse indices of the critical points of  are equal to the Morse indices of the corresponding geodesics; the null space
are equal to the Morse indices of the corresponding geodesics; the null space  of
of 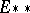 on a geodesic
on a geodesic 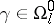 is finite dimensional and isomorphic to the null space of the Hessian of
is finite dimensional and isomorphic to the null space of the Hessian of 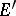 at the corresponding critical point; in particular, if
at the corresponding critical point; in particular, if  and
and  are not conjugate on any geodesic
are not conjugate on any geodesic  joining them, then
joining them, then 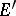 is a Morse function. Applying Morse theory 1, passing to the limit as
is a Morse function. Applying Morse theory 1, passing to the limit as 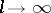 and noting that
and noting that 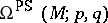 is homotopically equivalent to the space
is homotopically equivalent to the space 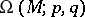 of all continuous paths joining
of all continuous paths joining 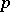 to
to 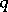 , one obtains the following fundamental theorem of Morse theory 2: Let
, one obtains the following fundamental theorem of Morse theory 2: Let 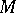 be a complete Riemannian manifold and let
be a complete Riemannian manifold and let 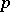 and
and  be two points not conjugate on any geodesic joining them. The space
be two points not conjugate on any geodesic joining them. The space 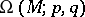 of all paths joining
of all paths joining  and
and 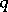 is homotopically equivalent to a CW-complex all cells of dimension
is homotopically equivalent to a CW-complex all cells of dimension 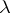 of which are in bijective correspondence with the geodesics of index
of which are in bijective correspondence with the geodesics of index 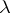 joining
joining 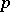 to
to 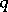 . Since the homotopy type of
. Since the homotopy type of 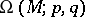 does not depend on the choice of
does not depend on the choice of 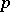 and
and  , this theorem gives, in particular, a description of the homotopy type of the loop space
, this theorem gives, in particular, a description of the homotopy type of the loop space 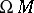 .
.
It is known
that for a non-contractible manifold 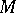 the space
the space  has non-trivial homology groups in arbitrarily high dimension. By the fundamental theorem of Morse theory 2 it follows that non-conjugate points in a complete Riemannian non-contractible manifold are joined by infinitely many geodesics (by the example of the sphere it is clear, in general, that these geodesics may be segments of one periodic geodesic).
has non-trivial homology groups in arbitrarily high dimension. By the fundamental theorem of Morse theory 2 it follows that non-conjugate points in a complete Riemannian non-contractible manifold are joined by infinitely many geodesics (by the example of the sphere it is clear, in general, that these geodesics may be segments of one periodic geodesic).
In the description of the homotopy type given by the fundamental theorem, Jacobi fields (cf. Jacobi equation and Jacobi vector field) (implicitly) appear, therefore Morse theory establishes a connection between the curvature of a manifold and its topology. For example, if 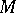 is a complete simply-connected Riemannian manifold of non-positive curvature in all two-dimensional directions, then any Jacobi field vanishing at two points of a geodesic is identically zero. Therefore the loop space
is a complete simply-connected Riemannian manifold of non-positive curvature in all two-dimensional directions, then any Jacobi field vanishing at two points of a geodesic is identically zero. Therefore the loop space 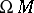 of such a manifold has the type of a zero-dimensional CW-complex, and consequently (in view of the simple connectedness of
of such a manifold has the type of a zero-dimensional CW-complex, and consequently (in view of the simple connectedness of  ) is contractible. Therefore
) is contractible. Therefore  is contractible, that is, is homotopically equivalent to
is contractible, that is, is homotopically equivalent to 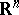 . A more precise use of Morse theory shows that
. A more precise use of Morse theory shows that  is even diffeomorphic to
is even diffeomorphic to 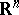 (see [3], [5]).
(see [3], [5]).
The application of Morse theory to the topology of Lie groups has turned out to be very effective [2]. For example, for any simply-connected Lie group 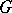 the space
the space 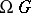 has the homotopy type of a CW-complex with only odd-dimensional cells. The apotheosis here is the Bott periodicity theorem, which plays a fundamental role in
has the homotopy type of a CW-complex with only odd-dimensional cells. The apotheosis here is the Bott periodicity theorem, which plays a fundamental role in 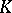 -theory and, consequently, in the whole of differential topology. Let
-theory and, consequently, in the whole of differential topology. Let 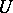 be the limit of the sequence of nested unitary groups
be the limit of the sequence of nested unitary groups 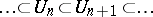 and let
and let 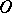 be the limit of the sequence of nested orthogonal groups
be the limit of the sequence of nested orthogonal groups 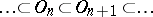 . Bott's periodicity theorem asserts that there are homotopy equivalences
. Bott's periodicity theorem asserts that there are homotopy equivalences 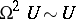 ,
, 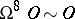 , where
, where 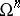 is the
is the  -th iterate of the functor of passing to the loop space. This theorem allows one to calculate the homotopy groups
-th iterate of the functor of passing to the loop space. This theorem allows one to calculate the homotopy groups  and
and 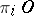 and, consequently, the homotopy groups
and, consequently, the homotopy groups 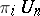 and
and  for
for 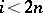 ,
, 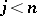 .
.
More theory 2 generalizes also to the case when instead of points 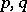 smooth submanifolds
smooth submanifolds 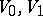 of
of 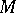 are considered. The action functional is studied on the space
are considered. The action functional is studied on the space 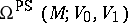 of all piecewise-smooth paths
of all piecewise-smooth paths 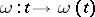 ,
,  ,
, 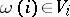 ,
, 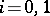 , that are transversal at the end-points to
, that are transversal at the end-points to 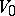 and
and 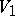 , and a relation between the extremals of this functional and the homotopy type of
, and a relation between the extremals of this functional and the homotopy type of 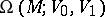 has been established. The corresponding fundamental theorem is analogous to the above-mentioned fundamental theorem of Morse theory 2; the difficulty is in the geometric interpretation of the Morse index of a geodesic.
has been established. The corresponding fundamental theorem is analogous to the above-mentioned fundamental theorem of Morse theory 2; the difficulty is in the geometric interpretation of the Morse index of a geodesic.
3) The natural development of Morse theory 2 is Morse theory for critical points of smooth functions on Banach (infinite-dimensional) manifolds — Morse theory 3, which is no longer an analogue, but a direct generalization of Morse theory 1. At present (1989) Morse theory 3 is at an initial stage and has been constructed only in a very preliminary context under very strong (and clearly not necessary) conditions on the model Banach space (on separable- and Hilbert-type spaces), when no specifically functional-analytic difficulties arise [9], although there have been attempts at a construction of Morse theory 3 in fairly general situations. Therefore, in its modern form, Morse theory 3 is an almost verbatim re-iteration of Morse theory 1. The only difference worth mentioning is that in Morse theory 3 the compactness of 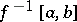 is replaced by condition
is replaced by condition 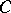 of Palais–Smale (see Morse function), which, besides, is not satisfied in all situations of interest. In addition, although it is possible to glue to a Banach manifold a handle of infinite index, in view of the homotopic triviality of infinite-dimensional spheres this handle has no effect on the homotopy type. Therefore only critical points of finite index occur in the fundamental theorem of Morse theory 3.
of Palais–Smale (see Morse function), which, besides, is not satisfied in all situations of interest. In addition, although it is possible to glue to a Banach manifold a handle of infinite index, in view of the homotopic triviality of infinite-dimensional spheres this handle has no effect on the homotopy type. Therefore only critical points of finite index occur in the fundamental theorem of Morse theory 3.
References
| [1] | M. Morse, "The calculus of variations in the large" , Amer. Math. Soc. (1934) |
| [2] | J.W. Milnor, "Morse theory" , Princeton Univ. Press (1963) |
| [3] | J. Milnor, "Lectures on the 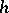 -cobordism theorem" , Princeton Univ. Press (1965) -cobordism theorem" , Princeton Univ. Press (1965) |
| [4] | H. Seifert, W. Threlfall, "Variationsrechnung im Groszen (Morsesche Theorie)" , Teubner (1938) |
| [5] | D. Gromoll, W. Klingenberg, W. Meyer, "Riemannsche Geometrie im Grossen" , Springer (1968) |
| [6] | R.L. Bishop, R.J. Crittenden, "Geometry of manifolds" , Acad. Press (1964) |
| [7] | M.M. Postnikov, "Introduction to Morse theory" , Moscow (1971) (In Russian) |
| [8] | M.M. Postnikov, "The variational theory of geodesics" , Saunders (1967) (Translated from Russian) |
| [9] | J. Eells, "A setting for global analysis" Bull. Amer. Math. Soc. , 72 (1966) pp. 751–807 |
| [10a] | J.-P. Serre, "Homologie singulière des espaces fibrés I" C.R. Acad. Sci. Paris , 231 (1950) pp. 1408–1410 |
| [10b] | J.-P. Serre, "Homologie singulière des espaces fibrés. Applications" Ann. of Math. (2) , 54 (1951) pp. 425–505 |
| [10c] | J.-P. Serre, "Homologie singulière des espaces fibrés II" C.R. Acad. Sci. Paris , 232 (1951) pp. 31–33 |
| [10d] | J.-P. Serre, "Homologie singulière des espaces fibrés III" C.R. Acad. Sci. Paris , 232 (1951) pp. 142–144 |
Comments
A useful survey of Morse theory is [a1]; historical remarks can be found in [a2] and [a3], Sect. 1.7.
There is an analogue (generalization) of (finite-dimensional) smooth Morse theory for suitable spaces with singularities, called stratified Morse theory. Let 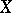 be a compact Whitney-stratified space contained in a smooth manifold
be a compact Whitney-stratified space contained in a smooth manifold 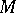 (cf. (the editorial comments to) Stratification). Let
(cf. (the editorial comments to) Stratification). Let  be the restriction to
be the restriction to 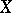 of a smooth real-valued function on
of a smooth real-valued function on  . A critical point of
. A critical point of 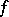 is any critical point of
is any critical point of 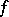 restricted to a stratum of
restricted to a stratum of  . In particular, all the zero-dimensional strata are critical points. The proper smooth function
. In particular, all the zero-dimensional strata are critical points. The proper smooth function 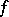 is called a Morse function on the stratified space
is called a Morse function on the stratified space  if:
if:
a) all critical values of 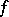 are distinct;
are distinct;
b) at each critical point 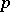 of
of 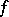 , the restriction of
, the restriction of 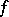 to the stratum containing
to the stratum containing 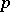 has a non-degenerate critical point at
has a non-degenerate critical point at 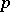 ;
;
c) the differential of 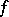 at a critical point
at a critical point  does not annihilate any limit of tangent spaces to any stratum
does not annihilate any limit of tangent spaces to any stratum 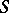 other than the stratum containing
other than the stratum containing 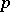 .
.
It follows that the set of critical points is discrete in 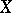 and that the critical values are discrete in
and that the critical values are discrete in 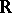 . If
. If 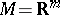 , the distance function on
, the distance function on 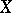 from a point
from a point 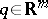 is a Morse function for almost-all
is a Morse function for almost-all 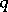 . The Morse functions also form an open dense set in the space of all proper smooth functions with the appropriate topology.
. The Morse functions also form an open dense set in the space of all proper smooth functions with the appropriate topology.
For each 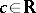 , let
, let  . Then (for a Morse function
. Then (for a Morse function 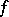 on
on 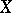 ) one has the following analogue of smooth finite-dimensional Morse theory. As
) one has the following analogue of smooth finite-dimensional Morse theory. As  varies in the open interval between two adjacent critical values, the topological type of
varies in the open interval between two adjacent critical values, the topological type of 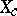 does not vary, and as
does not vary, and as  crosses a critical value
crosses a critical value 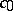 (from below), the topological type of
(from below), the topological type of  , with
, with  sufficiently small, is obtained from that of
sufficiently small, is obtained from that of 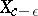 by glueing in a suitable (stratified) space
by glueing in a suitable (stratified) space 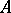 along a subspace
along a subspace 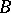 . The major difference is that the pair
. The major difference is that the pair 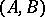 can be far more complicated than the pair
can be far more complicated than the pair 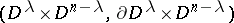 , where
, where 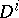 is the
is the 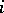 -dimensional solid ball, of the smooth theory. Also, the pair
-dimensional solid ball, of the smooth theory. Also, the pair 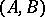 is not determined by a single integer. Intersection homology plays an analogous role vis à vis stratified Morse theory as ordinary homology does with respect to the smooth theory, in that if
is not determined by a single integer. Intersection homology plays an analogous role vis à vis stratified Morse theory as ordinary homology does with respect to the smooth theory, in that if 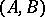 is the pair belonging to the critical point
is the pair belonging to the critical point  , then the intersection homology group
, then the intersection homology group 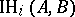 vanishes for all
vanishes for all  except
except 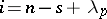 , where
, where 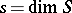 ,
, 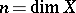 and
and 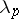 is the Morse index of
is the Morse index of 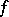 restricted to
restricted to 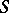 at
at 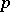 .
.
There are two other important generalizations of ordinary finite-dimensional Morse theory:
The non-isolated case.
This applies to functions with non-degenerate critical manifolds. One assumes that 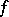 restricted to the normal direction is non-degenerate. Cf. [a5].
restricted to the normal direction is non-degenerate. Cf. [a5].
The equivariant case.
This applies to functions which are equivariant under the action of a Lie group. Cf. [a1]. There are applications e.g. to Yang–Mills theory (cf. Yang–Mills field) in 2 dimensions. Cf. [a4].
References
| [a1] | R. Bott, "Lectures on Morse theory, old and new" Bull. Amer. Math. Soc. , 7 : 2 (1982) pp. 331–358 |
| [a2] | R. Bott, "Marston Morse and his mathematical works" Bull. Amer. Math. Soc. , 3 : 3 (1980) pp. 907–950 |
| [a3] | M. Goreski, R. MacPherson, "Stratified Morse theory" , Springer (1988) |
| [a4] | M. Atiyah, R. Bott, "The Yang–Mills equations over Riemann surfaces" Phil. Trans. R. Soc. London A , 308 (1982) pp. 523–615 |
| [a5] | R. Bott, "Non-degenerate critical manifolds" Ann. of Math. (2) , 60 (1954) pp. 248–261 |
| [a6] | W. Klingenberg, "Lectures on closed geodesics" , Springer (1978) |
| [a7] | S. Smale, "Morse theory and a non-linear generalization of the Dirichlet problem" Ann. of Math. , 80 (1964) pp. 382–346 |
| [a8] | W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German) |
Morse theory. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Morse_theory&oldid=15676